Some Properties of Degenerate r-Dowling Polynomials and Numbers of the Second Kind

Hye Kyung Kimand Dae Sik Lee

1Department of Mathematics Education,Daegu Catholic University,Gyeongsan,38430,Korea

2School of Electronic and Electric Engineering,Daegu University,Gyeongsan,38453,Korea

ABSTRACT The generating functions of special numbers and polynomials have various applications in many fields as well as mathematics and physics.In recent years, some mathematicians have studied degenerate version of them and obtained many interesting results.With this in mind,in this paper,we introduce the degenerate r-Dowling polynomials and numbers associated with the degenerate r-Whitney numbers of the second kind.We derive many interesting properties and identities for them including generating functions,Dobinski-like formula,integral representations,recurrence relations,differential equation and various explicit expressions.In addition,we explore some expressions for them that can be derived from repeated applications of certain operators to the exponential functions,the derivatives of them and some identities involving them.

KEYWORDS Dowling lattice; Whitney numbers and polynomials; r-Whitney numbers and polynomials of the second kind;r-Bell polynomials;r-Stirling numbers;dowling numbers and polynomials

1 Introduction

The Stirling numberS2(n,k)of the second kind counts the number of partitions of the set{1,2,...,n} intok-nonempty disjoint set.The Bell polynomialsBn(x)are given by

Whenx=1,Bn=Bn(1)are called the Bell numbers.The Stirling numberS1(n,k)of the first kind counts the number of having permutations of the set{1,2,...,n}havingkdisjoint cycles.

Dowling [2] constructed a certain lattice for a finite group of orderm, called Dowling lattice,and using the Möbius function,he introduced the corresponding Whitney numbers of the first kindwm(n,k)and Whitney numbers of the second kindWm(n,k)(0 ≤k≤n,m≥1),which are independent of the group itself,but depend only on its order.For the trivial group,we havew1(n,k)=S1(n+1,k+1)andW1(n,k)=S2(n+1,k+1).Benoumhani[3,4]gave a detailed description of properties of these numbers.

Forx∈ R, the falling factorials(x)nare given by(x)n=x(x- 1)···(x-n+ 1),(n≥1)and(x)0=1,(see[1]).

As a generalization of the the Whitney numberswm(n,k)andWm(n,k)of the first and second kind associated withQn(G),respectively,Mezö[5]introducedr-Whitey numbers of the first and second kind given by

respectively,forn≥k≥0.Andwm,r(0,0)=1 andWm,r(0,0)=1.

Whenr=1,wm(n,k)=wm,1(n,k)andWm(n,k)=Wm,1(n,k).

We note that

Note that ther-Whitney numbers of the second kind are exactly the same numbers defined by Ruciński and Voigt et al.[6]and the(r,β)-Stirling numbers defined by Corcino et al.[7].

Ther-Whitey numbers of both kinds andr-Dowling polynomials were studied by several authors.The references [2-5,7-15] provided readers more information.In particular, Cheon et al.[8] and Corcino et al.[11]gave combinatorial interpretations of ther-Whitney numbers of the first and second kind, respectively.In recently years, many mathematicians have been studied the degenerate special polynomials and numbers, and have obtained many interesting results [14,16-24].In particular, the generating functions of (degenerate) special numbers and polynomials have various applications in many fields as well as mathematics and physics [1-32].Kim et al.[14] introduced the degenerate Whitney numbers of the first kind and the second kind of Dowling latticeQn(G)of ranknover a finite groupGof orderm,respectively,as follows:

With these in mind,we naturally introduce the degenerater-Dowling polynomials and numbers associated with the degenerater-Whitney numbersWm,r(n,k)of the second kind in this paper.We explore various properties and identities for the degenerater-Dowling polynomials and numbers including generating functions,Dobinski-like formula,integral representations,recurrence relations,various explicit expressions.Furthermore, we investigate several expressions for them that can be derived from repeated applications of certain operators to the exponential functions, the derivatives of them and some identities involving them.

2 Preliminaries

In this section, we introduce the basic definitions and properties of the degenerater-Dowling polynomials and numbers needed in this paper.

Forx∈ R, the rising factorials 〈x〉nare given by 〈x〉n=x(x+ 1)···(x+n- 1),(n≥1)and 〈x〉0=1,(see[1]).

Cheon et al.[8] introduced ther-Dowling polynomials associated with ther-Whitney numbersWm,r(n,k)of the second kind are given by

By(1)and(4),the generating function ofr-Dowling polynomials is given by

where exp(t)=et.

Corcino et al.[11]studied asymptotic formulas forr-Whitney numbers of the second kind with integer and real parameters.They also obtained the range of validity of each formula.

As is well known,for anyλ∈R,

where(x)n,λ=x(x-λ)···(x-(n-1)λ))(n≥1)and(x)0,λ=1.Whenλ→0,exλ(t)=ext.

The degenerate Stirling numbers of the second kind are given by

Kim et al.studied the unsigned degenerater-Stirling numbers of the second kind defined by

From(7),the generating function of the degenerater-Stirling numbers of the second kind is given by

wherejis a non-negative integer.

In view of(8),the degenerater-Bell polynomials are given by

From(9),it is easy to show that the generating function of degenerater-Bell polynomials is given by

whenx=1,Beln(r)(λ)=Beln(r)(1|λ)are called the degenerater-Bell numbers.

Kim et al.introduced theλ-binomial coefficients defined as

From(11),we easily get

From(12),we note that

3 Degenerate r-Dowling Polynomials and Numbers

In this section, we explore various properties for the degenerater-Dowling polynomials and numbers.

From(1),the degenerater-Whitney numbers,k)of the second kind are given by

Lemma 3.1.[14]Fork≥0,we have the generating function of the degenerater-Whitney numbers of the second kind as follows:

In Lemma 3.1,whenr= 1,we have the generating function of the degenerate Whitney numbers of the second kind as follows:

From Lemma 3.1,(6)and(8),we get

The next theorem is a recurrence relation of the degenerate Whitney numbers of the second kind.

Theorem 3.1.Forn≥0,we have

Wm,r,λ(n+1,j)=Wm,r,λ(n,j-1)+(mj+r-nλ)Wm,r,λ(n,j).

Proof.From(14),we observe that

By comparing the coefficients of both sides of(16),we get the desired recurrence relation.

The following theorem shows that the degenerater-Whitney numbers of second kind expresses the finite sum of degenerate falling factorials.

Theorem 3.2.Forn,j≥0,we have

Proof.By(5)and Lemma 3.1,we observe that

By comparing the coefficients of both sides of(17),we get the desired result.

In Theorem 3.2,whenr=1,forn≥k≥0,we get

In this paper, we naturally consider the degenerater-Dowling polynomials of the second kind given by

Whenx= 1,Dm,r,λ(n)=Dm,r,λ(n|1)are called the degenerater-Dowling numbers of the second kind.

Whenr= 1,Dm,λ(n,x)=Dm,1,λ(n|x)are the degenerate Dowling polynomials in of the second kind[14].

Whenr=1,the degenerater-Dowling polynomials of the second kind are different from the fully degenerate Dowling polynomials in[23].

Theorem 3.3.Form∈N, the generating function of degenerater-Dowling polynomials of the second kind is

Proof.From Lemma 3.1 and(18),we observe that

By(19),we have the generating function of degenerater-Dowling polynomials of the second kind.

Whenm=1,from Theorem 3.3,(10)and(15),we observe that

Whenm=1,r=1 andλ→0,we note that

Theorem 3.4.(Dobinski-like formula)

Forn≥0,we have

Whenr=1,we have

Proof.From(5)and Theorem 3.3,we note that

By comparing the coefficients of both sides of (20), we have Dobinski-like formula for the degenerater-Dowling polynomials.

In the following theorem and corollary, we have integral representations of the degenerater-Whitney numbers and the degenerater-Dowling polynomials,respectively.

Theorem 3.5.Forn,l∈Z withn≥l≥0,we have

Proof.From Lemma 3.1,we get

Therefore,by(21)we have the desired result.

Corollary 3.1.Forn≥0,we have

Proof.By Lemma 3.1 and Theorem 3.5,we have

From(22),we get the desired identity.

Lemma 3.2.Forn≥j≥0 andr,m∈N,we have

Proof.From Theorem 3.2 and(13),we get

By(23),we obtain the desired result.

The next theorem is a recurrence relation of degenerater-Dowling polynomials.

Theorem 3.6.Forn≥0,we have

Proof.From(18)and Lemma 3.2,we have

HereWm,r,λ(j,d)=0,ifd≥j.Thus,by(24),we get what we want.

Theorem 3.7.Forn≥j≥0,we have the recursion formula forWm,r,λ(n,j)as follows:

Proof.Forj≥1,from(5)and Lemma 3.1,we observe that

By comparing the coefficients of both sides of(25),we get what we want.

The following theorem is another recurrence relation of degenerater-Dowling polynomials.

Theorem 3.8.Forn≥0,we have the recurrence formula ofDm,r,λas follows:

Proof.From Theorem 3.3,we note that

On the other hand,by(26),we get

By comparing the coefficients of(26)with(27),we get the desired identity.

Remark.Whenu=1,we have

Next,we explore two identities including degenerater-Dowling polynomials that can be derived from repeated applications of certain operators to the degenerate exponential functions.

Theorem 3.9.Forn≥0,we have

Proof.First,we observe that

By(29)and Theorem 3.4,

From(30),we have what we want.

By Theorem 3.3,we have

From(31),the generating function ofAn,λis

Theorem 3.10.Forn≥0,we have

Proof.Letemλ(t)=u,Then we have

By(33),we get

By(34),we attain the desired result.

Remark.Whenx=1,we have

In Theorem 3.1,whenu=1 we observe that

From(35),we obtain

In(35),whenn=1,we get

In(35),whenn=2,we observe that

From(37),we get

Thus,by(38),we haveDm,r,λ(2)=m+(r+1)(r-λ+1).

In the same way,we get

By continuous this process,we get all ther-Dowling numbersDm,r,λ(n),forn∈N.

As you can see from(39),the largern,the more difficult it is to calculate by hand.Here we use Mathematica and Fortran language to find these values.

In Fig.1, whenm= 5, we can see the change ofD5,r,0.1(2)andD5,r,0.5(2)depending onrby using Mathematica(x-axis is the numbers ofr,y-axisD(2)is the numbers ofD5,r,0.1(2)andD5,r,0.5(2),respectively).

In Fig.2, whenm= 5, we can see the change ofD5,r,0.1(3)andD5,r,0.5(3)depending onrby using Mathematica(x-axis is the numbers ofr,y-axisD(3)is the numbers ofD5,r,0.1(3)andD5,r,0.5(3),respectively).

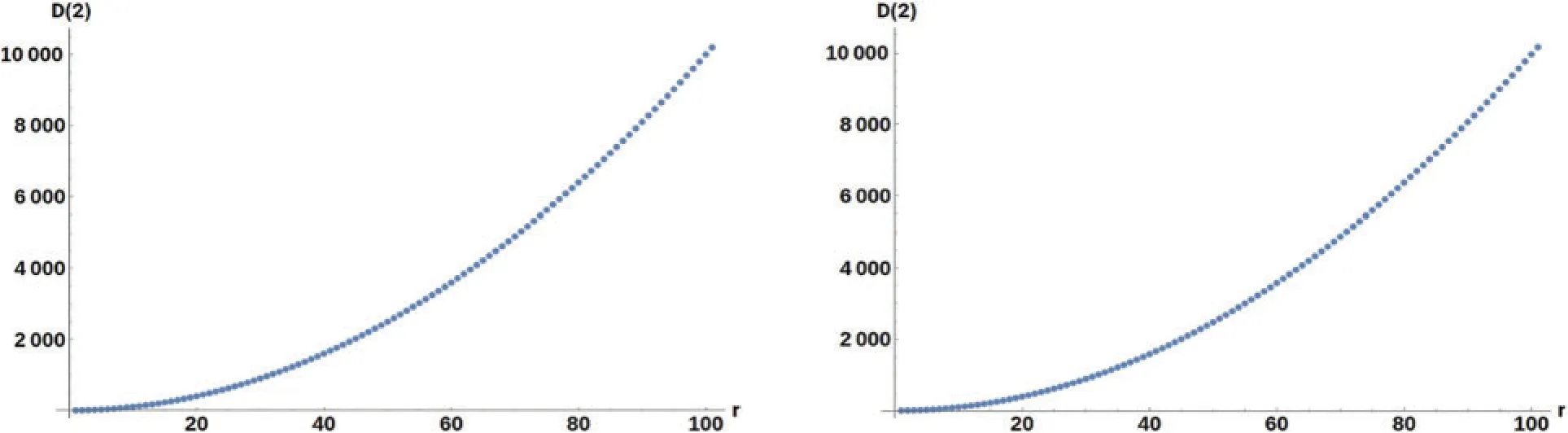
Figure 1:D(2)=D5,r,λ(2),when λ=0.1 and 0.5,respectively

Figure 2:D(3)=D5,r,λ(3),when λ=0.1 and 0.5,respectively

In Fig.3,whenλ= 0.1,we can see the change ofD10,1,0.1(n)andD50,1,0.1(n),respectively,by using Fortran language (x-axis is the numbers ofn,y-axis log10(D(n))is the value of log10(numbers ofD10,1,0.1(n)andD50,1,0.1(n),respectively).

In Fig.4,whenλ= 0.5,we can see the change ofD10,1,0.5(n)andD50,1,0.5(n),respectively,by using Fortran language (x-axis is the numbers ofn,y-axis log10(D(n))is the value of log10(numbers ofD10,1,0.5(n)andD50,1,0.5(n)),respectively).

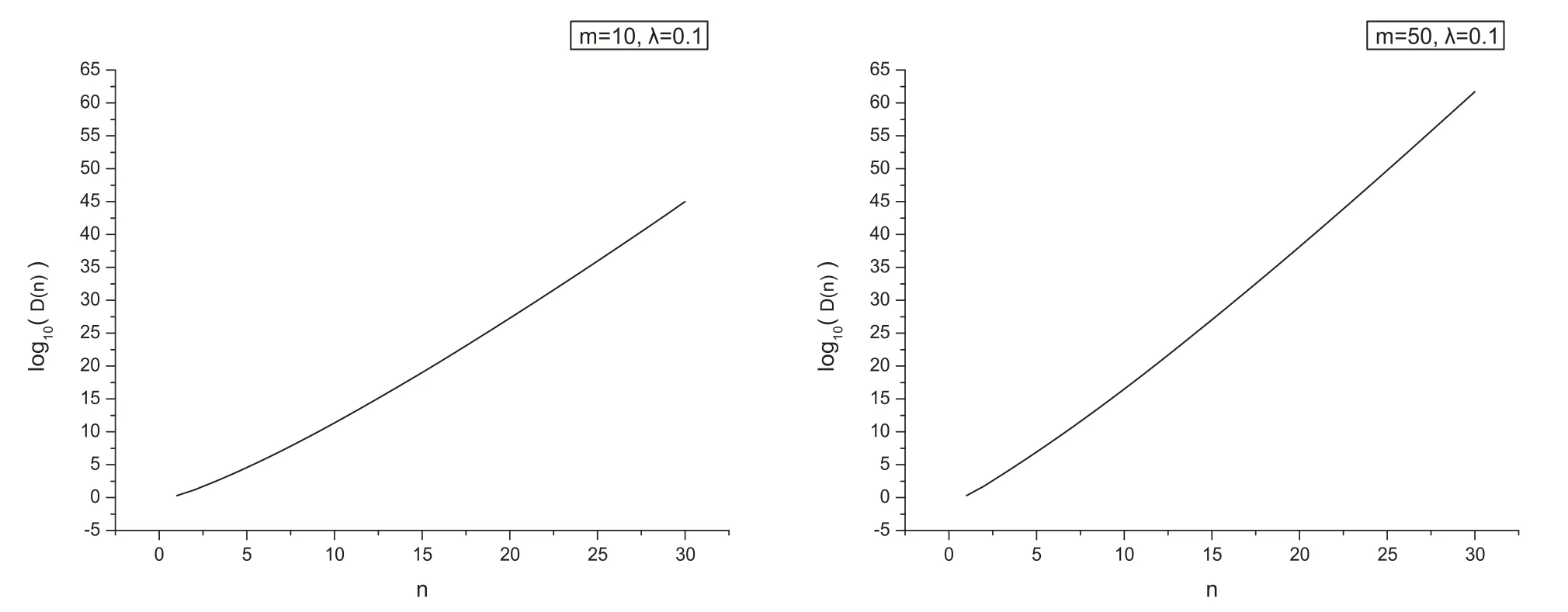
Figure 3:log10(D(n))=log10(Dm,1,0.1(n))when m=10 and 50,respectively

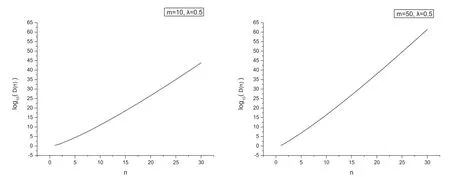
Figure 4:log10(D(n))=log10(Dm,1,0.5(n))when m=10 and 50,respectively

Next,we can get differential equation for degenerater-Dowling polynomials as follows:

Theorem 3.11.Forn≥0,we have

Proof.By using Theorem 3.4,we observe

On the other hand,we have

By(40)and(41),we have

From(42),we get

By(43),we obtain the desire result.

Now,we study the derivative of degenerater-Dowling polynomialsDm,r,λ(n|x).

Theorem 3.12.Forn≥1,we have

Proof.From(5)and Theorem 3.3,we observe that

By comparing the coefficients on both sides of(44),we attain the desired identity.

Theorem 3.13.Forn≥1,we have

Proof.From(5)and Theorem 3.3,we have

From(45),we observe that

By(46),we have

From(47),we obtain

By comparing the coefficients of both sides of(45),we have the desired identity.

If we puty=uq (q∈N ∪{0})and apply the next theorem, we get another interesting identity depending on the variableqdifferent from Theorem 3.10.

Theorem 3.14.Forn≥0,we have the operational formula as follows:

Proof.Lety=uq (q∈N ∪{0}).Then we have

Thus,by(49),we have

From(50),we attain the desired formula.

4 Conclusion

In this paper, we studied many interesting properties for the degenerater-Dowling polynomials and numbers associated with the degenerater-Whitney numbers of the second kind.Among these identity expressions, we obtained the generating function in Theorem 3.3, Dobinski-like formula in Theorem 3.4,recurrence relations in Theorem 3.6 and 3.8,differential equation in Theorem 3.11,the derivatives of them in Theorem 3.12 forr-Dowling polynomials of the second kind.In particular,we obtained some expressions for them that can be derived from repeated applications of certain operators to the exponential functions in Theorem 3.9,3.10 and 3.14,and some identities involving integration in Theorem 3.13.Furthermore, we found that all exact values of allr-Dowling numbers of the second kind can be obtained using (28).As a follow-up study of this paper, we can explore truncated degenerater-Dowling polynomials and degenerater-Dowling polynomials arising fromλ-Sheffer sequences.Hence,for future projects,we would like to conduct research into some potential applications ofr-Dowling polynomials of the first and second kind,respectively.

Acknowledgement:The author would like to thank the referees for the detailed and valuable comments that helped improve the original manuscript in its present form.Also, the authors thank Jangjeon Institute for Mathematical Science for the support of this research.

Authors’ Contributions:HKK structured and wrote the whole paper.DSL performed computer simulations in the paper.All authors checked the results of the paper and completed the revision of the article.

Consent for Publication:The authors want to publish this paper in this journal.

Ethics Approval and Consent to Participate:The authors declare that there is no ethical problem in the production of this paper.

Funding Statement:This work was supported by the Basic Science Research Program, the National Research Foundation of Korea(NRF-2021R1F1A1050151).

Conflicts of Interest:The authors declare that they have no conflicts of interest to report regarding the present study.