Negative tunnel magnetoresistance in a quantum dot induced by interplay of a Majorana fermion and thermal-driven ferromagnetic leads∗
Peng-Bin Niu(牛鹏斌),Bo-Xiang Cui(崔博翔),and Hong-Gang Luo(罗洪刚)
1Department of Physics,Shanxi Datong University,Datong 037009,China
2School of Physical Science and Technology,Lanzhou University,Lanzhou 730000,China
3Beijing Computational Science Research Center,Beijing 100084,China
Keywords:Majorana fermion,spin current,tunnel magnetoresistance,thermal-bias
1.Introduction
The central theme of spintronics is to manipulate spin degrees of freedom in solid-state systems,to understand the interaction between the particle spin and its solid-state environments and to make useful devices.[1,2]In spintronics,generation of spin-polarized currents usually uses ferromagnetic electrodes[3–6]or central system’s spin polarization.[7,8]The ferromagnetic electrodes have parallel or antiparallel configurations.With these configurations,the physical quantities can be measured by spin current,charge current or tunnel magnetoresistance(TMR).[9–11]Usually,the value of TMR is positive due to the charge current in parallel configuration is larger than the current in antiparallel configuration.[10]However,as will be seen below,the TMR can be negative when Majorana fermion is introduced.
Majorana fermions,with antiparticles being themselves,have been searched in high-energy physics for a long time.[12–14]The Majorana pair possesses topological properties,and in condensed matter physics it has been shown that the topology can be realized in many materials,such as topological insulators,[15,16]Bose–Einstein condensates,[17–19]Kagome lattice,[20,21]and silicene.[22]Recently,searches for Majorana fermions in condensed matter physics have attracted much attention,theoretically or experimentally,[23–30]due to the fact that they have non-Abelian statistics[31,32]and are promising in quantum computation.The predicted Majorana bound states,or Majorana zero modes correspond to the Majorana fermions in high-energy physics.They are coherent superpositions of hole and electron excitations of zero energy,which can be realized at the two ends of a topological superconductor wire.[33,34]In quantum transport,the readout and manipulation of Majorana zero mode in quantum dot-Majorana hybrid systems[35–39]have been extensively studied and many interesting results are found.For example,the presence of Majorana zero mode leads to a quantized 1/2 zero-bias conductance[35]G=2e2/h,when the dot is on resonance and symmetrically coupled to the probing leads.
Motivated by these achievements,in this paper we consider temperature-driven spin-polarized quantum transport in a quantum dot-Majorana hybrid system connected with ferromagnetic electrodes.The polarization are generated from two factors:the central system’s spin polarization induced by Majorana zero mode and the ferromagnetic electrodes’spin polarization.It is shown that the interplay of Majorana fermion and electrodes’spin polarization leads to some interesting results.For instance,we find that the interplay can induce nonlinear thermal-driven spin current.A thermal-driven negative tunnel magnetoresistance is also found.With large temperature difference and strong coupling between quantum dot and topological superconductor,the relationship between spin-up current and gate voltage becomes linear,revealing Majorana fermion’s robustness.
This paper is organized as follows.In Section 2 we describe the model of quantum dot coupled with Majorana fermion zero mode.Specific Hamiltonian,basic formula for currents and TMR are presented there.In Section 3 we present the numerical results and explain the behaviors of spin-related currents and TMR.Finally,Section 4 is devoted to a brief conclusion.
2.Model and method
We consider a system shown in Fig.1.It consists of a spin-degenerate quantum dot(QD)side-coupled with a topological superconductor wire,which supports two Majorana fermion zero modes.It is assumed that the length of the topological superconductor wire is larger than the superconducting coherence length,so that the overlap and interaction between the pair of two Majorana zero modes are neglected.[40]The QD is also coupled to two thermal-driven ferromagnetic leads.The Hamiltonian can be written as[35–40]
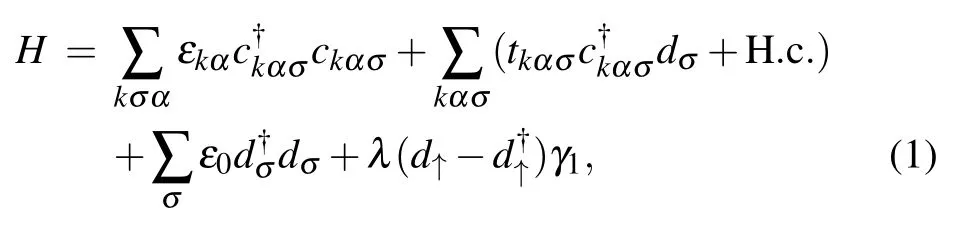
The standard equation of motion method yields the exact expression for the spin-up Green’s function[35]

For spin-down Green’s function,it is easy to obtain
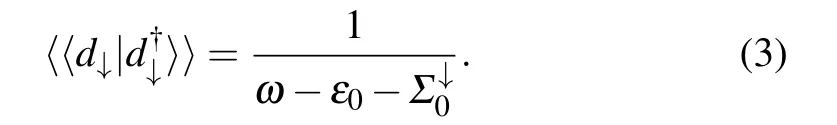
Within the non-equilibrium Green’s function formalism,the transport current is expressed as[41,42]
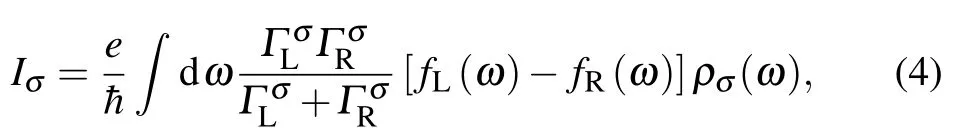

Fig.1.Schematic depiction of the model under consideration.The system is placed between two ferromagnetic electrodes with spindependent couplingΓσα.It consists of a quantum dot and a topological superconductor which supports Majorana fermion zero modes.One of the quantum dot’s spins is side-coupled with a Majorana zero mode(MZM)(see Hamiltonian in the text).The electrodes is applied with a temperature difference.
The spin-polarized transport properties,especially the tunnel magnetoresistance(TMR)effect,have been proven to be useful in information-storage devices.TMR is quantitatively characterized as[10]

3.Results and discussion
In the following we investigate the transport properties induced by the interplay of Majorana fermion and electrodes’spin polarization.Based on the formulas of currents and TMR,the numerical results are provided.
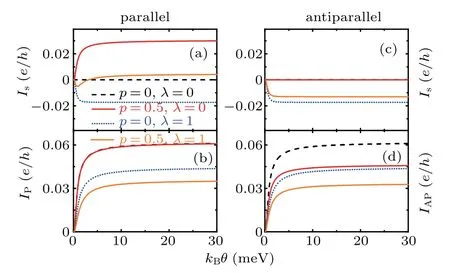
Fig.2.Spin current and charge current as a function of temperature difference for parallel configuration[(a),(b)]and antiparallel configuration[(c),(d)].The spin polarization and QD-MZM coupling strength in(a)–(d)are kept to be unchanged.In comparison,the curves for p=0 are also presented(dashed and dotted lines).The other parameters are ε0=1,Γ=0.02.The background temperature is chosen as kBT=0.01.All energies are in units of meV.
Firstly,in Fig.2 we consider the spin current Isand charge current IP(IAP)for parallel(antiparallel)configuration,as a function of the temperature differenceθ.We start first to analyze the behaviors of spin current with electrodes’parallel configuration in Fig.2(a).When p=0 andλ=0,i.e.,when there are no spin polarization and Majorana fermion,the magnitudes of spin-up and spin-down currents are equal,hence the spin current is zero.When the QD–MZM coupling appears(p=0 andλ=1),one can observe a negative thermal-driven spin current.This is because the appearance of Majorana fermion decreases spin-up current and induces a negative spin current.However,when p/=0 andλ=0,one can observe a positive spin current,because the majority spin-up electrons can flow through the QD with parallel configuration.Thus the interplay of Majorana fermion and spin polarization induces a nonlinear spin current,which decreases first and then increases,as seen in Fig.2(a)with p=0.5 andλ=1.Although ferromagnetic leads’spin polarization induces spin-up majority and spin-down minority,itself alone does not affect the total magnitude of the charge current.Thus in Fig.2(b)the two curves(p=0,λ=0 and p=0.5,λ=0)overlap.As shown in Fig.2(a),the QD–MZM coupling alone will decrease charge current,as seen in Fig.2(b)for p=0,λ=1.However,the union(or interplay)of Majorana fermion and spin polarization will further decrease the charge current,as seen in Fig.2(b)for p=0.5,λ=1.In the meantime,one can see from Fig.2(d)that the charge current also has the minimal magnitude for p=0.5,λ=1.Therefore,we can conclude that the interplay decreases the total magnitude of the charge current,in either parallel or antiparallel configuration.We then analyze the behaviors of currents with antiparallel configuration.The antiparallel configuration alone does not induce spin current,instead it decreases the total magnitude of the charge current,as seen in Figs.2(c)and 2(d).The QD–MZM coupling alone will decrease spin-up current,and generate negative spin current.However,the interplay of Majorana fermion and spin polarization will further decrease the spin current,as seen in Fig.2(c).
From Fig.2 one can see a characteristic of the thermaldriven currents:with large enough temperature difference,the currents tend towards stability,i.e.,thermal bias will not affect the currents anymore,and in this situation the currents will be sensitive to gate voltage(orε0).Hence in Fig.3 we discuss the currents with varying gate voltage for fixed large temperature difference.In Fig.3 we focus on the behaviors of spin-up current I↑(in which Majorana fermion appears and exhibit an interesting result,as will be shown below)and spin current Is.
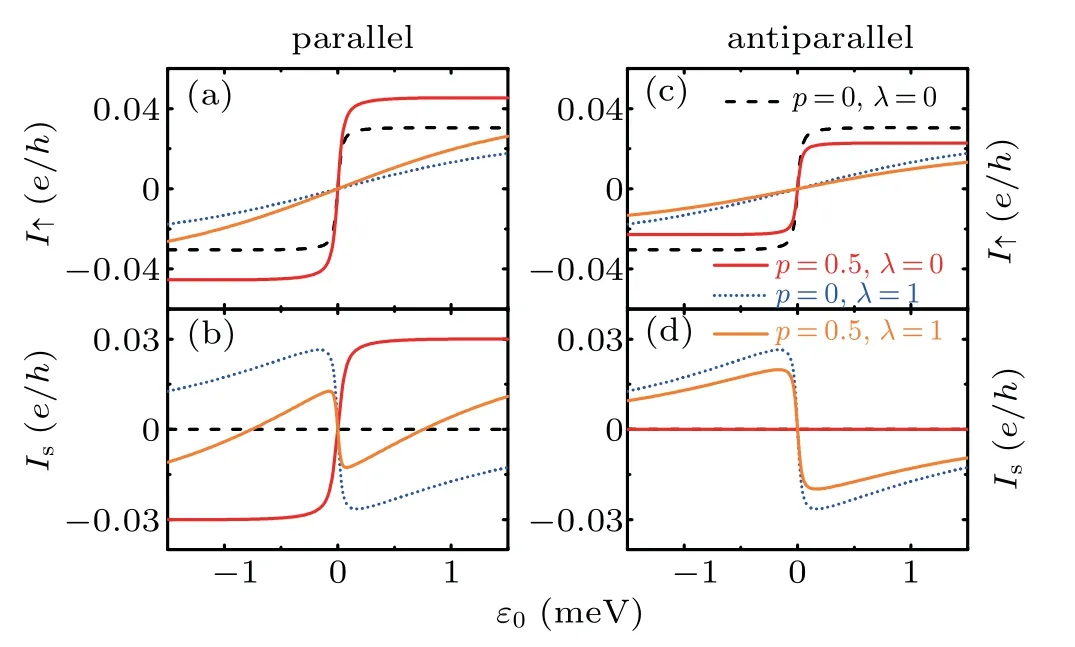
Fig.3.Spin-up current I↑and spin current Is as a function of energy levelε0 for parallel configuration[(a),(b)]and antiparallel configuration[(c),(d)].The spin polarization and QD–MZM coupling strength in(a)–(d)are kept to be constant.In comparison,the curves for p=0 are also presented(dashed and dotted lines).The other parameters areθ=30,Γ=0.02.The background temperature is chosen as kBT=0.01.All energies are in units of meV.
Let us discuss the spin-resolved current first.In Figs.3(a)and 3(c),for the curves ofλ=0,one sees a step located at ε0=0,where the gate tunable energy levelε0passes through the Fermi surface.Whenε0<0,there is a heat-generated current flowing from right lead to left(which is negative).When ε0>0,there is a positive current flowing from left to right.It should be pointed out that in bias-driven quantum transport the phenomenon of step in current is also observed,where the gate voltage is fixed.Here the situation is opposite.An interesting result found here is that whenλ=1,the step disappears and the functional relation between spin-up current and energy level becomes linear.This reveals Majorana fermion’s robustness.Due to this linear relationship,i.e.,Majorana fermion’s robustness,the spin currents forλ=1 show an antisymmetric oscillating structure,as seen in Figs.3(b)and 3(d).The union of Majorana fermion and spin polarization decreases the magnitude of current,which is verified again in Figs.3(b)and 3(d).Interestingly,the spin current has three zero points for parallel configuration,while in the case of antiparallel configuration or zero spin polarization,the spin current has only one zero point.
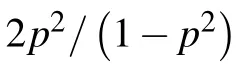
In Fig.5 the TMR varying with energy levelε0is presented.In Fig.5(a)the TMR without Majorana fermion is shown.The TMR changes intensely aroundε0=0,when it passes through the Fermi level.The whole curve of TMR is positive,because IP>IAP.In Fig.5(b)the TMR with QDMZM coupling and spin polarization is plotted.It is seen that the gated-tuned TMR with fixed large temperature difference exhibits a symmetric structure,and two regions of negative TMR are observed,which is accompanied with multiple sign changes.

Fig.4.Tunnel magnetoresistance(TMR)as a function of temperature difference.The parameters used in(a)areε0=1,λ=0,p=0.4/0.5/0.6,and in(b)areλ=0.5,p=0.5,ε0=0.5/0.75/1.The other parameters areΓ=0.02 and background temperature kBT=0.01.All energies are in units of meV.
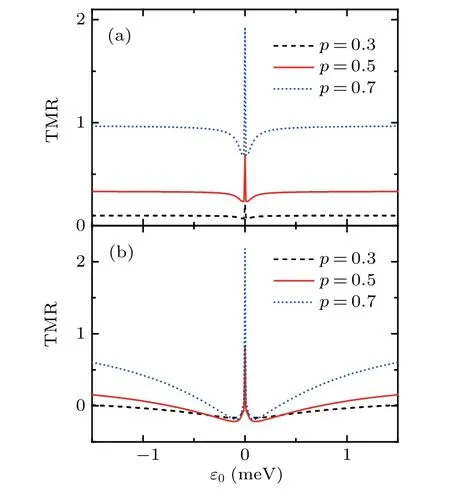
Fig.5.Tunnel magnetoresistance(TMR)as a function of energy level ε0 for(a)λ=0 and(b)λ=1.The spin polarizations in(a)and(b)are the same,as indicated.The other parameters areθ=30,Γ=0.02,and background temperature kBT=0.01.All energies are in units of meV.
4.Conclusions
In summary,we have used an exactly solvable model to discuss thermal-driven quantum transport involved with the interplay of Majorana fermion and spin polarization.It is found that the interplay of Majorana fermion and spin polarization can induce a nonlinear thermal-bias spin current.A thermaldriven negative tunnel magnetoresistance is also found.With large temperature difference,the gate tuned spin-up current becomes linear,when the coupling between quantum dot and topological superconductor is strong enough,revealing Majorana fermion’s robustness.These results may be useful in the future design of thermal-based spintronic devices.
- Chinese Physics B的其它文章
- Multiple solutions and hysteresis in the flows driven by surface with antisymmetric velocity profile∗
- Magnetization relaxation of uniaxial anisotropic ferromagnetic particles with linear reaction dynamics driven by DC/AC magnetic field∗
- Influences of spin–orbit interaction on quantum speed limit and entanglement of spin qubits in coupled quantum dots
- Quantum multicast schemes of different quantum states via non-maximally entangled channels with multiparty involvement∗
- Magnetic and electronic properties of two-dimensional metal-organic frameworks TM3(C2NH)12*
- Preparation of a two-state mixture of ultracold fermionic atoms with balanced population subject to the unstable magnetic field∗