Multiple induced transparency in a hybrid driven cavity optomechanical device with a two-level system∗

Wei Zhang(张伟),Li-Guo Qin(秦立国),Li-Jun Tian(田立君),‡,and Zhong-Yang Wang(王中阳)

1Department of Physics,Shanghai University,Shanghai 200444,China

2School of Mathematics,Physics and Statistics,Shanghai University of Engineering Science,Shanghai 201620,China

3Shanghai Advanced Research Institute,Chinese Academy of Sciences,Shanghai 201210,China

Keywords:hybrid optomechanical device,optomechanical induced transparency,two-level system

1.Introduction

As an excellent candidate for studying the transform of a macroscopic degree of freedom from the classical to the quantum regimes,cavity optomechanics composed of an Fabry–P´erot(FP)cavity with its end-mirror replaced by a nanomechanical resonator(NMR)has received considerable attention over the last decade.[1–5]In this emerging field,remarkable progress has been made in both theory and experiment,including quantum ground-state cooling of NMR,[6–8]slowing and storage of the light pulse,[9–11]information processing,[12–14]and optomechanical induced transparency(OMIT).[15–21]

When a strong control field and a weak probe field are applied to an optomechanical cavity,an optomechanical interference can lead to generation of OMIT,which has been reported theoretically[17]and then demonstrated experimentally.[22]Some exciting phenomena including the tunable slow and fast light,[23,24]four-wave mixing,[25]electro-optic interconnect[26]and squeezing of the mechanical model in the resolved sideband regime have been proposed based on the mechanism of OMIT.[27–29]

As a preferred component in the construction of complex quantum systems,NMR with the wide frequency range is a promising candidate to explore various applications of quantum behavior.[30–32]To find the properties and applications of NMR in the quantum region,it can be combined with other quantum systems to form hybrid systems,such as optomechanical systems[33,34]and quantum electromechanical systems.[14]A two-level system(for example,superconducting qubit,quantum dot and the inherent defect in materials)can also be embedded in NMR to study various quantum phenomena,such as controllable coupling,[35,36]quantum entanglement[37]and phonon blockade.[38]As a two-level system,the defect inside the NMR can interact with the NMR through the deformation force.Especially,when the transition frequency of an intrinsic two-level defect inside NMR is near resonance with the NMR frequency,the great effects of the defect on the quantum properties of NMR should not be ignored.[39–42]

Motivated by recent progress of two-level systems in optomechanical devices,here we study the interaction between a two-level system and a hybrid optomechanical device.Our model consists of an FP cavity tunneling coupled to an optomechanical cavity,where the two-level system is embedded in the movable cavity mirror of the optomechanical cavity.The two-level system can be considered as a defect inside the material of the movable cavity mirror or a superconducting charge qubit.The movable cavity mirror can be viewed as an NMR,which is coupled to the two-level system via the Jaynes–Cummings coupling.In this hybrid optomechanical system,we find the multiple optomechanically induced transparency and discuss the effects of two-level system on OMIT.

The paper is organized as follows.In Section 2,we describe the model by an effective Hamiltonian and give Langevin motion equations and their stable solutions.In Section 3,a profile of multiple OMIT windows of the output field is presented.In Section 4,we discuss the effect of the qubit(two-level system)on the OMIT.A conclusion is given in Section 5.

2.Model and quantum Langevin equations

As schematically shown in Fig.1(a),our model consists of an optical cavity a1tunneling-connected to an optomechanical cavity a2,where as a moveable mirror of the optomechanical cavity,NMR b mechanically couples to a two-level system.The optical cavity a1is driven by a strong control fieldεcwith frequencyωcand a weak probe fieldεpwith frequencyωp.The two cavity fields are coupled via the photon tunneling interaction with the coupling strength J.The NMR with the frequencyωmis coupled to the optomechanical cavity a2by the radiation pressure with the optomechanical coupling strength χ.

Fig.1.(a)Schematic diagram of a hybrid optomechanical system.The optomechanical cavity a2 with frequencyωa2 is coupled to the optical cavity a1 with frequencyωa1(via the tunneling interaction)and to the NMR(via the optomechanical coupling).As a two-level system,superconducting qubit or a defect is embedded inside the NMR,where|g〉and|e〉denote the ground and excited states,respectively.The hybrid optomechanical system is driven by a strong control field with the frequencyωc and a weak probe field with the frequencyωp.(b)The double-well potential model for the two-level system.Δ0 andΔT are tunnel splitting frequency and the asymmetry frequency in the doublewell potential,respectively.

In our model the intrinsic defect inside NMR or a superconducting charge qubit attached on NMR can be effectively described by the two-level state in a double-well potential at low temperature,as shown in Fig.1(b).It is worth noting that the frequency gap of two energy levels is resonant or nearresonant with the frequency of the NMR,and the other energy levels are highly detuned with the frequency of the NMR,i.e.,two-level approximation.For an isolated two-level system and the single mechanical mode of NMR,the Hamiltonian reads

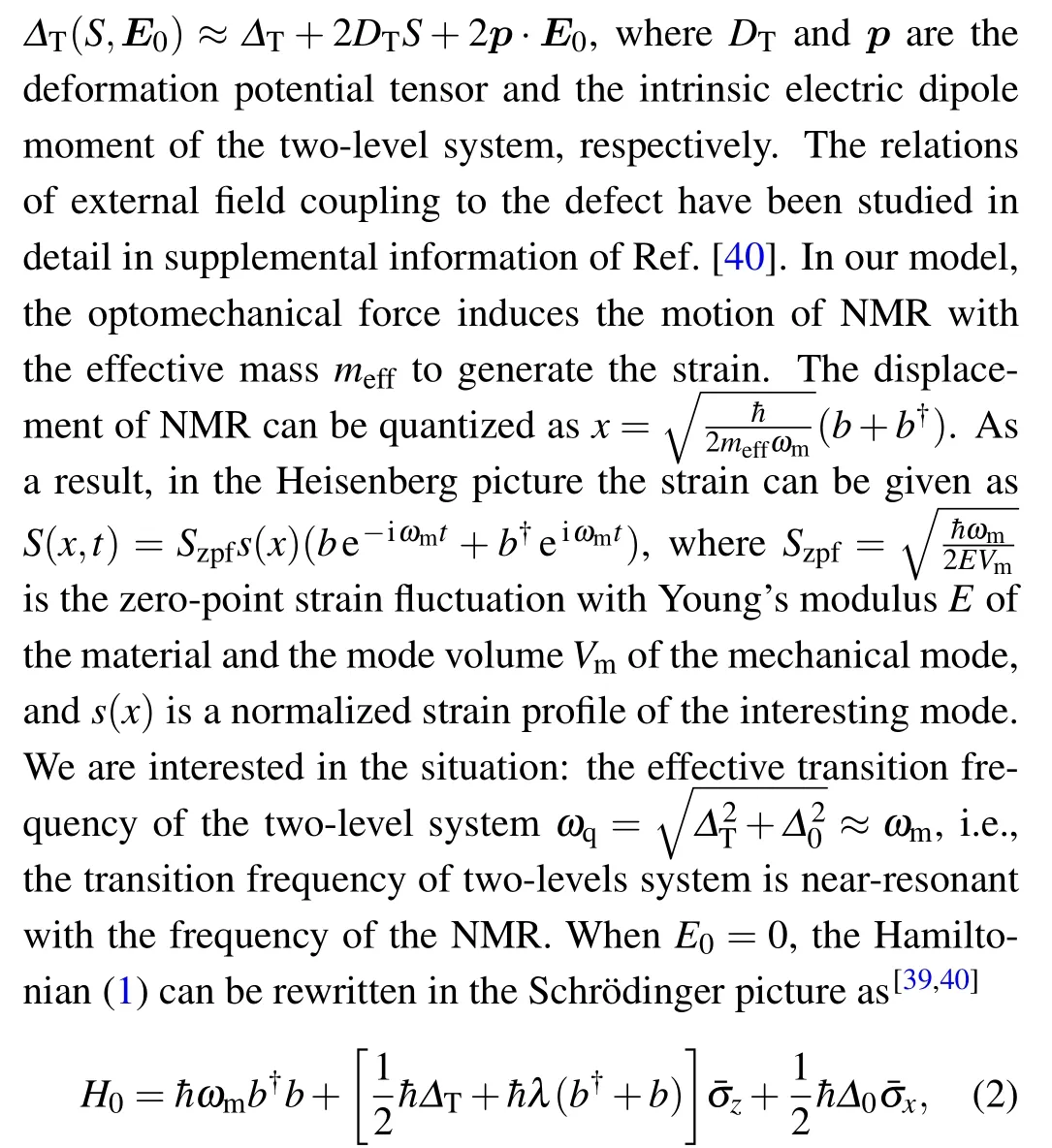
where cosθ=ΔT/ωqand sinθ=Δ0/ωq.Equation(2)can be transformed to

where g=λsinθdenotes the effective coupling strength between the NMR and the two-level system,andσ−(σ+)is the lowering(raising)operator for the two-level system.Making use of the rotating-wave approximation to omit counterrotating terms,the Hamiltonian in Eq.(3)can be rewritten as[16,39,40,43,44]

The first and second terms in Eq.(2)describe the energies of the NMR and qubit,respectively.The last term in Eq.(4)describes the JC-type coupling interactions between the twolevel system and the NMR.In summary,for the JC-type coupling the following realizable physical conditions should be met:(a)The two-level system and the NMR are in near resonance,and are far-resonance with the cavity field.(b)The two-well potential satisfies the two-level approximation condition.(c)The conditions of the rotating-wave approximation,i.e.,the fast oscillation term of far-resonance,can be ignored.(d)Only consider the effects of the strain on the asymmetry frequency from NMR.Thus,the total Hamiltonian of the hybrid system in the rotating frame can be given by

where the detuningsΔ1(2)=ωa1(2)−ωc,aiis the annihilation operators of the i-th cavity field with the decay rateκi(i=1,2),respectively.The third and fourth terms in Eq.(5)describe the coupling interactions between the two cavities,the optomechanical cavity a2and the NMR,respectively.The driven Hamiltonian Hdrcan be written as

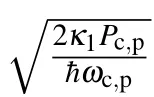
From the Hamiltonian(5),the evolution of the system is determined by the quantum Langevin equations,which can be given as follows:

whereγmandγqare the decay rates of the NMR and qubit,respectively.The operators a1(2),in(t),bin(t),Γ−(t)andΓz(t)denote the zero-mean environment noises corresponding to the two cavities fields,the NMR and the two-level system,respectively.In order to solve the nonlinear equations,the mean field approximation〈AB〉=〈A〉〈B〉is used here,where A and B are two arbitrary operators.By the linear way,each operator can be split as a sum of its steady-state value and an additional small fluctuation operator,i.e.,O=Os+δO(O=a1,2,b,σ−andσz).Oscorresponds to the stable solution of O,when the strong driving fields applied to the system;δO denotes the first order fluctuation operator of the injected weak fieldεp.With these approximations,the steady-state values can be calculated by

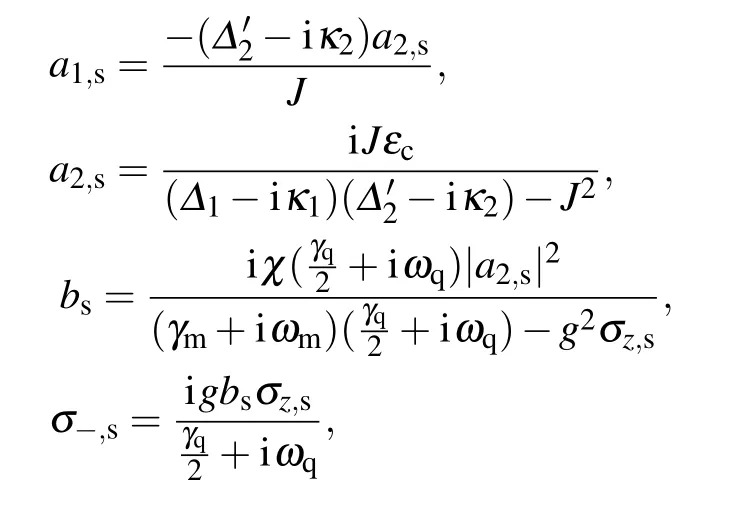
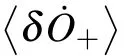
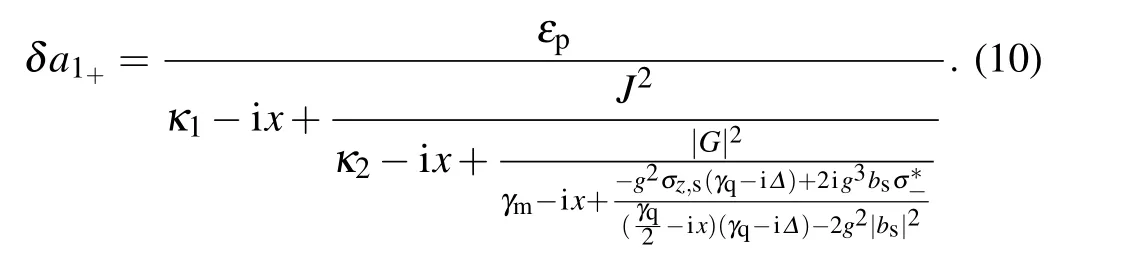
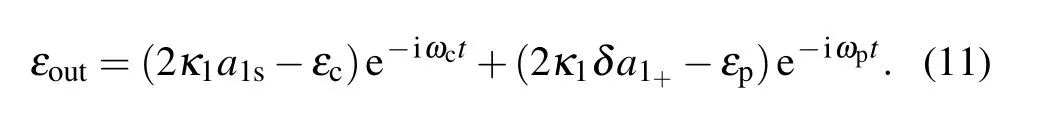
Here,the first term in Eq.(11)on the right side corresponds to the output field at the control field frequencyωc.The second term corresponds to the output field at the probe field frequencyωp.We are interested in the output field at the probe field frequency,therefore we can define the transmission of the probe field as

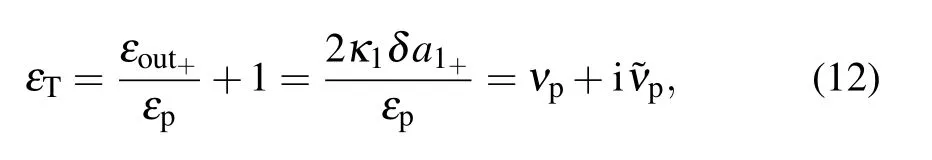
whereνp=Re(εT)and˜νp=Im(εT)describe the absorption and dispersion of the whole system at the probe frequency,respectively.

3.The multiple induced transparency windows profile in the output field

In this section,we numerically study the transmission of a weak probe field through a hybrid optomechanical system.The parameters we used in the simulation[16,45]areωm/2π=ωq/2π=51.8 MHz,κ1/2π=15.0 MHz,κ2/2π=1.5 MHz,γm/2π=41.0 kHz,γq/2π=51.8 kHz,J/2π=15.0 MHz,χ/2π=2.16 kHz,and g/2π=10.0 MHz.

In order to more intuitively show the interactions in the hybrid system and to better understand the physical meaning of our results,the energy level diagram of the model is plotted as shown in Fig.2.We can find that there are three interaction relationships associated with the different subsystem in our model,i.e.,the tunneling coupling interaction between the two cavities,the optomechanical interaction between the cavity and the NMR,and the JC coupling interaction between the qubit and NMR.Next,we study the effects of three interactions on the output probe field.

Fig.2.Energy-level diagram of the hybrid system.The number state of the cavity i-th photons and phonons are denoted by Ni and n.The tunneling coupling strength between|N1+1,N2,n〉and|N1,N2+1,n〉is J;the effective optomechanical coupling strength between|N1,N2+1,n〉and|N1,N2,n+1〉is G;and the qubit–NMR coupling strength is g.

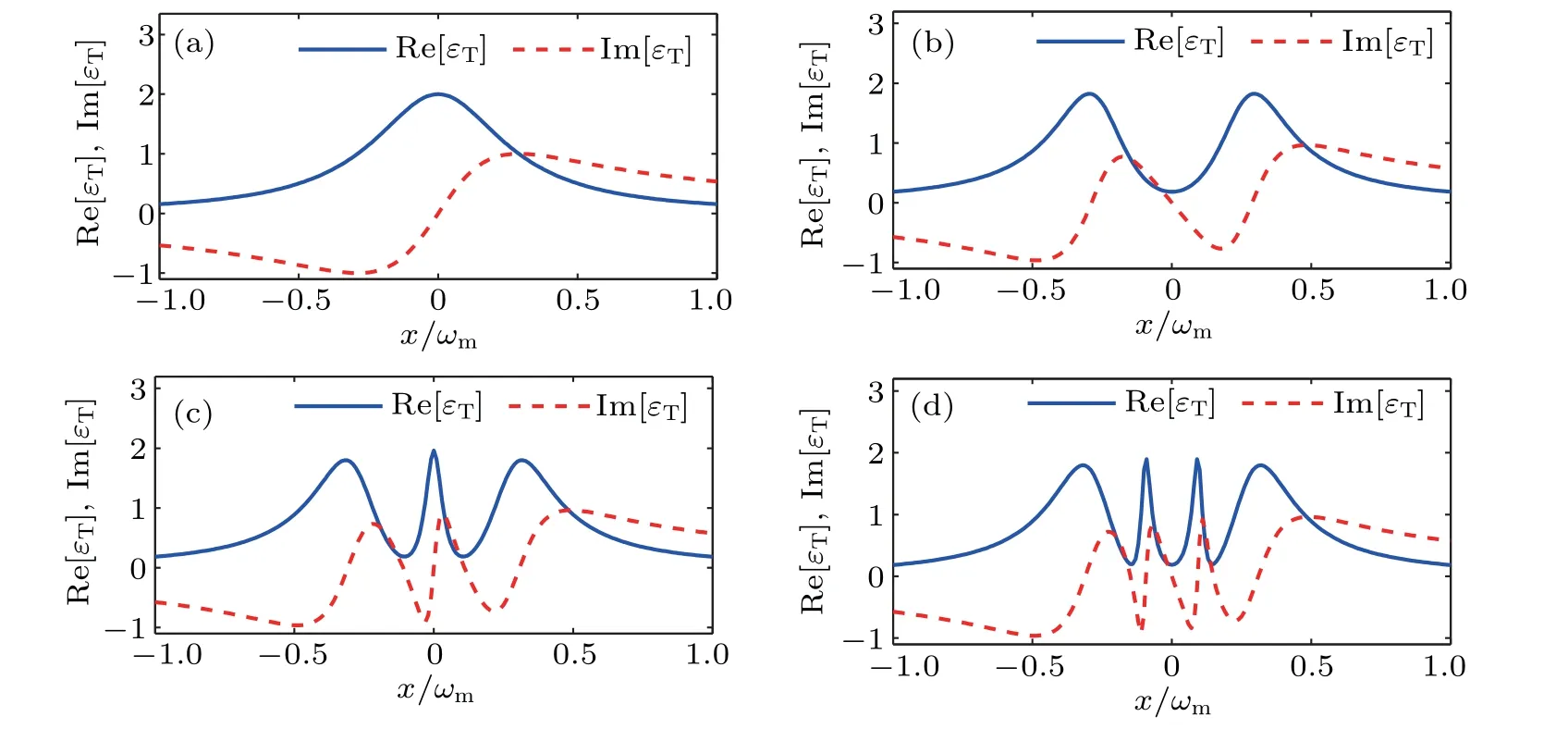
Fig.3.The absorption Re[εT](blue-solid line)and dispersion Im[εT](red-dotted line)as a function of x/ωm in the different coupling cases;i.e.,(a)g=0,χ=0,J=0;(b)g=0,χ=0,J/2π=15.0 MHz;(c)g=0,χ/2π=2.16 kHz,J/2π=15.0 MHz;(d)g/2π=10.0 MHz,χ/2π=2.16 kHz,J/2π=15.0 MHz.The other parameters are given asκ1/2π=15 MHz,κ2/2π=1.5 MHz,γq/2π=0.1 MHz,γm/2π=41.0 kHz,andωm/2π=51.8 MHz.

4.Effect of the two-level system on the output field

The two-level system here can be considered as artificial modulation systems such as qubit,quantum dot or defect inside the NMR material.When the transition frequency of the two-level system is at near resonance with the oscillation frequency of the NMR,the effect of the two-level system on the motion of NMR cannot be neglected,especially quantum properties of NMR.[40,41]Therefore,in this section we give the discussions about the effects of the two-level system(qubit)on the output field.In Fig.4,we show that the absorption spectra of the probe field are plotted as a function of x/ωmfor different values of the qubit–NMR detuning(Δq=ωq−ωm)and coupling strength.In the absence of the qubit–NMR coupling(i.e.,g=0),a symmetrical double OMIT arising from the photon tunneling and optomechanical coupling is shown in Fig.4(a)and corresponds to the case of Fig.2(c).[45]However,when the qubit–NMR coupling is considered,a additional transparency window appears because the two-level system introduces extra channels with the two dressed states.In the same qubit–NMR coupling cases,Figs.4(b)–4(d)show that the symmetry of the double transparency window is broken due to the qubit–NMR strong coupling with the different detuning.In the cases of the red-detunedωq(i.e.,ωq<ωm)in Fig.4(c)and blue-detuned ωq(i.e.,ωq>ωm)in Fig.4(d),the transparency window will have the corresponding redshift and blueshift by comparing it with the case ofωq=ωmin Fig.4(b).[16]As the coupling strength g increases,the width of the central transparency window will be widened as shown in Fig.4(e)compared with Fig.4(b).Therefore the two-level system attached on NMR can greatly effect on the frequency width and intensity of the output field by changing the coupling strength g and detuning Δq.

Fig.4.The absorption Re[εT]of the probe field as a function of x/ωm for different values of qubit–NMR detuning and coupling strength:(a)g=0,Δq=0;(b)g=0.2ωm,Δq=0;(c)g=0.2ωm,ωq<ωm;(d)g=0.2ωm,ωq>ωm;(e)g=0.25ωm,Δq=0.The other parameters are the same as those in Fig.3.

Furthermore,we study the variations of the triple OMIT windows in the different cases of the laser powers of the control field and the decay rates of the two-level system.As shown in Fig.5(a),the absorption peaks and the transparency dips on both sides move away from the center of symmetry x/ωm=0,whereas both the center absorption peaks come closer x/ωm=0 as the laser power value Pcincreases with the fixed decay of qubitγq.The changes come from both the enhanced cavity fields due to the high laser power,which are different from the effects of the qubit–NMR coupling strength g in Fig.4.The effects of the decay rateγqon the output field are shown when the other parameters are fixed in Fig.5(b).We can find that the positions of absorption peaks and transparency dips remain almost unchanged in the horizontal direction,while the transparency dips get more in-depth,and the absorption peaks become higher as the decay rateγqdecreases.

Fig.5.The absorption Re[εT]as a function of x/ωm in the different cases of the modulation Pc with the fixedγq/2π=0.1 MHz in(a),the modulationγq with the fixed Pc=8 mW.The inset in(b)is the enlarged figure around x/ωm=0.17 MHz.The other parameters are same as those in Fig.3.

In addition,we also study the qubit population inversion σz,sas a function of the steady-state phonon number n.In Fig.6,at the initial time the qubit is prepared on the ground state|g〉,the population inversionσz,sfirstly rapidly increases,then reaches a saturation value near zero when phonon number n increases.This indicates that one can modulate the population inversionσz,sof the two-level system by the phonon number.We can find that the population inversion changes faster in the red-detuning case than the blue-detuning due to the enhanced anti-Stokes and suppressed Stokes fields.

Fig.6.The population inversionσz,s as a function of the steady-state phonon number n.The other parameters are same as those in Fig.3.

5.Conclusion

In summary,we have investigated the multiple-induced transparency of a hybrid optomechanical device consisting of an FP cavity tunneling coupled with an optomechanical cavity,where the end mirror of the optomechanical cavity is the NMR coupled to a two-level system.We have analyzed the physical mechanism of each transparency window by discussing the generation process of three transparency windows in detail.It is found that each of the three couplings(including photon tunneling,photomechanical and phonon–two-level system couplings)introduces a transition channel.Each of them will produce a transparent window;finally,the triple transparent windows are displayed.In addition,we focus on the effects of the two-level system on the output field and find that the qubit–NMR coupling strength can change the width of the transparency windows,and the qubit–NMR detuning can break the symmetry and change the positions of the windows.Furthermore,the effects of the laser power Pcof the control field and the decay rateγqof the two-level system on the transparency windows are considered.As the power Pcincreases,the two transparency windows on both sides become wider,while the central transparent window becomes narrower.Although the decay rateγqdecreases,the position of the absorption spectrum peak is slightly changed,andγqhas only a slight effect on the width of the middle transparent window.In addition,we discuss the relations between the population inversion of the qubit and the steady-state phonon number in the different detuningΔqcases.