Temperature-induced phase transition of two-dimensional semiconductor GaTe*

Xiaoyu Wang(王啸宇), Xue Wang(王雪), Hongshuai Zou(邹洪帅),Yuhao Fu(付钰豪), Xin He(贺欣),‡, and Lijun Zhang(张立军),§

1State Key Laboratory of Integrated Optoelectronics,Key Laboratory of Automobile Materials of MOE,College of Materials Science and Engineering,Jilin University,Changchun 130012,China

2State Key Laboratory of Superhard Materials,College of Physics,Jilin University,Changchun 130012,China

Keywords: two-dimensional semiconductor GaTe, temperature-induced phase transition, first-principles calculation,quasi-harmonic approximation

1. Introduction

In recent years, two-dimensional (2D) semiconductors,bonded through van der Waals forces, have attracted extensive research interests due to their extraordinary properties and potential applications in electronic and optoelectronic devices.[1–5]They have unique layer-dependent electronic properties. III–VIA compound gallium telluride(GaTe)is a 2D layered semiconductor with a moderate direct bandgap of ～1.65 eV[6–8]and a high photoresponsivity for few-layer sheets (104A/W),[9]which render it great potential applications in the field of optoelectronic devices.[10–12]However,current studies on 2D GaTe have obvious limitations. There are two phases of GaTe reported, the monoclinic phase (m-GaTe, space group C2/m) and the hexagonal phase (h-GaTe,space group P63/mmc),[13–16]but current studies are still exclusively restricted to the m-GaTe owing to the difficulty in the fabrication of 2D h-GaTe. Unlike the situation in other III–VIA 2D semiconductor materials,such as GaS,GaSe,and InSe,[17–19]the most stable phase of GaTe under room temperature is the low symmetry monoclinic phase, rather than the high symmetry hexagonal phase.[20]

The phase transition between the monoclinic and hexagonal phases of GaTe was reported experimentally. Gillan et al.fabricated h-GaTe using the metal-organic chemical vapor deposition technique. They found that h-GaTe gradually transformed into m-GaTe upon annealing at 500◦C.[15]Yu et al.succeeded in driving the GaTe phase transition from h-GaTe to m-GaTe using laser irradiation.[20]Zhao et al. obtained h-GaTe by stripping several layers of m-GaTe. They proposed a two-stage transformation mechanism. Firstly, m-GaTe transformed into a tetragonal phase (t-GaTe), then t-GaTe transformed into h-GaTe spontaneously.[21]Both annealing and laser irradiation were accompanied by temperature changes.Gillan et al. mentioned that controlling the synthesis temperature affects the lattice structure of the product.Therefore,temperature variation should be the most important factor,which influences the phase transition process of GaTe.

In this paper,we investigated the phase transition of GaTe by using quasi-harmonic approximation(QHA)to estimate the Gibbs free energy.[22]We predicted a phase transition from h-GaTe to m-GaTe when the temperature is lowered to 261 K within the QHA method. The calculated results are consistent with the phase transition process from h-GaTe to m-GaTe with the cooling annealing and laser cooling irradiation treatment in the experiments.[15,20]We also used the nudged elastic band(NEB) method[23,24]to estimate the barriers and transition state structures of the phase transition from m-GaTe to t-GaTe and from t-GaTe to h-GaTe. We obtained that the m-GaTe to t-GaTe transition process has a barrier of 199 meV/formula and the t-GaTe to h-GaTe phase transition has a barrier of 288 meV/formula. The relatively high energy barriers demonstrate the irreversible nature of the phase transition, which is consistent with the experimentally observed results. In addition, we further investigated the thermodynamic stable, electronic,and phonon properties of m-GaTe to t-GaTe phases. It was found that the calculated bandgap values and the Raman spectra are in satisfactory agreement with the experimental results,indicating the reliability of our results.

2. Method

We performed first-principles calculations based on density functional theory,as implemented in the Vienna ab initio simulation package.[25–27]The electron–core interaction was described by using the projected augmented wave pseudopotentials. The 4s24p1(Ga) and 5s25p4(Te) were treated explicitly as valence electrons. We used the generalized gradient approximation in the Perdew–Burke–Ernzerhof[28]form as the exchange-correlation functional. Structure optimization(including lattice parameters and internal atomic positions)was performed using the conjugate gradient technique[29]until the energy converged to 10-6eV and the force converged to 0.005 eV/˚A.A kinetic energy cutoff of 230 eV was used for the wave-function expansion and a grid spacing of 2π×0.03 ˚A-1was used for electronic Brillouin zone integration.To properly take into account the long-range van der Waal(vdW)interactions, after a serious of tests to vdW-optB86b, vdW-optB88,vdW-optPBE, and vdW-DF2 functional, the vdW-optB86b functional was adopted.[30]Since the standard density functional tends to underestimate the band gap of semiconductors, the higher-level hybrid density functional HSE06[31]was used to calculate the electronic structures.[32,33]Spinorbit coupling was taken into consideration since it is potentially important for electronic structures of heavy p-electron systems. The phase transition barriers were calculated using the NEB method in conjunction with the climbing image method.[23,24,34]Harmonic phonons properties were calculated using the real-space supercell approach as implemented in the PHONOPY code.[35,36]We obtained the temperature dependence of the Gibbs free energy for m-GaTe and h-GaTe by using the QHA theory,[37]which has been successfully used in describing temperature-induced phase transition processes.[22,38–40]

3. Results and discussion

Figures 1(a) and 1(b) show the crystal structures of m-GaTe and h-GaTe, respectively. Both phases have layered structures, with two Ga atoms sandwiched between two Te atoms in each layer. But it is clear that the two structures are quite different in the atom arrangements. In h-GaTe phase,each Ga atom is bonded to one Ga atom and three Te atoms,and the two sublayers present an AA stacking along the perpendicular direction to form bulk,with the weak van der Waals interaction between layers. In contrast,the crystal of m-GaTe demonstrates a distorted layer-structure in which one-third of the Ga–Ga bonds turn from vertical to horizontal towards to the layer plane. The optimized lattice parameters are shown in Table 1, and the corresponding experimental data are also list for reference.[16,41,42]The monoclinic phase m-GaTe with space group C2/m has the lattice parameters of a=17.440 ˚A,b=4.129 ˚A, c=10.511 ˚A, α =γ =90◦, and β =103.8◦.The hexagonal phase h-GaTe with space group P63/mmc has the lattice parameters of a = b = 4.106 ˚A, c = 16.918 ˚A,α =β =90◦, and γ =120◦. Our structural optimization results well agree with the reported experimental values,with an error less than 1%.

Fig.1. The side and top views of crystal structures of m-GaTe(a)and h-GaTe(b). The elements of Ga and Te are in blue and brown,respectively.

Table 1. Experimental(Exp.) and calculated(Cal.) lattice parameters(in ˚A)of m-GaTe and h-GaTe.

Now, we turn to the phase transition temperature of GaTe. We determined the temperature of phase transition by comparing the Gibbs free energy as a function of temperature at constant pressure. The quasi-harmonic approximation is a phonon-based model of solid-state physics used to describe volume-dependent thermal effects. Within the QHA method,[37]the Helmholtz free energy at temperature T and equilibrium lattice volume V is defined as

where q is all wave vectors and λ is all three phonon branches in the first Brillouin zone. kBis the Boltzmann constant, ħ is the reduced Planck constant, and ωqλ(V)is the frequency of the phonon. Then the Gibbs free energy is obtained by minimizing the free energy,

where minV[function of V]means to find the unique minimum value in the brackets by changing the volume. Figure 2(a)shows the curves of the Gibbs free energy with respect to temperature of m-GaTe and h-GaTe. The curve intersects at 261 K.Therefore,we proved the possibility of a temperatureinduced phase transition between the two phases and predicted the theoretical phase transition temperature of 261 K.

We further obtained the trend of volume change with temperature, as shown in Fig. 2(b). The volume of m-GaTe is smaller than that of h-GaTe over the studied temperature range. Meanwhile, the thermal expansion coefficient of m-GaTe is higher.Therefore,in the phase transition process from m-GaTe to h-GaTe,the crystal lattice of h-GaTe suffers growing compressive stress during the nucleation and growth process.As a result,the phase transition requires a greater driving force,and the actual phase transition temperature of the heating process might be higher than the theoretical phase transition temperature. The current analysis also provides a new perspective for understanding the spontaneous phase transition from m-GaTe to h-GaTe caused by the exfoliating process:when the bulk GaTe is exfoliated into layered GaTe,the stress is released. According to the relationship of Gibbs free energy described above, there will be a spontaneous phase transition from m-GaTe to h-GaTe under room temperature.

Fig. 2. (a) Gibbs free energy versus temperature of h-GaTe (red line)and m-GaTe (black line) curve. (b) Volume versus temperature of h-GaTe(red line)and m-GaTe(black line).

In order to have an in-depth understanding of the phase transition of GaTe, we further calculated the energy barrier to obtain the energy required to accomplish the phase transition. We adopted a two-step phase transition process mechanism,which has been reported by Zhao et al.[21]The structure evolution and energy barriers have been identified by using NEB method with five and eight images as intermediate states for the phase transition process from m-GaTe to t-GaTe and from t-GaTe to h-GaTe, respectively. The results are shown in Fig.3. Both m-GaTe and h-GaTe have a layered structure,which means that the phase transformation occurs within the layers. The first step is the phase transition from m-GaTe to t-GaTe. In this process,the Ga–Ga bonds parallel to the layers are reversed and transformed into positions perpendicular to the layers. The process is accompanied by the breakage of the original Ga–Te bonds and the generation of new Ga–Te bonds.The transition state structure of the first step corresponds to the structure whose Ga–Te bonds are between the bond breaking and bond formation processes. The second step is the movement of the entire layer of Te atoms, resulting in the phase transition from t-GaTe to h-GaTe. The transition state structure of the second step corresponds to the structure whose Te atoms move to the intermediate critical positions between the Ga atoms. The barrier of the first process is 199 meV/formula and that of the second process is 288 meV/formula. Previous studies have shown that the phase transition barrier of～300 meV/formula is sufficiently large to demonstrate the stability of the phase transition product of indium selenide and tungsten ditelluride.[43,44]Therefore, the relatively high energy barriers ensure that the phase transition of GaTe is irreversible. We note that the phase transition from m-GaTe to t-GaTe is accompanied by bond formation and bond breaking,but the barrier is lower than that of the phase transition from t-GaTe to h-GaTe. This is due to the considerable atom movement in the t-GaTe to h-GaTe transition process, even half of the Te atoms are involved in the movement.

Fig.3. Phase transition barriers from m-GaTe to h-GaTe and schematic representation of the bond and atom rearrangement in the phase transition process.

Fig.4. (a),(b)Calculated orbital projected electronic band structures for m-GaTe(a)and h-GaTe(b). Red and blue colors represent projections onto constituting orbital species Te p and Ga p. (c) Calculated bandgap results by using HSE06 + SOC functional. (d) Calculated effective masses of electron(m*e)and hole(m*h).

Figures 5(a) and 5(b) show the calculated phonon dispersion curves of m-GaTe and h-GaTe, which exhibit no imaginary modes in the whole Brillouin region, thus indicating that the two phases are kinetically stable. Figures 5(c) and 5(d) show our calculated Raman spectra, and the corresponding experimental data from previous works. The most active two peaks are 109.04 cm-1and 114.85 cm-1for m-GaTe in our calculation. They are in good agreement with the experimental measurements of 109 cm-1and 115 cm-1for freshly cleaved GaTe(Exp.1).[48]Due to oxidization,the m-GaTe Raman peaks decrease whereas the new peaks at wavenumbers 123 cm-1and 140 cm-1become prominent over time under ambient condition (Exp. 2).[49]However, previous works insisted on the two peaks belonging to intrinsic Raman peaks of h-GaTe.[20,49,50]In our calculated results for h-GaTe,the most active peak is 96.75 cm-1. The Raman spectra need more experimental and theoretical approaches for further clarification.

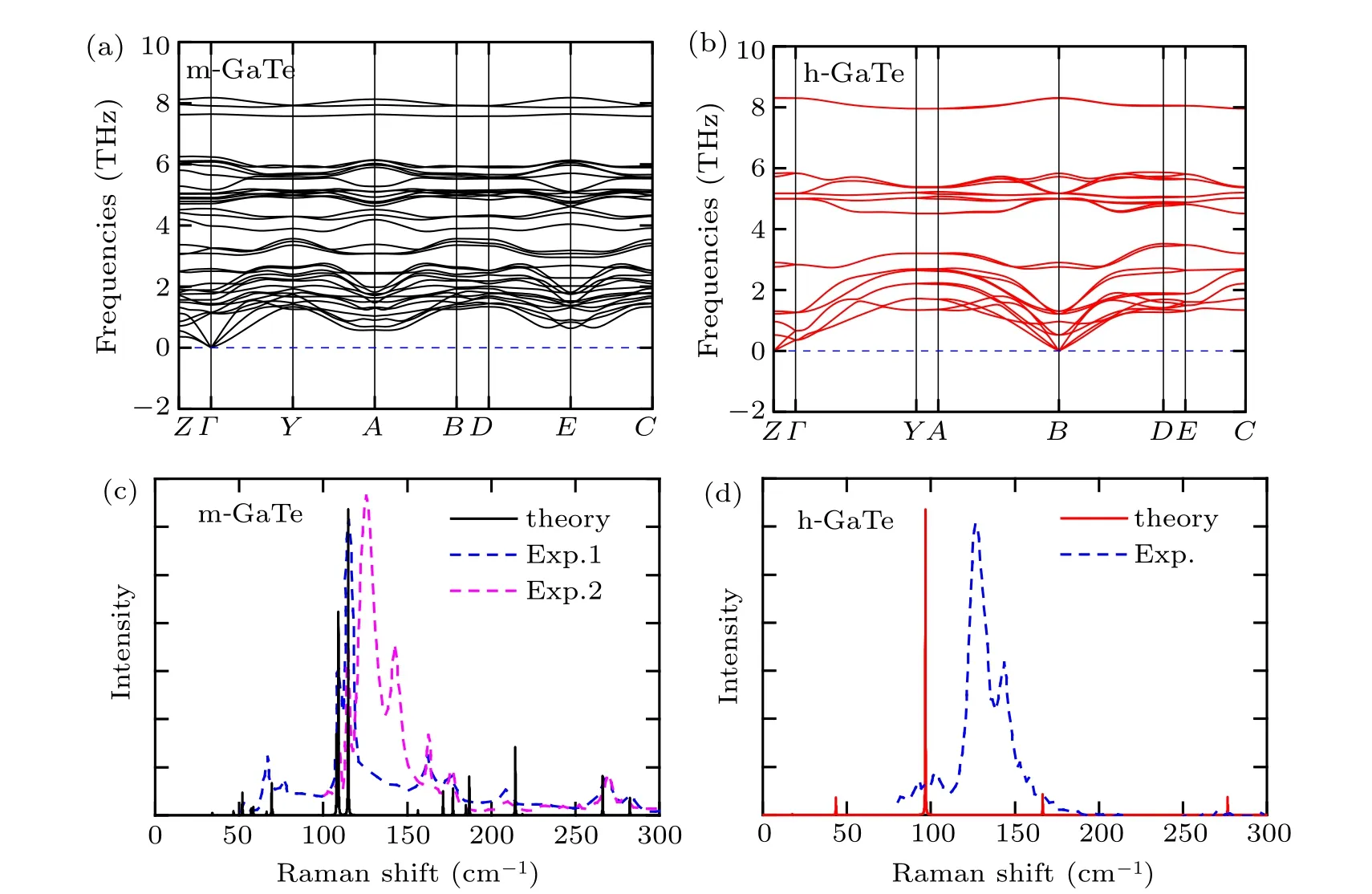
Fig. 5. Calculated phonon dispersion and Raman spectra of (a), (c) m-GaTe and (b), (d) h-GaTe. Here theory is our result, compared with experiment results from previous reports(Exp.1,[48] Exp.2,[49] Exp.[20]).

4. Conclusion and perspectives

By using first-principles energetic and phonon calculations within the quasi-harmonic approximation framework,we investigated the temperature-induced phase transition process in two-dimensional semiconductor GaTe. We predicted that the phase transition from h-GaTe to m-GaTe will occur at the temperature decreasing to 216 K. Our results are consistent with the phase transition condition from h-GaTe to m-GaTe observed in the experiments, such as the cooling process during annealing and laser irradiation. Based on the previously reported two-step phase transition process, we used the nudged elastic band method to calculate the phase transition barriers and investigate the corresponding transition state structures. The m-GaTe to t-GaTe phase transition barrier is 199 meV/formula and the t-GaTe to h-GaTe phase transition barrier is 288 meV/formula. The large phase transition barriers demonstrate the irreversible nature of the phase transition. The structure evolution in the phase transition process indicated that the bond broken is responsible for the high energy barriers. The electronic and phonon properties of the two phases were further investigated by comparison with available experimental and theoretical results. The m-GaTe is a direct bandgap semiconductor with a gap of 1.449 eV, which is close to the experimental value. The h-GaTe is an indirect bandgap semiconductor whose fundamental energy bandgap is 0.608 eV (0.48 eV lower than the direct bandgap). Our calculated Raman spectra are in general agreement with the experimentally measured data. This work provides insightful understanding on the process of temperature-induced phase transition of GaTe.

Acknowledgments

We acknowledge stimulating discussions with Prof. Xuetao Gan (Northwestern Polytechnical University). Calculations were performed in part at the high-performance computing center of Jilin University.