Magnetohydrodynamic Stagnation Point Flow of a Maxwell Nanofluid with Variable Conductivity
M.Irfan,M.Khan, W.A.Khan, and M.Alghamdi
1Department of Mathematics,Quaid-i-Azam University,Islamabad 44000,Pakistan
2School of Mathematics and Statistics,Beijing Institute of Technology,Beijing 100081,China
3Department of Mathematics,Faculty of Science,King Khalid University,Abha 61413,Kingdom of Saudi Arabia
Abstract This article reports the simultaneous properties of variable conductivity and chemical reaction in stagnation point flow of magneto Maxwell nanofluid.The Buongiorno’s theory has been established to picture the inducement of Brownian and thermophrotic diffusions effects.Additionally,the aspect of heat sink/source is reported.The homotopic analysis method (HAM) has been worked out for the solution of nonlinear ODEs.The behavior of inferential variables on the velocity,temperature,concentration and local Nusselt number for Maxwell nanofluid are sketched and discussed.The attained outcomes specify that both the temperature and concentration of Maxwell fluid display analogous behavior,while the depiction of Brownian motion is quite conflicting on both temperature and concentration fields.It is further noted that the influence of variable thermal conductivity on temperature field is similar to that of Brownian motion parameter.Moreover,for the confirmation of our study comparison tables are reported.
Key words: Maxwell nanofluid,stagnation point flow,magnetohydrodynamic (MHD),variable conductivity,chemical reaction
Recently,owing to their multifaceted and numerous micro- structures,non-Newtonian liquids[1−3]constantly persist an arena of actual desire for engineers and researchers.Therefore,widespread endeavors have been prepared to study their worth in numerous built-up applications.The noteworthy feature of these liquids are their advanced apparent viscosities thus,laminar flow conditions rise more habitually when allied with Newtonian liquids and Prandtl numbers are maximized.Numerous materials in the biological liquids,compound or foodstuff developments and petrochemical are non-Newtonian in nature.These materials comprise plasma,salvia,microelectronic cooling structures,firewood in aquatic and china soil,sewage slurry,greasepaints and vapor–liquid disseminations categorized as non-Newtonian liquids.The liquids follow nonlinearity properties between shear rate and shear stress named as non-Newtonian liquids.These liquids modify flow aspects of liquid,which outcomes affect the aptitude of the liquid’s in transferring heat.Additionally,diverse liquid models wished-for to clarify the structure and rheological aspects of nonlinear materials.The Maxwell model is a rate type nonlinear model which forms the stress relaxation of numerous polymeric liquids.Limited studies covering Maxwell fluid under a diverse flow structure can be discussed via Refs.[4–8].Fractional Maxwell liquid with variable thickness was reported by Liu and Liu.[9]They attained elucidations of the problem numerically via L1-scheme.Khanet al.[10]studied the performance of nanoparticles condition and heat sink/source in radiative Maxwell nanomaterial.They inspected that the Deborah number and radiation parameter have possess behavior on temperature field.Impact of nonlinear radiation in Maxwell nanofluid subject to rotating disk was elaborated by Ahmedet al.[11]They noted that the thermal radiation parameter enhances the fluid temperature.
Modern growths in nanotechnology have fetched new discussion of heat transfer liquids acknowledged as nanofluids.Nanofluids are solid-fluid,complex materials prepared of condensed nanoparticles with size(1–100)nm and possess an aptitude of advanced thermal conductivity.These particles,mostly a metal or metal oxide,intensify conduction and convection coefficients,letting for new heat transfer outside the coolant.Owing to their worth in numerous built-up and biomedical applications nanofluids have established thoughtfulness in modern years.Moreover,nanofluids and nanofluids constructed phase conversion materials have been acknowledged as prospective candidate for numerous energy storage and heat transport applications,still they are facing numerous experiments for example pressure drop,stability and extraordinary inflating power.Nano size particle shows a better role in obstructing nanofluid applications; however,the impact of nanoparticles size does not acquire the thoughtfulness as it deserves.The inconsistence and conflict of material provide an impression that the region is not well assumed,thus additional material essential to be prepared.In that instance,overview of nanofluids applications via nanotechnology may be incorporated into each of these technological ranges that are: drag diminutions,nanofluids in boring,magnetic fluids,heating and cooling of constructions,cooling of microchip technology and welding,astral and defense,high-control lasers,warm cylinders and thermal storing.Numerous authors scrutinized critically the applications of nanofluids and acknowledged investigation breakdowns for advance research.Additionally,experiments and forthcoming directions and applications of these liquids with diverse properties have been reviewed and presented in Refs.[12–20].In addition,Irfanet al.[21]scrutinized the properties of MHD and heat sink/source in convective flow of Maxwell nanofluid.They inspected that the curvature and Maxwell parameter spectacle conflicting performance on the velocity field while analogous impact on temperature field is being identified.Hayatet al.[22]reported the behavior of thermal radiation and chemical reaction on 3D MHD flow of nanofluid.The non-linear radiative mixed convection flow with deferred nanoparticles in melting surface was studied by Mahanteshet al.[23]Their study indicates that the melting and moving parameters diminish the drag forces or friction.The aspects of activation energy and binary chemical reaction on Williamson nanomaterial was analyzed by Hamidet al.[24]Their upshots specify that the temperature of Williamson fluid intensifies for thermophoresis parameter while the heat transport amount diminishes for reaction parameter.
The consideration of this study is to inspect the influence of stagnation point and variable conductivity in MHD flow of Maxwell nanofluid due to stretching cylinder.The aspects of heat sink/source and chemical reaction are also incorporated.Solutions of the formulated problem are attained via homotopic analysis method.Dynamic features of diverse inferential variables are presented graphically and discussed.Additionally,comparison tables are reported to authenticate the solutions of the problem in limiting sense.
2 Mathematical Formulation
We scrutinize steady 2D stagnation point flow of a Maxwell nanomaterial influenced by stretching cylinder of radiusR.Additionally,variable thermal conductivity,heat sink/source,chemical reaction are incorporated for heat and mass transport phenomena.The stretching and free stream velocities of the cylinder are assumed to be (U0z/l,U∞z/l) alongz-direction,where (U0,U∞) are the reference velocities andlthe specific length,respectively.Let us take cylindrical polar coordinates (z,r) in such a way thatz-axis goes close to axis of the cylinder andr-axis is restrained along the radial direction.Furthermore,a uniform magnetic field of lengthB0is applied alongr-direction and further assumed that the features of induced magnetic field are insignificant owing to small magretic Reynolds number (as clarified in Fig.1).Under the norm of these circumstances,the flow problem of Maxwell nanofluid is as follows
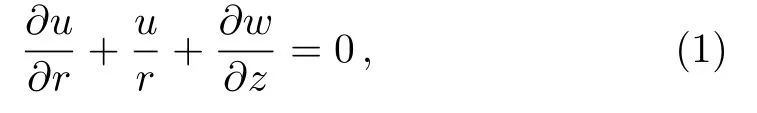
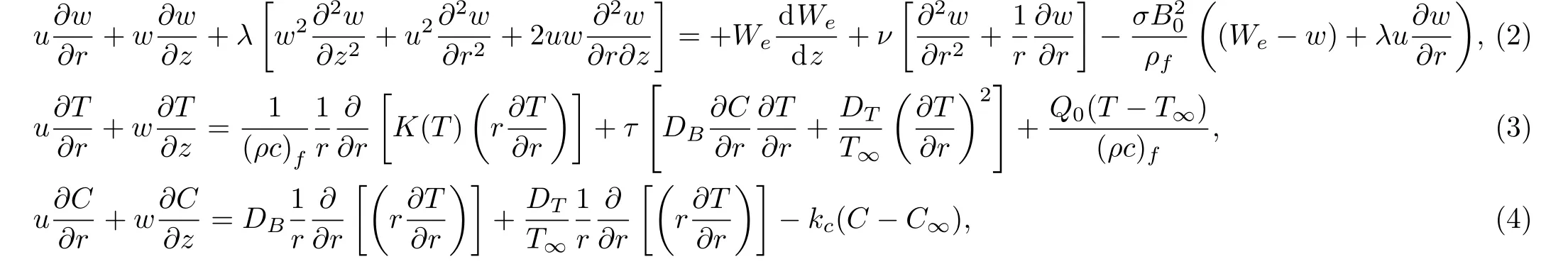
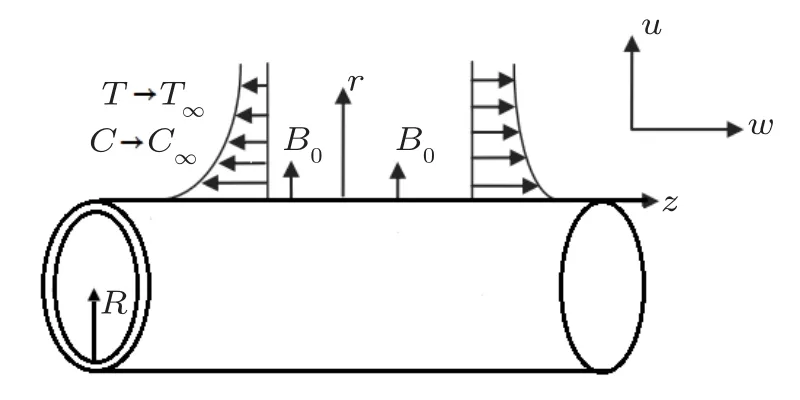
Fig.1 Geometry of coordinates system.
subject to the boundary conditions
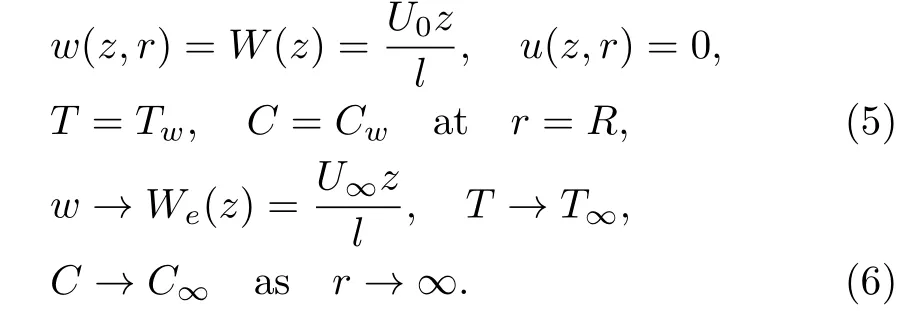
Here (u,w) denotes the velocity components in therandz-directions,respectively,νthe kinematic viscosity,λthe relaxation time,(ρf,cf) the liquid density and specific heat respectively,(T,C) the nanoliquid temperature and concentration,respectively,(DB,DT) the Brownian and thermophoresis diffusion coefficients,respectively,τthe ratio of effective heat capacity of nanoparticles material to the heat capacity of the base liquid,Q0the heat sink/source coefficient andkcthe coefficient of chemical reaction.Moreover,the variable thermal conductivityk(T) is defined as[25−27]

wherek1is the nanoliquid thermal conductivity,εthe small scale thermal conductivity parameter and ∆Tthe temperature difference between the liquid temperature of stretched surface and far away from the surface of cylinder.
2.1 Appropriate Conversions
Letting

In outlook of Eq.(7)and overhead alterations(8),Eq.(1)is satisfied automatically and Eqs.(2)–(6) compact to
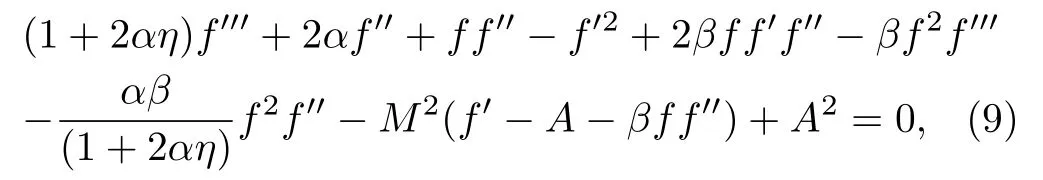


Here(α,β,A,M,Nb,Nt,δ,Pr,Le,Cr)are,respectively,the curvature parameter,Deborah number,velocities ratio parameter,magnetic parameter,Brownian motion parameter,thermophoresis parameter,heat sink/source parameter,Prandtl number,Lewis number and chemical reaction parameter and are defined as

3 Physical Quantities of Interest
The rates of heat and mass transport (Nuz,Shz) are
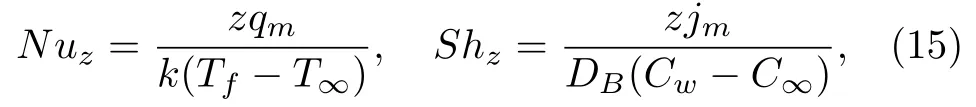
where (qm,jm),respectively,the heat and mean fluxes
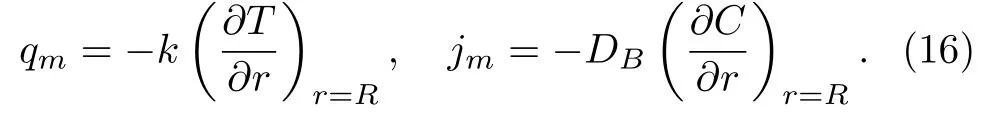
Utilizing the above equations we attain
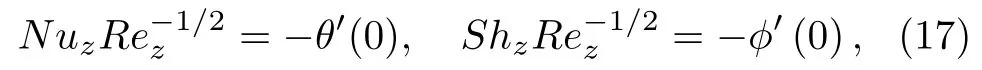
whereRez=W(z)z/νdepicts the local Reynolds number.
4 Homotopic Solutions
The homotopy analysis method provides us excessive independence and an informal approach to regulate and control the convergence constituency of the series elucidations.To scrutinize the stimulus of inferential parameters a well-organized analytical methodology,namely homotopy analysis method (HAM) has been betrothed which solves nonlinear ODEs.The initial estimates (f0,θ0,ϕ0)and linear operators (£f,£θ,£ϕ) for such scheme are defined by

with the properties
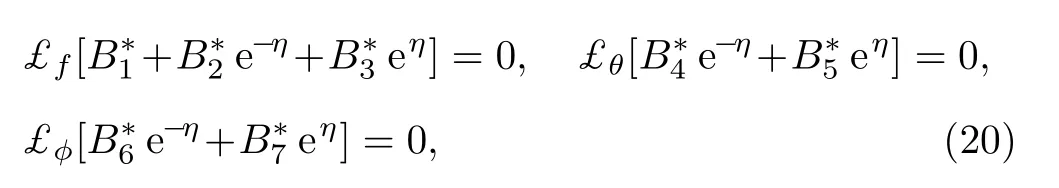
in whichB∗i(i=1–7) are the arbitrary constants.
5 Graphical Depiction and Analysis
To explore the aspect of influential variables in MHD flow of Maxwell nanofluid near the stagnation region with the inducement of chemical reaction and variable thermal conductivity the current section is structured.The graphs are intended and conferred for the velocity,temperature,concentration and reduced Nusselt number with detail.
Figure 2 portrays the aspect of stagnation pointAon the velocity componentf′(η).Remarkably,we noted thatf′(η) intensified when the value ofAexaggerates.The thickness of boundary layer progresses forA <1 as stretching rate exceeds the free stream amount.Moreover,the straining motion close to the stagnation area rises which intensifies the acceleration of the external stream and declines the thickness of the boundary layer with rise inA.However,it falloffs whenA >1,i.e.the amount of free stream velocity is higher when correlated with amount of stretching velocity.There is no development of boundary layer whenA= 1 as the stretching and free stream velocities are equivalent as noted via Fig.2.Furthermore,we concluded that the conflicting performance is being acknowledged for bothA>1 andA<1.

Fig.2 (Color online) Influence ofA onf′(η).
An escalation in thermal conductivity parameterεand Deborah numberβhave conflicting behavior onθ(η) as portrayed in Figs.3(a),3(b).Caused by the enormous quantity of heat transfer from surface to material the liquid thermal conductivity of Maxwell liquid intensifies with the temperature field boost up.Moreover,enrichment inβspectacles divergent performance in temperature and decays the temperature of Maxwell liquid as shown in Fig.3(b).As collision between liquid components boost up whenβintensifies resulting in a decay the liquid temperature.To picture the stimulus of heat sink/source for heat transfer feature,Figs.4(a),4(b)are strategized.It is remarked that the temperature field falloff forδ >0 and enriched forδ >0.Furthermore,in instance of heat source parameter results in heat to be delivered to the liquid owing to which the liquid temperature rise while heat is fascinated in instance of heat sink parameter due to which the temperature of liquid decline.Thus,a differing behavior is being commented forδ <0 andδ >0.Figures 5(a),5(b)are delineated to picture the influence of nanoparticlesNbandNtonθ(η).An augmenting enactment of temperature field is observed for bothNbandNt.Physically,higherNbhas advancedDBwhich improves the temperature of nanoliquid becauseNbhas direct relation withDB.Additionally,the theromophoretic force intensifies forNt.Thus,the liquid particles transport rapidly from hot to cold area.Therefore,the nanoliquid temperature heightens.Figures 6(a),6(b) report the graphical sketch of magnetic parameterMand Prandtl numberPron temperature fieldθ(η).These displays portray that the temperature rises whenMintensifies while it declines forPr.The thermal thickness of thermal layer and temperature rise for boosting the value ofM.The conflicting enactment is being acknowledged forPr.The progressive value ofPrindicates to the lesser thermal diffusivity and decreases the aptitude of energy transfer,which spectacles the reduction of temperature of Maxwell liquid.Furthermore,the relative thickness of velocity and thermal thickness of boundary layers is controlled byPr.
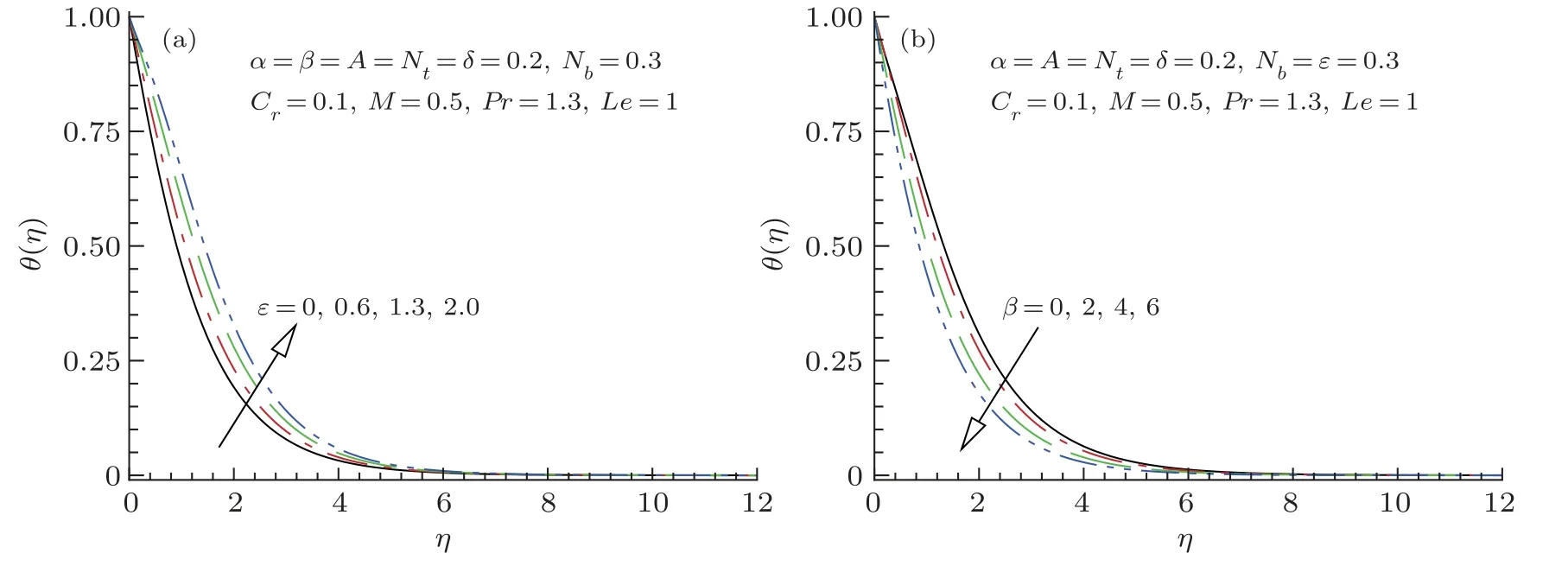
Fig.3 (Color online) Influence ofε (a) andβ (b) onθ(η).

Fig.4 (Color online)Influence of(δ <0)(a)and(δ >0)(b)onθ(η).

Fig.5 (Color online) Influence ofNb (a) andNt (b) onθ(η).

Fig.6 (Color online)Influence ofM (a)andPr (b)onθ(η).
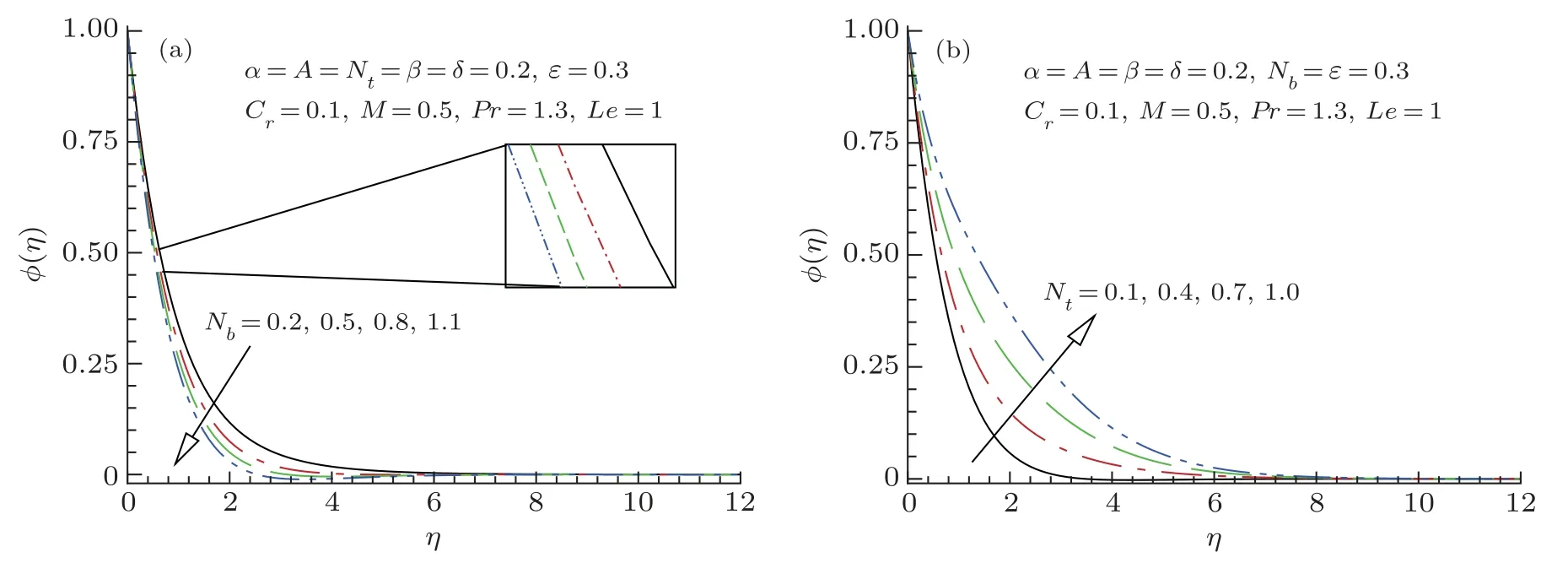
Fig.7 (Color online) Influence ofNb (a) andNt (b) onϕ(η).
The aspects of diverse values of BrownianNband thermophroticNtnanoparticles on concentration fieldϕ(η)are described via Figs.7(a),7(b).TheNbis diminishing function of concentration of Maxwell liquid,but its augmenting function ofNt.The behavior ofNboccurred becauseNbintensifies the unsystematic gesture of the liquid particles which generates extra heat and diminish the concentration of Maxwell liquid.Moreover,the liquid thermal conductivity enhances in the existence of nanoparticles.HigherNtcontributes intensification in the liquid thermal conductivity which displays higher concentration and allied thickness.Hence,we established that both parametersNbandNthave opposite trend onϕ(η).Figures 8(a),8(b) illustrate the features of chemical reactionCr(Cr<0 andCr>0) on concentration fieldϕ(η).These plots specify a reverse propensity on concentration field.The larger destructive chemical reaction parameterCr>0 decreases the concentration field and an opposite behavior is reported for generative chemical reactionCr<0 and forCr=0 no reaction occurs.Physically,the higher value ofCr>0 reasons to decline in the chemical molecular diffusivity,which decays the concentration field.Hence,conflicting behavior is noted for both cases on concentration field.
To pinpoint the performance ofNt,Nb,Prandεon heat transfer amount,Figs.9(a),9(b) and 10(a),10(b)are drafted.These plans exhibit similar behavior forNt,Nbandεwhereas conflicting trend is being reported forPr.The heat transfer amount declines forNt,Nbandεbut enhances forPr.

Fig.8 (Color online) Influence of (Cr<0) (a) and (Cr>0) (b) onϕ(η).

Fig.9 (Color online)Behavior ofNt (a)andNb (b)on−θ′(0).
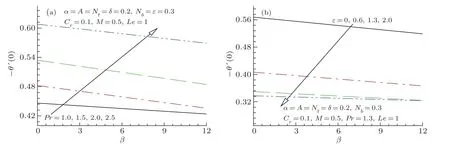
Fig.10 (Color online)Behavior ofPr(a)andε(b)on−θ′(0).
Table 1 establishes the numerical value of−f′′(0) associated with that of Refs.[28–29].Additionally,Tables 2 and 3 spectacle the comparison values ofPrfor−θ′(0)with previous studies.A remarkable settlement between these upshots is being detected in these tables.Hence,we are assured that the latest consequences are very precise.
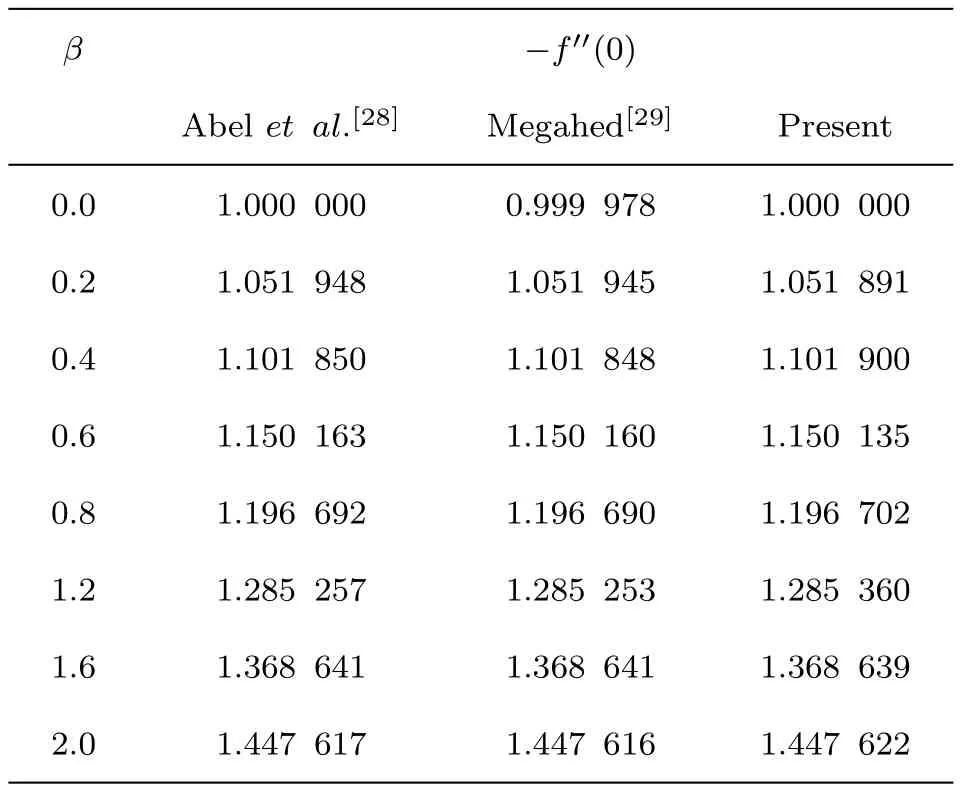
Table1 Numerical values of−f′′(0) forβ in limiting cases whenα=M =A=0.

Table2 Numerical values of−θ′(0) whenα=β =δ =A=M =Nt =ε=Cr =0 andNb→0.

Table3 Numerical values of−θ′(0) whenα=β =δ =A=M =Nt =ε=Cr =0 andNb→0.
6 Closing Remarks
To disclose the aspects of the stagnation point flow in MHD Maxwell nanofluid caused by stretching cylinder this section has been established.For the solution of the problem homotpy analysis method (HAM) has been consumed.The crucial outlooks of this study are as follows;
•ForA<1 the thickness of the momentum boundary layer enriched; however,forn >1 it diminished whenAincreases.
•The larger magnetic parameterMenhanced the temperature of Maxwell fluid while the Deborah numberβdecayed the temperature field.
•On temperature field quite conflicted behaviors were being detected for heat sink/source parameterδ.
•The variable thermal conductivityεincreased nanoliquid temperature field.
•A similar trend was being acknowledged for thermophrotic parameterNton temperature and concentration of Maxwell nanofluid.
•Local Nusselt number enhanced for increasing Prandtl numberPrand declined for increasing thermophrotic parameterNtand variable conductivityε.
Acknowledgment
The authors extend their appreciation to the Deanship of Scientific Research at King Khalid University for funding this work through Research Groups Program under Grant number (R.G.P2./26/40).
Conflict of interest
Authors have no conflict of interest.
 Communications in Theoretical Physics2019年12期
Communications in Theoretical Physics2019年12期
- Communications in Theoretical Physics的其它文章
- An Optimal Analysis for 3D Flow of Prandtl Nanofluid with Convectively Heated Surface
- The Effects of Wettability on Primary Vortex and Secondary Flow in Three-Dimensional Rotating Fluid∗
- An Improved Heterogeneous Mean-Field Theory for the Ising Model on Complex Networks∗
- Relativistic Self-Focusing of Hermite-cosh-Gaussian Laser Beam in Magnetoplasma with Exponential Plasma Density Ramp
- Periodic Orbits Around Kerr Sen Black Holes∗
- Parameterized Post-Post-Newtonian Light Propagation in the Field of One Spherically-Symmetric Body∗
