基于颗粒缩放的小麦粉离散元参数标定

李永祥，李飞翔，徐雪萌，申长璞，孟坤鹏，陈 静，常东涛

基于颗粒缩放的小麦粉离散元参数标定

李永祥1，李飞翔1，徐雪萌1※，申长璞1，孟坤鹏1，陈 静1，常东涛2

（1. 河南工业大学机电工程学院，郑州 450001；2. 河南金谷实业有限公司，郑州 450001）

为获得小麦粉离散元仿真精确的接触参数，将不规则形状的小麦粉简化成软质球形颗粒，利用颗粒接触缩放原理和量纲分析进行颗粒缩放，将平均粒径0.212 mm的小麦粉放大至1.2 mm，选择“Hertz–Mindlin with JKR”接触模型，利用休止角对接触参数进行标定。首先通过Plackett-Burman 试验筛选出对休止角影响显著的参数：表面能JKR（Johnson Kendall Roberts）、小麦粉-小麦粉滚动摩擦系数、小麦粉-不锈钢静摩擦系数；然后根据Box-Behnken 试验建立并优化休止角与显著性参数的二阶回归模型，得到显著性参数的最佳组合为JKR为0.157、小麦粉-小麦粉滚动摩擦系数为0.25、小麦粉-不锈钢静摩擦系数为0.58；最后用标定参数仿真所得休止角大小与真实试验值进行对比，二者相对误差为0.61%。结果表明标定所得的接触参数可用于小麦粉放大颗粒的离散元仿真，为定量供送螺杆的设计提供参考。

农产品；颗粒尺寸；离散元；参数标定；休止角

0 引 言

自动包装生产线上小麦粉的输送与计量大多采用螺旋输送装置，螺旋输送装置的合理设计是提高小麦粉输送效率及包装精度的关键因素。利用离散元法（DEM，discrete element method）全面、系统研究粉体与螺杆间的相互作用机理及粉体流的运动状态，可以优化螺杆参数，提高小麦粉包装的速度及精度，同时也可以提高研发效率、降低研发成本。Gao等建立了螺旋集料装置工作过程的离散元数值模型并进行仿真研究，获得了螺旋集料装置设计所需的最优参数，为螺旋集料装置的结构改进提供了参考[1]；Mazor等采用离散元(DEM)和有限元(FEM，finite element method)相结合的方法，通过分析粉末在给料区和压实区的运动状态，得出了粉体密度参数的变化趋势曲线[2]；Sun等通过对螺旋进料头的离散元仿真，提出了不同摩擦系数下进料头的曲线方程并得出计算输送量的公式，对提高螺旋进料头研发的效率具有一定意义[3]。离散元仿真所需参数众多，一般通过直接测量和虚拟标定来进行获得，在此研究领域，国内外学者基于JKR Cohesion模型对含湿物料的参数标定开展了大量研究[4-8]，但对于小麦粉、淀粉等颗粒较小物料的离散元仿真及参数标定的相关研究还比较少。

小麦粉粒径较小，螺旋输送料筒内物料颗粒的数量达到上千万甚至数十亿，普通计算机能力有限而不能有效模拟，颗粒缩放法是目前较为可行的处理方式，已广泛应用于工程研究中，该方法将原系统中颗粒放大，降低了模型中的离散单元数，使原物理模型问题能在合理有效的时间内解决。如任建莉等基于颗粒缩放理论，通过对垂直螺旋输送中铸铁煤粉颗粒运动的离散元仿真，验证了颗粒缩放法的准确性[9]；Sakai等建立了粗粒模型并对其在流化床中进行数值模拟，得出了粗粒模型能较精确模拟原始粒子行为的结论[10]；Weinhart等为表明对高度局部化系统中空间粗粒宽度及时间间隔选择的重要性，以粗粒模型对筒仓进行了离散元仿真[11]。本研究利用缩放理论，以小麦粉休止角作为响应值，通过Plackett-Burman、最陡爬坡以及Box-Behnken试验对放大颗粒的离散元仿真接触参数进行标定，得到接触参数的最佳组合，以期为小麦粉、淀粉等其他粉体的离散元模拟提供参考。

1 材料与方法

1.1 试验材料获取

原材料：郑州海嘉食品有限公司生产的普通粉：含水率13.5%，蛋白质11.5%，灰分0.51%；将原材料通过70目的标准孔筛制得试验所用小麦粉，其平均粒径为0.212 mm。

1.2 颗粒缩放原理

1.2.1 量纲分析

为使离散元能够合理有效仿真，通过调整离散元仿真参数，使缩放粒子仿真结果尽可能表现出与原系统粒子相同的动态和静态特性，以减小由于粒子缩放而造成的模拟误差。Feng等采用简单方法，建立了原系统物理模型和缩放模型之间单个物理量间的比例因子[12]。

式中为轴向力，N；为杨氏模量，Pa；为横截面积，m2；为轴向位移，m。

式中为速度，m/s；为长度，m；为时间，s。

对于黏合弹性颗粒接触模型来说，2个黏着弹性球间接触力可表示为

由量纲分析结果可知，缩放模型密度保持不变，接触刚度与颗粒尺寸成线性关系，不是固定常数，参数弹性模量的设置不是固定的原始数值，而是随变径缩放而被缩放，根据参数范围，取其较大值。

1.2.2 缩放接触原理

Stefan基于原始系统和缩放系统中相同的转动动能，对线性弹性模型进行分析，根据牛顿运动定律，颗粒间法向重叠的微分方程可表示为[13]

式中m为有效质量，kg；k为颗粒刚度，N/m；c为阻尼系数，kg/s；δ为重叠量，m。

式中为颗粒密度，kg/m3；为颗粒粒度比，R为原系统颗粒半径，m。

式中R为有效半径，m；R为缩放系统颗粒半径，m。

将（8）、（9）2式用无量纲量转换。

式中*为无量纲数，δ为重叠量，m；为时间，s；0为速度，m/s。

代入（9）式可得

式中k为颗粒刚度，N/m；c为阻尼系数，kg/s。

简化可得

式（12）中系数可用以下无量纲数表示为

范德华力是细颗粒黏附的主要来源。在细颗粒离散元建模中，一般采用理论黏着弹性模型来表示范德华力[14]。对于JKR模型，将范德华力与颗粒半径关联：

式中为每单位接触面积的表面能，J/m2。

Subhash等给出了具有随机各向同性物料的硬质单分散球体系拉伸强度与颗粒间接触力的关系[14]：

这表明颗粒间接触力与缩放粒子半径的平方成正比，随着粒子半径的增加，单对颗粒间接触表面积也相对增加。由于粘附力与颗粒间接触面积有关，而接触面积与颗粒半径的平方成比例，表明了粘附力与颗粒半径二次方的比例关系。参数JKR设置中，JKR随缩放比例变动，没有特定的参考数值，根据其范围，通过标定确定。为了尽量减小仿真误差及获得合理有效的仿真时间，缩放比例根据相关文献[15-16]，本文将小麦粉颗粒放大近6倍进行仿真模拟。

2 参数标定过程

2.1 物理模型

试验参照GB/T 16913.5-1997国家标准，并结合已有文献对休止角的相关研究[17-21]，采用注入法测量小麦粉休止角，测量装置如图1所示，漏斗下口内径为5 mm，锥度为60o，圆柱底盘直径为80 mm，漏斗下端口距圆柱底盘上表面距离75 mm。测量时，将所制备的小麦粉缓慢倒入漏斗中，使用玻璃棒轻微搅动，防止小麦粉颗粒堵住漏斗出口，待圆柱底盘溢出一定数量的小麦粉后，停止向漏斗添加小麦粉，待颗粒堆积高度不再发生变化，用钢尺测出底盘上小麦粉的堆积高度。根据公式（16）计算小麦粉休止角，重复5次取其平均值，测得小麦粉休止角为52.37o。

2.2 仿真模型

2.2.1 仿真参数

结合国内外文献对粉体颗粒与不锈钢离散元仿真参数的设置[22-25]及软件内置 GEMM 数据库，本研究中各仿真参数的变化范围如表1所示。结合粉体仿真相关文献[26-29]以及颗粒缩放理论规则，模拟所需小麦粉本征参数设定为：密度1 960 kg/m3、泊松比0.25、小麦粉剪切模量6.0´107Pa。材料的接触参数随材料密度、形状、粒径等不同变化较大，无法通过查阅物性手册或文献资料获取，采用虚拟试验进行标定。

2.2.2 仿真模型

仿真参照GB/T11986-98《表面活性剂粉体和颗粒休止角的测量》标准，采用注入法，漏斗出口内径为10 mm，接收圆柱底面直径=100 mm，漏斗下端口距圆柱底盘上表面距离75 mm，放大颗粒粒径设置为=1.2 mm，由于模拟条件及时间限制，仿真采用球形颗粒[30-32]。仿真模型如图2所示，颗粒生成方式为Dynamic，生成速率设为2 000个/s，生成数量设为不限，仿真时间设为20 s，待圆柱底面接收的颗粒处于溢出状态，将生成速率设为0个/s，继续仿真，待漏斗中颗粒落完后，采用软件后处理中记录颗粒位置的功能，记录颗粒堆积高度随时间的变化趋势，导出数据，找到处于相对静止下的高度值，采用自带的量角器工具，测量休止角。

表1 离散元仿真参数表

图2 小麦粉颗粒堆积的模拟仿真

2.3 仿真参数的响应面设计

2.3.1 Plackett-Burman试验

Plackett-Burman试验通过考察目标响应与各因子间关系，比较各个因子2水平间的差异来确定因子显著性。本文Plackett-Burman 设计以小麦粉休止角为响应值，对仿真接触参数的显著性进行筛选。低水平设定为最初原始水平，高水平设为低水平的2倍，试验参数如表2所示。

表2 Plackett-Burman试验参数列表

Plackett-Burman设计及结果如表3所示，利用Design Expert软件对该结果进行方差分析，得到各个接触参数的显著性如表4所示。由表4可知，JKR、小麦粉-小麦粉滚动摩擦系数、小麦粉-不锈钢静摩擦系数的<0.01，对放大颗粒休止角的影响极其显著；小麦粉-不锈钢滚动摩擦系数的<0.05，对放大颗粒的休止角影响显著；而其余参数>0.05，对放大颗粒的休止角影响极小。为方便后续试验，在最陡爬坡以及 Box-Behnken 试验中只考虑这3个影响极其显著（<0.01）的参数。其余参数结合相关文献[30-32]取值为（小麦粉-小麦粉恢复系数0.2、小麦粉-小麦粉静摩擦系数0.6、小麦粉-不锈钢恢复系数0.2、小麦粉-不锈钢滚动摩擦系数0.25）来进行最陡爬坡以及响应面试验设计。

表3 Plackett-Burman试验设计及结果

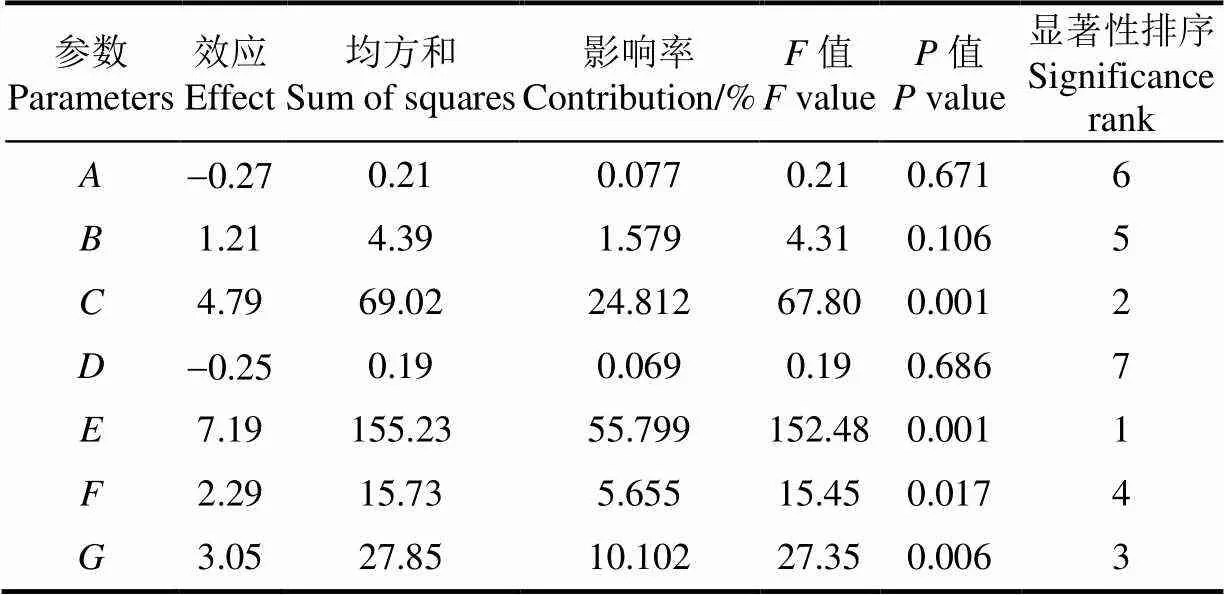
表4 Plackett-Burman试验参数显著性分析

2.3.2 最陡爬坡试验

Plackett-Burman 试验后，根据筛选的显著性参数，进行最陡爬坡试验，以便能快速进入到最优值的附近区域。最陡爬坡试验从PB试验中心点开始，根据PB试验所得的回归系数来确定爬坡步长，为能尽快逼近最优值，爬坡步长通常取较大值。本爬坡试验选定步长以及结果如表5所示。根据表5结果可知，在4号水平休止角相对误差最小，由3号到5号水平相对误差由大变小再变大，由此选取4号水平为中心点，3号、5号水平为低、高水平进行后续响应面设计。

表5 最陡爬坡试验设计及结果

2.3.3 Box-Behnken试验及回归模型

根据最陡爬坡试验结果及响应面设计原理，选取显著性参数的低、中、高3水平进行试验设计，试验选3个中心点对误差进行评估。Box-Behnken试验结果如表6所示，应用Design-Expert建立3个显著性参数与休止角的二阶回归方程为

表6 Box-Behnken试验设计及结果

Box-Behnken试验模型方差分析结果如表7所示，根据表7结果可知，该拟合模型<0.0001；JKR 表面能()、小麦粉颗粒间的滚动摩擦系数()、小麦粉-不锈钢的静摩擦系数（）、JKR表面能-滚动摩擦系数（Í）以及JKR表面能的二次项（2）值都<0.01；JKR表面能-静摩擦系数（Í）<0.05，说明各个参数对休止角的影响显著，表明了回归模型的有效性。失拟项=0.5405>0.05，表明模型良好，没有弯曲失拟现象发生。试验中变异系数CV=0.74%，说明试验有较高的可靠性。决定系数2=0.994；校正决定系数2adj=0.984；预测决定系数2pre=0.943；三值都>0.9，表明模型能够真实的反应实际情况。试验精密度Adep Precision=33.455，说明模型具有良好的精确度。

表7 Box-Behnken试验设计二次多项式模型方差分析

根据表7结果，在保证模型良好前提下，剔除对休止角影响不显著的项（Í、2、2），优化模型后的方差分析结果如表8所示，失拟项=0.511 9；变异系数CV=0.80%；决定系数2=0.989；校正决定系数2adj=0.981；预测决定系数2pre=0.939；试验精密度Adep Precision=37.684。可知，模型拟合性，可靠性以及精确性良好，较优化前有了一定改善，优化后回归方程为

表8 Box-Behnken试验优化回归模型方差分析

2.3.4 回归模型交互效应分析

根据优化回归模型方差分析结果，可知JKR表面能-滚动摩擦系数（Í）以及JKR表面能-静摩擦系数（Í）的<0.01，这2个交互项对小麦粉休止角影响极其显著。在小麦粉-不锈钢静摩擦系数（）为0.22以及颗粒间滚动摩擦系数（）为0.5的2种情况下，应用Design-Expert软件对JKR表面能-滚动摩擦系数（Í）以及JKR表面能-静摩擦系数（Í）交互作用的三维响应曲面进行绘制，如图3所示，可以直观的反应交互项对休止角的影响。由Í曲面可知，相对于颗粒间滚动摩擦系数（），JKR表面能（）的效应面曲线比较陡，表明其对休止角影响较为显著。由Í曲面可知，相对于JKR表面能（），小麦粉-不锈钢静摩擦系数（）的效应面曲线比较陡，表明其对休止角影响较为显著。

图3 HJ与HK的交互效应图

3 最佳参数组合的确定及仿真验证

应用Design Expert 软件以小麦粉实际休止角为目标，对优化后的回归方程进行寻优求解可知，欲使仿真与试验所得休止角误差最小，则JKR表面能为0.157，小麦粉-小麦粉滚动摩擦系数为0.25，小麦粉-不锈钢静摩擦系数为0.58。用最佳参数组合进行休止角仿真试验，仿真与物理试验的对比如图4所示。仿真试验所得休止角为52.69o，与实际值52.37o的误差为0.61%，表明仿真结果与真实试验值无显著性差异。

图4 仿真试验与物理试验对比

4 结 论

1）采用颗粒缩放法将粒径0.212 mm的小麦粉颗粒放大至1.2 mm，基于离散元中JKR模型对放大颗粒的接触参数进行标定。由 Plackett-Burman 试验筛选出对小麦粉放大颗粒休止角影响显著的因素为表面能JKR、小麦粉-小麦粉滚动摩擦系数及小麦粉-不锈钢静摩擦系数。

2）根据Box-Behnken试验结果，建立并优化3个显著性参数与休止角间的二次回归模型，根据优化模型方差分析的结果可知，除了3个显著性参数（JKR表面能、小麦粉-小麦粉滚动静摩擦系数及小麦粉-不锈钢静摩擦系数）的一次项外，交互项JKR表面能-滚动摩擦系数、JKR表面能-静摩擦系数以及JKR表面能的二次项对小麦粉放大颗粒休止角影响也极其显著。

3）以小麦粉实际休止角为目标，对回归方程进行寻优求解，得到显著性参数的最佳组合为小麦粉-小麦粉滚动摩擦系数为0.25、小麦粉-不锈钢静摩擦系数为0.58、表面能JKR为0.157，进行试验对比，仿真所得休止角与实际所得休止角无显著性差异（>0.05），表明应用响应面分析标定离散元仿真参数的可行性。

4）以试验设计所得的最佳参数组合来进行休止仿真试验，测得仿真试验的休止角为52.69°，与试验所测的小麦粉休止角52.37°，两者误差为0.61%，模拟值与试验测试值无显著差异。结果表明，基于颗粒缩放理论标定所得到的接触参数可用于小麦粉离散元仿真。

[1] Gao K D, Xu W B, Zhang X, et al. Analysis of spiral aggregate device on the sump cleaning machine by discrete element method [J]. International Journal of Simulation Modelling (IJSIMM), 2018, 17(4): 643－656.

[2] Mazor A, Orefice L, Michrafy A, et al. A combined DEM & FEM approach for modelling roll compaction process[J]. Powder Technology, 2018, 337: 3－16.

[3] Sun Xiaoxia, Meng Wenjun, Yuan Yuan. Feeding head of relative rotary based on EDEM+ FLUENT[J]. Advances in Mech­an­ic­al Engineering, 2018, 10(7): 1－13.

[4] 罗帅，袁巧霞，杨龙元. 基于JKR粘结模型的蚯蚓粪基质离散元法参数标定[J]. 农业机械学报，2018，49(4)：343－350. Luo Shuai, Yuan Qiaoxia, Yang Longyuan.Parameters calibration of vermicomposting nursery substrate with discrete element method based on JKR contact model [J]. Transactions of the Chinese Society for Agricultural Machinery, 2018, 49(4): 343－350. (in Chinese with English abstract)

[5] 袁全春，徐丽明，邢洁洁，等. 机施有机肥散体颗粒离散元模型参数标定[J]. 农业工程学报，2018，34(18): 21－27. Yuan Quanchun, Xu Liming, Xing Jiejie, et al. Parameter calibration of discrete element model of organic fertilizer particles for mechanical fertilization [J]. Transactions of the Chinese Society for Agricultural Engineering (Transactions of the CSAE), 2018, 34(18): 21－27. (in Chinese with English abstract)

[6] 王宪良，胡红，王庆杰，等. 基于离散元的土壤模型参数标定方法[J]. 农业机械学报，2017，48(12): 78－85. Wang Xianliang, Hu Hong, Wang Qingjie, et al.Calibration method of soil contact characteristic parameters based on DEM theory [J]. Transactions of the Chinese Society for Agricultural Machinery, 2017, 48(12): 78－85. (in Chinese with English abstract)

[7] Alizadeh, Mohammadreza, Ghadiri, et al. A methodology for calibration of DEM input parameters in simulation of segregation of powder mixtures: a special focus on adhesion[J]. Powder Technology, 2018, 339: 789－800.

[8] Figueroa I, LI H, Mccarthy Joseph. Predicting the impact of adhesive forces on particle mixing and segregation[J]. Powder Technology, 2009, 195.3: 203－212.

[9] 任建莉，周龙海，韩龙，等. 基于颗粒缩放理论的垂直螺旋输送离散模拟[J]. 过程工程学报，2017，17(5): 936－943.Ren Jianli, Zhou Longhai, Han Long, et al. Discrete simulation of vertical screw conveyor based on particle scaling theory [J]. Chinese Journal of Process Engineering, 2017, 17(5): 936－943. (in Chinese with English abstract)

[10] Sakai M, Takahashi H, Pain C, et al. Study on a large-scale discrete element model for fine particles in a fluidized bed[J]. Advanced Powder Technology, 2012, 23(5): 673－681.

[11] Weinhart T, Labra C, Luding S, et al. Influence of coarse-graining parameters on the analysis of DEM simulations of silo flow[J]. Powder Technology, 2016, 293: 138－148.

[12] Feng Y T, Owen D R J. Discrete element modelling of large scale particle systems-I: Exact scaling laws[J]. Computational Particle Mechanics, 2014, 1(2): 159－168.

[13] Radl S, Radeke C, Khinast J G, et al. Parcel-based approach for the simulation of gas-particle flows[C]. 8th International Conference on CFD in Oil & Gas, Metallurgical and Process Industries, Trondheim. 2011.

[14] Thakur SC, Ooi JY, Ahmadian H. Scaling of discrete element model parameters for cohesionless and cohesive solid[J]. Powder Technology, 2016, 293: 130－137.

[15] 周龙海. 垂直螺旋输送的EDEM仿真与实验研究[D]. 杭州: 浙江工业大学，2017. Zhou Longhai. EDEM Simulation and Experiment of Vertical Screw Conveyoring [D].Hangzhou: Zhejiang University of Technology, 2017. (in Chinese with English abstract)

[16] Sakai M, Abe M, Shigeto Y, et al. Verification and validation of a coarse grain model of the DEM in a bubbling fluidized bed[J]. Chemical Engineering Journal, 2014, 244: 33－43.

[17] 彭飞，王红英，方芳，等. 基于注入截面法的颗粒饲料离散元模型参数标定[J]. 农业机械学报，2018，49(4): 140－147.

Peng Fei, Wang Hongying, Fang Fang, et al. Parameter calibration of discrete element model parameters for pellet feed based on injected section method [J]. Transactions of the Chinese Society for Agricultural Machinery, 2018, 49(4): 140－147. (in Chinese with English abstract)

[18] 刘凡一，张舰，李博，等. 基于堆积试验的小麦离散元参数分析及标定[J]. 农业工程学报，2016，32(12): 247－253. Liu Fanyi, Zhang Jian, Li Bo, et al. Calibration of parameters of wheat required in discrete element method simulation based on repose angle of particle heap [J]. Transactions of the Chinese Society for Agricultural Engineering (Transactions of the CSAE), 2016, 32(12): 247－253. (in Chinese with English abstract)

[19] 贺一鸣，向伟，吴明亮，等. 基于堆积试验的壤土离散元参数的标定[J]. 湖南农业大学学报：自然科学版，2018，44(2): 216－220. He Yiming, Xiang Wei, Wu Mingliang, et al. Parameters calibration of loam soil for discrete element simulation based on the repose angle of particle heap[J]. Journal of Hunan Agricultural University: Natural Science Edition, 2018, 44(2): 216－220. (in Chinese with English abstract)

[20] 张锐，韩佃雷，吉巧丽，等. 离散元模拟中沙土参数标定方法研究[J]. 农业机械学报，2017，48(3): 49－56. Zhang Rui, Han Yulei, Ji Qiaoli, et al.Calibration methods of sandy soil parameters in simulation of discrete element method [J]. Transactions of the Chinese Society for Agricultural Machinery, 2017, 48(3): 49-56. (in Chinese with English abstract)

[21] 贾富国，韩燕龙，刘扬，等. 稻谷颗粒物料堆积角模拟预测方法[J]. 农业工程学报，2014，30(11): 254－260. Jia Fuguo, Han Yanlong, Liu Yang, et al. Simulation prediction method of repose angle for rice particle materials [J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2014, 30(11): 254－260. (in Chinese with English abstract)

[22] 张西良，张建，李萍萍，等. 粉体物料流动性仿真分析[J].农业机械学报，2008(8): 196－198.

[23] 郝友莉. 粉体包装螺旋式密实输送研究[D]. 无锡：江南大学，2017. Hao Youli. Research on Densifying Transportation of Spiral Powder Packaging Machine [D]. Wuxi：Jiangnan University, 2017. (in Chinese with English abstract)

[24] 张帅，单忠德，张杰. 基于离散元方法的型砂流动性仿真研究[J].铸造技术,2016,37(2):288－291. Zhang Shuai, Shan Zhongde, Zhang Jie.Simulation of self-hardening resin sand mobility based on DEM [J]. Foundry Technology, 2016, 37(2): 288－291. (in Chinese with English abstract)

[25] 张建. 粉体定量加料器及应用研究[D]. 镇江:江苏大学，2008. Zhang Jian. Powder Dosing Devices and its Application [D]. Zhenjiang: Jiangsu University, 2008. (in Chinese with English abstract)

[26] 李明菲. 不同热处理方式对小麦粉特性影响研究[D].郑州: 河南工业大学，2016. Li Mingfei. Effect of Different Heat Treatment Methods on Wheat Flour Characteristics[D]. Zhengzhou: Henan University of Technology, 2016. (in Chinese with English abstract)

[27] 王崇崇. 粒度对小麦粉及面条品质特性的影响机理研究[D]. 郑州: 河南工业大学, 2018. Wang Chongchong. Study on the Effects of Different Heat Treatment on Wheat Flour Characteristics [D]. Zhengzhou: Henan University of Technology, 2018. (in Chinese with English abstract)

[28] Chichti E. Multi-scale Approach for Understanding the Link between Triticum Aestivum Grain Texture and Milling Behaviour: Focus on the Endosperm Microstructure and Local Nano-mechanical Properties[D]. Montpellie: SupAgro, 2013.

[29] Kumar S, Saini C S. Study of various characteristics of composite flour prepared from the blend of wheat flour and gorgon nut flour[J]. International Journal of Agriculture, Environment and Biotechnology, 2016, 9(4): 679－689.

[30] 石林榕，赵武云，孙伟. 基于离散元的西北旱区农田土壤颗粒接触模型和参数标定[J]. 农业工程学报，2017，33(21): 181－187.Shi Linwei, Zhao Wuyun, Sun Wei.Parameter calibration of soil particles contact model of farmland soil in northwest arid region based on discrete element method[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2017, 33(21): 181－187. (in Chinese with English abstract)

[31] Mio H, Akashi M, Shimosaka A, et al. Speed-up of computing time for numerical analysis of particle charging process by using discrete element method[J]. Chemical Engineering Science, 2009, 64(5): 1019－1026.

[32] Weinhart T, Hartkamp R, Thornton A R, et al. Coarse-grained local and objective continuum description of three-dimensional granular flows down an inclined surface[J]. Physics of Fluids, 2013, 25(7): 1－26.

Parameter calibration of wheat flour for discrete element method simulation based on particle scaling

Li Yongxiang1, Li Feixiang1, Xu Xuemeng1※, Shen Changpu1, Meng Kunpeng1, Chen Jing1, Chang Dongtao2

(1.,,450001,; 2..,450001)

In order to obtain the precise parameters for the wheat flour discrete element simulation, the actual repose angle of wheat flour was firstly measured by injection method. The experimental material was ordinary wheat flour, which average particle diameter is 0.212 mm and went through a 70-mesh standard sieve. Refer to GB 16913.5-1997, the inner diameter of the used funnel was 5 mm, the taper was 60°, and the cylindrical chassis was 80 mm in diameter. The result indicated the repose angle of the wheat flour was 52.37°, which was average value of five experiments. The irregular wheat flour was simplified into soft spherical particles, and then those particles with the size of 0.212 mm were enlarged to 1.2 mm for simulation thanks to the particle scaling and dimensional analysis, during those analyses, the3D modeling and simulation were finished by SolidWorks and EDEM software respectively. Considering the bonding characteristics between wheat flour particles, the “Hertz-Mindlin with JKR” contact model was selected to calibrate the contact parameters of wheat flour for discrete element simulation with the repose angle as a reference. Then, through the design-expert software, the parameters that have significant influence on the repose angle of wheat flour by Plackett-Burman test design are surface energy JKR, the rolling friction coefficient for wheat flour-wheat flour, the static friction coefficient for wheat flour-stainless steel. According to the significance parameters designed and screened by the Plackett-Burman test, the steepest ascent test was carried out so that it could be quickly close to the optimal value. The steepest ascent test was stared at the center of the Plackett-Burman test and the step size was determined by the regression coefficients obtained from the test. The Box-Behnken test was then carried out by selecting the low, medium and high levels of the significant parameters according to the results of steepest ascent test and the design principle of response surface, and then the three mediate points were selected to evaluate the errors. At last, the quadratic polynomial model for the repose angle and the significant parameters was successfully established and optimized by the Box-Behnken test. The analysis of variance (ANOVA) of the quadratic polynomial model showed that this model was significant and the lack-of-fit term was non-significant, which means the model can be applied to determine whether the parameters combination is the best. However, some terms in the quadratic polynomial model were non-significant. Therefore, a modified regression model was established by deleting those non-significant terms. The ANOVA of the modified model showed all of the terms were desirable, and the first-order term of those 3 significant parameters, the interactive term of the wheat flour-wheat flour static friction coefficient and JKR surface energy, and the interactive term of the wheat flour-wheat flour rolling friction coefficient and JKR surface energy had a significant effect on the repose angle. The best combination of the significant parameters could be achieved when the JKR value was 0.157, the rolling friction coefficient of wheat flour-wheat flour was 0.25, and the static friction coefficient of wheat flour-stainless steel was 0.58. Finally, the rest simulation test was carried out with the optimal combination of parameters obtained from the experiments, which showed that the repose angle of the simulation test was 52.69°, the error of the repose angle measured by the test was 0.61%, and there was no significant difference between the simulation results and the actual test values. In conclusion, the contact parameters obtained based on the particle scaling calibration can be used for wheat flour discrete element simulation which was shown by the experimental results.

agricultural products; particle size; discrete element method; calibration of parameters; repose angle

2019-03-15

2019-06-28

国家重点研发计划项目（2018YFD0400704）；河南省科技厅自然科学项目（182102110163）

李永祥，教授，博导，粮食机械及理论。Email：liyongxiang@haut.edu.cn

徐雪萌，副教授，主要从事粮油食品包装工艺与装备研究。Email：xuxuemeng7439@163.com

10.11975/j.issn.1002-6819.2019.16.035

O347.7; TP391.9

A

1002-6819(2019)-16-0320-08

李永祥，李飞翔，徐雪萌，申长璞，孟坤鹏，陈 静，常东涛.基于颗粒缩放的小麦粉离散元参数标定[J]. 农业工程学报，2019，35(16)：320－327. doi：10.11975/j.issn.1002-6819.2019.16.035 http://www.tcsae.org

Li Yongxiang, Li Feixiang, Xu Xuemeng, Shen Changpu, Meng Kunpeng, Chen Jing, Chang Dongtao. Parameter calibration of wheat flour for discrete element method simulation based on particle scaling[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2019, 35(16): 320－327. (in Chinese with English abstract) doi：10.11975/j.issn.1002-6819.2019.16.035 http://www.tcsae.org