威布尔帕累托(Ⅳ)分布:性质及应用
宋晓琳


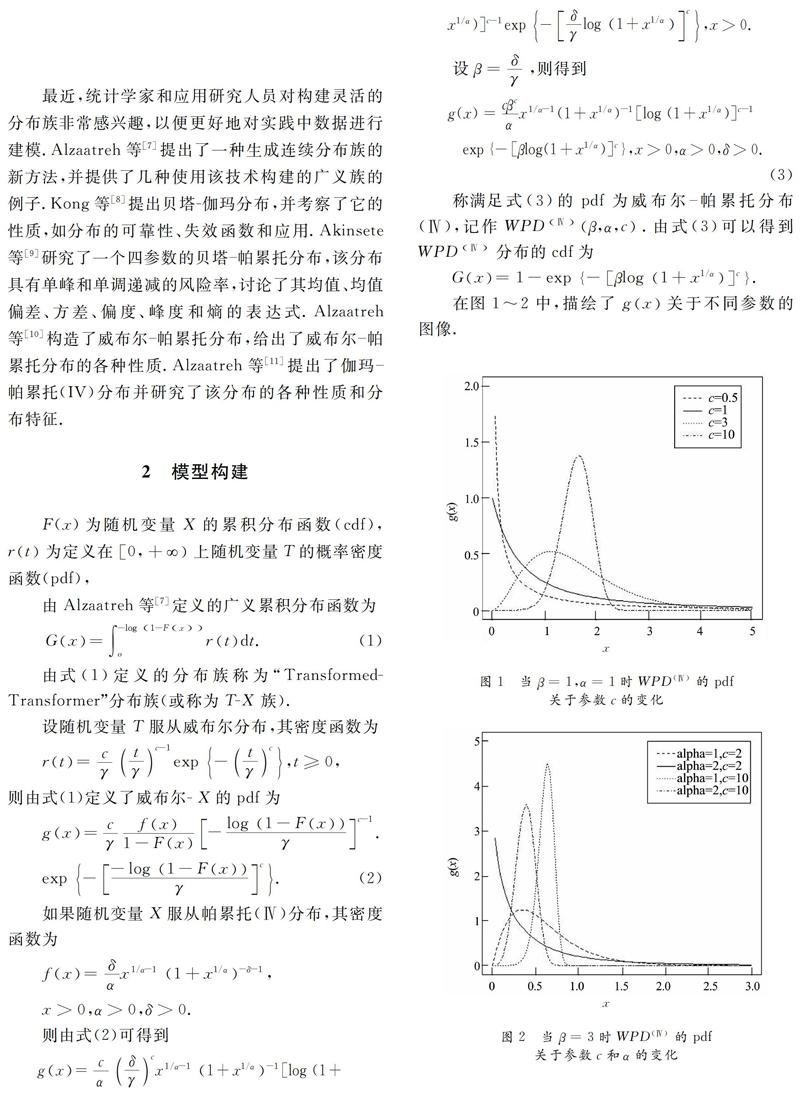
摘 要 利用T-X变换技巧将威布尔分布及帕累托(Ⅳ)分布组合,构建了威布尔帕累托(Ⅳ)分布并研究极限,单峰性,香农熵,力矩等相关统计性质;利用R语言对两组经典数据进行分布拟合;给出几种模型的参数估计及拟合优度的比较,并根据似然比检验,对威布尔帕累托(Ⅳ)分布和其他几种分布做对比分析,结果表明威布尔帕累托(Ⅳ)分布具有更优的拟合效果.
关键词 威布尔分布; 帕累托(Ⅳ)分布; T-X分布; EM算法
中图分类号 O212 文献标识码 A
Abstract We used T-X transform techniques to combine the two distributions, built the Weibull-Pareto (Ⅳ) distribution and studied the related statistical properties, including the limit, unimodal, shannon entropy and moment etc. Two groups of classic data distribution were fitting by using the R language.The comparison of parameter estimation and fit optimization of several models were given.And according to the likelihood ratio test for Weibull-Pareto (Ⅳ) distribution and several other distribution analysis of the contrast,the results show that Weibull-Pareto(Ⅳ) distribution has a better fitting effect.
Key words Weibull distribution; Pareto(Ⅳ) distribution; T-X family of distributions;EM algorithm
1 引 言
威布尔分布有单调的失效率,常用来模拟生命周期数据.帕累托分布及其推广提供了非常灵活的厚尾分布族,可以用来模拟收入分配、金融、保险等相关领域的数据.威布尔分布广泛应用于各种类型的数据建模,尤其在生存分析和可靠性分析中得到了广泛的关注.然而,它无法刻画具有非单调失效率函数的数据集,因此统计文献对威布尔分布进行了各种形式的推广[1-2].经典的帕累托分布是一类具有幂律概率尾的统计模型,常被用来模拟具有高度正倾斜和右厚尾数据[3].但是,帕累托分布的密度函数是单调递减的,因而无法处理具有驼峰形状的数据集.在各种类型的帕累托模型中,帕累托(Ⅳ)更值得关注,它是由Cronin[4]最早提出的.值得注意的是,大多数关于帕累托(Ⅳ)分布的分布理论都可以通过使用KOTZ等[5]提出的布尔分布获得,也可以参考Harris[6]的早期文献.帕累托(IV)模型的一个显著特征是,它包含了帕累托密度族的最大参数.
最近,统计学家和应用研究人员对构建灵活的分布族非常感兴趣,以便更好地对实践中数据进行建模.Alzaatreh等[7]提出了一种生成连续分布族的新方法,并提供了几种使用该技术构建的广义族的例子.Kong等[8]提出贝塔-伽玛分布,并考察了它的性质,如分布的可靠性、失效函數和应用.Akinsete等[9]研究了一个四参数的贝塔帕累托分布,该分布具有单峰和单调递减的风险率,讨论了其均值、均值偏差、方差、偏度、峰度和熵的表达式.Alzaatreh等[10]构造了威布尔帕累托分布,给出了威布尔帕累托分布的各种性质.Alzaatreh等[11]提出了伽玛帕累托(IV)分布并研究了该分布的各种性质和分布特征.
参考文献
[1] KHAN M S, KING R . Transmuted modified weibull distribution: a generalization of the modified weibull probability distribution[J]. European Journal of Pure and Apllied Mathematics.2013, 6(1): 66-88.
[2] IBRAHIM B, ABDUL M.Transmuted burr type III distribution[J]. Journal of Statistics: Advances in Theory and Applications.2015, 14 (1):37-47.
[3] KLUGMAN S A, PANJER H H,WILLMOT G E. Loss models from data to decisions[M].New York: John Wiley & Sons, Inc,1998.
[4] CRONIN D C. Function for size distribution of incomes: a further comment[J]. Econometrica, 1979, 47(3): 773-774.
[5] KOTZ S,BALAKRISHNAN N,JOHNSON N L.Continuous multivariate distributions.[M]. New York: John Wiley & Sons, Inc,2000.

