单位球面中Clifford环面的刚性定理*
胡传峰, 姬 秀
(长江大学 文理学院, 湖北 荆州 434000)
单位球面中Clifford环面的刚性定理*
胡传峰, 姬 秀
(长江大学 文理学院, 湖北 荆州 434000)
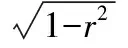
主曲率; 数量曲率; Clifford环
0 引 言
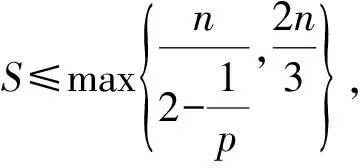
1 预备知识
设Mn是单位球面Sn+1(1)中的紧致极小超曲面, 在Sn+1中选取标准正交标架场e1,…,en+1, 使得限制于Mn时,e1,…,en与Mn相切. 令w1,…,wn+1是上述标架的对偶标架, 并约定各类指标范围为
1≤A,B,C…≤n+1, 1≤i,j,k…≤n.
在Sn+1(1)上, 结构方程为
KABCD=δACδBD-δADδBC.
限制于Mn时,
wn+1=0,wn+1i=hijwj,hij=hji,
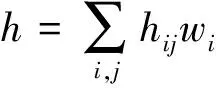
由式(2)得
用hijk及hijkl分别表示hij的一阶, 二阶共变导数, 则有
进而有如下Codazzi方程和Ricci恒等式
定义hij的Laplace为
2 主要定理及证明

证明为方便起见, 用g表示gj.


充分性: 设βij=0,i=1,…,n, 则由已知条件可得gj=n.
证毕.

证明选取适当的标架, 使得hij=λiδij, 且设λn<0<λ1≤λ2≤…≤λn-1, 定义

则有
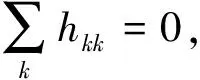
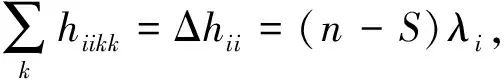

由S为常数可得
由式(11), 式(13)和式(14)及等式
可得
SΔF=
将等式
代入式(15)得
SΔF=


即第三项也非正. 接下来, 证明第四项也非正.
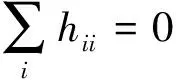
n.
定义βij=hiij, 则λi,βij满足定理1的条件. 若固定j∈{1,…,n-1}, 则由定理1得到|hnnj|≠max{|hiij|,i=1,…,n} 或者hnnj=0. 用gj∈In-1={1,…,n-1} 表示使得等式|hgjgjj|=max{|hiij|,i=1,…,n} 成立的指标. 考虑In-1的子集A={j∈In-1;gj=j},B={j∈In-1;gj≠j,n},C={j∈In-1;gj=n}. 不失一般性可令A={1,…,r},B={r+1,…,t},C={t+1,…,n-1}. 这里我们约定若A为空集, 则r=0; 若B为空集, 则t=r; 若C为空集, 则t=n-1. 比如若B为空集, 则A={1,…,r},C={r+1,…,n-1}.
下面我们用更简单的方式记
(n-S).


因此有
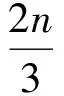
由Mn紧致可得

[1] Lawson B H. Local rigidity theorems for minimal hypersurfaces[J]. Annals of Mathematics, 1969, 89(1): 187-197.
[2] Li A M, Li J M. An intrinsic rigidity theorem for minimal submanifolds in a sphere[J]. Archiv der Mathematik, 1992, 58(6): 582-594.
[3] Peng C K, Terng C L. Minimal hypersurfaces of spheres with constant scalar curvature[J]. Annals of Mathematics Studies, 1983, 103: 177-198.
[4] Suh Y J, Yang H Y. The scalar curvature of minimal hypersurfaces in a unit sphere[J]. Communications in Contemporary Mathematics, 2007, 9(2): 183-200.
[5] Zhu P. The scalar curvature of minimal hypersurfaces in a unit sphere[J]. Journal of Mathematical Analysis and Applications, 2015, 430(2): 742-754.
[6] Wu B Y. On hypersurfaces with two distinct principal curvatures in a unit sphere[J]. Differential Geometry and Its Applications, 2009, 27(5): 623-634.
[7] Xu H W, Xu Z Y. A new characterization of the Clifford torus via scalar curvature pinching[J]. Journal of Functional Analysis, 2014, 267(10): 3931-3962.
[8] Wang Q L, Xia C Y. Rigidity theorems for closed hypersurfaces in a unit sphere[J]. Journal of Geometry and Physics, 2005, 55(3): 227-240.
[9] Yang B G, Liu X M. R-minimal hypersurfaces in space forms[J]. Journal of Geometry and Physics, 2009, 59(6): 685-692.
[10] Wang M J, He J X. Scalar curvature of minimal hypersurfaces in a sphere[J]. Journal of Mathematical Analysis and Applications, 2012, 391(1): 291-297.
[11] Li T Z. Compact Willmore hypersurfaces with two distinct principal curvatures inSn+1[J]. Differential Geometry and Its Applications, 2014, 32: 35-45.
[12] Ding Q, Xin Y L. On Chern’s problem for rigidity of minimal hypersurfaces in the spheres[J]. Advances in Mathematics, 2011, 227(1): 131-145.
[13] Zhang Q. The pinching constant of minimal hypersurfaces in the unit spheres[J]. Proceedings of the American Mathematical Society, 2010, 138(5): 1833-1841.
[14] Zhang Y T. Rigidity theorems of Clifford torus[J]. Acta Mathematica Scientia, 2010, 30(3): 890-896.
[15] Wei G X. Rigidity theorem of hypersurfaces with constant scalar curvature in a unit sphere[J]. Acta Mathematica Sinica, English Series, 2007, 23(6): 1075-1082.
RigidityTheoremofCliffordTorusinaUnitSphere
HU Chuan-feng, JI Xiu
(College of Arts and Science, Yangtze University, Jingzhou 434000, China)

principal curvature; scalar curvature; Clifford torus
1673-3193(2017)03-0260-04
2016-09-06
湖北省教育厅科学技术研究基金资助项目(B2016453, B2016458)
胡传峰(1978-), 男, 讲师, 硕士, 主要从事微分几何的研究.
O186.12
A
10.3969/j.issn.1673-3193.2017.03.002

