一类广义代数Riccati方程的数值求解算法

周立平



一类广义代数Riccati方程的数值求解算法

周立平

（湖南科技学院 理学院，湖南 永州 425199）

广义代数Riccati方程的数值方法在非稳定系统模型降阶，滤波和动力系统控制中有十分重要的作用。本文基于牛顿迭代法设计了一种求解代数Riccati方程的预条件共轭算法，数值实验验证了该方法具有良好的收敛性。

代数Riccati方程；牛顿迭代法；预条件共轭梯度法

1　引　言

本文旨在研究如下一类广义代数 Riccati 方程(ARE)的数值解：

ARE(1.1)的求解在非稳定系统模型降阶，滤波，线性二次校正问题和动力系统控制中有十分重要的作用。ARE（1.1）是二次矩阵方程，且是非线性的，在一定条件下，ARE(1.1)具有唯一的半正定解[1]。

目前，对ARE进行数值求解大多是在Newton迭代法框架下进行。如Chandrasekhar迭代法[3,4]和NM-Kleinman 迭代法[5,6]等，它们的主要思想是将二次方程线性化后再求解. 为给出Peter Benner等人在文[7]中给出了低秩Newton Cholesky因子法(LRCF-NM)和低秩Newton Galekin-ADI 方法(LRCF-NM-GP-ADI)。PFADI

基于Newton迭代法框架求解ARE(1.1)，通常会导出一系列Lyapunov方程。因此，针对这些Lyapunov方程的高效稳定算法成为求解ARE（1.1）的关键。目前，已有一系列研究成果[8,9]。最近，Lin与V.Simoncini在文[10]中提出了基于最小残差法（MINRES）求解大规模Lyapunov 方程的方法。受此启发，本文将在Newton迭代法框架下，结合MINRES方法和预条件共轭最小二乘法(PCGLS)求解Lyapunov方程，进而得到ARE（1.1）的数值解。

2　基于Newton 迭代的预条件共轭梯度法

算法2.1

不失一般性，去掉方程（2.）的各项下标，得到如下一般形式的(广义)Lyapunov方程

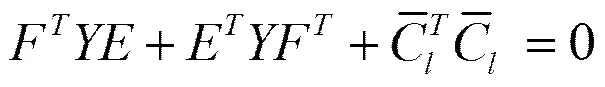
对于方程(2.2)，现在最常用的方法是Krylov子空间方法[12-14]。本文取一种简单Krylov子空间为

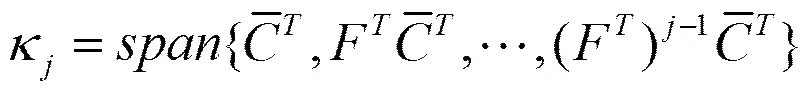
(2.3)

注意到方程(2.3)的解其实质就是使残差范数最小，设方程(2.3)的近似解为，则它可作为如下优化问题的解

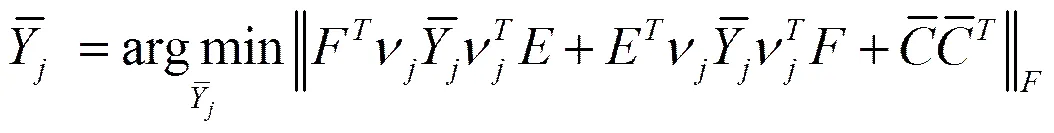
引入算子

，

则(2.4)等价为

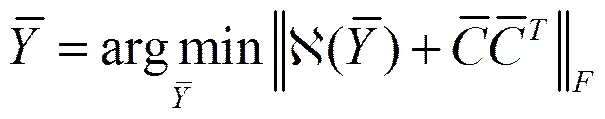
对于方程(2.5)，为加快收敛速度，本文采用采用预条件共轭梯度最小二乘方法(PCGLS)求(2.5)的解。事实上，利用拉直算子可将(2.5)化为矩阵-向量方程形式，但为了避免计算结束时再次对向量形式的解进行转换，我们直接使用其矩阵-矩阵形式。记算子的伴随算子为，则。记预条件子为，求解 (2.5) 的PCGLS算法[10]。可描述如下：

算法 2.2

需要指出的是，布尔量flag用于标记Krylov 子空间的基是否能满足算法 2.2的收敛性要求。

结合算法2.1和2.2，我们得到了如下求解ARE(1.1)的基于最小残差的预条件共轭最小二乘牛顿迭代算法。

算法 2.3

3　数值实验

下面我们将给出运用算法2.3求解ARE(1.1)的实例。为简单起见，取ARE(1.1)中为单位矩阵。考察如下二维抛物型偏微分方程

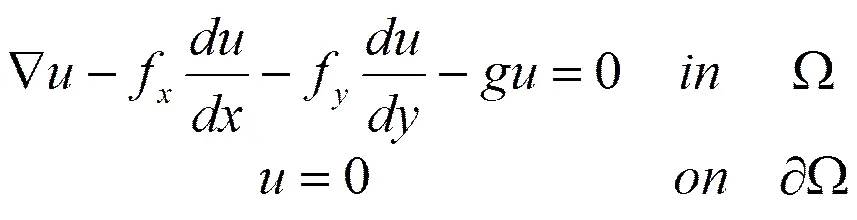
调用Lyapack 软件包[2]中的子程序fdm_matrix和fdm_vector对方程 (5.1) 运用有限差分法进行离散化后生成的网格矩阵..

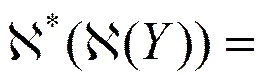
因此，可取算子M满足

.

模型（3.1）的数值结果如下：

阶数残差向量范数迭代次数 328.2952e-0123 641.0045e-0117 1287.6582-01112

[1]P.Lancaster and L.Rodman.The Algebraic Riccati Equation[M].Oxford University Press:Oxford,1995.

[2]T.Penzl.LYAPACK:A MATLAB toolbox for large Lyapunov and Riccati equations,model reduction problems,and linear-quadratic optimal control problems,users’ guide (ver.1.0).2000[J].Available at: www. tu-chemnitz.de/sfb393/lyapack.

[3]H.T.Banks,K.Ito.A numerical algorithm for optimal feedback gains in high dimensional linear quadratic regulator problems [J].SIAM J.Control.Optim.,1991,29(3):499-515.

[4]J.A.Burns,K.P.Hulsing.Numerical methods for approximating functional gains in LQR boundary control problems [J].Mathematical and Computer Modelling,2001,33(1):89-100.

[5] D.Kleinman.On an Iterative Technique for Riccati Equation Computations [J].IEEE Transactions on Automat.Control.,1968, 13(1):114-115.

[6]I.G.Rosen and C.Wang.A multilevel technique for the approximate solution of operator Lyapunov and algebraic Riccati equations [J].SIAM J.Numer.Anal.,1995,32(2):514-541.

[7]P.Benner and J.Saak. A Galerkin-Newton-ADI method for solving large-scale algebraic Riccati equations[R].Technical Report SPP1253-090,Deutsche Forschungsgemeinschaft - Priority Program 1253,2010.

[8]Gajic Z,Qureshi M.Lyapunov Matrix equation in system stability and control [M].Academic Press:San Diego,1995.

[9]P.Benner,J.R.Li,T.Penzl.Numerical solution of large-scale Lyapunov equations,Riccati equations,and linear-quadratic optimal control problems [J].Numer.Linear Algebra Appl.,2008,15(9):755–777.

[10]Lin Y,V.Simoncini. Minimal residual methods for large scale Lyapunov equations[J].Appl.Numer.Math.,2013,72(2):52-71.

[11]Badía J M,Benner P,Mayo R,et al.Parallel Solution of Large-Scale and Sparse Generalized Algebraic Riccati Equations[C].International Conference on Parallel Processing.Springer-Verlag,2006:710-719.

[12]Y.Saad.Iterative methods for sparse linear systems [M].2nd ed..Society for Industrial and Applied Mathematics:Philadelphia,PA, 2003.

[13]Kemin Zhou,John C.Doyle,Keith Glover.Robust and optimal control[M].New Jersey:Prentice Hall,2007.

[14]V.Simoncini.A new iterative method for solving large-scale Lyapunov matrix equations [J].SIAM J.Sci.Comput.,2007, 29(3):1268–1288.

（责任编校：何俊华）

2017－03－20

湖南省教育厅资助科研项目（项目编号12C0688）。

周立平（1978－），男，湖南永州人，副教授，硕士，研究方向为数值代数和偏微分方程数值解。

O241.6/O151.21

A

1673-2219（2017）06-0001-04