Optimal design of the aerodynamic parameters for a supersonic twodimensional guided artillery projectile

Ke Ling,Zheng Hung,Jing-min Zhng

aXi'an Institute of Electromechanical Information Technology,Norinco Group,No.99 Jixiang Road,Xi'an 710065,Shaanxi,China

bNo.208 Research Institute of China Ordnance Industries,1023 Changping,Beijing 102202,China

Optimal design of the aerodynamic parameters for a supersonic twodimensional guided artillery projectile

Ke Lianga,*,Zheng Huanga,Jing-min Zhangb

aXi'an Institute of Electromechanical Information Technology,Norinco Group,No.99 Jixiang Road,Xi'an 710065,Shaanxi,China

bNo.208 Research Institute of China Ordnance Industries,1023 Changping,Beijing 102202,China

A R T I C L E I N F O

Article history:

Two-dimensional guided projectile

Aerodynamic parameters

Canard pro file

Numerical simulation

Optimal design

An optimization method is introduced to design the aerodynamic parameters of a dual-spin twodimensional guided projectile with the canards for trajectory correction.The nose guidance component contains two pairs of canards which can provide lift and despin with the projectile for stability.The optimal design algorithm is developed to decide the pro files both of the steering and spinning canards, and their de flection angles are also simulated to meet the needs of trajectory correction capabilities. Finally,the aerodynamic ef ficiency of the speci fic canards is discussed according to the CFD simulations. Results that obtained here can be further applied to the exterior ballistics design.

©2017 Published by Elsevier Ltd.This is an open access article under the CC BY-NC-ND license(http:// creativecommons.org/licenses/by-nc-nd/4.0/).

1.Introduction

As improving artillery projectile accuracy is obviously bene ficial for the fire ef ficiency,nowadays the precision-guided munitions are of interest to the Army as a means of both reducing collateral damage and increasing the chance of desired effect with the first round fired[1,2].

In this paper,some fundamental studies on the structural and aerodynamic features for the guided projectile in the preliminary design of its exterior ballistics were discussed.There were many previous works that were contributed to the methods involved in this paper.Theodoulis et al.introduced the guidance and control modules for a class of spin-stabilized fin-controlled projectiles[[3-6],and the complete nonlinear dynamical model is developed and analyzed.Chang et al.analyzed the impact of the spin-rate on the forward section ofthe trajectory,their results indicated thatthe spin-rate property is in fluenced by the canards actuation[7-9].As the dual-spin guided projectiles are fundamentally less stable than the conventionalballistic spin-stabilized projectiles,Wernert et al. modelled and analyzed the stability conditions of the guided projectiles[10,11].Hamel,Youn,Sahu and et al.studied the aerodynamic characteristics of different kinds of trajectory correction projectiles[12-14].Those studies gave us the ideas to design, modeland analyze the complicated dynamics of the guidance and controlsystem ofthe guided projectile.In particular,they provided some helpful references to investigate the aerodynamic characteristics in the preliminary design.

The purpose ofthis work is to design the controlcanards for the dual-spin two-dimensional guided projectile.An optimal design method was developed in this paper to obtain the aerodynamic parameters of the control canards for trajectory correction.Numerical simulations were performed to study the aerodynamic ef ficiency of the guided projectile with control canards.

2.Model and method

2.1.Model of the 2-D guided artillery projectile

The two-dimensional guided projectile in this study includes a conventional 155 mm projectile body and a nose guidance component which is used for trajectory correction.The design model the two-dimensional guided projectile is shown in Fig.1. There are two pairs of canards fixed on the nose component of the projectile.The first pair of canards,called the steering canards,is mounted in the same direction on the nose componentto create liftforce.Meanwhile,the second pair of canards,named the spin canards,is differentially canted in a manner to create a suf ficient amount of moment to rotate the head component in an opposite direction of the projectile spin.

2.2.Optimal design method

In design of the Two-dimensional guided projectile,it is absolutely essential that the aerodynamic parameters for different canard wings'structures are analyzed and optimized.Therefore, the optimal aerodynamic con figuration can be obtained,and as well as the required correction forces and moments can be guaranteed.In detailed design,severaldesigned parameters,such as the wing area,the pro file,the aspectratio,the sweepback angle and the taper ratio,are indispensable for in fluencing the aerodynamic con figuration of the projectile.

The general guideline of the wing area design is to provide the necessary trajectory correction ability as much as possible in the limited shape space.As the changes of aerodynamic con figuration are comparatively limited due to the restraints both from the shape of the projectile and the lift force of the canard wing,the study of the trajectory correction ability is focused on the calculations of additional force and additional moment about the projectile with corrective canards.By adding the additionalforces and moments to the equations ofmotion[15],the trajectory correction abilities with respect to the different wing areas can be investigated.

There are two types of pro file that can be divided as the supersonic pro file and the subsonic pro file in application.For the frequently used supersonic pro files,such as diamond shape,lens shape,hexagon and blunt trailing edge,their features are simply shaped airfoils with sharp leading edges to cut down the shock wave.For the subsonic pro files,such as symmetric arc,asymmetric arc and laminar flow,they are usually streamlined with relatively smooth leading edges to enhance the leading-edge suctions and to reduce the atmospheric drags.

While increasing the aspect ratio,generally,the slope of lift curve will be elevated.For a speci fic length of the wing root,both the span and the aspect ratio will be raised at the same time. However,the span must not exceed the caliber of the artillery.The length ofmean chord willdecrease while the friction willincrease, and the wave drag willalso increase for a low mach number during the supersonic flying.

The sweepback angle willmainly impactthe resistance property of the projectile.The reasons for using the sweepback angle are to increase the criticalmach number,delay the shock wave,decrease the peak value ofthe drag coef ficient and make the drag coef ficient change smoothly with the increasing of the mach number.The taper ratio has less in fluence on aerodynamics of the projectile when the other geometric parameters had been finalized.

Changing of any mentioned parameters above will affect its aerodynamic ef ficiency of the 2-D guided projectile.In order to obtain the optimalaerodynamic con figuration,both the constraint of the structural strength and the adjustment of the canard's aerodynamic shape should be considered.In this study,the optimal design algorithm is developed by coupling of the fluid and solid,as shown in Fig.2,which make sure the aerodynamic ef ficiency to be optimized under all the given requirements.

From Fig.2,there are two types of parameters need to be optimized for selecting the canards,which are structure parameters and aerodynamic parameters.Meanwhile,there are strong connections between these two types of parameters.Firstly,we calculated the structure parameters,such as parameters of the pro file,by using engineering prediction methods,and made those results as the initial inputs of the optimization process.Then,the aerodynamic parameters are simulated and optimized to meet the trajectory correction capability of the guided projectile.During the optimizing process,both the structure parameters and aerodynamic parameters might be redesigned under their boundary conditions.And finally,the local optimal solution can be obtained as well as the canards can be selected.

After the optimization method is used to obtain the pro files of steering or spinning canards,the relationship between the de flection angle of control surface and the angle of attack can benumerically simulated by CFD method.Then,the aerodynamic efficiency of the designed canards can be analyzed.

3.Simulations and results

Increasing of the wing area will be bene fit to improve the trajectory correcting capability of the aerodynamic controlling canards.However,it will be caused not only the loss of firing range but also the flight instability of the spinning projectile.In order to obtain the optimal aerodynamic shape of the canard,its aerodynamic ef ficiency should be simulated and analyzed to optimize the structure of the canards.Both the correction capability and the flightstability for the 2-D guided projectile were considered during the whole simulation process.

3.1.Selecting for the canard pro file

In the preliminary design,the engineering prediction methods [16,17]are used to getthe values ofthe aerodynamic characteristics from the determined structure parameters.There are eighttypes of pro files have been evaluated,and their structure speci fic parameters are shown in Table 1.By applying allthe pro files to the guided projectile,their aerodynamic characteristics can be obtained.And these parameters can be used as the initial inputs of the optimization process.The selecting criteria ofthe bestpro file are large liftto-drag ratio,little changes of pressure center and smallde flection angle.

The requirements of the lifts for the steering canards and the spin moments for the spin canards were estimated from the simulations of the 6-DOF externalballistics model,as seen in Table 2. The values of the lifts and the spin moments with different Mach numbers must meet the capability of trajectory correction for the guided projectile on its entire ballistic trajectory.Therefore,it can be the finalcriterion to verify the capability ofthe designed canard.

According to the capabilities both oflift force and spin moment, the No.8 canard pro file in Table 1 is selected as the best matching pro file to meet the demands of trajectory correction capabilities. Results of the comparison between the requirements and capabilities can be seen in Fig.3 and Fig.4.The required value curves in Figs.3 and 4 are obtained by using the curve fitting method from the data in Table 2.

Simulation results of the aerodynamic parameters indicate that their capabilities willmeet the design requirements with an eleven degree de flection angle for the pair ofsteering canards and the plus or minus six degrees de flection angles for the spin canards.

3.2.CFD numerical simulation

UG is used to construct the three dimensionalmodelofthe 2-D guided projectile,and the Pointwise software also used for the CFD meshing.As seen in Fig.5,the boundaries of the computational domains for the external flow fields are setup with reference to the length of projectile.They are ten times of the length in the X direction,and four times of the length in the Y direction and Z direction.The meshing details at the head and tail of the projectile can be seen in Fig.6.The amount of total grids in this CFD simulation is around 9 million units.To insure the convergence of the results,the grid quality has been checked,and itshows the nice grid qualities with very low cellsquish and skewness.

As shown in Fig.6,the coordinate system is de fined as follows: The origin ofthe coordinate is fixed atthe head ofthe projectile,the X axis is pointing to the projectile tail along its body,the Z axis is pointing to the direction of the normalforce which is verticalwith respect to the steering canards,and the Y axis is vertical with respect to the pair of the spin canards.

The points of reference in the calculation are described as follows:The reference point to calculate the center of pressure coefficient,the center of Magnus pressure coef ficient,pitching moment coef ficient and yawing moment coef ficient is the apex of the head; the reference point to calculate pitch-damping moment coef ficient is the center of mass.

Some other speci fic values that involved in the simulation can be found as below.Noted that the reference area is determined based on the projectile caliber.

Reference area:S=0.01897 m2;

Reference length:l=0.9365 m;

Mach number:Ma=0.6,0.7,0.8,0.9,1,1.1,1.2,1.5,1.8,2,2.5,3;

Angle of attack:α=0°，2°，4°，6°，8°.

3.3.Calculating formulas

The formulas for calculating the aerodynamic parameters are summarized in this section,and can be seen as bellow[16,18].

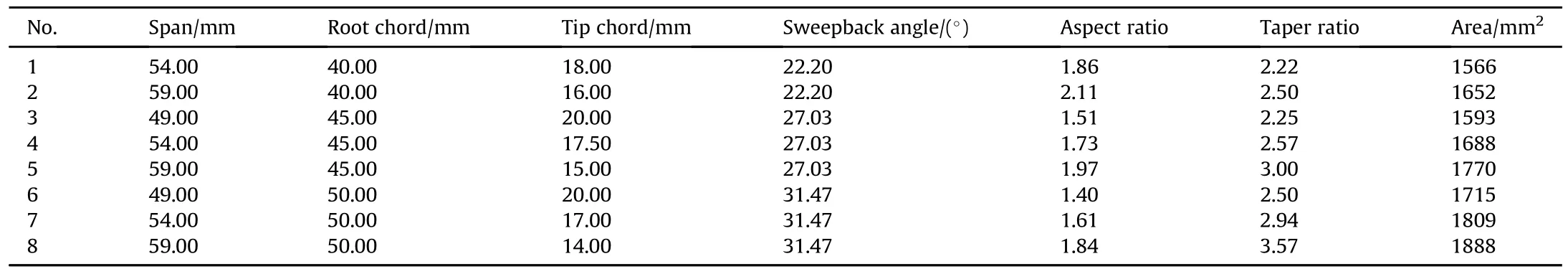
Table 1 Structure parameters for eight types of pro files.

Table 2 Requirements of the lift and spin moment.

3.4.Aerodynamics coef ficients results

FLUENT is used to calculate the aerodynamics coef ficients ofthe guided projectile that with the preferred canards.Results for different attack angles(α=0°，2°，4°，6°，8°)are shown from Figs.7-11.

In Figs.7-11,it was shown that how the de flection angle affects with the normal force of the projectile under the speci fic attack angles and Mach numbers.From these results,the ef ficiency of the canards can be approximately evaluated when the de flection angle and the normalforce have a linear relationship.

3.5.Ef ficiency analysis of the canards

The aerodynamic ef ficiency of the control canards is analyzed from the results shown in Figs.7-11.When the Mach number is greater than one(Ma＞1),the normalforce coef ficientofthe whole projectile is increasing linearly during the de flection angle of the steering canards growth.It is indicated that the aerodynamic ef ficiency of the canards had changed linearly in this supersonic segment.

When the Mach number is equal to one(Ma=1),the normal force coef ficient is shown irregular alterations with respect to the changes ofboth the de flection angle ofcanards and the attack angle ofprojectile.The aerodynamic ef ficiency ofcanards is in fluenced by the angle of attack.For detailed discussion,(1)when a=0°and Ma=1,the normal force coef ficient is raising linearly as the de flection angleδ＜15°;(2)whenα=2°and Ma=1,the normal force coef ficient is raising linearly as the de flection angleδ＜15°; (3)whenα=4°and Ma=1,the normalforce coef ficient is raisinglinearly as the de flection angleδ＜12°;(4)whenα=6°and Ma=1, the normal force coef ficient is raising linearly as the de flection angleδ＜10°;(5)whenα=8°and Ma=1,the normal force coef ficient is raising linearly as the de flection angleδ＜8°.

When the Mach number is less than one(Ma＜1),the normal force coef ficient is also demonstrated irregular variations by changing from both the de flection angle of canards and the attack angle ofprojectile.In this subsonic flightphase,the linear segments of the aerodynamic ef ficiency of canards are shortened during the angle ofattack growth.For further discussion,(1)whenα=0°and Ma＜1,the normal force coef ficient is increased linearly while δ＜12°;(2)whenα=2°and Ma＜1,the normalforce coef ficient isincreased linearly whileδ＜10°;(3)whenα=4°and Ma＜1,the normalforce coef ficient is increased linearly whileδ＜8°;(4)when α=6°and Ma＜1,the normalforce coef ficientis increased linearly whileδ＜4°;(5)whenα=8°and Ma＜1,the normal force coefficient is weakened to be negative.

4.Conclusions

This study has shown some fundamental works in the preliminary design of exterior ballistics.An optimization method is developed to obtain the applicable aerodynamic parameters of the controlling canards for a 2-Dguided artillery projectile.The optimal canard pro file is designed to make the guidance component meet the needs oftrajectory correction capabilities.And on this basis the ef ficiency of the canards is simulated and analyzed.The results of the aerodynamic parameters obtained in this study could be the valuable inputs for the further design of exterior ballistics.

While adding of the canards may improve the accuracy of the artillery projectile,the dynamicalstability ofthe spinning projectile willbe affected at the same time.Some basic suggestions from this study are:Firstly,the design of the canards must not destroy the spinning stability of the guided projectile during the entire time of flight;secondly,the accuracy of the guided projectile should be increased by optimizing the structure and aerodynamic parameters,not just by extending the size of the canards.

[1]Grignon C,Cayzac R,Heddadj S.Improvement of artillery projectile accuracy, 23rd International Symposium on Ballistics,International Ballistics Committee,Tarrgona,Spain,2007,pp.747-754.

[2]Fresconi F,Cooper G,Celmins I,DeSpirito J,Costello M.Flight Mechanics of a Novel Guided Spin-Stabilized Projectile Concept,AIAA Atmospheric Flight Mechanics Conference,Toronto,ON Canada,2010.

[3]Theodoulis S,Gassmann V,Werner P,Dritsas L,Kitsios I,Tzes A.Guidance and control design for a class of spin-stabilized fin-controlled projectiles.J Guid Control,Dyn 2013;36(2):517-31.

[4]S`eve F,Theodoulis S,Wernert P,Zasadzinski M,Boutayeb M.Pitch/Yaw Channels Control Design for a 155mm Projectile with Rotating Canards,using a H∞Loop-Shaping Design Procedure,AIAA Guidance,Navigation,and Control Conference,National Harbor,Maryland,2014.

[5]Theodoulis S,Gassmann V,Brunner T,Wernert P.Robust Bank-to-Turn Autopilot Design for a Class of 155mm Spin-Stabilized Canard-Guided Projectiles,AIAA Atmospheric Flight Mechanics Conference,Boston,MA,2013.

[6]Spagni J,Theodoulis S,Wernert P.Flight control for a class of 155 mm spinstabilized projectile with reciprocating canards,AIAA Guidance,Navigation, and Control Conference,Minneapolis,Minnesota,2012.

[7]Chang SJ,Wang ZY,Liu TZ.Analysis of spin-rate property for dual-spin-stabilized projectiles with canards.JSpacecr Rockets 2014;51(3).985-966.

[8]Chang S.J.Dynamic response to canard control and gravity for a dual-spin projectile.J Spacecr Rockets 2014;53(3):558-66.

[9]Wang Y,Cheng J,Yu JY,Wang XM.In fluence of yawing force frequency on angular motion and ballistic characteristics of a dual-spin projectile.Def Technol 2016;12(2):124-8.

[10]Wernert P.Stability analysis for canard guided dual-spin stabilized projectiles, AIAA Atmospheric Flight Mechanics Conference,Chicago,Illinois,2009.

[11]Wernert P,Theodoulis S.Modeling and stability analysis for a class of 155 mm spin-stabilized projectiles projectiles with course correction fuse(CCF),AIAA Atmospheric Flight Mechanics Conference,Portland,OR,2011.

[12]Hamel N,Gagnon E.CFD and parametric study on a 155 mm artillery shell equipped with a roll-decoupled course correction fuze,29th AIAA Applied Aerodynamics Conference,Honolulu,Hawaii,2011.

[13]Youn E B,Silton S I.Numerical Study on Bending Body Projectile Aerodynamics,34th AIAA Applied Aerodynamics Conference,Washington,D.C., 2016.

[14]Sahu J.Time-accurate computations of free-flight aerodynamics of spinning projectile with and without flow control,AIAA Atmospheric Flight Mechanics Conference,Keystone,Colorado,2006.

[15]Costello M,Peterson A.Linear theory of a dual-spin projectile in atmospheric flight.J Guid Control,Dyn 2000;23(5):789-97.

[16]Anderson D.J.Fundamentals of aerodynamics.3rd ed.New York:McGraw-Hill;2001.

[17]Lei JM,Wu JS.Engineering prediction methods of aerodynamics characteristics for guided weapon.Beijing:Beijing Institute of Technology Press;2015.

[18]Han ZP.Exterior ballistics of projectiles and rockets.Beijing:Beijing Institute of Technology Press;2008.

24 January 2017

*Corresponding author.

E-mail addresses:liangke3039@163.com(K.Liang),huangzheng82369@163. com(Z.Huang),zjm_208suo@163.com(J.-m.Zhang).

Peer review under responsibility of China Ordnance Society

http://dx.doi.org/10.1016/j.dt.2017.05.003

2214-9147/©2017 Published by Elsevier Ltd.This is an open access article under the CC BY-NC-ND license(http://creativecommons.org/licenses/by-nc-nd/4.0/).

Received in revised form 30 March 2017

Accepted 10 May 2017

Available online 10 May 2017