Research on the temperature field of a partially freezing sand barrier with groundwater seepage

LiYan Lao, ZhiQiang Ji,2,3*, LiangLiang Huang, ShangJing Li

1. State Key Laboratory of Nuclear Resources and Environment, East China University of Technology, Nanchang, Jiangxi 330133, China

2. State Key Laboratory for Geomechanics &Deep Underground Engineering, China University of Mining and Technology, Xuzhou, Jiangsu 221008, China

3. State Key Laboratory of Frozen Soil Engineering, Northwest Institute of Eco-Environment and Resources, Chinese Academy of Sciences, Lanzhou, Gansu 730000, China

Research on the temperature field of a partially freezing sand barrier with groundwater seepage

LiYan Lao1, ZhiQiang Ji1,2,3*, LiangLiang Huang1, ShangJing Li1

1. State Key Laboratory of Nuclear Resources and Environment, East China University of Technology, Nanchang, Jiangxi 330133, China

2. State Key Laboratory for Geomechanics &Deep Underground Engineering, China University of Mining and Technology, Xuzhou, Jiangsu 221008, China

3. State Key Laboratory of Frozen Soil Engineering, Northwest Institute of Eco-Environment and Resources, Chinese Academy of Sciences, Lanzhou, Gansu 730000, China

To study the distribution characteristics and variation regularity of the temperature field during the process of seepage freezing, a simulated-freezing test with seepage of Xuzhou sand was completed by using a model test developed in-house equipment. By means of three group freezing tests with different seepage velocities, we discovered the phenomenon of the asymmetry of the temperature field under the influence of seepage. The temperature upstream was obviously higher than that downstream. The temperature gradient upstream was also steeper than that downstream. With a higher seepage velocity, the asymmetry of the temperature field is more pronounced. The asymmetry for the interface temperature profile is more strongly manifest than for the main surface temperature profile. The cryogenic barrier section is somewhat "heartshaped". With the increasing velocity of the seepage flow, the cooling rate of the soil decreases. It takes much time to reach the equilibrium state of the soil mass. In our study, seepage flow velocities of 0 m/d, 7.5 m/d, and 15 m/d showed the soilcooling rate of 4.35 °C/h, 4.96 °C/h, and 1.72 °C/h, respectively.

freezing temperature field; seepage; freezing soil barrier; model test

1 Introduction

Ground freezing is a method of ground improvement with a nearly 140-year history. The principle is the unstable, water-bearing, soft soil layer will be frozen and high-strength water tightness of the cryogenic barrier underground will be formed by artificial refrigeration. The cryogenic barrier can resist the underground stress, hydraulic pressure, and other external loads around the underground engineering. At the same time, it can also cut off the hydraulic connection of groundwater with underground engineering and achieve the protection of underground engineering construction.

Over the past 60 years, this technology has been widely used in our country in mine shafts, foundation pit excavation, municipal engineering construction, subway tunnel construction, and other underground engineering construction (Chenet al., 2000; Zhanget al., 2011; Askar and Zhanbolat, 2015; Shiet al., 2015). The development and utilization of underground space will entail more complex engineering geological conditions and strict construction requirements (Ji and Xu, 2009; Wanget al., 2009; Xuet al.,2010). During artificial-freezing construction, if the seepage velocity is too great, it will be difficult for the cryogenic barrier to reach the closure stage or the designed thickness (Yuanet al., 2010; Zhouet al., 2011). The temperature field of the barrier under the seepage condition is more complicated under the change of seepage field (Mao and Liu, 2013; Fenget al., 2014). Therefore, it is very important to understand the characteristics of the temperature field of the cryogenic barrier under seepage conditions and to regulate cryogenic barrier formation.

Many domestic and foreign scholars have studied the temperature field of the cryogenic barrier under seepage conditions. Jumikis (2006) derived the similarity criterion of the model test and expounded the basic theory of that test of artificial ground-freezing engineering; Junget al. (2011) adopted the time-varying thermal conductivity and the constant steady-flow equations and with a finite element simulated the seepage of saturated soil; Yao and Chen (2006) carried out modeling designed to obtain the distribution rule of the freezing temperature field in static water and diverse water-flow speeds. The greater the flow velocity, the more heat can be taken away through water flow. Under the condition of dynamic water, the spreading speed of frozen soil in the upper soil layer decreases as the flow velocity increases. Zhouet al. (2011) studied the closure time of two ice poles, ascertaining the upstream and downstream temperature development was influenced by different seepage velocities and hole spaces. From the study, they found that the closure time increases sharply when seepage velocity increases; and the seepage velocity is the most important factor affecting the development of the upstream freezing-soil wall.

To study the effect of the temperature gradient on seepage freezing and reveal the developmental trend of the cryogenic barrier under seepage flow, which provides theoretical support for effective control of the ground-freezing process and design of freezing engineering. In this paper, the freezing test of Xuzhou sand soil under the condition of simulated seepage is carried out by using a model test developed in-house; and the temperature-field characteristics of the local frozen-soil barrier under seepage conditions are studied.

2 Experimental program

The test device was composed of four parts: the test chamber, the simulated-seepage system, the freezing–refrigeration system, and the temperature-measurement system. The size of test chamber (Figure 1) was 1,200mm×1,000mm×800mm, mainly consisting of the sample and buffer chambers. The design height of the sand layer in the sample chamber was 400 mm, which was the main body of the sample for the test. The compacted clay was 300 mm thick in the lower part of the sand layer, and the upper part of the sand layer was filled with 250-mm-thick compacted clay and 50-mm-thick asphalt, which were mainly used for water resistance and buffering the effect of frost heave. The buffer chamber was filled with a mixture of gravel and coarse sand 700 mm high, which acted as a buffer of water flow, allowing uniform laminar flow of water through the sand layer in the sample chamber and better simulating the groundwater flow. From left to right, there were three freezing pipes, perpendicular to the flow direction of horizontal alignment; and the freezing pipes (1 to 3) were separated by 110 mm. Each freezing pipe was 700 mm long and located 400 mm from the front of the sample. Except for the middle of each freezing pipe and the sand layer part of the contact, other parts of the pipe were wrapped with thermal-insulating materials.

The simulated-percolation system (Figure 2) included the clean-water pipeline system and the watertemperature control system. The pipeline system consisted of water pumps, pressure gauges, glass rotor flowmeter, valves, bypass channels, water tank, and connecting piping. The water-temperature control system contained temperature control, temperature sensors, and heating rods. During the test, the seepage velocity across the sand layer was adjusted to the designed value by the bypass valve system and the glass rotor flowmeter; and the flow was measured repeatedly at the inlet and outlet end of the test chamber until the rate difference between the inlet and the outlet was less than 10%.

The temperature sensor adapted a water-repellent, high-precision DS18B20 thermistor (measuring-temperature accuracy, 0.5 °C); 104 temperature-measuring points were arranged in the soil sample. The temperature-measurement points were arranged on three planes—100 mm, 200 mm, and 300 mm from the bottom of the sand; the serial numbers are H1, H2, H3, where H2 was the main temperature-measurement plane (Figure 3), which had 64 temperature measurement points; and the planes H1 and H3 had 18 and 22 temperature measurement points, respectively. The four lines in each plane were the L line on the axial surface of three freezing pipes, the L1 line on the main surface of the No. 1 freezing pipe, the L2 line on the main surface of the (middle) No. 2 freezing pipe, and the L3 line on the interface between the No. 2 and No. 3 freezing pipes.

Temperature dynamic monitoring uses a central, computerized collection and automatic recording system. The electrical signals returned by a sensor are collected by the Multifunctional Temperature Tester, which was developed by the State Key Laboratory for Geomechanics & Deep Underground Engineering,China University of Mining and Technology. The multifunctional temperature tester communicates directly with the COM interface of the notebook computer through the RS232 adapter cable. Temperature data is transmitted to the computer, to ensure realtime recording and reliability of data.

The soil sample of the test was Xuzhou medium sand. In accordance with the standard for soil test method (GB/T50123-1999) requirements, the sample was dried and sifted through a 5-mm sieve, and the large particles and the debris were removed to make the soil sample with a certain dry density and in a state of saturation; the main physical properties are shown in Table 1.

Figure 1 The model test chamber (unit: mm)

3 Analysis of experiment results

The four sets of seepage freeze tests were carried out in the State Key Laboratory for Geomechanics & Deep Underground Engineering. Under the same interval of freezing pipes, the temperature field of artificial freezing of a sand barrier with different seepage velocities was studied, in order to understand how the seepage affects the temperature field of artificial freezing a sand barrier; the experimental arrangements and results are shown in Table 2.

3.1 Temperature distribution in seepage direction

The curves of the temperature distribution on the main surface and the interface at the time of the barrier closure are shown in Figure 4. By comparing and analyzing the temperature curves in the condition of the seepage velocity of 0 m/d, 7.5 m/d, and 15 m/d, it can be found that the temperature distribution curve is obviously symmetrical without seepage; and the temperature distribution curve of the main surface and the interface shows upstream and downstream symmetry. While under the influence of the seepage, the upstream temperature is significantly higher than that downstream; with the increase of the seepage velocity, this asymmetry is also increasingly apparent; and the asymmetry of temperature distribution at the interface is greater than that at the main surface, which due to the seepage brings the upstream of the exothermiclow-temperature water downstream, which will lead to the impact of the downstream freezing pipe being greater than the upstream. At the same time, it can be seen from Figure 4 that the temperature gradient near the upstream of the freezing pipe is greater than that in the downstream; but the high gradient temperature zone shows that the upstream is smaller than the downstream, which is especially obvious in the temperature distribution at the interface. In the condition of seepage, the interface temperature gradient decreases significantly. It shows that the interface becomes the dominant channel of heat flow when the cryogenic barrier reaches the stage of closure and the main surface position of maximum thickness of the frozen soil has been completed. It verifies the law that the thickness of the cryogenic barrier region reaches uniform development after closure (Liuet al., 2012; Hu and Yang, 2015).

Figure 2 Seepage system schematic plot

3.2 Characteristics of temperature variation upstream and downstream

To study the characteristics of the temperature variation in the upstream and downstream of the freezing pipes, the No. 2 freezing pipe upstream and downstream of 25 mm and the No. 2 and No. 3 freezing pipe interface upstream and downstream of 25 mm are taken as the research object; and the temperature curves of the four temperature-measurement-points variation with time are shown in Figure 5. From Figure 5, it can be seen that the temperature at the measurement point is primarily steady after 6 h without seepage. With the increase of the seepage velocity, the temperature of the soil changes significantly with time, and the time of the equilibrium state increases, from 8 h to 12 h to be basically stable. Because of the effect of seepage, the uninterrupted flow of continuous temperature of groundwater heat making the measurement point within the soil has a warming trend, prompting the temperature of the slow rate of decline. This is due to the role of the seepage, due to the continuous flow of the constant temperature of the groundwater heat, so that the measured point within the soil has a warming trend, prompting the slow rate of temperature decline.

Figure 3 Position of measuring holes (unit: mm)

In contrast to the main-surface and interface cooling curves of the temperature-measurement points in Figure 5 can be obtained, the no-seepage cooling curve of the main surface temperature-measurement points is logarithmic; and the curve is smooth. The slope of the cooling curves at the interface obviously slows down at 0 °C. The reason may be that the latent heat is released when the water in the soil is frozen. In the condition of seepage, measuring the cooling curve point without this feature appeared at near 0 °C, which may because of the influence of the latent heat released from water condensation on the soil-temperature field under the condition of seepage.

The range of measuring points of the average cooling rate under the different test conditions is listed in Table 3. Take the freezing pipe column in the upstream and downstream of the range of 25 mm as an example; comparing the rate of cooling under different test conditions shows that the average cooling rate of the soil gradually decreases with the increase in seepage velocity. Without seepage, the average cooling rate is about 4.35 °C/h. With seepage velocities of 7.5 m/d and 15 m/d, the average cooling rate is about 3.96 °C/h and 1.72 °C/h, respectively. Compared with the average cooling rate of the upstreamand downstream freezing points, the average temperature-change rate of the downstream point of the barrier is higher than that of the upstream point, and the seepage velocity is faster. Under the no-seepage condition, there is no obvious difference in the rate of temperature change between areas upstream and downstream of the barrier.

The phenomenon shows that the condition of seepage has a great influence on the development of the cryogenic barrier; especially under the condition of high seepage velocity, the development of the cryogenic barrier has been greatly hindered, and the estimation of the seepage velocity in the engineering design should be particularly careful.

Table 1 Physical properties of the soil tested

Table 2 Results of model test of partially freezing of a sand barrier, with groundwater seepage

Figure 4 Temperature distribution of the freezing process

Figure 5 Temperature variation with time of the freezing process

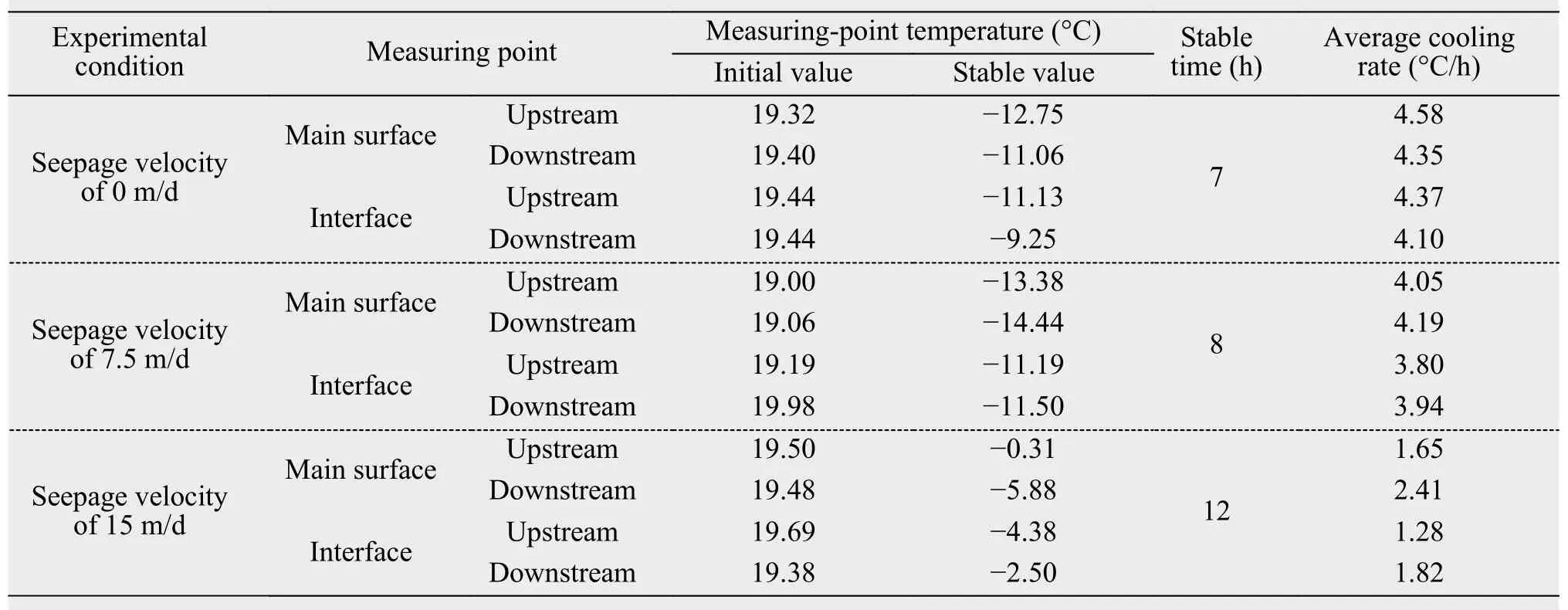
Table 3 The average cooling rate of the freezing process

3.3 Characteristics of temperature-field distribution during freezing

Based on the measured temperature data, the temperature cloud map of the H2 temperature-measurement surface is plotted; and the cloud map of the cryogenic barrier-closure process and the maximum thickness of the cryogenic barrier are studied. The distribution characteristics of the cryogenic barrier temperature field under the seepage freezing condition are analyzed.

Under the condition of 0 m/d, the cryogenic barrier reaches the state of closure after 1.3 h of freezing. It can be seen from Figure 6b, that the cryogenic barrier at this time is vertically symmetrical with the connecting line of freezing pipes. At the same time, there are some differences in the range of frozen-soil columns that are formed by the three freezing pipes; and the freezing speed on the right is slightly faster, showing the soil layers around the three freezing pipes or the experimental conditions are not completely uniform. After 8 hours of freezing, the thickness of the cryogenic barrier basically developed slowly. From Figure 6d, it can be seen that the cryogenic barrier is also vertically symmetrical with the connecting line of the freezing pipes, and the shape of the cryogenic barrier is similar to rectangular. The unilateral thickness of the cryogenic barrier is about 50 mm in the up-and-down direction and about 40 mm in the left-to-right direction, which is slightly smaller than the upstream and downstream direction and that is mainly caused by mutual influence of the freezing pipes. Comparing the temperature cloud maps of 1.3 h and 8 h, it was found that the difference of cryogenic barriers in the left-to-right direction is gradually narrowing. After 8 hours of freezing, the cryogenic barriers in the left-to-right direction are also nearly symmetrical. As the cryogenic barriers develop, the differences in their shapes are gradually reduced; and the shape of the cryogenic barrier is mainly affected by the configuration of the freezing pipes.

With a seepage velocity of 7.5 m/d, the cryogenic barrier reached the state of closure after freezing 3.6 h. From Figure 7b, it can be seen that the cryogenic barrier at this time is no longer vertically symmetrical with the connecting line of freezing pipes. The section of cryogenic barrier formed by the single freezing pipe is elliptic, and the development speed of the cryogenic barrier in the downstream is larger than that in the upstream. In addition, the temperature within the soil in a wide range of cryogenic barrier in the downstream (150 mm away from the freezing pipe) is affected by the freezing pipe; the temperature within the soil in the upstream is only affected by the freezing pipe in the smaller range (50 mm away from the freezing pipe). The temperature of a majority of the soil in the upstream is the initial temperature during the test and that is mainly caused by a steady flow of groundwater erosion, making the freezing pipe temperature significantly reduced. Under the influence of low-temperature groundwater, the temperature of a wider area of soil declines in the downstream cryogenic barrier. The cryogenic barrier is basically stable and no longer develops after freezing for 8 h. From Figure 7d, it is found that the shape of the cryogenic barrier is somewhat heart-shaped. The unilateral thickness of the upstream barrier is 50 mm, while the thickness of the downstream barrier is about 100 mm, twice that of the upstream. The unilateral thickness of the cryogenic barrier on the left and right sides is about 30 mm, which is much smaller than the thickness on the upstream and downstream sides of the barrier. With the blocking of the cryogenic barrier andthe flow of groundwater around the barrier, the velocity of the groundwater increases on the left and right sides of the barrier and makes it more difficult for the thickness to increase on either side of the cryogenic barrier.

Under the condition of a seepage velocity of 15 m/d, the cryogenic barrier reaches the state of closure after freezing for 8 h. From Figure 8b, it can be seen that the asymmetry of the cryogenic barrier is more obvious. The influence of the freezing pipe in the upstream of the cryogenic barrier is further reduced. After 12 h of freezing, the cryogenic barrier is basically stable. As shown in Figure 8d, the cryogenic barrier is heart-shaped and symmetrical in the left-toright direction. The thickness of the cryogenic barrier is significantly less than that of the above two group experiments, and the thickness of the cryogenic barrier has become more uneven. The unilateral thickness of the upstream barrier is only about 20 mm, and the unilateral thickness of the downstream barrier is uneven. The unilateral thickness of the No. 2 freezing pipe is about 60 mm, and its thickness is 40% and 60% under the seepage velocity of 0 m/d and 7.5 m/d, respectively. By contrast, the unilateral thickness of the No. 1 and No. 3 freezing pipes is just 20 mm, and the thickness is 20% and 40% under the seepage velocity of 0 m/d and 7.5 m/d. Thus it can be seen that seepage on the outside of the cryogenic barrier erosion is very significant.

Figure 6 Temperature isogram with seepage velocity of 0 m/d

4 Conclusions

(1) Under the influence of seepage, the flow brings a lot of heat, causing the temperature distribution to no longer be symmetrical. The upstream temperature is significantly higher than that downstream; and with increase in the seepage velocity, this asymmetry becomes more obvious, with the asymmetry of the interface temperature distribution being larger than that of the main surface.

(2) Under the influence of seepage, the average cooling rate of the soil gradually decreases with the increase of the seepage velocity; and the time to reach the equilibrium state progressively becomes longer. In the range of 25 mm upstream and downstream beside the freezing pipe column, the average cooling rate is about 4.35 °C/h without seepage. Under the condition of seepage velocity of 7.5 m/d and 15 m/d, the average cooling rate is about 3.96 °C/h and 1.72 °C/h, respectively. The condition of seepage has a great influence on the development of the cryogenic barrier, especially under the condition of high seepage velocity, and the development of the cryogenic barrier is greatly hindered.

Figure 7 Temperature isogram with seepage velocity of 7.5 m/d

Figure 8 Temperature isogram with seepage velocity of 15 m/d

(3) Under the condition of a seepage velocity of 15 m/d, the maximum stable thickness of the cryogenic barrier is about 60 mm; and its thickness is 40% and 60% less than that of the seepage velocity of 0 m/d and 7.5 m/d, respectively. The minimum thickness is only 20 mm, and its thickness is 20% and 40% under the seepage velocity of 0 m/d and 7.5 m/d, respectively. Affected by seepage, erosion outside of the cryogenic barrier is very significant. Thus focusing on monitoring the edge of the thickness of the barrier is important to ensuring construction safety.

Acknowledgments:

This work is financially supported by the National Natural Science Foundation of China (No. 41201070), Project of Education Department of Jiangxi Province (GJJ14494), Development Fund Project of State Key Laboratory of Frozen Soil Engineering (SKLFSE 201508), and Development Fund Project of State Key Laboratory for Geomechanics & Deep Underground Engineering (SKLGDUEK1505).

Askar Z, Zhanbolat S, 2015. Experimental investigations of freezing soils at ground conditions of Astana, Kazakhstan. Sciences in Cold and Arid Regions, 7(4): 399–406. DOI: 10.3724/SP.J.1226.2015. 00399.

Chen RJ, Cheng GD, Li SX,et al., 2000. Development and prospect of research on application of artificial ground freezing. Chinese Journal of Geotechnical Engineering, 22(1): 40–44.

Feng MM, Yang WH, Gao J, 2014. Research on distribution law of frozen temperature field of fractured rock mass with groundwater seepage. Journal of Mining & Safety Engineering, 31(6): 976–981.

Hu J, Yang P, 2015. Numerical analysis of temperature field within large-diameter cup-shaped frozen soil wall. Rock and Soil Mechanics, 2: 523–531.

Ji ZQ, Xu XY, 2009. Thermal analysis of artificially frozen wall in seasonal frozen area. Rock and Soil Mechanics, 30(4): 971–975.

Jumikis A, 2006. Thermal Soil Mechanics. New York: Rutgers University Press, pp. 1966.

Jung YB, Park ES, Chung SK,et al., 2011. Coupled hydro-thermal modeling of ice ring formation around a pilot LNG cavern in rock. Engineering Geology, 118(3–4): 122–133. DOI: 10.1016/j.enggeo. 2010.12.005.

Liu B, Li Y, Dai HD,et al., 2012. Study on temperature field distribution law of freezing wall for inclined shaft. Coal Science and Technology, 40(12): 4–7. DOI: 10.13199/j.cst.2012.12.10.liub.012. (in Chinese)

Mao WN, Liu JK, 2013. Different discretization method used in coupled water and heat transport mode for soil under freezing conditions. Sciences in Cold and Arid Regions, 5(4): 413–417. DOI: 10.3724/SP.J.1226.2013.00413.

Shi EC, Gong YR, Jeong CG, 2015. Field application of freezing technology for social infrastructures. Sciences in Cold and Arid Regions, 7(5): 637–644. DOI: 10.3724/SP.J.1226.2015.00637.

Wang CH, Shi R, Cui Y,et al., 2009. Simulation analysis on characteristics of land surface over western Qinghai-Xizang Plateau during freezing-thawing period. Sciences in Cold and Arid Regions, 1(4): 329–340.

Xu XY, Ji ZQ, Zhang CX, 2010. Model test of frozen soil wall beneath seasonally frozen soil layer. Rock and Soil Mechanics, 31(6): 1705–1708.

Yao ZS, Chen J, 2006. Physical simulation study on temperature field of underwater freezing project. Coal Science and Technology, 34(10): 24–28.

Yuan YH, Yang P, Jiang TQ, 2010. Study of thermal field of soil freezing in shallow covered tunnel with subsurface excavation passing through ground with thin aquifer under complex conduction. Rock and Soil Mechanics, 31(S1): 388–393.

Zhang M, Wang LM, Wang BW,et al., 2011. Horizontal freezing study for cross passage of river-crossing tunnel. Sciences in Cold and Arid Regions, 3(4): 314–318. DOI: 10.3724/SP.J.1226. 2011.00314.

Zhou XM, He ZP, Ji HG, 2011. Design method of freezing rock wall under high water pressure. Journal of China Coal Society, 36(12): 2121–2126. DOI: 10.13225/j.cnki.jccs.2011.12.029. (in Chinese)

:Lao LY, Ji ZQ, Huang LL, et al., 2017. Research on the temperature field of a partially freezing sand barrier with groundwater seepage. Sciences in Cold and Arid Regions, 9(3): 0280–0288.

10.3724/SP.J.1226.2017.00280.

December 10, 2016 Accepted: January 10, 2017

*Correspondence to: ZhiQiang Ji, East China University of Technology. No. 418, Guanglan Road, Changbei Economic Development Zone, Nanchang, Jiangxi 330133, China. E-mail: 328910681@qq.com