费米子隧穿辐射研究

陈兵兵

(四川民族学院数学系，四川康定 626001)



费米子隧穿辐射研究

陈兵兵

(四川民族学院数学系，四川康定 626001)

本文在对标量粒子和矢量粒子隧穿辐射研究的基础上探讨了费米子的隧穿辐射。首先对霍金辐射研究作了综述，然后讨论了狄拉克方程，利用WKB近似，研究了二维时空中费米子的隧穿辐射，通过对隧穿率的讨论，最后求得黑洞的霍金温度。研究结果表明：黑洞的霍金温度同样可以通过费米子的隧穿行为得到。

二维时空；费米子；狄拉克方程

霍金利用弯曲时空的量子观点证明了黑洞存在热辐射现象[1]，在此基础上，人们运用不同的方法对黑洞的热力学性质进行了广泛、深入的探讨。其中一种有效的方法就是Kraus和Wilczek采用的半经典隧穿[2]，之后，Wilczek和Parikh对Kraus-Wilczek量子隧穿方法进行了改进，运用半经典的WKB近似来讨论隧穿辐射谱。同时，半经典的哈密顿-雅克比方法也被提出，这一方法极大简化了隧穿方法的计算。利用这些方法，研究者讨论了稳态黑洞的隧穿辐射，并且进行了一系列的推广，研究了标量粒子和矢量粒子的隧穿辐射[3-5]，对出射粒子具有质量和带点情况也作了讨论[6-10]。研究表明，他们所得到的结论与Parikh等人的结论一致。

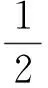
1 狄拉克方程

通过修改海森堡代数的基本对易关系式，可得到一个重要的(GUP)模型

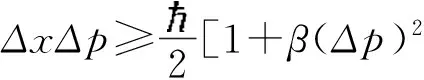
(1)

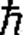
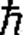
(2)

其中，xi和pi可以表示为

xi=x0i，

pi=p0i(1+βp2)，

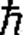
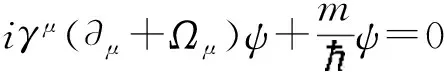
(4)

(5)

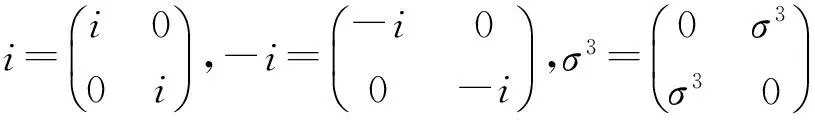
2 费米子隧穿辐射

通过讨论Jackiw-Teitelboim(JT)模型可以得到二维引力理论，该模型作用量如下[8]

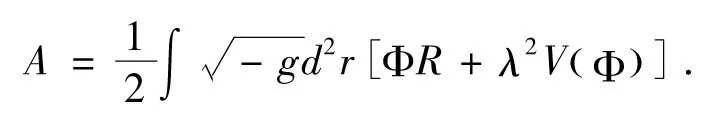
(6)

其中，V(Φ)=(a+1)Φa,a>-1，λ是二维宇宙常数，Φ是一个标量场，该作用量产生Ads空间的二维黑洞解如下

(7)

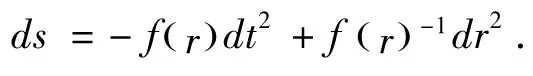
(8)

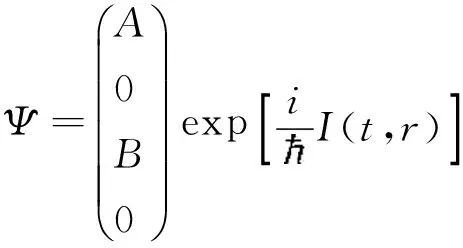
(9)

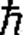
(10)

为了能求解出粒子作用量的表达式，同时基于时空特性考虑，对作用量进行变量分离

I=-ωt+(r).

(11)

其中，ω是隧穿粒子的能量。将(11)带入(10)，同时消去A、B可得

(12)

讨论事件视界处并积分可得

(13)

在(13)式中，+(-)分别表示出射解(入射解)，讨论事件视界，令

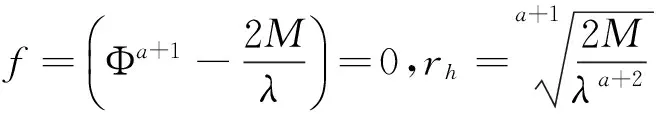
(14)

(14)式可表示为

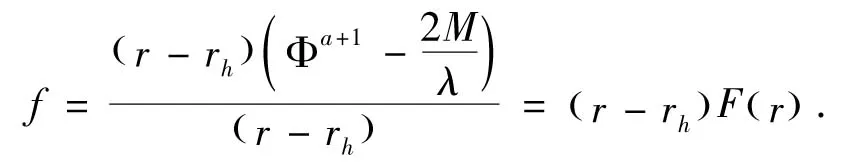
(15)

将(15)式带入(13)式求解可得

.

(16)

在经典极限下进入黑洞的粒子几率要统一，即黑洞可以将视界附近的所有物质都吸收进去[12]，那么在黑洞视界吸收率P(absorption)=1。因此，费米子在视界处的隧穿率为

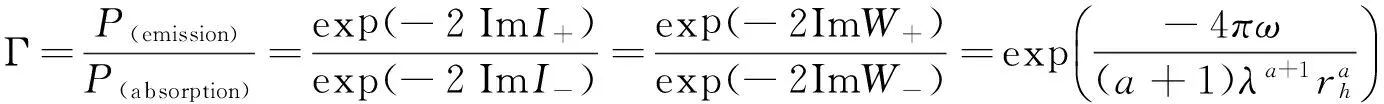
(17)

根据玻尔兹曼关系式Γ∝exp(-βE)，其中β是宇宙视界处的逆变温度，与温度的关系是β=T-1，E为粒子的能量，因此黑洞视界处标准霍金温度为

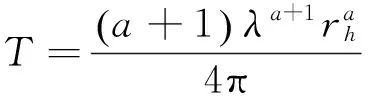
(18)

3 结论

综上所述，本文研究了费米子在二维时空中的隧穿行为。费米子的运动遵循狄拉克方程，利用WKB近似，我们讨论了费米子在二维黑洞中的隧穿率，然后由得到的隧穿率可以求得黑洞的霍金温度。通过研究表明，黑洞的霍金温度可以通过讨论费米子隧穿效应求得，黑洞隧穿率与黑洞的质量等因素有关。

[1]Hawking S W.Particle creation by Black Holes[J].Commun Math Phys,1975,43(3):199-220.

[2]Parikh M P,Wilczek F.Hawking radiation as tunneling[J].Phys.Rev.Lett,2000,85(24):5042-5045.

[3]Kraus P,Wilczek F.Self-interaction correction to Black Hole radiance[J].Nucl.Phys.B,1995:403-420,433.

[4]Kerner R，Mann R B.Tunnelling,temperature and Taub-NUT Black Holes[J].Phys.Rev.D,2006,73(10):381-385.

[5]Zhang J Y,Zhao Z.Hawking radiation of charged particles via tunneling from the Reissner-Nordstrom Black Hole[J].Journal of High Energy Physics,2005(10):55-61.

[6]Jiang Q Q.Dirac Particles’ tunneling from Black Rings[J].Physical Review D Particles &Fields,2008(4):298-317.

[7]Link,Yang S Z.Fermion tunneling from Higher-Dimensional Black Holes[J].Phys.Rev.D,2009(6):064035.

[8]Cadoni M,Mignmi S．Entropy of 2D Black Holes from counting microstates[J].Phys.Rev.D,1995(8):088501.

[9]Chen D Y,Wu H W,Yang H T.Fermion’s tunnelling with effects of quantum gravity[J].Advances in High Energy Physics,2013(24):412-421.

[10]Zeynali K,Darabi F,Motavalli H.Black Hole thermodynamics and modified GUP consistent with doubly special relativity[J].Modern Physics Letters A,2012(45):345-351.

[11]Nozari K,Karami M.Minimal,length and generalized Dirac equation[J].Modern Physics Letters A,2005(20): 3095-3099.

[12]Mitra P.Hawking temperature from tunnelling formalism[J].Phys.Lett.B,2007(2-3):240-242.

Research on the Fermions’ Tunneling Radiation

CHEN Bing-bing

(Department of Mathematics,Sichuan Minzu College,Kangding Sichuan 626001,China)

The tunneling radiation of scalar particles and vector particles has been investigated.In this paper we discuss the fermions’ tunneling radiation.First of Hawking radiation research are reviewed,and then discuss the Dirac equation.Using the WKB approximation,we investigate the tunneling probability in two-dimensional black hole and get the Hawking tempera-ture via the probability.The result shows that the Hawking temperature of the black hole can also be obtained by the fermion’s tunneling radiation.

2-dimensional spacetimes;fermions;Dirac equation

2016-03-22

四川省教育厅自然科学重点资助项目“高维时空中费米子的隧穿辐射研究”(15ZA0325)。

陈兵兵(1980- )，男，副教授，从事黑洞物理研究。

P145.6

A

2095-7602(2016)10-0018-03