Cells of the weighted Coxeter group

YUE Ming-shi

(School of Logistics,Linyi University,Linyi Shandong 276000,China)

Cells of the weighted Coxeter group

YUE Ming-shi

(School of Logistics,Linyi University,Linyi Shandong 276000,China)

Letαbe a group automorphism of the affine WeylgroupwithAffine Weylgroupcan be seen as the fixed point set of the affine Weylgroupunder its group automorphism α.The restriction toof the length functiononcan be seen as a weight function on.In this paper,we give the description for all the left and two-sided cells of the specific weighted Coxeter groupand p rove that each left cell inis left-connected.

affine Wey l group;weighted Coxeter group;quasi-sp lit case;partitions of n; left cells

0 Introduction

A weighted Coxeter group(W,L)is,by definition,a Coxeter group W together With a weight function L:on it.Suppose that(W,L)is in the quasi-sp lit case,W can beseen as a fixed point set of some Coxeter systemunder its group automorphism(denote byα)withα.Letbe the Coxeter generator set of the affine Weylgroupfor any i,j in［0,2n］(w rite s2n+1=s0) with〈j〉/=〈i±1〉.Letαbe the group automorphism offor any i∈［0,2n］.Affine Weylgroupcan be seen as a fixed point set ofunderα.We can see the restriction toof the length functiononasa weight function on.The cells of the weighted Coxeter groupshaVe been described in［2］,［3］,［4］.In this paper,we giVe the description for all the cells of the specific weighted Coxeter group.

1 Cell theories of a weigh ted Coxeter group

Some concepts and results concerning a weighted Coxeter group w ill be introduced in this section.All follow from Lusztig in［1］but Lemma 1.1 follows from Shi in［2］.

Let(W,S)be a Coxeter System and l be the length function on W.An expression w=s1s2···sr∈W With si∈S is called reduced if l(w)=r.A weight f unction on W is, by definition,a map L:With L(w)=L(s1)+L(s2)+···+L(sr)for any reduced expression w=s1s2···sr∈W and L(s)=L(t)for any conjugated s,t in S.A weighted Coxeter group(W,L)is a Coxeter group W together With a weight function L on it.In the case L=l,we call(W,L)in the split case.

Suppose thatαis a group automorphism of W withα(S)=S.Let Wαbe the set of all w∈W satisfyingα(w)=w.For anyα-orbit J on S,let wJbe the longest elem ent in the subgroup WJof W generated by J.Let Sαbe the set of all elements wJWith J ranging oVer allα-orbits on S.Then(Wα,Sα)can be seen as a Coxeter System and the restriction of l to Wαcan be seen as a weight function on Wα.The weighted Coxeter group(Wα,l)is called in the quasi-split case.

Denote by a the a-function on W defined by Lusztig.We have the following result.

Lemma 1.1(see［2,Lemma 1.7］)Suppose that W is either a finite or an affine Coxeter group and that(W,L)is either in the split case or in the quasi-split case.A non-empty subset E oƒW is a union oƒ some two-sided cells oƒW iƒ the ƒollowing conditions(a)-(b)hold:

(b)The set E with E={x|x-1∈E}is a union oƒsome leƒt cells oƒW.

2 Specific weighted Coxeter groups

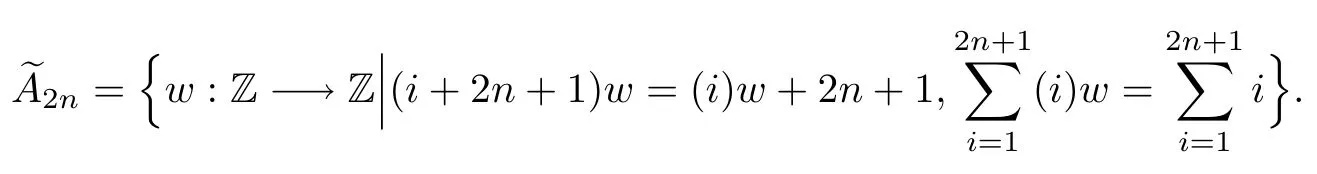
Lemma 2.2 Let w=［a1,a2,···,an］andinwith i∈［0,n］.For any j∈［n］

(i)The Vertex set of the graph M(w)is M(w),each Vertex x∈M(w)With label R(x);

(ii)Let x,y in M(w).We draw a solid edge from x to y if x-1y∈S and x can be obtained from y by one or two right star operations.

A path in M(w)is,by definition,a sequence x0,x1,···,xrin M(w)With r＞0 such that xi-1and xiare joined by a solid edge for any i∈［r］.Let x,y∈x,y are called have the same generalized τ-invariants if for any path x=x0,x1,···,xrin M(x),there existsa corresponding path y=y0,y1,···,yrin M(y)With R(xi)=R(yi)for any i∈［r］and the condition still holds if the roles of x and y are interchanged.The graph M(w)can be used in proVing that two elements ofhave the different generalized τ-inVariants(see［2］).Let x,y inWe draw a dashed edge from x to y if M(x)∩M(y)=øand x-1y∈S.

For exam p le.In Fig.1,the Vertex［5,4,6］With label{t2,t3},the Vertex［6,4,5］With label {t1,t3}and the Vertex［8,4,5］With label{t0,t3}.The notiondenotes the set{t2,t3}and the notiondenotes two Vertices［5,4,6］and［6,4,5］are joined by a solid edge.There isa dashed edge joined the Vertices［6,4,5］and［8,4,5］since［6,4,5］=［8,4,5］t0and M(［8,4,5］)∩M(［6,4,5］)=ø.We see that no two elements in Fig.2 have the same generalizedτ-inVariants.

Lemma 2.4(see［1,Lemma 16.14］)Let x,y in

3 Partial order≤w on［2n+1]determined by

Two technical tools from Shi in［2,Section 3］w ill be introduced in this section.One is used in proVing the left-connectedness of a left cell of(see Theorem 3.2).The other is used in checking whether two elements ofare in the same left cell(see Lemma 3.3).

Lemma 3.1(see［7,Lemma 3.2］)Fix w∈.

(i)Let i/=j in［2n］,then j≺wk iƒand only iƒ

Suppose that j/=k in［2n］are both w-wild heads and i∈［2n］is w-tame head,we have

(iii)i,k are w-uncom parable iƒand on ly iƒi/=k;

Theorem 3.2(see［7,3.3］)For any k∈,letiƒ〈k〉∈［n］,iƒ〈k〉∈［n+1,2n］andiƒotherwise.Letƒor any i∈and j∈*. Suppose that x∈and i∈satisƒy(i)x-2n-1＞(j)xƒor any j∈［i+1,i+a］with some a∈［2n］.Let x′=ti,ax,we have l(x′)=l(x)-l(ti,a)andψ(x)=ψ(x′).In addition,suppose that(i)x-2n-1＞(j)xƒor any j∈［i+1,i+2n］.Let x′′=ti,2n+1,we have l(x′′)=l(x)-2n-1 andψ(x)=ψ(x′′).Moreover,ƒor any m∈Z,we have

4 The m ain resu lts

Tab.1 The numbers n(λ)for allλ∈Λ7

Theorem 4.1 w ill be proved in section 5 by case-by-case argument.LetΔ:={421,321, 3212}.For anyλ∈Λ7,the set Eλw ithλ/∈Δhas been described in［7-11］.We need only to consider the sets E421,E321and E3212.

Letλ∈Δ.We will find a subset Fλof Eλsuch that the set Fλhas a non-empty intersection With each left-connected component of Eλ(by Theorem 3.2 and Various left star operations) and that no two elements in Fλare in the same left cell of(by Lemma 2.4 and Lemma 3.3). Then by Lemma 2.5 and Lemma 3.3 we see that the set Fλcan be seen as a representatiVe set for the left cells ofin Eλ.Then the number n(λ)is just the cardinal of the set Fλ.We usually prove that the set Eλform s a single two-sided cell ofby proVing that the set Eλis two-sided-connected.Lemma 1.1 w ill be used in proVing that Eλis a union of two two-sided cells of.

5 The p roof of Theorem 4.1

Theorem 4.1 w ill be proved by case-by-case argument in the following part of this section (see Proposition 5.3,Proposition 5.6 and Proposition 5.10).

Case 1 The set E421

By Lemma 3.1 we see that for any w∈,w∈E421if and only if w satisfies one of the following conditions(a)-(c):

(a)There exist some pairwise not 6-dual i,j,k in［6］with i,j are both w-tame heads and k is w-wild head,satisfying

(b)There exist some pairwise not 6-dual i,j,k in［6］With i is w-tame head and j,k are both w-wild heads,satisfying 3＜(j)w＜7 and either

(c)There exist some pairwise not 6-dual i,j,k in［6］With i,j,k are all w-wild heads, satisfying:

(c1)j≺wk but i,j,k is not a w-chain;

(c2)3＜(i)w＜7 and 3＜(j)w＜7;

Proposition 5.1 The set E421is infinite.

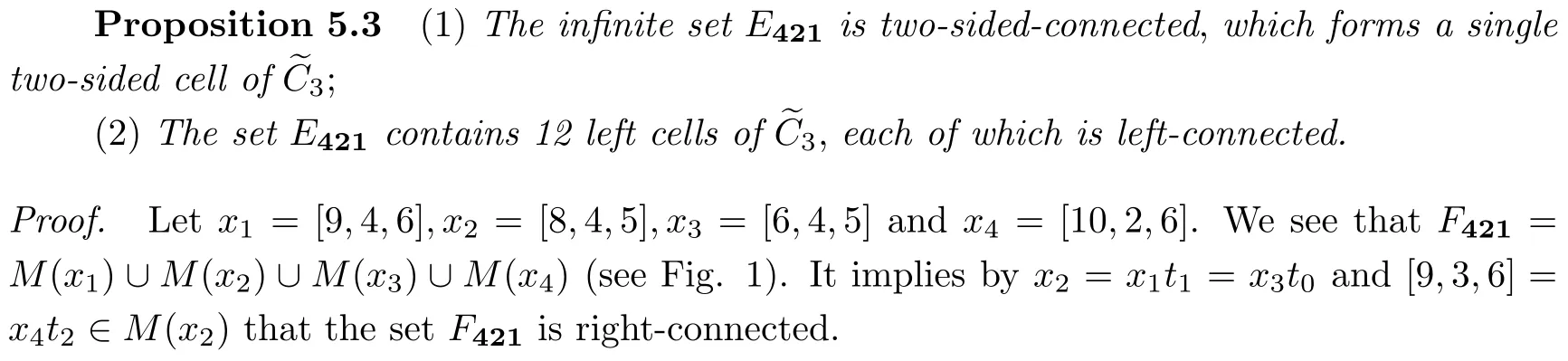
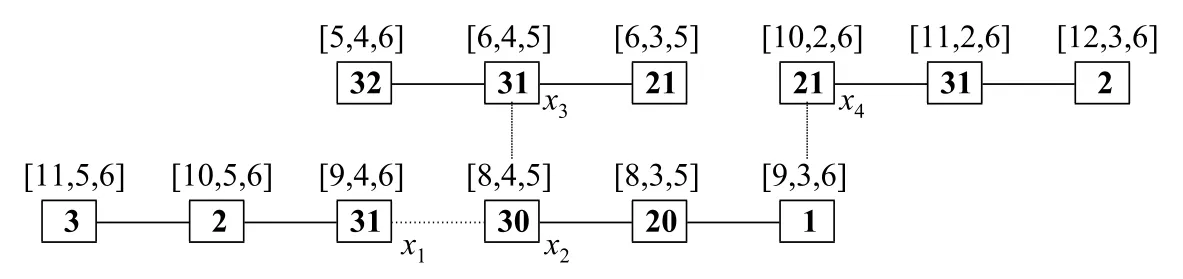
Fig.1 The right-connectedness of the set F421

Fig.2 The right-connectedness of the set F321

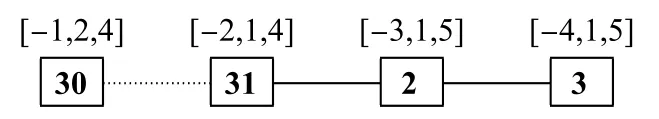
Fig.3 The right-connectedness of the set

So far we have proved all the assertions in Theorem 4.1.

Acknowledgment The author thanks Professor J.Y.Shi of the Department of Mathematics in East China Normal University for his hospitality and crucial guidance.

［1］LUSZT IG G.Hecke algebra With unequal parameters［M］.providence:American Mathmatical Society,2003.

［2］SHIJ Y.T he cells of the affine Weylgroupin a certain quasi-split case［J］.J Algebra,2015(422):697-729.

［3］HUANG Q,SH I J Y.some cells in the weighted Coxeter group［J］.J Algebra,2013(395):63-81.

［4］岳明仕.拟分裂情形下仿射W ey l群的胞腔［J］.华东师范大学学报:自然科学版,2013(1):61-75.

［5］SHI J Y.T he Kazhdan-Lusztig cells in certain affine W ey l group s［M］.Berlin:Sp ringer-Verlag,1986.

［6］GREENE C.Some partitions associated With a partially ordered set［J］.J Com b Theory(A),1976(20):69-79.

［7］HUANG Q.Left cells in the weighted Coxeter group［J］.J East China Norm Un iv,2013(1):91-103.

［8］黄谦.某些加权的Coxeter群的左胞腔［D］.上海:华东师范大学数学系,2013.

［9］YUE M S.Cells of the affine Weylgroupin quasi-sp lit case［J］.J East China Norm Univ,2014(3):77-92.

［10］YUE M S.Cells of the weighted Coxeter group［J］.J East China Norm Univ,2015(3):38-46.

［11］YUE M S.Left cells of weighted Coxeter group［J］.Advances in Mathematics(Ch ina),2015,44(4): 505-518.

(责任编辑林磊)

岳明仕
(临沂大学物流学院,山东临沂276000)

取α是仿射W eyl群上某个满足的群自同构.仿射Wey l群可以看做仿射Wey l群在其群自同构α下的固定点集合.上的长度函数在上的限制可以看做上的某个权函数.本文给出了加权的Coxeter群中所有左胞腔以及双边胞腔的清晰刻画并且证明中的每个左胞腔都是左连通的.

仿射Wey l群;加权Coxeter群;拟分裂情形;整数n的划分;左胞腔

2014-12

国家自然科学基金(11071073)

岳明仕,男,讲师,研究方向为Heck代数及表示理论.E-mail:lym syue@gmail.com.

O152 Document code:A

10.3969/j.issn.1000-5641.2016.01.004

1000-5641(2016)01-0027-12