INITIAL BOUNDARY VALUE PROBLEMS FOR A MODEL OF QUASILINEAR WAVE EQUATION

NIE Da-yong，WANG Lei

(1.Department of Basic Sciences，Yellow River Conservancy Technical Institute，Kaifeng 475000，China）

(2.Department of Basic Sciences，University for Science and Technology of Zhengzhou，Zhengzhou 450064，China）

INITIAL BOUNDARY VALUE PROBLEMS FOR A MODEL OF QUASILINEAR WAVE EQUATION

NIE Da-yong1，WANG Lei2

(1.Department of Basic Sciences，Yellow River Conservancy Technical Institute，Kaifeng 475000，China）

(2.Department of Basic Sciences，University for Science and Technology of Zhengzhou，Zhengzhou 450064，China）

In this paper，the authors consider the IBVP for a class of second-order quasilinear wave equation.By the method of characteristic analysis，the global smooth resolvability are obtained under certain hypotheses on the initial data，which extend the result of Yang and Liu［8］.

wave equation；IBVP；global classical solution；characteristic analysis

2010 MR Subject Classification：35G31；35L50

Document code：AArticle ID：0255-7797（2016）05-1005-06

1 Introduction

In this paper we consider the initial-boundary value problems（IBVP）for the following quasilinear wave equation

where k（v）is a sufficiently smooth function such that

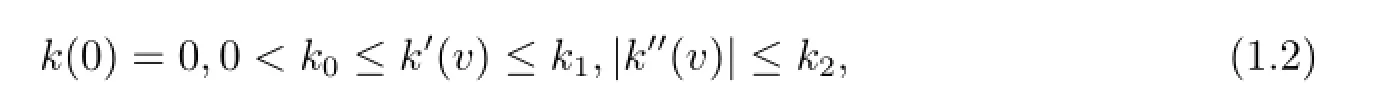
and k0，k1，k2，γ are positive constants.

Equation（1.1）arises in a variety of ways in several areas of applied mathematics and physics.When γ=0，equation（1.1）serves to model the transverse vibrations of a finite nonlinear string，for its Cauchy problem，Klainerman and Majda［1］proved that the second order derivatives of the C2solution u=u（t，x）must blow up in a finite time，Greenberg and Li［5］proved global smooth solutions do exist under the dissipative boundary condition.

For the case that γ/=0，in a significant piece of work Nishida［2］considered the initialvalue problem for（1.1），using a Riemann invariant argument，the global smooth resolvability has been proved if the initial data are small in an appropriate sense.

For other results related to（1.1）and nonlinear string equation，we may refer to［3，4，etc］.

In this paper，we consider equation（1.1）on the strip［0，1］×（0，∞）with the following initial and fixed boundary data

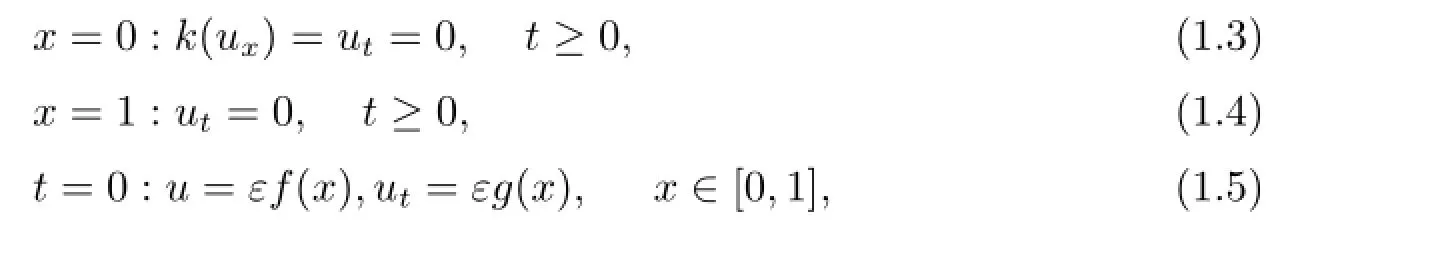
where

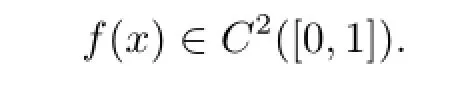
We also require the compatibility conditions

We will show that problem（1.1）and（1.3）-（1.5）admits a unique global C1solution.

2 Preliminaries and Main Theorem

If in（t，x）space we set ut=w，ux=v，then（1.1）is transformed into the dissipative quasilinear system

The eigenvalues λ1，λ2and the Riemann invariants r and s for system（2.1）are，respectively，

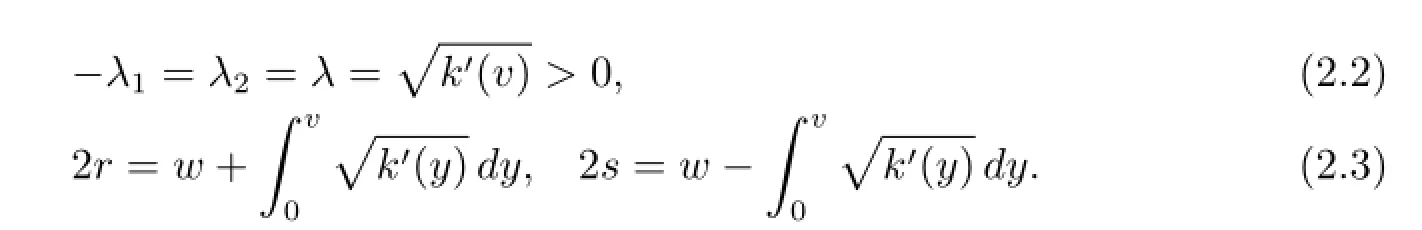
Thus problems（2.1）and（1.3）-（1.5）can be written as

where

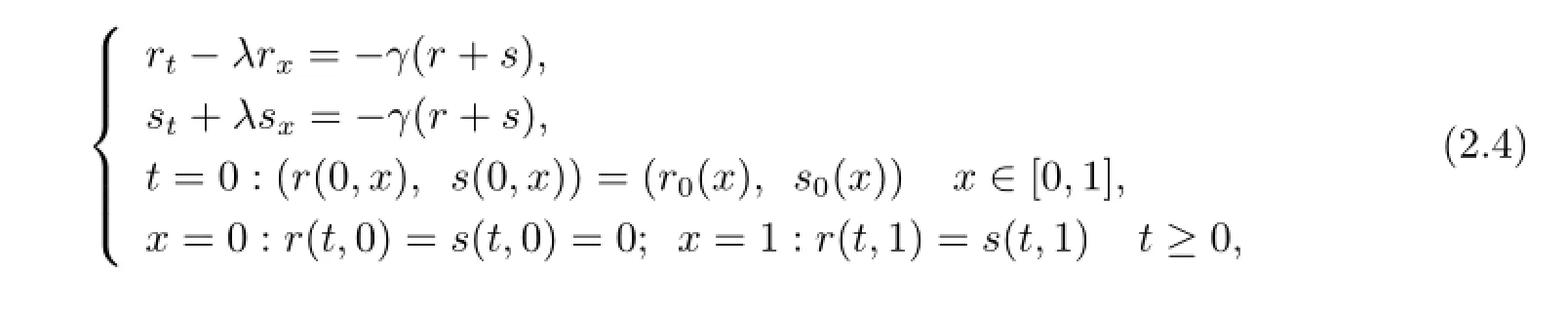
Our main result of this paper may be stated as

Theorem 2.1Assume that（1.2）and（1.6）hold，if ε is small enough，then IBVP（1.1）and（1.3）-（1.5）admits a unique global C1solution.

Remark 2.1Theorem 2.1 shows that the interior dissipative effect of the equation in guaranteeing the global existence of classical solution which is different to that of the dissipative effect of boundary in［5］.

3 Proof of Main Theorem

By the local existence theorem of smooth solutions（see［7］），we only need to establish the uniform C1estimates for the solutions of（2.4）a priori.For our purpose，we give the following lemma which play an important role in our analysis.

Lemma 3.1Let r（t，x），s（t，x）be the solution to problem（2.4），then it holds for any t≥0 that

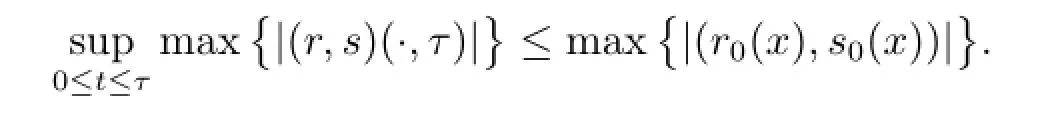
ProofLet

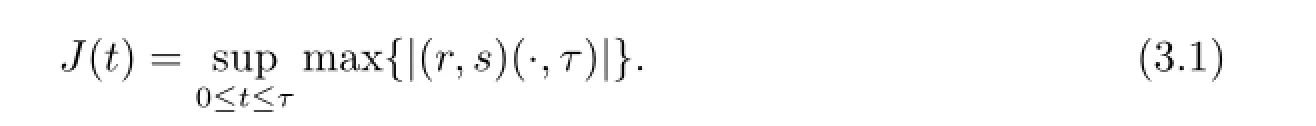
For every fixed T＞0，without loss of generality，we assume that J（t）is reached by r（t，x）first at some point

then for arbitrary（t，x）∈D，let

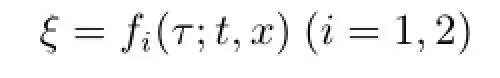
be the forward and backward characteristics passing through point（t，x），that is，

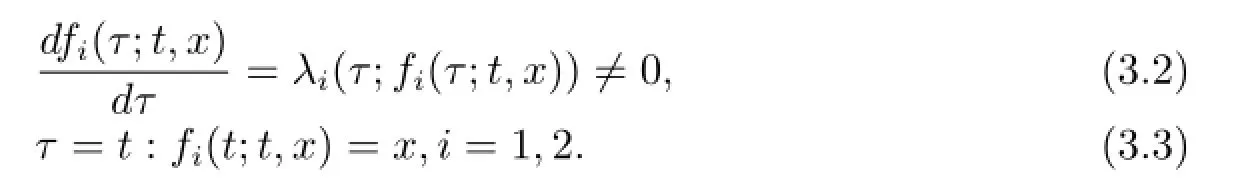
Now we discuss the backward characteristics，the other cases can be treated similarly. For the backward characteristics ξ=f2（τ；t，x），there are two possibilities.

（1）ξ=f2（τ；t，x）interacts the interval［0，1］on the x-axis at（0，x0），thus we have

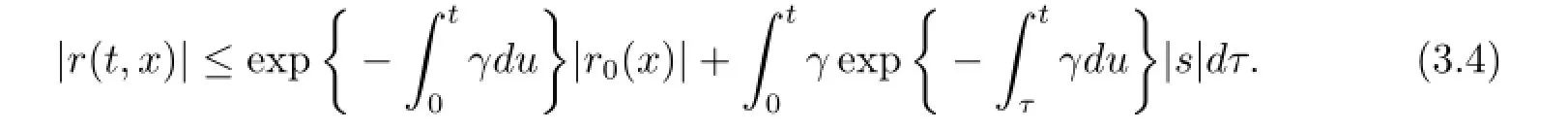
Due to

and

then it follows from（3.4）-（3.6）that

（2）ξ=f2（τ；t，x）interacts the boundary x=1 at（t1，1），then by（2.4）we have

Then from（t1，1）we draw a forward characteristic which interacts the boundary x=0 at （t2，0），along this characteristic，similar to（3.8），it holds that

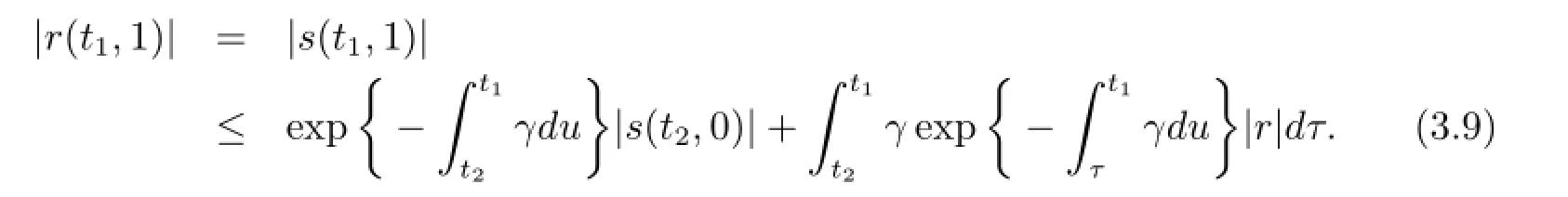
Thus，for the backward characteristic ξ=f2（τ；t2，0）passing through point（t2，0），there are still two possibilities：

（2a）the backward characteristic interacts the interval［0，1］on the x-axis；

（2b）the backward characteristic interacts the boundary x=1.

Noting that the monotonicity of the characteristic，after finite times refraction，the characteristic must interacts the interval［0，1］on the x-axis.Without loss of generality，we may assume that the backward characteristic from（t2，0）interacts the interval［0，1］at （x0，0），so we have

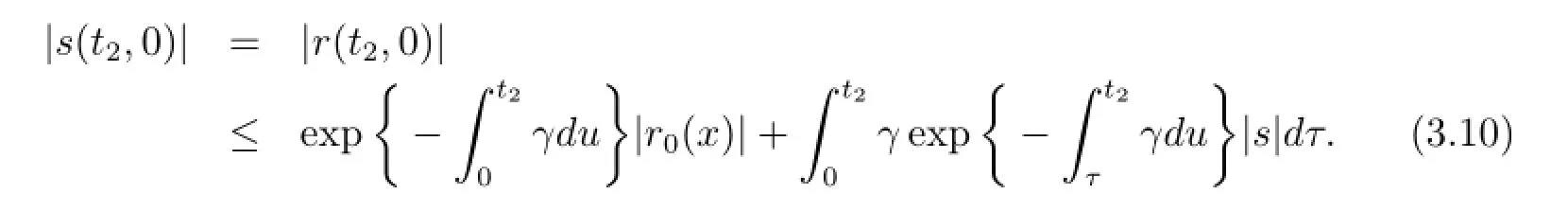
Combining（3.8）-（3.10），we can obtain

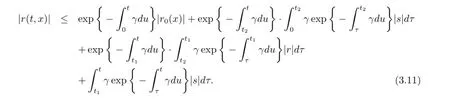
The combination of（3.1）and（3.11）yields

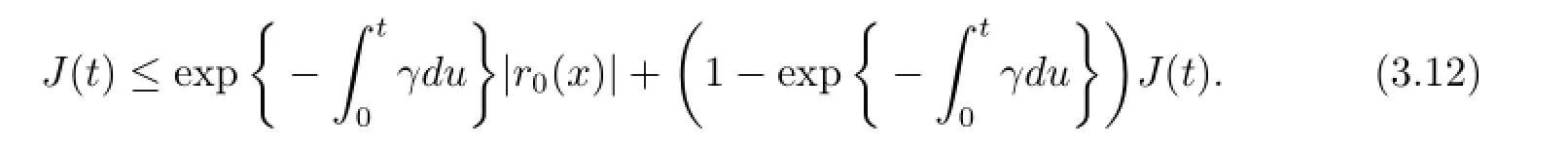
Noting that（3.5），（3.12）imply（3.7）too.

By（3.7），we immediately get the conclusion of Lemma 2.1.

Next，in order to prove Theorem 2.1 it suffices to establish a uniform a priori estimate on C0norm to the first order derivatives of the C1solution to IBVP（2.4）.To this end，we differentiate（2.4）with respect to x，it is easy to see that

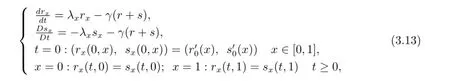
where

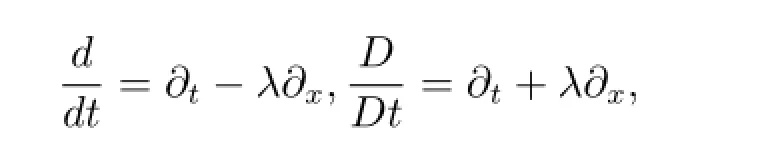
and the initial data for（rx，sx）can be easily derived from（2.3）and（2.4）.

Lemma 3.2Assume that（1.2）holds，if ε is small enough，then we have

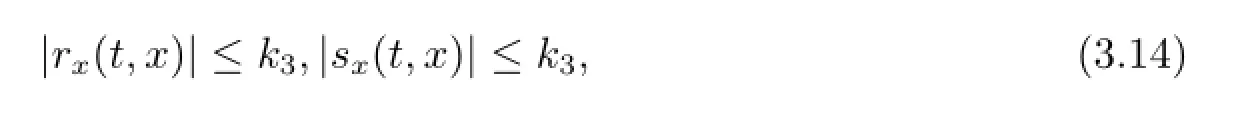
where

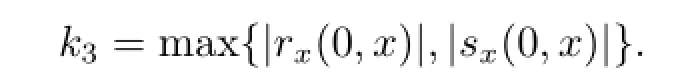
ProofNoting that（1.2），by the continuity of λ，with the help of the local result and a standard continuity argument，for the time being we suppose that

then we can use the method similar to Lemma 3.1 and easy verify the following facts

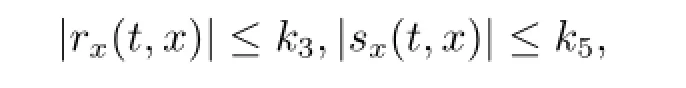
where k5＞0 is a constant，and we have，which verifies the a priori assumption（3.15）.The details will be omitted.

Applying Lemma 3.1 and Lemma 3.2，Theorem 2.1 is obtained.

Acknowledgements

The authors would like to express their sincere thanks to professor Liu Fagui for his enthusiastic and valuable suggestions.

References

［1］Klainerman S，Majda A.Formation of singularities for wave equation including the nonlinear vibrating string［J］.Comm.Pure Appl.Math.，1980，33：241-264.

［2］Nishida T.Nonlinear hyperbolic equations and related topics in fluid dynamics［J］.Nishida T.（ed.）Pub.Math.D'orsay，1978：46-53.

［3］Liu Fagui.Global classical solutions for a nonlinear systems in viscoelasticity［J］.Chinese Ann.Math.，2008，29A（5）：709-718.

［4］Li Tatsien.Global solutions to systems of the motion of elastic strings［J］.Comput.Sci.，1997：13-22.

［5］Greenberg J M，Li Tatsien.The effect of boundary damping for the quasilinear wave equation［J］.J. Diff.Equ.，1984，52：66-75.

［6］Hsiao Ling，Pan Ronghua.Initial boundary value problem for the system of compressible adiabatic flow through porous media［J］.J.Diff.Equa.，1999，159：280-305.

［7］Li Tatsien，Yu Wenci.Boundary value problems for quasilinear hyperbolic systems［M］.Durham，NC：Duke University，1985.

［8］Yang Han，Liu Fagui.Boundary value problem for quasilinear wave equation［J］.J.Math.Study，1999，32（2）：156-160.

一个拟线性波动方程模型的初边值问题

聂大勇1，王磊2
（1.黄河水利职业技术学院基础部，河南开封475000）
（2.郑州科技学院基础部，河南郑州450064）

本文研究了一类二阶拟线性波动方程的初边值问题.利用特征分析和局部解延拓的方法，在一定的假设条件下得到了经典解的整体存在性，进一步推广了杨晗和刘法贵的结果［8］.

拟线性波动方程；初边值问题；整体经典解；特征分析

MR（2010）主题分类号：35G31；35L50O175.27

date：2014-05-17Accepted date：2014-09-03

Supported by National Natural Science Foundation of China（11126323）；Key Science and Technology Program of Henan Province（142102210512）.

Biography：Nie Dayong（1982-），male，born at Dengzhou，Henan，lecturer，major in hyperbolic partial differential equations.