First Principles Investigation of Electronic Property and High Pressure Phase Stability of SmN
Ai-min Hao,Jing Bai,Shao-hua Luo,Xi-wei QiKey Laboratory of Dielectric and Electrolyte Functional Material Hebei Province,School of Resources and Materials,Northeastern University at Qinhuangdao,Qinhuangda 066004,China(Dated:Received on July 7,2015;Accepted on October 8,2015)
First Principles Investigation of Electronic Property and High Pressure Phase Stability of SmN
Ai-min Hao∗,Jing Bai,Shao-hua Luo,Xi-wei Qi
Key Laboratory of Dielectric and Electrolyte Functional Material Hebei Province,School of Resources and Materials,Northeastern University at Qinhuangdao,Qinhuangda 066004,China
(Dated:Received on July 7,2015;Accepted on October 8,2015)
An investigation of electronic property and high pressure phase stability of SmN has been conducted using first principles calculations based on density functional theory.The electronic properties of SmN show a striking feature of a half metal,the majority-spin electrons are metallic and the minority-spin electrons are semiconducting.It was found that SmN undergoes a pressure-induced phase transition from NaCl-type(B1)to CsCl-type structure(B2)at 117 GPa.The elastic constants of SmN satisfy Born conditions at ambient pressure,indicating that B1 phase of SmN is mechanically stable at 0 GPa.The result of phonon spectra shows that B1 structure is dynamically stable at ambient pressure,which agrees with the conclusion derived from the elastic constants.
First principles calculation,Electronic property,Phase stability,Elastic constants
I.INTRODUCTION
Anomalous physical properties of the rare-earth nitrides(RE-Ns)have attracted numerous attentions for several decades.The emergence of the spintronics field provides new interest in this class of materials because of their unique magnetic and electronic properties[1,2].
There have been a lot of theoretical and experimental studies on the electronic structures of the RE-Ns.Aerts et al.systematically studied the electronic structures of the RE-Ns using ab initio self-interaction corrected local spin density approximation(LSDA),results showed that these materials had a broad range of electronic properties including forming a various class of halfmetallic magnets[3].Preston et al.investigated the electronic structures of DyN and SmN using LSDA+U method,results showed that the band gap of SmN was zero[4].Larson et al.investigated the electronic structures of the RE-Ns in B1 structure using density functional theory calculations within the LSDA+U method and found that the early members of the RE-Ns before GdN(with the exception of NdN)are half-metals,while the later members after GdN have a small indirect gap[5].
SmN is reported to be antiferromagnetic with a very small net moment below 25 K,which is the earliest magnetic investigation of SmN[6].Subsequently,a neutron scattering study suggested a ferromagnetic phase with near cancellation between the spin and orbital moment[7].Very recently,Anton et al.experimentally and theoretically investigated spin/orbit magnetic property. Their results show that SmN is ferromagnetic with its net magnetic moment of 0.03µBper formula unit[8]. The near-zero moment is a result of the nearly equal and opposing spin and orbital moments in the ground state of Sm3+ion.
The pressure-induced structure transition of the RE-Ns has been an interesting topic for the last two decades.Vaitheeswaran et al.investigated the structural phase stability of LaN,and it was predicted that LaN undergoes a transition from B1 to B2 structure at 27 GPa[9].CeN was theoretically predicted to transform from B1 to B2structure at 62 GPa[10].The structural and elastic properties of CeN and TbN under high pressure have been investigated using two body inter ionic potential theory,result showed that CeN and TbN exhibited a structural phase transition from B1 to B2 structure at 88 and 136 GPa,respectively[11]. The pressure-induced structure transformation of PmN was studied using first-principles tight-binding linear muffin-tin orbital method,and it was predicted a structural phase transformation from B1 to B2 structure at 3.4 GPa[12].Jakobsen et al.experimentally and theoretically investigated high pressure behavior of TbN[13].Ciftci et al.theoretically investigated the elastic and thermodynamic properties of LaN[14].Hao et al.studied the elastic properties of NdN using firstprinciples calculations[15].Elastic properties and hardness of lanthanide nitrides in B1 structure were investigated using first-principles calculations[16,17].The result reveals that the ligand field stabilization energy and lanthanide contraction play an important role in d-etermining the hardness of lanthanide nitrides in B1 structure.
To the best of our knowledge,the pressure-induced structure transition of SmN has not been investigated up to now.In this work,we discuss the electronic property and high pressure phase stability of SmN.
II.COMPUTATIONAL DETAILS
The calculations were performed using the planewave pseudopotential method as implemented in the CASTEP code[18].For all calculations in the present work,the norm-conserving pseudopotential was employed to model the ion-electron interaction.The exchange-correlation functional was treated by the generalized gradient approximation(GGA)of Perdew-Burke-Ernzerhof(PBE)[19].The energy cutoff of the plane-wave basis was set to 770 eV.Integration in the Brillouin zone was performed using the Monkhorst method with 4×4×4[20].The chosen plane-wave cutoff and numbers of k points were carefully checked to ensure good convergence.
The self-consistent convergence accuracy was set to be 5.0×10-6eV/atom.The convergence criterion for the maximal force between atoms was 0.01 eV/˚A.The maximum displacement was 5.0×10-4˚A,and the stress was set to be 0.02 GPa.For a given external hydrostatic pressure,lattice constants and internal coordination were fully relaxed.
III.RESULTS AND DISCUSSION
A.Electronic property
SmN is a strongly correlated system with 4f electrons. 4f bands are generally very narrow,which significantly differs from the bands dominated by s,p,and d states. Therefore there exist strong on-site Coulomb repulsions among the highly localized f electrons[21].In the process of electronic structure calculations,the GGA+U(U=6.0 eV)method is used to correct the strong onsite Coulomb repulsion of Sm 4f states.
We have carried out spin-polarized electronic structure calculations of SmN in B1 structure.The result is shown in Fig.1.It can be seen that an overlap of the majority-spin bands and an energy gap of 0.9 eV of the minority-spin bands coexist at Fermi level,indicating that SmN is a half metal.A striking feature of a half metal is that the minority-spin band structure has a semiconductor gap straddling the Fermi level,whereas the majority-spin band structure has metallic intersections[22].Our results are in good agreement with those of Aerts et al.[3]and Larson et al.[5].
Figure 2 presents the partial density of states(DOS)of SmN in B1 structrue.Just below the Fermi level EF,the bands are predominantly due to N2p states with a substantial hybridization with Sm 5d and 4f states. Sm4f majority-spin electrons create an exchange filed that leads to a N2p small spin splitting of 0.2 eV[23]. Above EFare mainly Sm p-f states with a substantial hybridization with N s and p states.It was found that p-f hybridized states play a main role in the electronic bonding.

FIG.1 Band structures of SmN in B1 structure.(a)Majority-spin states and(b)minority-spin states.

FIG.2 Partial density of states of SmN in B1 structure.(a)N atom and(b)Sm atom.The vertical dash line at zero indicates Fermi level.
The occupied majority-spin f bands fall below N2p bands and push Sm5p bands lower in energy.The unoccupied majority-spin f bands sit just above EF.There are two Sm f peaks between 5.0 and 8.0 eV,which are the unoccupied minority-spin bands.
A very large splitting of about 13.0 eV between Sm4f majority-spin and minority-spin states is responsible for the spin magnetic moments.A small spin splitting ofSm5d states implies that it has a weak contribution to the spin magnetic moments.It is the occupancy of the highly localized 4f states that determines the magnetic properties,while other electronic properties are principally determined by the itinerant s-d electrons[24].
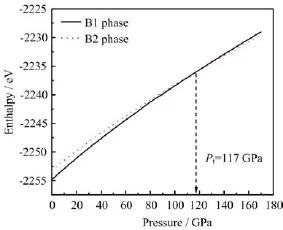
FIG.3 Calculated enthalpies as a function of pressure for B1 and B2 phases of SmN.

FIG.4 The phonon spectra of SmN at 0 GPa.(a)B1 structure and(b)B2 structure.

TABLE I Calculated elastic constants Cij(GPa),bulk modulus B(GPa)and shear modulus G(GPa)of SmN at 0 GPa,together with the previous result for comparison.
B.High pressure phase stability
Here,we calculated the phase transition pressure of SmN.Our computational approach is based on constant-pressure static quantum mechanical calculations at T=0 K.The relative stability of different phases can be deduced from the pressure dependence of the enthalpy instead of the Gibbs free energy[25,26].The pressure corresponding to∆H=H-HB1approaching zero is the phase transition pressure(Pt).At a pressure higher than the predicted transition pressure,B1 phase becomes thermodynamically unstable while B2 phase becomes thermodynamically stable.The result shows that the transition pressure is 117 GPa,as shown in Fig.3.
C.Elastic constants and phonon spectra
To check the mechanical stability,we calculated the elastic constants of SmN at 0 GPa using the“volumeconserving technique”.The results are presented in Table I.As can be seen from Table I,our result is in reasonable agreement with the previous calculations[16].
The mechanical stability criteria(Born conditions)for a cubic crystal system are given by[27]∶

From Table I,it can be seen that the elastic constants of SmN in B1 structure at 0 GPa satisfy Born conditions,indicating that B1 structure is mechanically stable at 0 GPa.While the elastic constants of B2 structure at 0 GPa do not satisfy Born conditions,indicating that B2 structure is not mechanically stable at 0 GPa.
To check the dynamic stability,we calculated the phonon spectra of SmN in B1 and B2 structures at 0 GPa.The result is shown in Fig.4.It can be seen from Fig.4(a)that there is not any imaginary frequency in the phonon spectra of SmN in B1 structure at 0 GPa. Therefore,our result shows that B1 structure is dynamically stable,which is in agreement with the result of elastic constants.From Fig.4(b),it can be seen that there are several imaginary frequencies in the phonon spectra of B2 structure,indicating that B2 structure is dynamically unstable at 0 GPa.
IV.CONCLUSION
A computational study of the electronic property and pressure-induced structure transition of SmN is reported.The result of electronic structure shows that an overlap of the majority-spin bands and an energy gap of 0.9 eV of the minority-spin bands coexist at Fermi level,indicating that SmN is a half metal.The result of relative enthalpy versus pressure indicates that SmN undergoes a pressure-induced phase transition from B1 to B2 structure.The transition pressure is determined to be 117 GPa.Our result reveals that the elastic constants of B1 structure satisfy Born conditions,indicating that B1 structure is mechanically stable at 0 GPa.There is not any imaginary frequency in the phonon spectra of B1 structure,indicating that B1 structure is dynamically stable at 0 GPa.This conclusion is in agreement with that derived from elastic constants.
V.ACKNOWLEDGMENTS
This work was supported by the Natural Science Foundation of Hebei Province(No.A2014501010 and No.E2013501135),the Program for New Century Excellent Talents in University(No.NCET-10-0304),and the Special Fund for Basic Scientific Research of Central Colleges,Northeastern University(No.N100123003 and No.N120523001).
[2]S.A.Wolf,D.D.Awschalom,R.A.Buhrman,J. M.Daughton,S.Von Molnar,M.L.Roukes,A.Y. Chtchelkanova,and D.M.Treger,Science 294,1488(2001).
[3]C.M.Aerts,P.Strange,M.Horne,W.M.Temmerman,Z.Szotek,and A.Svane,Phys.Rev.B 69,045115(2004).
[4]A.R.H.Preston,S.Granville,D.H.Housden,B.Ludbrook,B.J.Ruck,H.J.Trodahl,A.Bittar,G.V. M.Williams,J.E.Downes,A.DeMasi,Y.Zhang,K. E.Smith,and W.R.L.Lambrecht,Phys.Rev.B 76,245120(2007).
[5]P.Larson,W.R.L.Lambrecht,A.Chantis,and M.van Schifgaarde,Phys.Rev.B 75,045114(2007).
[6]F.Hulliger,Handbook on the Physics and Chemistry of Rare Earths,New York:North-Holland,(1978).
[7]R.Moon and W.Koehler,J.Magn.Magn.Mater.14,265(1979).
[8]E.M.Anton,B.J.Ruck,C.Meyer,F.Natali,H.Warring,F.Wilhelm,A.Rogalev,V.N.Antonov,and H. J.Trodahl,Phys.Rev.B 87,134414(2013).
[9]G.Vaitheeswaran,V.Kanchana,and M.Rajagopalan,Solid State Commun.124,97(2002).
榆阳区是榆林市政府所在地,地处毛乌素沙漠和黄土高原接壤地带。境内有粮食作物3类、21种、126个品种,草本植物60多种,木本植物40多种,栽培作物79种,羊子饲养规模居陕西省前列,猪、鸡、牛的饲养也初步形成气候,已建成全省畜牧业基地和舍饲养羊示范县区,极具特色的农副土畜产品为生态产业开发提供了丰富的资源;四大农业主导产业---玉米、马铃薯、羊子、生猪都处于低利润原料出售的现状,与产业化开发可以得到的实际利润相差甚远,为生态产业开发提供了极大的利润空间。因此,大力发展林果、林牧、种植、养殖等林业生态产业,促进产业升级开发,是解决“三农”问题的有效途径。
[10]A.Svane,Z.Szotek,W.M.Temmerman,J.L.Lægsgaard,and H.Winter,J.Phys.:Condens.Matter 10,5309(1998).
[11]A.Rukmangad,M.Aynyas,and S.P.Sanyal,Indian J. Pure Appl.Phys.47,114(2009).
[12]P.Pandit,V.Srivastava,M.Rajagopalan,and S.P. Sanyal,Physica B 403,4333(2008).
[13]J.M.Jakobsen,G.K.H.Madsen,J.E.Jøgensen,J. Staun Olsen,and L.Gerward,Solid State Commun. 121,447(2002).
[14]Y.O.Ciftci,K.C¸olakoglu,E.Deligoz,and H.Ozisik,Mater.Chem.Phys.108,120(2008).
[15]A.Hao,X.Yang,L.Zhang,and Y.Zhu,J.Phys.Chem. Solid 74,1504(2013).
[16]J.Yang,F.Gao,H.Wang,H.Gou,X.Hao,and Z.Li,Mater.Chem.Phys.119,499(2010).
[17]J.Yang,Ph.D.Dissertation,Qinhuangdao:Yanshan University,(2010).
[18]S.J.Clark,M.D.Segall,C.J.Pickard,P.J.Hasnip,M. I.J.Probert,K.Refson,and M.C.Payne,Z.Kristallogr.220,567(2005).
[19]J.P.Perdew,K.Burke,and M.Ernzerhof,Phys.Rev. Lett.77,3865(1996).
[20]H.J.Monkhorst and J.D.Pack,Phys.Rev.B 13,5188(1976).
[21]P.W.Anderson,Rev.Mod.Phys.50,191(1978).
[22]R.A.de Groot,F.M.Mueller,P.G.van Engen,and K.H.J.Buschow,Phys.Rev.Lett.50,2024(1983).
[23]V.N.Antonov,B.N.Harmon,A.N.Yaresko,and A. P.Shpak,Phys.Rev.B 75,184422(2007).
[24]J.Jensen and A.R.Mackintosh,Rare Earth Magnetism.Oxford:Oxford Science,(1991).
[25]A.Hao and Y.Zhu,J.Appl.Phys.112,023519(2012).
[26]Y.Ma,A.R.Oganov,Z.Li,Y.Xie,and J.Kotakoski,Phys.Rev.Lett.102,065501(2009).
[27]J.Hanies,J.M.L´eger,and G.Bocquillon,Annu.Rev. Mater.Res.31,1(2001).
∗Author to whom correspondence should be addressed.E-mail:haoam@neuq.edu.cn
 CHINESE JOURNAL OF CHEMICAL PHYSICS2016年2期
CHINESE JOURNAL OF CHEMICAL PHYSICS2016年2期
- CHINESE JOURNAL OF CHEMICAL PHYSICS的其它文章
- Incorporation of Reactive Corrosion Inhibitor in Waterborne Acrylic Polyurethane Coatings and Evaluation of its Corrosion Performance
- Response Ability to External Signal Enhanced by Biological Spatial Configuration in Coupled Hindmarsh-Rose Neural System
- Synthesis and Surface Activity of Heterogemini Imidazolium Surfactants
- Substituent Effects on Reduction Potentials of Meta-substituted and Para-substituted Benzylideneanilines
- Preparation of Nitrogen-Doped Carbon Catalyst to Oxygen Reduction Reaction and Influence of Protective Gas Flowing on Its Activity
- Structural and Magnetic Properties of Chemically Synthesized Pd-Modified NiFe2O4Nanoparticles
