关于双曲函数的Cusa-Huygens型不等式的改进
何灯,李云杰
(福清第三中学,福建福清350315)
关于双曲函数的Cusa-Huygens型不等式的改进
何灯,李云杰
(福清第三中学,福建福清350315)
本文将双曲函数的Cusa-Huygens型不等式作了进一步的改进,所建立的双边不等式优于现有的诸多结果,文末导出一条涉及算术平均、几何平均、对数平均的不等式链.
双曲函数;Cusa-Huygens型不等式;Seiffert平均;不等式
0 引言
文献[1-2]建立了著名的Cusa-Huygens不等式,文献[3]给出了双曲函数的Cusa-Huygens不等式,针对文献[3]所建立的不等式的改进与推广,现有诸多结果[4-13].本文研究sh x/x更优的上下界形式,从而可将双曲型Cusa-Huygens不等式作进一步的推广和改进,并由此建立了涉及算术平均、几何平均、对数平均的一条不等式链.
1 预备知识
Cusa-Huygens不等式[1-2]:设,则有.
双曲函数的Cusa-Huygens不等式[3]:设x∈(0,+∞),则有
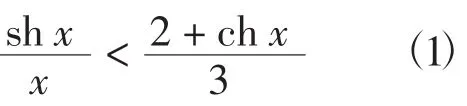
朱灵[7]将式(1)推广为:设x>0,,则有.
E.Neuman与J.Sándor改进式(1)为:设x>0,则.

成立当且仅当q≥3.
朱灵[15]将式(2)推广为:设x>0,p>1或p≤8/15,则当且仅当q≥3(1-p).特别地,令p=1/2,q=3/2,可得
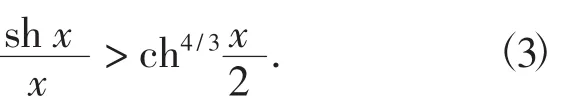
杨镇杭[11]将式(3)推广为:

最近,杨镇杭[16]证得如下两个结论:
结论1设p,x>0,双边不等式
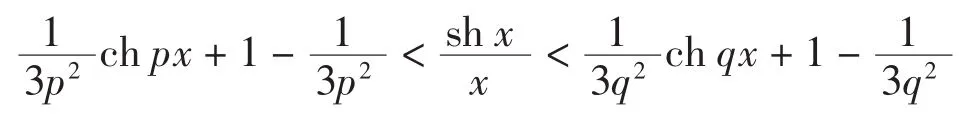
结论2设x>0,则
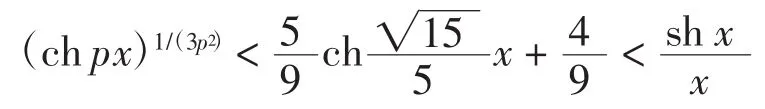
综合上述结论,可得不等式链

2 引理及证明
引理1设n∈N*,n≥7,则22n>(1+p)2n+0.57n(1+p)2n,其中(下同).
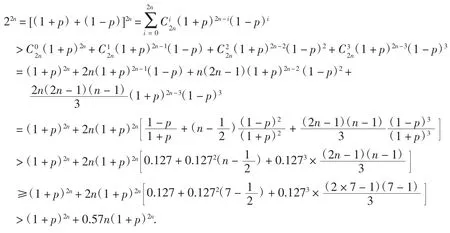
引理2设an=89×22n+121-(2n+1)[25(1+p)2n+25(1-p)2n+34+75p2n],n∈N*,则an≥0.
证明当n=1,2,3,可求an=0.可求.
当n≥7,由引理1得

综上,引理2成立.
3 主要结论及其证明
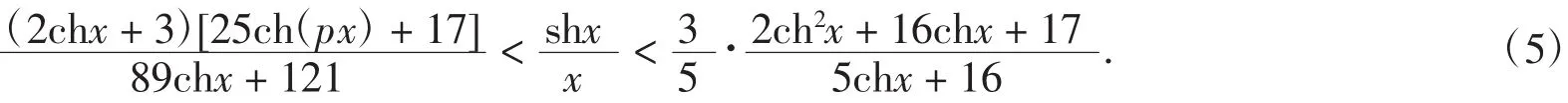
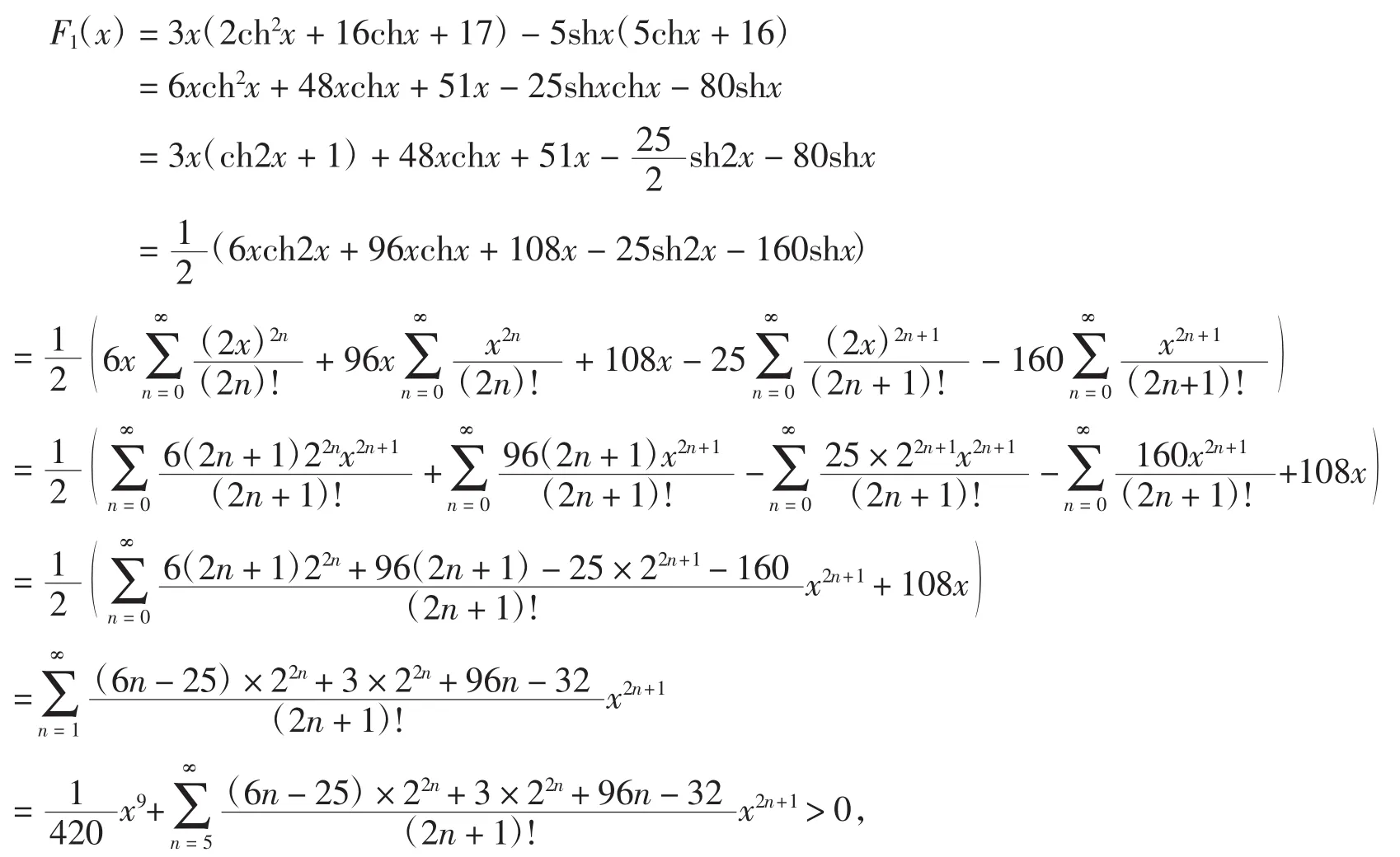
从而式(5)右端不等式成立.又

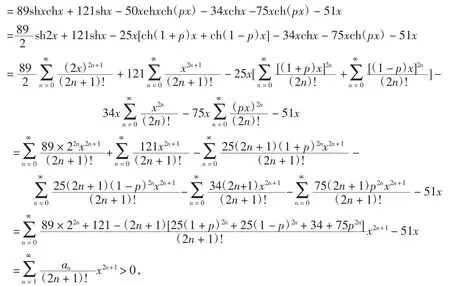
由引理2可证最后一个不等式成立,则有式(5)左端不等式成立.
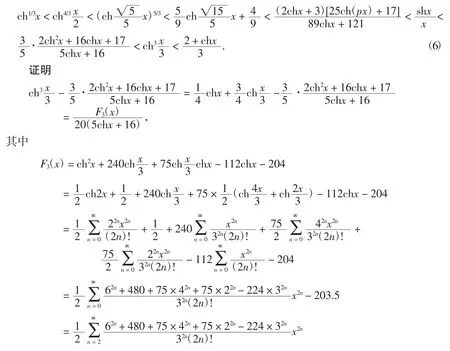

结合式(4),可得式(6)成立.
4 定理2的等价形式
两个正数a,b的幂平均定义为[17]

A2,A1,A0分别称为这两个数的平方根平均,算术平均及几何平均.
四类Seiffert平均分别定义为


从而定理2等价于下定理3.
定理3设a,b>0,a≠b,则如下不等式链成立
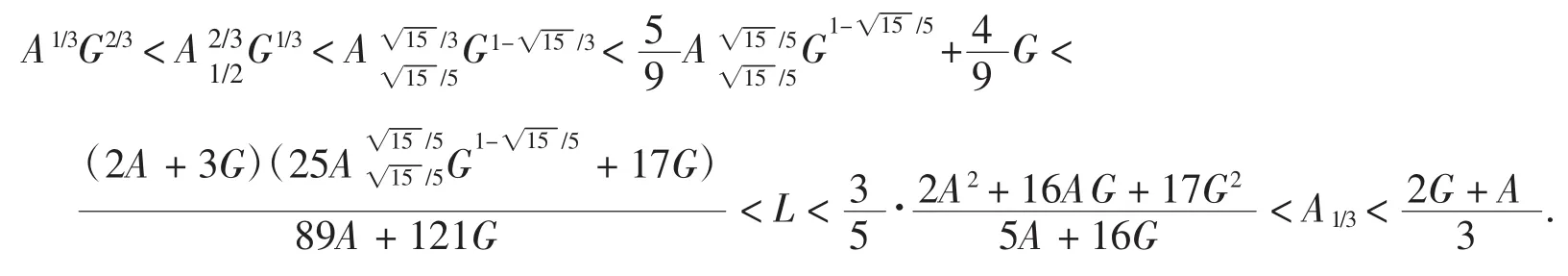
[1]Cajori F.A history of mathematics[M].2nd ed,New York:BibioLife,1929.
[2]Campan F T.The story of number[M].Romania:Ed Albatros,1977.
[3]Neuman E,Sándor J.On some inequalities involving trigonometric and hyperbolic functions with emphasis on the Cusa-Huygens,Wilker and Huygens inequalities[J].Math Inequal Appl,2010,13(4):715-723.
[4]Zhu L.New inequalities for means in two variables[J].Math Inequal Appl,2008,11(2):229-235.
[5]Zhu L.Some new inequalities for means in two variables[J].Math Inequal Appl,2008,11(3):443-448.
[6]Zhu L.Some new inequalities of the Huygens type[J].Comput Math Appl,2009,58(6):1180-1182.
[7]Zhu L.Inequalities for hyperbolic functions and their applications[J/OL].J Inequal Appl,2010. [2013-10-10].http://www.journalofinequalitiesandapplications.com/content/2010/1/130821.
[8]Neuman E,Sándor J.Inequalities for hyperbolic functions[J].Appl Math Comp,2012,218(18):9291-9295.
[9]Wu S H,Debnath L.Wilker-type inequalities for hyperbolic functions[J].Appl Math Lett,2012,25(5):837-842.
[10]Zhu L.New inequalities for hyperbolic functions and their applications[J/OL].J Inequal Appl,2012.[2013-10-20].http://www.journalo?nequalitiesandapplications.com/content/2012/1/303.
[11]Yang Z H.New sharp bounds for logarithmic mean and identric mean[J/OL].J Inequal,Appl,2013. http://www.journalo?nequalitiesandapplications.com/content/2013/1/116.
[12]Chen C P,Sándor J.Inequality chains for Wilker,Huygens and Lazarevitype inequalities[J].J Math Inequal,2014,8(1):55-67.
[13]Yang Z H.Three families of two-parameter means constructed by trigonometric functions[J/OL].J Inequal Appl,2013.[2014-1-10].http://www.journalofinequalitiesandapplications.com/content/2013/1/541.
[15]Zhu L.Generalized Lazarevic’s inequality and its applications-Part II[J/OL].J Inequal Appl,2009. [2014-1-20].http://www.journalofinequalitiesandapplications.com/content/2009/1/379142.
[16]Yang Z H,Chu Y M.Jordan type inequalities for hyperbolic functions and their applications[J/OL]. Journal of Function Spaces.2014.[2014-3-10].http://www.hindawi.com/journals/jfs/aip/370979/.
[17]匡继昌.常用不等式[M].第四版.济南:山东科学技术出版社,2010.
[18]Seiffert H J.Werte zwischen dem geometrischen und dem arithmetischen Mittel zweier Zahlen[J]. Elemente der Mathematik,1987,42(4):105-107.
[19]Seiffert H J.Aufgabe 16[J].Die Wurzel,1995,29:221-222.[20]Neuman E,Sándor J.On the Schwab-Borchardt mean[J].Math Pannon,2003,14(2):253-266.
[21]Neuman E.Bounds for symmetric elliptic integrals[J].J Approx Theory,2003,12(2):249-259.
Im provement of Cusa-Huygens Type Inequality for Hyperbolic Functions
HE Deng,LI Yunjie
(Number 3 Middle School,Fuqing 350315,Fujian,China)
In this paper,Cusa-Huygens type inequalities for Hyperbolic Functions are improved.The double inequality is obtained.An inequality chain about arithmetic mean,geometric mean,logarithmic mean is derived.
Hyperbolic functions;Cusa-Huygens type inequality;Seiffert mean;inequality
O 178
A
1001-4217(2015)02-0028-07
2014-09-08
何灯(1984-),男,福建福清人,学士,全国不等式研究会成员.研究方向:解析不等式及不等式机器证明.E-mail:hedeng123@163.com.

