Self-consistent sources and conservation laws for a super classical-Boussinesq equation hierarchy

WU Jingzhu,XING Xiuzhi

(School of Mathematics and Statistics,Zhoukou Normal University,Zhoukou 466001,China)

Soliton equations with self-consistent sources have received much attention in the recent research literature.Physically,the sources appear in solitary waves with a non-constant velocity and lead to a variety of dynamics of physical models.With regard to their applications,these kinds of systems are usually used to describe interactions between different solitary waves.They are also relevant to some problems related to hydrodynamics,solid state physics and plasma physics,amongst others.In[1,2],Ma.Strampp and Fuchssteiner systematically apply explicit symmetry constraints and binary nonlinearization of Lax pairs for generating the solution equation with sources.Furthermore,Ma presents the soliton solutions of the SchrÒinger equation with self-consistent source in[3].The discrete case of using variational derivatives in generating sources is discussed in[4].Conservation laws play an important role in discussing the integrability for soliton hierarchy.An infinite number of conservation laws for Kd V equation were first discovered by Miura et al.in 1968[5],and then lots of methods have been developed to find them.This may be mainly due to the contributions of Wadati and others[6-8].Conservation laws also play an important role in mathematics and engineering as well.Many papers dealing with symmetries and conservation laws were presented.The direct construction method of multipliers for the conservation laws was presented[9].

Recently,with the development of integrable systems,super integrable systems have attracted much attention.Many scholars and experts do research on the topic,and get lots of results.A supertrace identity on Lie super-algebras and super-Hamiltonian structures of a super-AKNS soliton hierarchy and a super-Dirac soliton hierarchy are obtained in[10].The super classical-Boussinesq hierarchy and its super-Hamiltonian structure are considered in[11].Binary nonlinearization of the super-AKNS system under an implicit symmetry constraint is given[12].A super-Burgers hierarchy and its super-Hamiltonian structure is obtained respectively based on Lie super-algebra[13].

In this paper,with the help of variational identity,super classical-Boussinesq hierarchy and its Hamiltonian structure,then based on the theory of self-consistent sources,the self-consistent sources super classical-Boussinesq hierarchy are established.Finally,the conservation laws for the two types of super classical-Boussinesq hierarchy are also obtained.

1 A super soliton hierarchy with self-consistent sources

Based on a Lie superalgebra sl(3),

that is along with the communicative operation[e1,e2]=2e2,[e1,e3]=-2e3,[e2,e3]=e1,[e1,e4]=[e2,e5]=e4,[e1,e5]=[e4,e3]=-e5,[e4,e5]+=e1,[e4,e4]+=-2e2,and[e5,e5]+=2e3.

We consider an auxiliary linear problem

where u=(u1,…,un)T,Un=R1+=ui(i=1,…,5),φi=φi(x,t)are field variables defining x∈R,t∈R,ei=ei(λ)∈l(3),where the loop algebral(3)is defined by span{λnA|n≥0,A∈sl(3)}and R1is a pseudore-gular element.

The compatibility of(2)gives rise to the well-known zero curvature equation as follows

If an equation

can be worked out through(3),we call(4)a super evolution equation.If there is a super Hamiltonian operator J and a function Hnsuch that

where

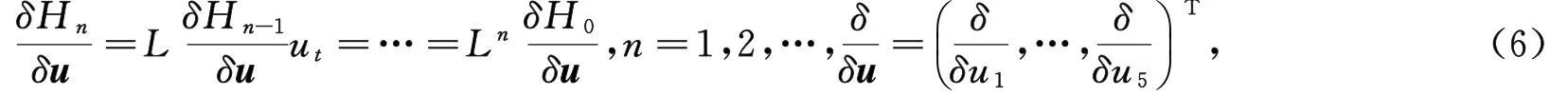
then(4)possesses a super Hamiltonian equation.If so,we can say that(4)has a super Hamiltonian structure.According to(2),now we consider a new auxiliary linear problem.For N distinctλj,j=1,2,…,N,the systems of(2)become as follows

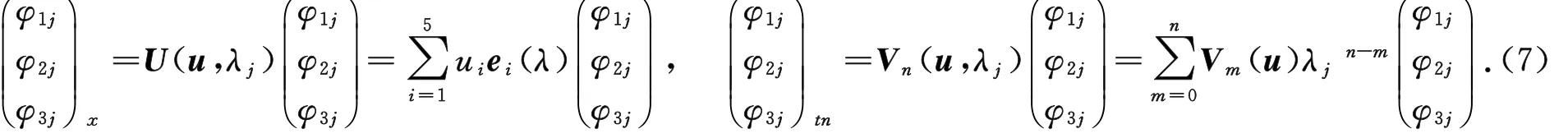
Based on the result in[14],we can show that the following equation:

holds true,whereαjare constants.Eq(8)determines a finite dimensional invariant set for the flows in(6).From(7),we know that,

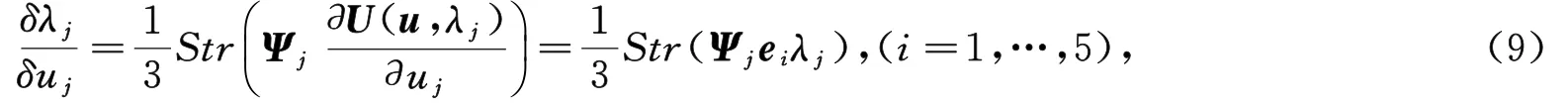
where Str denotes the trace of a matrix and

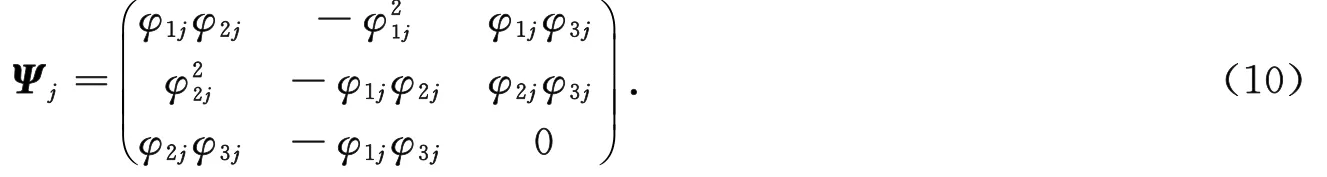
From(8)and(9),a kind of super Hamiltonian soliton equation hierarchy with self-consistent sources is presented as follows

2 The super classical-boussinesq hierarchy with self-consistent sources

The super classical-Boussinesq spectral problem associated with the Lie super algebra is given in[11]

where

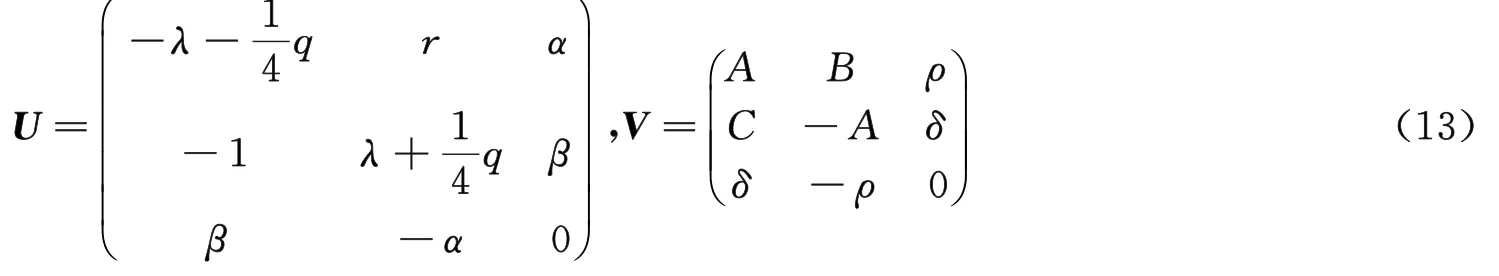
and

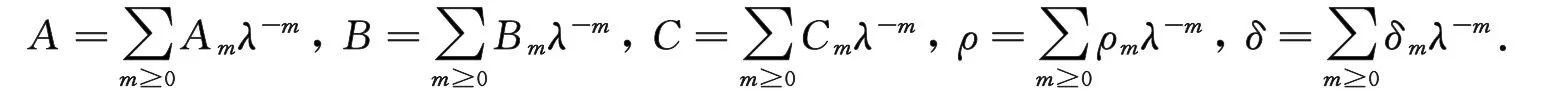
Starting from the stationary zero curvature equation

We have

Then we consider the auxiliary spectral problem

where

Considering

Substituting(18)into the zero curvature equation

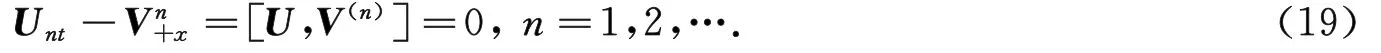
We get the super classical-Boussinesq hierarchy

where Pn+1=LPn,

According to super trace identity on Lie super algebras,a direct calculation reads as

When we take n=2 the hierarchy(20)can be reduced to super nonlinear integrable couplings equations

Next,we will construct the super classical-Boussinesq hierarchy with self-consistent sources.Consider the linear system

From(8),for the system(12),we set

whereΨj=(φj1,φj2,…,φjN)T,j=1,2,3.

According to(11),the integrable super classical-Boussinesq hierarchy with self-consistent sources is proposed as follows:

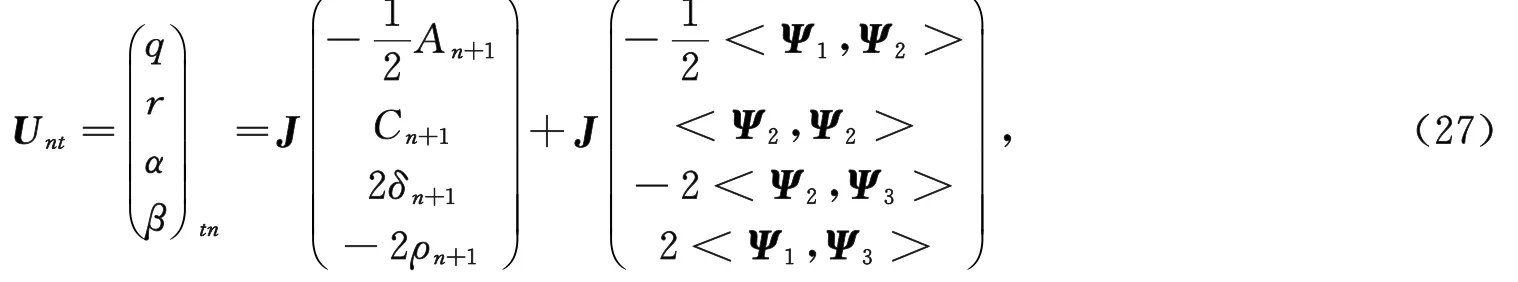
whereΨj=(φj1,φj2,…,φjN)T,j=1,2,3.satisfy

For n=2,we obtain the super classical-Boussinesq equation with self-consistent sources as follows

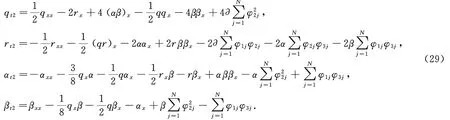
whereφij(i=1,2,3;j=1,2,…,N)satisfy(28).

3 Conservation laws for the super classical-boussinesq hierarchy

In the following,we will construct conservation laws of the super classical-Boussinesq hierarchy.We introduce the variables

From(7)and(12),we have

We expand E,K in the power ofλas follows

Substituting(32)into(31)and comparing the coefficients of the same power ofλwe obtain

and a recursion formula forωn,kn,

Because of

we derive the conservation laws of(20)

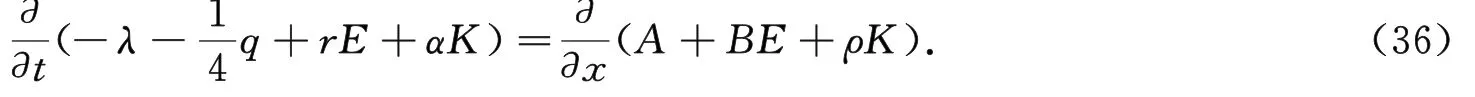
where

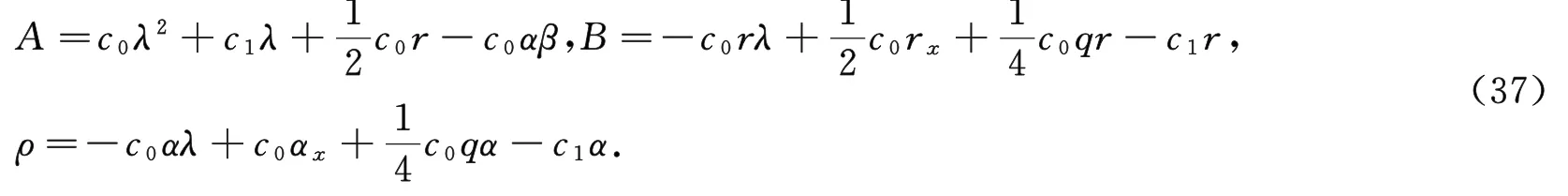
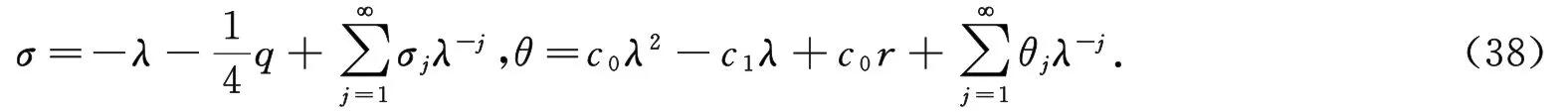
Where c0,c1are constants of integration.The first two conserved densities and currents are read as follows

The recursion relation forσnandθnare

whereωnand kncan be calculated from(34).The infinitely many conservation laws of(29)can be easily obtained from(31)-(40),respectively.

[1]Ma W X,Strampp W.An explicit symmetry constraint for the Lax pairs and the adjoint Lax pairs of AKNS systems[J].Phy.Lett.A,1994,185(3):277-286.

[2]Ma W X,Fuchssteiner B.Binary nonlinearization of Lax pairs,in Proceedings of the First Workshop on Nonlinear Physics in Gallipoli[M].Italy:World Scientific,1996:217224.

[3]Ma W X.Enlarging spectral problems to construct integrable couplings of soliton equations[J].Physics Letters A,2003,316:72-76.

[4]Ma W X,Geng X G.Backlund transformations of soliton systems from symmetry constraints[J].CRM Proc.Lect.Notes,2001,29:313-323.

[5]Miura R M,Gardner C S,Kruskal M D.Korteweg-de Vries equation and generalizations.II.existence of conservation laws and constants of motion[J].J.Math.Phy.,1968,9(8):1204-1209.

[6]Ma W X,Fuchssteiner B.Integrable theory of the perturbation equation[J].Chaos,Solitons and Fractals,1996,7(8):1227-1250.

[7]Ma W X.Integrable cuoplings of soliton equations by perturbations:I.A general theory and application to the Kd V hierarchy[J].Math.Appl.Anal.,2000,7(1):21-56.

[8]Wang X Z,Liu X K.Two types of Lie super-algebra for the super-integrable Tu-hierarchy and its super-Hamiltonian structure[J].Commun.Nonlinear Sci.Numer.Simulat,2010,15(8):2044-2049.

[9]Bluman G W,Anco S C.Symmetry and integration methods for differential equations[M].New York:Springer-Verlag,2002.

[10]Ma W X,He J S,Qin Z Y.A supertrace identity and its applications to superintegrable systems[J].J.Math.Phy,2008,49(3):1-13.

[11]Tao S X,Xia T C.Lie algebra and Lie super algebra for integrable couplings of C-Kd V hierarchy[J].Chin.Phy.Lett.,2010,27(4):1-4.

[12]He J S,Yu J,Zhou R G.Binary nonlinearization of the super AKNS system[J].Modern Phy.Lett.B,2008,22(4):275-288.

[13]Li Z.Super-Burgers soliton hierarchy and its super-hamiltonian structure[J].Modern Phy.Lett.B,2009,23(24):2907-2914.

[14]Tu G Z.An extension of a theorem on gradients of conserved densities of integrable systems[J].Northeastern Math,1990,6(1):26-32.