对数平均的最优凸组合界
孟祥菊,潘学功,高梦涵
(1.保定学院数学与计算机系,河北保定 071000;2.河北软件职业技术学院学生处,河北保定 071000)
对数平均的最优凸组合界
孟祥菊1,潘学功2,高梦涵1
(1.保定学院数学与计算机系,河北保定 071000;2.河北软件职业技术学院学生处,河北保定 071000)
考虑对数平均、调和平均、第2类反调和平均之间的估计式,建立了对数平均关于调和平均、第2类反调和平均的最优凸组合界.这些结果都是经典平均构建的最佳双边不等式的推广和发展.
对数平均;调和平均;第2类反调和平均;不等式
MSC2010:26D20
近几年来,二变量平均值理论已经成为数学研究的热门课题,它在物理学、经济学、气象学等方面都有广泛的应用.国内外学者们建立了一系列精确的不等式[19].


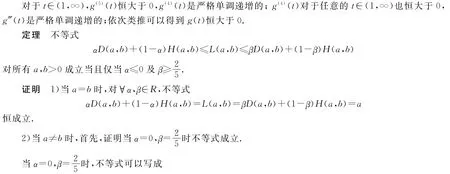
[1] TOMINAGA M.Specht's ratio and logarithmic mean in the Young inequality[J].Math Inequal Appl,2004,7(1):113-125.
[2] KAHLIG P,MATKOWSKI J.Functional equations involving the logarithmic mean[J].Z Angew Math,1996,76(7):385 390.
[3] PITTENGER A O.The logarithmic mean in variables[J].Amer Math Monthly,1985,92(2):99 -104.
[4] STOLARSKY K B.Generalizations of the logarithmic means[J].Math Mag,1975,48:87 -92.
[5] KOUBA O.New bounds for the identric mean of two arguments[J].JIPAM.J Inequal Pure Appl Math,2008,9(3):6.
[6] BURK F.The geometric logarithmic and arithmetic mean inequality[J].Amer Math,1987,94(6):27 -528.
[7] QI F,CUO B N.An inequality between ratio of the extended logarithmic and ratio of the exponential means[J].Taiwanese J Math,2003,7(2):229-237.
[8] CARLSON B C.The logarithmic mean[J].Amer Math,1972,79:615 -618.
[9] CHU Yuming,ZONG Cheng,WANG Fendi.Optimal convex combination bounds of Seiffert and geometric means for the arithmetic mean[J].Journal Math Inequal,2011,5(3):429 -434.
(责任编辑:王兰英)
Optimal convex combination bounds for logarithmic mean
MENG Xiangju1,PAN Xuegong2,GAO Menghan1
(1.Department of Mathematics and Computer Science,Baoding College,Baoding 071000,China;
2.Division of Students Affairs,Hebei Software Institute,Baoding 071000,China)
The esimates among the logarithmic mean,the second contraharmonic mean and the harmonic mean were considered.The optimal convex combination bounds of the logarithmic mean in terms of the second contraharmonic mean and the harmonic mean were established.These results are extensions and developments of classical optimal bilateral inequalities.
logarithmic mean;harmonic mean;the second contraharmonic mean;inequality
O178
A
1000 -1565(2014)05 -0471 04
10.3969/j.issn.1000 -1565.2014.05.005
2013 11 -06
河北省科技厅软科学基金资助项目(11457242);保定学院自然科学基金资助项目(2012Z06);保定市科协课题资助项目(KX2013A21)
孟祥菊(1971 ),女,河北卢龙人,保定学院副教授,主要从事均值不等式方向研究.E-mail:mengxiangju328@163.com
book=34,ebook=31

