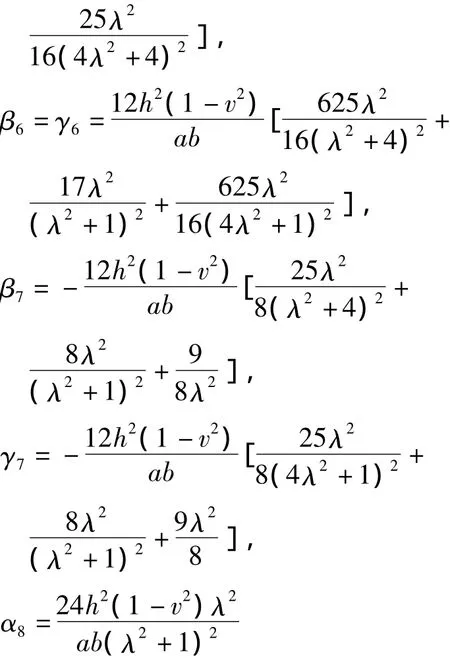参数激励和外激励联合作用下薄板的非线性动力学*
郝五零 张伟
(1.云南师范大学数学学院,昆明 650500)(2.北京工业大学机械工程与应用电子技术学院,北京 100124)
参数激励和外激励联合作用下薄板的非线性动力学*
郝五零1†张伟2
(1.云南师范大学数学学院,昆明 650500)(2.北京工业大学机械工程与应用电子技术学院,北京 100124)
研究了在参数激励和外激励联合作用下四边简支矩形薄板的非线性动力学.基于von Karman理论,推导出了在参数激励和外激励联合作用下四边简支矩形薄板的动力学方程.利用Galerkin法对偏微分方程进行三阶离散,得到一个三自由度的常微分方程.考虑1:2:4内共振-主参数共振-1/2亚谐共振的情况,利用多尺度法得到了薄板系统的六维的平均方程.最后,采用数值方法研究了薄板的周期和混沌运动.结果发现外激励对薄板的混沌运动是敏感的.
非线性系统, 矩形薄板, 多尺度法, 平均方程, 混沌
引言
众所周知,薄板的应用是非常广泛的,但是在应用过程中常常会出现由于外力的作用发生变形的现象,因此研究薄板的大变形具有很重要的应用价值.事实上,这些大的变形往往不是简单的线性问题,而是复杂的非线性问题.为了能够更加细致的研究大变形的特性,我们需要研究薄板的更高维数的非线性动力学,这与低维的系统相比会更加复杂.因此研究高维非线性薄板系统的动力学将有更大的意义!
目前,关于薄板的非线性振动、分叉和混沌动力学的研究取得了一些进展.1990年,Hadian和Nayfeh[1]利用多尺度法分析了谐波激励作用下的非线性夹紧圆板混合内共振情形的响应.Yang和Sethna[2]用平均法分析了参数激励下正方形板的局部分叉和全局分叉,研究结果表明系统存在异宿环和Smale马蹄意义的混沌运动.根据 Yang和Sethna 的研究结果,Feng 和 Sethna[3]用全局摄动方法进一步分析了参数激励下1:1内共振薄板的全局分叉和单脉冲混沌动力学,他们得到了Shilnikov同宿轨道和混沌运动存在的条件.2001年,Zhang[4]研究了在参数激励下的简支矩形薄板全局分叉和混沌动力学.首先,基于von Karman理论[5]得到了薄板的运动方程,然后,应用Galerkin方法和多尺度方法得到了薄板的平均方程.接下来应用规范形理论[6]对系统进行化简,最后,应用高维Melnikov方法[7]研究了系统的异宿分岔和混沌动力学.后来Zhang等人[8]又用同样的方法研究了参数激励和横向激励联合作用下的简支矩形薄板全局分叉和混沌动力学.Awrejcewicz等[9]研究了在一侧受到纵向的随时间变化的载荷的柔性薄板的周期和概周期及混沌运动.Awrejcewicz和 Krysko[10]利用 Bubnov- Galerkin 法研究了柔性薄板和壳在有限自由度离散系统下的动力学.Han等人[11]利用Galerkin方法和平均法研究了大变形弹性矩形板的非线性动力学.2007年,Yao和Zhang[12]利用规范形方法和能量-相位法研究了参数激励和外激励联合作用下的矩形薄板的多脉冲Shilnikov类型动力学.在这篇文章中所研究的系统是个自治的系统.在此文章的基础上,随后Zhang等人[13-15]应用改进的广义 Melnikov 方法研究了四维非自治屈曲薄板的全局分叉和多脉冲混沌动力学.2010年,Li等人[16]运用指数二分法和广义平均法[17]研究了面内激励和横向激励联合作用下屈曲矩形薄板的混沌动力学.Yu和Chen[18]研究了受横向间谐激励的简支矩形金属板的全局分叉和单脉冲混沌动力学.
以上这些文献都是对二自由度的薄板系统进行了分析,本文将运用Galerkin方法对薄板系统进行三阶离散,得到一个三自由度的非线性控制方程;接下来运用多尺度法对参数激励和外激励联合作用下四边简支矩形薄板进行摄动分析;然后对薄板系统进行数值模拟;最后给出了结论.
1 薄板的三阶离散
下面我们对要研究的薄板模型进行简要的概述.四边简支矩形薄板的边长为a和b,厚度是h,薄板同时受参数激励和外激励,所建立的直角坐标系如图1.坐标系Oxy位于薄板的中面上.u、v和w分别表示薄板中面上的一点在x、y和z方向的位移.薄板的参数激励为p=p0-p1cosΩ2t,外激励为F(x,y)cosΩ1t.
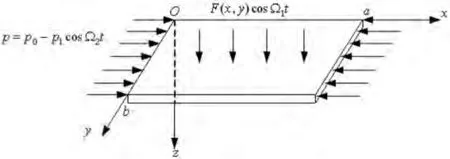
图1 矩形薄板的模型及坐标系Fig.1 The model of a rectangular thin plate and the coordinate system
根据薄板的von Karman方程,可以得到矩形薄板的运动方程如下
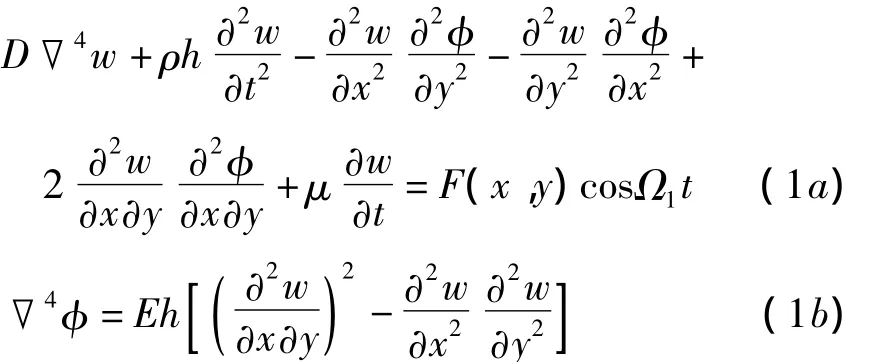
式中,ρ是薄板的密度;D=Eh3/(12(1-v2))是薄板的弯曲刚度;E是薄板的杨氏模量;v是Poisson比;φ为薄板的应力函数;μ为薄板的阻尼系数.
薄板的简支边界条件为

在满足边界条件的情况下,可以得到应力函数φ应该满足如下条件
当x=0和a时
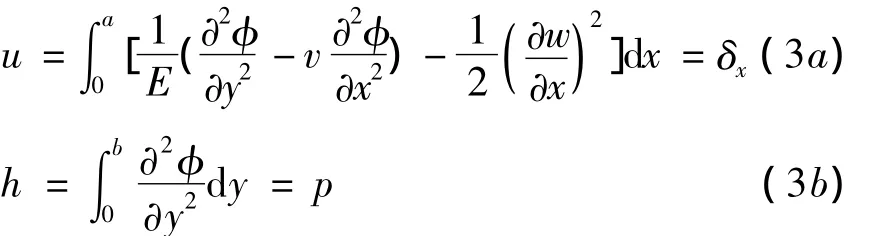
当y=0和b时
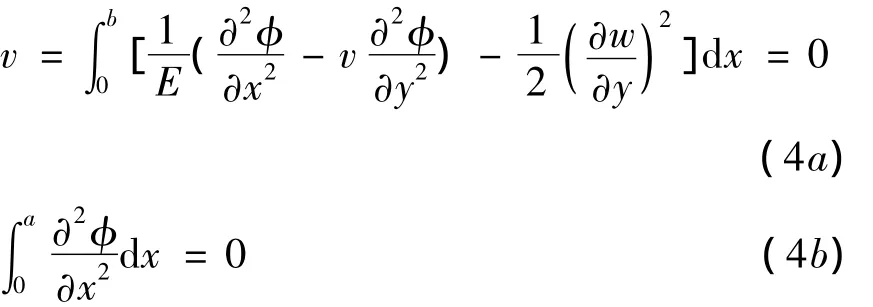
式中δx为边界上x方向的位移.
我们考虑薄板的前三阶模态的非线性振动,则w可以表示为
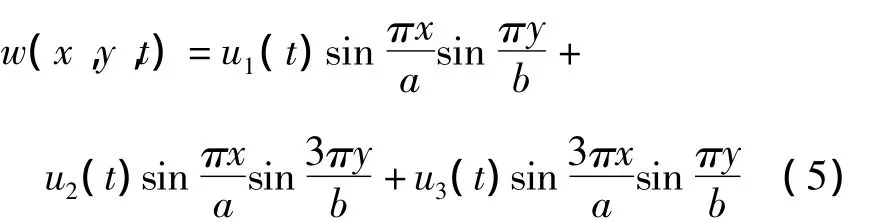
式中ui(t)(i=1,2,3)为三个模态的振幅.
横向的激励可以表示成如下的形式

式中Fi(i=1,2,3)为三个非线性模态的横向激励的振幅.
将方程(3)~(6)代入方程(1b),同时考虑边界条件(3)和(4),并且积分,得到如下的应力函数
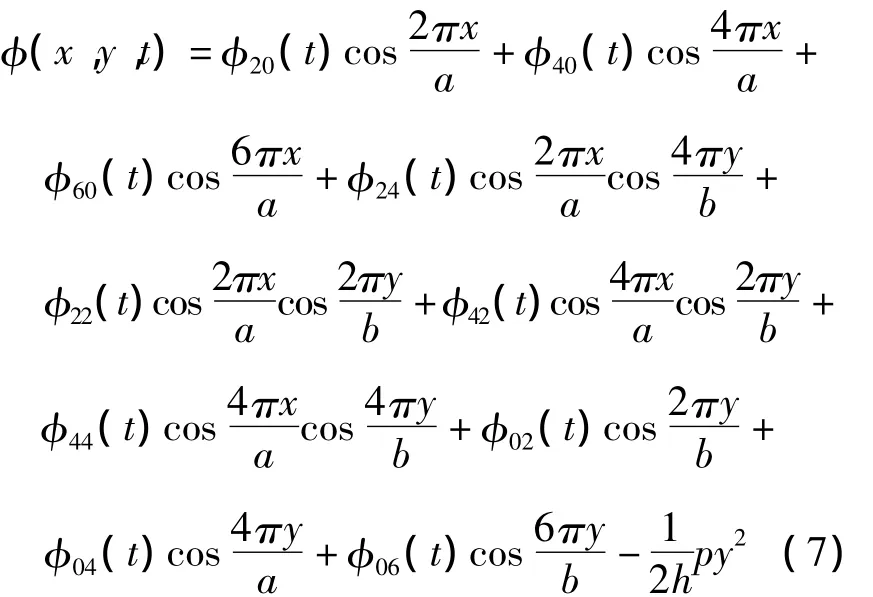


为了得到无量纲方程,我们引入变量和参数变换如下

为了便于分析,去掉参数和变量上面的符号“-”,将方程(5)~(8)代入方程(1),应用 Galerkin方法并积分,可以得到无量纲运动方程如下

式中的系数可以参看附录;其中 ωk(k=1,2,3)为薄板的三个线性固有频率;fk(k=1,2,3)为参数激励的振幅;Fk(k=1,2,3)为外激励的振幅.
2 摄动分析
在本节中,我们运用多尺度法对四边简支矩形薄板系统进行摄动分析.为了便于分析,我们对系统(9)引入如下的尺度变换
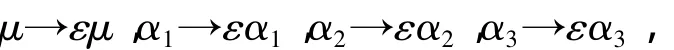
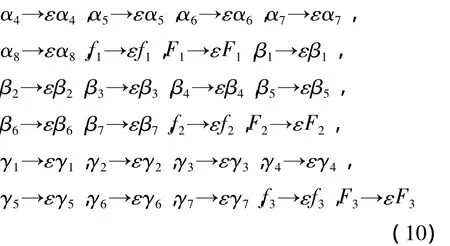
把变换(10)代入方程(9),可以得到含有小参数的运动方程
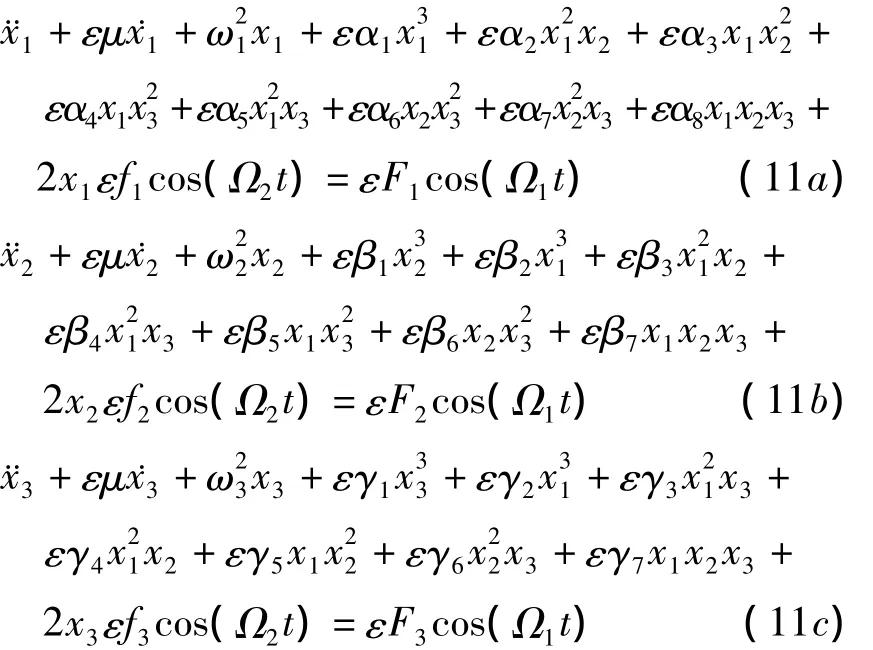
为了得到方程(11)的平均方程,我们使用多尺度法对系统进行摄动分析.设方程有如下形式的解

其中T0=t,T1= εt.
可以得到式子(12)有如下形式的微分算子

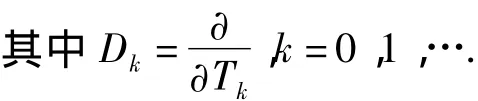
我们研究薄板系统的1:2:4内共振-主参数共振-1/2亚谐共振情况下的运动,则共振关系如下:
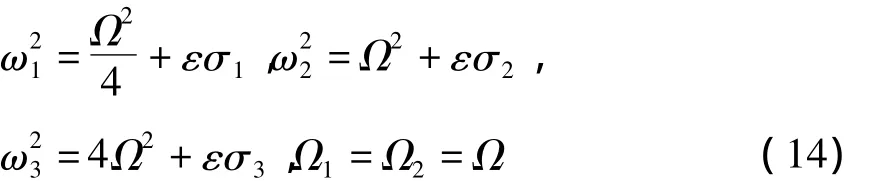
其中 ω1、ω2和 ω3为薄板系统前三阶的频率;σ1、σ2和σ3为三个调谐参数.
不妨令Ω=2,把公式(12)、(13)和(14)代入到公式(11),并且比较方程两边小摄动参数ε同阶次的系数,可以得到如下的微分方程

方程(15)的解的复数形式可以表示如下:

将方程(17)代入方程(16),可以得到如下的方程
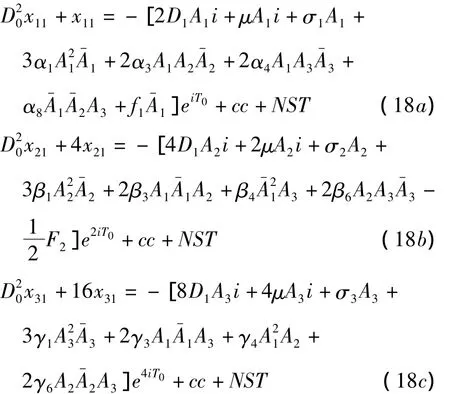
其中cc和NST分别表示方程(18)右边函数的共轭项和非长期项.令方程的长期项等于零,可以得到如下复数形式的平均方程

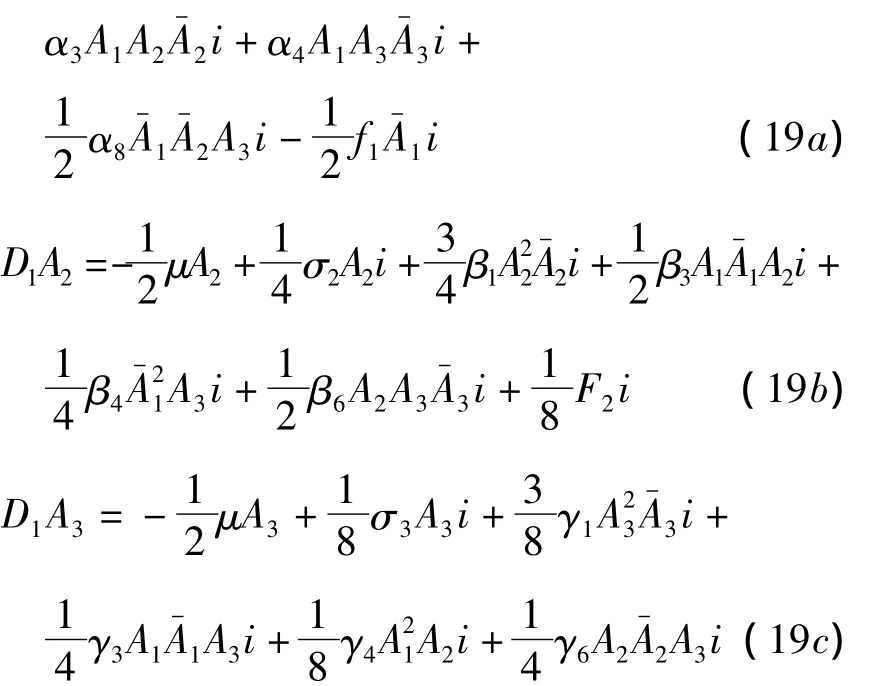
为了得到直角坐标形式的平均方程,可以将A1、A2和A3表示成如下的形式
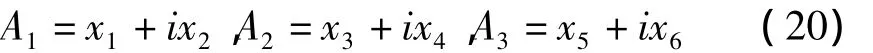
将(20)代入(19),可以得到如下形式的平均方程
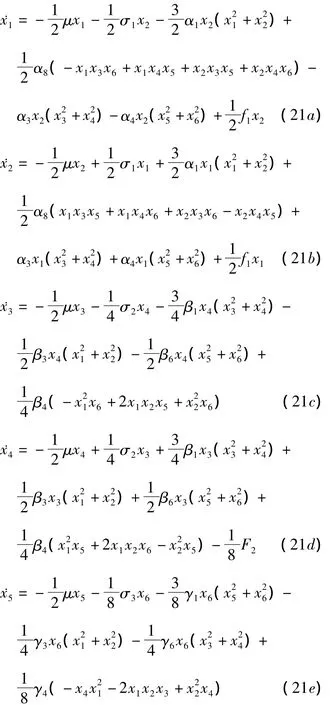
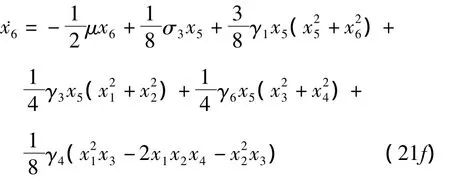
3 非线性动力学分析
本节利用数值方法对平均方程(21)进行数值模拟分析,得到薄板系统的非线性动力学响应.图2 -5的(a)、(c)和(e)分别表示二维平面(x1,x2)、(x3,x4)和(x5,x6)的相图;(b)、(d)和(f)分别表示(t,x1)、(t,x3)和(t,x5)的波形图;(g)和(h)分别表示(x1,x2,x3)和(x4,x5,x6)的三维相图.
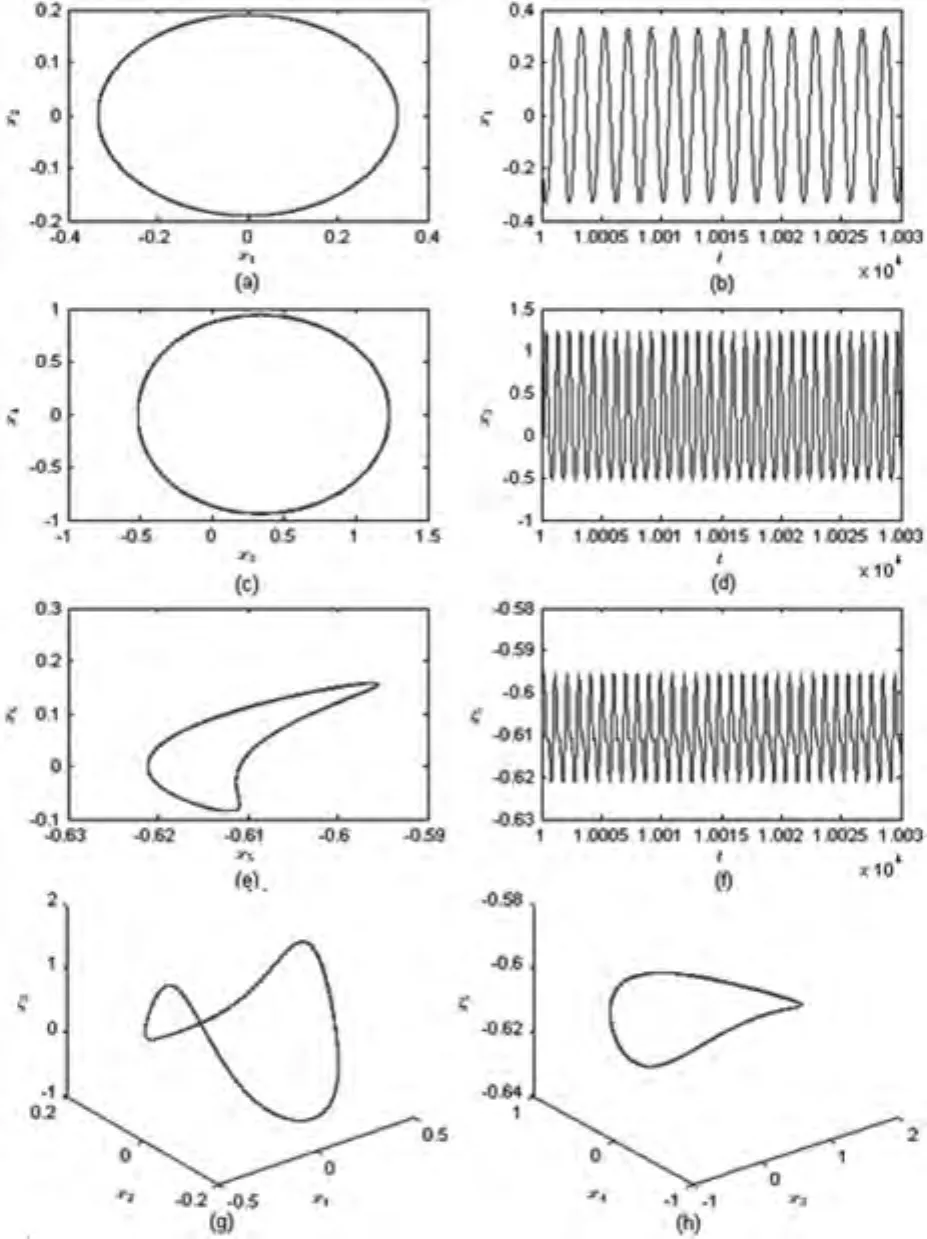
图2 薄板的单倍周期运动Fig.2 The single-periodic motion of the thin plate
方程(21)的初始值为:

参数取下面的值:
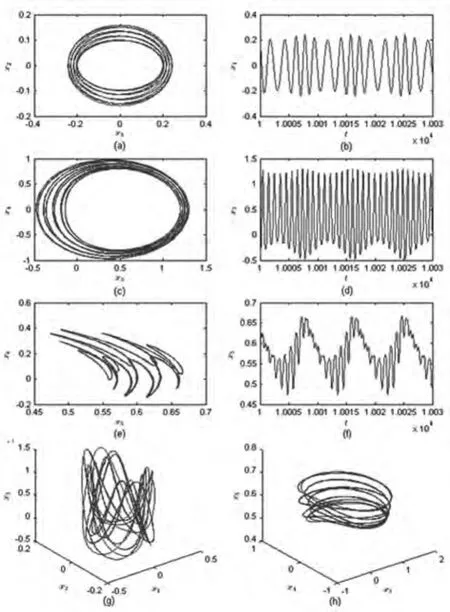
图3 薄板的多倍周期运动Fig.3 The multi- periodic motion of the thin plate
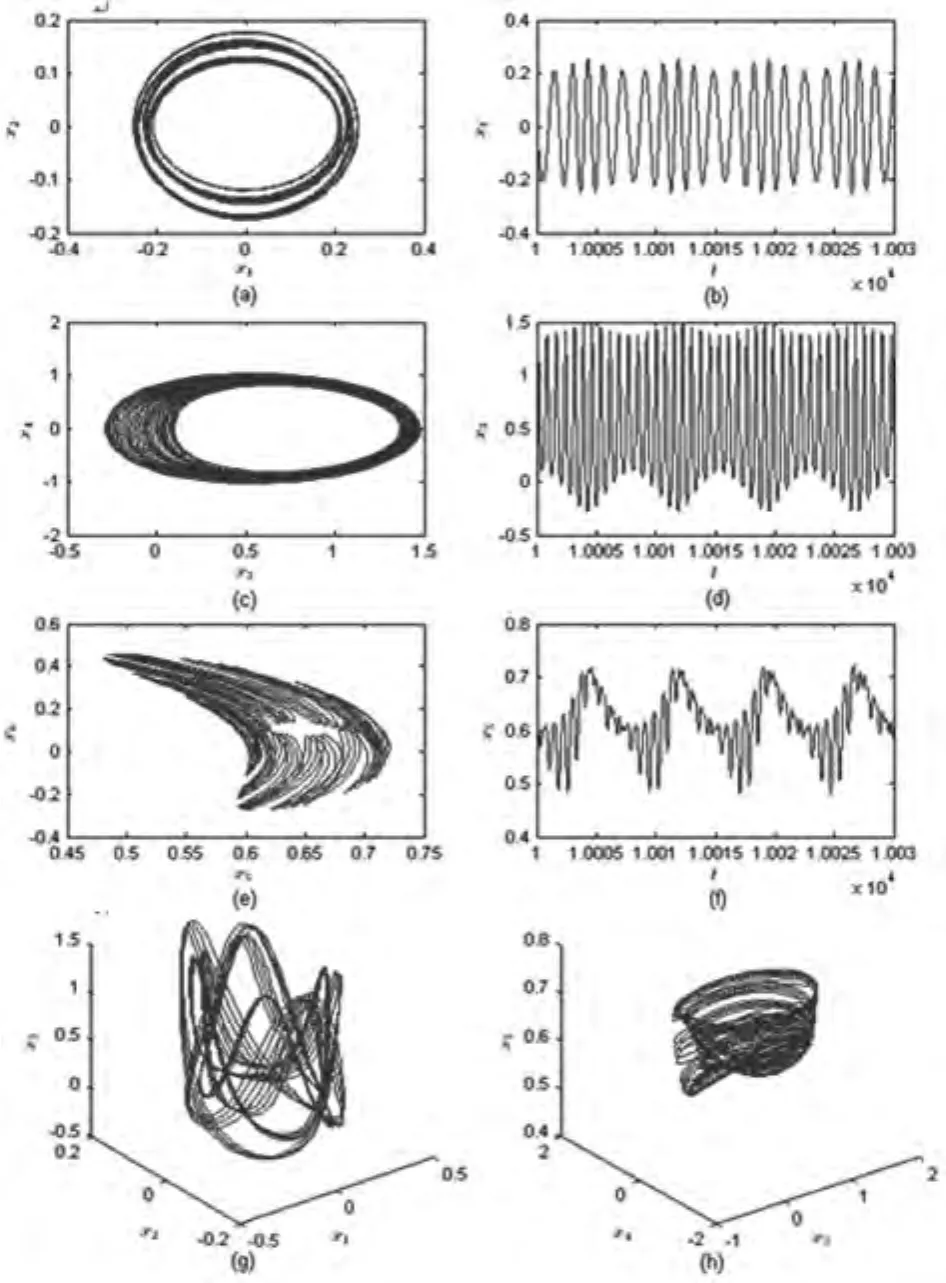
图4 薄板的概周期运动Fig.4 The quasi- periodic motion of the thin plate
薄板系统出现了单倍周期响应.如图2所示.当F2=22时,薄板系统出现了多倍周期响应.如图3所示.继续变化F2的取值,可以发现,当F2=37时,薄板系统出现了概周期响应,如图4所示.当F2=44时,薄板系统出现了混沌运动,如图5所示.由此可得,当逐渐变化F2的取值时,薄板系统发生从单倍周期-多倍周期-概周期-混沌的变化过程.

图5 薄板的混沌运动Fig.5 The chaotic motion of the thin plate
4 结论
本文研究了在参数激励和外激励联合作用下四边简支矩形薄板的非线性动力学.在研究过程中,运用Galerkin法对四边简支矩形薄板系统进行了三阶离散,得到了一个三自由度的非自治常微分方程.然后利用多尺度法得到了平均方程.最后通过数值计算,得到四边简支矩形薄板系统随着参数的变化发生从周期运动-概周期-混沌的变化规律.通过本文的研究,一方面对以后继续研究高维的薄板系统的非线性动力学奠定了基础;另一方面对研究其他的高维板结构的非线性动力学行为具有重要的参考意义.
1 Hadian J,Nayfe A H.Modal interaction in circular plates.Journal of Sound and Vibration,1990,142(2):279~292
2 Yang L,Sethna P R.Local and global bifurcations in parametrically excited vibrations nearly square plates.International Journal of Non-linear Mechanics,1990,26(2):199~220
3 Feng Z C,Sethna P R.Global bifurcations in the motion of parametrically excited thin plate.Nonlinear Dynamics,1993,4:389~408
4 Zhang W.Global and chaotic dynamics for a parametrically excited thin plate.Journal of Sound and Vibration,2001,239(5):1013~1036
5 Chia C Y.Nonlinear analysis of plate.New York:1980
6 Yu P,Zhang W,Bi Q.Vibration analysis on a thin plate with the aid of computation of normal forms.International Journal of Non-linear Mechanics,2001,36(4):597 ~627
7 Yagasaki K.Periodic and homoclinic motions in forced,coupled oscillators.Nonlinear Dynamics,1999,20(4):319~359
8 Zhang W,Liu Z M,Yu P.Global dynamics of a parametrically and externally excited thin plate.Nonlinear Dynamics,2001,24:245~268
9 Awrejcewicz J,Krysko V A,Krysko A V.Spatio-temporal chaos and solitons exhibited by von kármán model.International Journal of Bifurcation and Chaos,2002,12(7):1465~1513
10 Awrejcewicz J,Krysko A V.Analysis of complex parametric vibrations of plates and shells using Bubnov-Galerkin approach.Archive of Applied Mechanics,2003,73:467 ~532
11 Han Q,Dai L,Dong M.Bifurcation and chaotic motion of an elastic plate of large deflection under parametric excitation.International Journal of Bifurcation and Chaos,2005,15(9):2849~2863
12 Yao M H,Zhang W.Multi-pulse Shilnikov orbits and chaotic dynamics of a parametrically and externally excited thin plate.International Journal of Bifurcations and Chaos,2007,17(3):1~25
13 Zhang J H,Zhang W.Global bifurcation and chaotic dynamics for a non-autonomous buckled thin plate.Journal of Dalian University of Technology,2006,46(S1):1~6
14 Zhang J H,Zhang W,Yao M H,et al.Multi-pulse Shilnikov chaotic dynamics for a non-autonomous buckled thin plate under parametric excitation.International Journal of Nonlinear Sciences and Numerical Simulation,2008,9(4):381~394
15 Zhang W,Zhang J H,Yao M H.The extended Melnikov method for non-autonomous nonlinear dynamical system of a buckled thin plate.Nonlinear Analysis:Real World Application,2010,11(3):1442~1457
16 Zhang W,Li S B.Resonant chaotic motions of a buckled rectangular thin plate with parametrically and externally excitations.Nonlinear Dynamics,2010,62(3):673 ~686
17 Feckan M,Gruendler J R.The existence of chaos for ordinary differential equations with a center manifold.Bulletin of the Belgian Mathematical Society,2004,11(1):77~94
18 Yu W Q,Chen F Q.Global bifurcations of a simply supported rectangular metallic plate subjected to a transverse harmonic excitation.Nonlinear Dynamics,2010,59:129~141

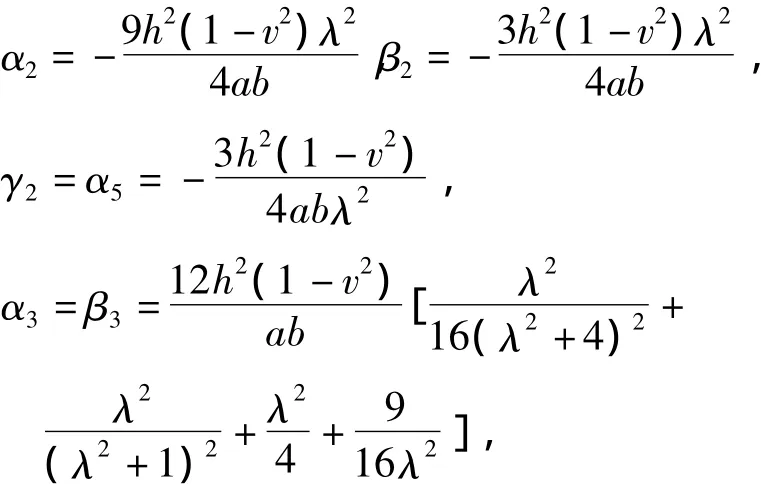
*The project supported by the State Key Program of National Natural Science Foundation of China(10732020),the National Natural Science Foundation of China(11072008 and 10972011)and the National Natural Science Foundation of China Youth Project(11002005)
† Corresponding author E-mail:haowuling@sohu.com
NONLINEAR DYNAMICS OF A PARAMETRICALLY AND EXTERNALLY EXCITED THIN PLATE*
Hao Wuling1†Zhang Wei2
(1.School of Mathematics,Yunnam Normal University,Kunming650500,China)(2.College of Mechanical Engineering,Beijing University of Technology,Beijing100124,China)
The nonlinear dynamics of a four-edge simply supported rectangular thin plate under the combination of the parametrical and external excitations were investigated.Based on the von Karman theory,the formulas of motion for the four-edge simply supported rectangular thin plate under the combination of the parametrical and external excitations were derived.The partial differential equations were discretized to the ordinary differential equations with three-degree-of-freedom using the Galerkin approach.Considering the resonant cases of 1:2:4 internal resonance and principal parametric resonance-1/2 subharmonic resonance,the method of multiple scales was utilized to obtain the six-dimensional averaged equations.Furthermore,numerical method was carried out to investigate the periodic and chaotic motions of the thin plate.The results show that the chaotic responses of the thin plate are sensitive to the external excitation.
nonlinear system, rectangular thin plate, the method of multiple scales, averaged equation,chaos
2 July 2012,
20 July 2012.
10.6052/1672-6553-2014-009
2012-07-02 收到第 1 稿,2012-07-20 收到修改稿.
*国家自然科学基金重点资助项目(10732020),国家自然科学基金资助项目(11072008和10972011)和国家自然科学青年基金资助项目(11002005)
E-mail:haowuling@sohu.com
附录