Hom-quadri-代数和Hom-octo-代数
安慧辉,薛 晨,康 健
(辽宁师范大学 数学学院,辽宁 大连 116023)
0 引言
Dendriform 代数、quadri-代数、octo-代数都称为Loday 代数,这些代数都有共同“分裂结合性”的特性,即将结合代数的乘法表示成一串二元运算的和[1]。同时,Loday 代数在许多领域中也有广泛的应用,甚至某些代数还成为了一个独立的代数体系发展起来,例如1995 年Loday 在研究代数K-理论时首先发现的dendriform 代数[2],后来随着人们对dendriform 代数的深入研究,发现它在许多数学、物理领域中都有广泛的应用,例如operads 理论[3],同调[4],Hopf 代数[5,6],李代数和Leibniz 代数[4],以及量子场[7]等等。本文就在octo-代数、quadri-代数基础上分别给出Hom-quadri-代数和Hom-octo-代数的定义和结构,并讨论了它们之间的关系。
1 基本内容
定义1.1(Hom-associative-代数)[8]
定义1.2(Hom-pre-Lie-代数)[8]
定义1.3(Hom-dendriform-代数)[8]
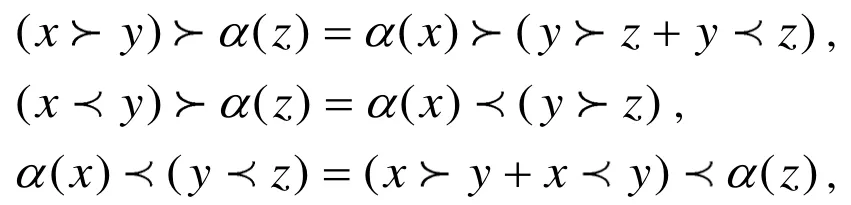
命题1.4[8]
命题1.5[8]
定义1.6(quadri-代数)[1]
假设A 为线性空间,在A 上定义代数运算


定义1.7(octo-代数) [1]
假设A 为一个线性空间,在A 上定义代数运算


其中:

2 Hom-quadri-代数
定义2.1(Hom-quadri-代数)

注:
定理2.2
证明:
对于 ∀x , y ,z ∈ A ,有
命题2.3
证明:
对于 ∀x , y ,z ∈ A ,由Hom-dendriform-代数的定义可得:

推论2.4
证明:
利用定义1.2、命题1.5 和命题2.3 即可证明。
推论2.5
证明:
利用定义1.1、命题1.4 和命题2.3 即可证明。
3 Hom-octo-代数
定义3.1(Hom-octo-代数)


其中:



注:
定理3.2
证明:
对于 ∀x , y ,z ∈ A ,有


命题3.3
证明:


推论3.4
证明:
由定义1.3 和命题3.3 即可证明。
推论3.5
证明:
由定义1.2、命题1.5 和推论3.4 即可证明。
推论3.6
证明:
由定义1.1、命题1.4 和推论3.4 即可证明。
[1] Bai CM. O-operators of Loday algebrasand analogues of the classical Yang-Baxter equation[J]. Comm. in Alg., 2010, 38(11): 4277-4321.
[2] Loday J L, Dialgebras, in Dialgebras and related operads [J]. Lec. Not. in Math., 2001, 1763: 7-66.
[3] Loday J L, Scindement d’associativité et algèbres de Hopf [J]. Pro. of the Con. in hou. of hou. of Jean Leray, 2004, 9: 155- 172.
[4] Frabetti A, Leibniz homology of dialgebras of matrices [J]. Jour. of Pure and App.Alg., 1998, 129(2): 123-141.
[5] Ronco M, Eulerian idempotents and Milnor-Moore theorem for certain non-cocommutative Hopf algebras [J]. Jou. Alg., 2002, 254(1): 151-172.
[6] Chapoton F, Un théorème de Cartier-Milnor-Moore-Quillen pour les bigèbres dendriforms et les algebras braces [J]. Pure and App. Alg., 2002, 168(1): 1-18.
[7] Koszul J L, Variétés localement plates et convexitè [J]. Ocaka Jour. of Math., 1965, 2(2): 285-290.
[8] Makhiouf Abdenacer. Hom-dendriform algebras and Rota- Baxter Hom-algebras [J/OL]. (2011-1-2) [2014-06-10]. http:// arxiv.org/pdf/1101.0435.pdf.

