Coexistence of antiferromagnetism and unconventional superconductivity in a quasi-one-dimensional flat-band system:Creutz lattice
Feng Xu(徐峰) and Lei Zhang(张磊)
School of Physics and Telecommunication Engineering,Shaanxi University of Technology,Hanzhong 723001,China
Keywords: flat-band unconventional superconductivity,antiferromagnetism,strong electron-electron interaction,superfluid weight
1.Introduction
Antiferromagnetism (AFM) and unconventional superconductivity (SC) are two key phenomena that appeared in strongly correlated electronic materials such as cuprates and iron pnictide high temperature superconductors, and heavy fermion systems.[1-3]In particular, an unusual quantum state
where both AF and superconducting orders coexist microscopically has been widely studied in many theoretical works.[4-10]Some experimental works have revealed that several quantum materials display unique AFM-SC coexistence states in their phase diagrams.[12-15]It is noteworthy that a staggered spintriplet pairing superconducting order parameter is induced in the coexistence of AFM and singlet SC.[4,10]The spin-triplet superconductors are ideal materials that can keep SC under a strong magnetic field and are also important candidate materials for quantum computation.[11]Hence, it is of great interest to study the interplay between unconventional SC and AFM, in particular for systems with strong electron-electron correlations.[7]
The highly-degenerated Bloch band with constant energy,named flat band, with the quench of the kinetic energy is an ideal platform to study the behavior of strongly correlated electrons.As shown in the recent works,adding an attractive Hubbard interaction in a flat band can carry a nonzero superfluid weight.[16-19]This conclusion is universal for flat bands in the strongly correlated limit,in which the superfluid weight is proportional to effective attractive interaction.[20-22]Flatband magnetism is another interesting topic, such as the famous ferromagnetism in the Lieb lattice,the spin-imbalanced effect on the superfluidity is also studied in this system.[17,44]The interplay of magnetism and SC in the flat-band system is a meaningful topic that deserves further study.
A quasi-one dimensional flat band system such as Creutz lattice has been of great interest because it is much easier to solve than two-dimensional models in the theory and is easy to simulate in an ultracold atom experiment.[18,19,23-25]It is essentially one-dimensional system with two-dimensional characteristics because of the interchain degrees of freedom that appear.Another quasi-one-dimensional system, named the two-leg square ladder, has been attracting attention for both theoretical and experimental reasons because it is directly related to the stripe-ordered cuprate superconductor La2-xBaxCuO4.[26,27]
Flat band systems have attracted attention since the experimental discovery of a superconducting state in twisted bilayer graphene related to dispersionless bands.[29-33]The original interest in the flat band has arisen from the prediction that it has a dramatically higher critical temperature in a flat band based on the Bardeen-Cooper-Schrieffer theory.[34,35]There are many systems that have flat bands,e.g.,sawtooth,kagome,Lie lattice,and others.These systems have recently been studied extensively,in both fermionic and bosonic language.[36-38]A superfluid state is supported by bosons paired on different sites or Cooper pairs using a fermionic language in these flat band systems.[39]There is a rich phase diagram exhibiting a condensate, a pair condensate, a supersolid and phase separation phases in the study of bosons on the flat band Creutz lattice.[23,24]The Creutz model with an attractive Hubbard interaction using the extended Bardeen-Cooper-Schrieffer theory and the density matrix renormalization group has been studied, which displays finite superfluid weight with lower bound related to the winding number.[18]
在认证档案信息时,可以运用原始性认证技术,通过水印技术,提高电子档案的可靠性认证,比如可以对篡改电子档案、原始档案数据等内容进行甄别。可逆水印技术运用了一种精确预测算法,可以对模板边缘类型进行细分预测,提高了精度,为档案安全提供了保障。
2.Model and methodology
We study a quasi-one-dimensional system named Creutz lattice governed by the following Hamiltonian:
whereσ=±for↑↓.It is difficult to treat the strong coupling constraint of no double occupancy analytically, hence Zhanget al.first introduced Gutzwiller renormalization factors to approximate the constraint and did a lot of research work with great significance.[24,48,49]In this paper, we use the explicit renormalization factors as follows:
where〈-Kx〉 is the kinetic energy density andΛxxis the current-current correlation function.[40-42]The superfluid weight in the flat-bands systems has been widely considered,especially the geometric origins of superfluidity in topologically nontrivial flat bands.[16]According to the Bardeen-Cooper-Schrieffer theory, the superfluid weight should go to zero due to the fermi velocity being zero in the single flat band.However, the geometric contributions of energy bands will lead to nonzero superfluid weight with a well-defined lower bound,even for an isolated and strictly flat band.[17-19]
再次,乌江保护联动抓手不足。近年来,重庆、贵州两省市不断强化乌江环境保护,不断深化经济产业合作,各级地方政府间交流日益密切。在乌江生态保护方面,两省市均在习近平总书记“共抓大保护,不搞大开发”思想指导下展开大量细致的工作,但基于生态保护体制机制的联动合作相对不足,缺少跨区域、常态化、工作化和考核化的生态保护体制机制与平台途径,省市间生态保护工作互动顺畅度不够、联动性不强。因而,应当建立渝黔地区乌江经济带绿色生态廊道联动机制平台,强化跨区域政府工作联动考核机制,找准文化旅游产业联动抓手,为乌江生态廊道建设保护提供制度保障和产业经济保障。
whereJ=4t2/Uis the AF coupling,Siandniare the spin and number operators for electrons atisite, respectively, andPG=∏i(1-ni↑ni↓)is the Gutzwiller projection operator that projects out states with doubly-occupied sites and〈ij〉 labels the nearest-neighbor bond.
where|Ψ0〉 is the unprojected wavefunction andQ=πfor AFM.In addition, the superconducting pairing order parameters and the bond fields parameters and hole density are defined as
In this paper, we use the renormalized mean-field theory to study the interplay of SC and AFM on the Creutz lattice.The no double occupancy constraint can be treated the same as in previous works, the projection operator is replaced by Gutzwiller factors leading to a renormalized Hamiltonian.[27,28]For simplicity, we use the link betweenAi-Ai+1as an example to get the renormalized Hamiltonian.The same procedure has also been done on the links betweenAi-Bi+1,Bi-Bi+1,Ai-Bi+1.To study the interplay of SC and AFM, the local values of the magnetic order parameters are defined as follows:
高校行政权力和学术权力在运行机制方面的问题其主要表现:决策机制方面,各学术权力机构在实际运行中的教授治学氛围不浓,民主程度有待提高,行政权力在决策中发挥主导作用;运行机制方面,行政权力主导学术权力,虽基本上都成立了校学术委员会,但教学委员会等发展不充分,其运行机制难以发挥作用,学术权力运行受限;监督机制方面:普通老师参与不足,各高校对学术权力机构的设置随意性大。事实上高校学术权力和行政权力之间确实存在着冲突与矛盾,但是通过建立有效的机制和保障措施,最终学术权力和行政权力可以协调发展。
(4)色谱技术。如果转基因作物中的组分产生变化,比如蛋白质含量以及油分含量等,采用色谱检测技术,能够实现精准分析以及定量检测。
Here, we study unconventional SC on the Creutz model with strong repulsive electron-electron interaction using the renormalized mean-field method with Gutzwiller factors.This paper is organized as follows.In Section 2,we describe the basic theoretical model and renormalized mean-field theory.In Section 3, we present our numerical results and discuss their physical meanings.Finally, some conclusions are drawn in Section 4.


whereAandBlabel the two chains; the intrachain hopping energy on chainA(B) becomest(-t); interchain hopping energy between siteion chainA(B) and sitei+1 on chainB(A) is given by-t(t); andσ=↑,↓is the fermion spin.For noninteracting cases, the system shows two strictly flat bandsE=±2t.Due to the flat band structure, electrons will be localized in the near lattice without correlation effects.To include correlation effects between electrons, the appropriate Hubbard model with repulsive potential is used,ˆℋ= ˆℋ0+U∑i,αˆni,α,↑ˆni,α,↓, whereα=A,Bis the chain index.In the strong interacting limit whenU ≫t, an effectivet-Jmodel in which the ground state is energetically confined to the singly occupied space is given by
We first show physical orders with the increase of the hole-doping concentrationδin Fig.1 under the zero temperature limit withJ=0.5t.The notable point is that the flat band can extend the region of AFM and SC in the holes-doping case.The spin-singlet superconducting order parameter exists unless the system is nearly empty.The spin-triplet superconducting order and the AFM order also exist untilδ ≈0.19 is much higher than the result from another Gutzwiller approximation scheme on a normal system,in which AFM and SC are coexisting up to the dopingδ ≈0.1.[43]It also clearly shows a staggered spin-triplet component of superconducting order parameter accompanied with AF order,which has been discussed and analyzed in the previous works.[4,10]The staggered spintriplet superconducting order parameter has a spatial oscillation in the real space, and can then be seen as Cooper pairs with center-of-mass momentumQin the momentum space.This unconventional superconducting state now is named as pair density wave state, in an analogy to the Fulde-Ferrell-Larkin-Ovchinnikov state.[46]The staggered spin-triplet pairing is induced in the coexistence state of AFM and singlet SC and its free energy based on Ginzburg-Landau analysis has been shown in previous works, and some similar discussions have been done from the point of view of the symmetry of systems.[8,10,47]The staggered spin-triplet superconducting order parameter shows a small dome shape.It increases with hole-doping near half-filled with smallδand reaches a maximum value.It then decreases until it disappears.In other words,the spin-singlet SC is a dominating phase in the ground state of the flat band system, while spin-triplet SC and AFM are accompanied in the lightly-doped region.
3.Results and discussion
4.人民生活水平全面提高。报告提出:“基本公共服务均等化总体实现。全民受教育程度和创新人才培养水平明显提高,……就业更加充分。收入分配差距缩小,……社会保障全民覆盖”[1]。
We show that the physical order parameters vary with the AF coupling interactionJwith fixed hole-dopingδ=0.125 under the zero-temperature limit in Fig.2.This result indicates that the emergence of staggered spin-triple superconducting order and AF order need strong effective AF interaction,which is also the effective attractive potential between Cooper electrons pairs.There is a quantum critical point nearJ ≈0.28tif the AF coupling interactionJis larger than the critical value,then both the staggered spin-triple superconducting order and AF order emerge.At the same time,the spin-singlet superconducting order always exists fromJ=0.15tto 0.65t.No matter how weak the effective attractive potential is,the spin-singlet Copper pairs can be formed.In the flat band system,the electrons’kinetic energy is quenched and the electrons go to spinsinglet pairs with tiny any effective attraction, but staggered spin-triple pairs and AFM need a certain effective interaction in this hole doping level.
二是大力发展民营经济和小微经济。将民营经济占比、市场主体数量、民营经济竞争力等指标纳入季度年度统计运行指标体系,开展横向同类城市民营经济发展比较研究,推动各级干部从重视 “大项目”向重视 “中小微”转变。对中小微企业和民营经济开展定向降成本举措,借鉴广东新政 “实体经济新十条”做法,在工业用地、企业用电、运输和融资、综合税负等方面定向精准降成本。力争“大项目顶天立地,中小微企业铺天盖地”,为提升创新试错成功率储备大数法则数量基础。

Fig.1.The physical orders vary with the hole-doping concentration δ.The spin-singlet superconducting orders Δs increase with the holedoping concentration until δ ≃0.75 and then descend.The spin-triple superconducting orders coexist with magnetic order parameters in the lightly doped region, and are shown as a dome.The parameters are chosen as J=0.5t,T =10-5t.
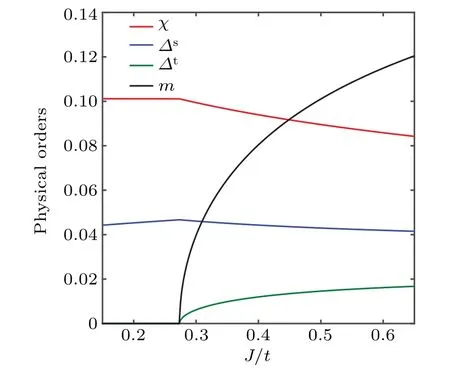
Fig.2.The physical orders vary with the AF coupling interaction J.The spin-triple superconducting order and AF order increase with J and emerge reach the critical value, the spin-singlet superconducting order always exists in spite of weak effective attractive potential between the electrons.The parameters are chosen as δ =0.125, J =0.15t-0.65t,T =10-5t.
The thermodynamics of physical orders are shown in Fig.3.It is interesting and confirmed that the staggered spintriplet superconducting order is induced by both spin-singlet and AF order,Δt∝Δsm0,Δtvanishes regardless ofΔsorm0disappears.An unconventional superconducting state with both spin-singlet and spin-triplet Cooper pairs coexists with AFM.The AF order has a much higher critical temperature than the superconducting order, even though it has a small initial value near the critical hole-dopingδ=0.187.Without AF and staggered spin-triplet superconducting order, the spin-singlet superconducting order vanishes at a relatively low temperature.The superfluid weight is unchanged near zero temperature because when the temperature increases, the energy gap declines to a critical point.Near the critical temperature, the superfluid weight shows linear behavior.The disparity between the critical temperature of superfluid weight and superconducting order shows that the electrons go to preformed pairs but do not cause supercurrent.
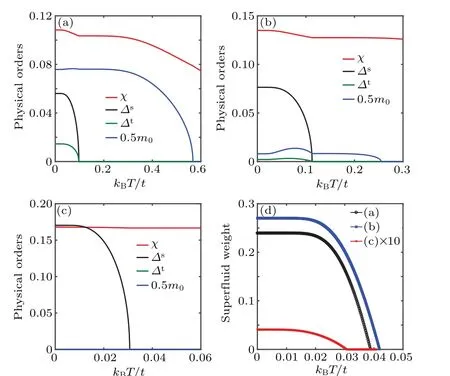
Fig.3.The superconducting orders, AF order, and the bond order evolution as a function of temperature with fixed hole-doping level with J=0.5t,(a) δ =0.125, (b) δ =0.186, (c) δ =0.5.The corresponding superfluid weight is shown in subfigure (d).It is clear that the spin-triple superconducting order exists on the premise of both spin-singlet superconducting order and the AF order.The superfluid weight stays changeless near zero temperature and linearly declines near the critical temperature.
We show the critical temperature of AF orderTN, superconducting orderTΔ, and superfluid weightTswith the increase of hole doping withJ=0.5tin Fig.4.The critical temperature of AF orderTNis much higher than superconducting orderTΔand superfluid weightTs, the Neel temperature is much higher than the superconducting critical temperature,which has been established in both theoretical and experimental works.A thermodynamics state with both AFM and unconventional SC is present at the low-temperature region,which is an unconventional superconducting state with both spin-singlet and spin-triplet pairs.The optimal hole-doping level near theδ=0.187 is the transition point at which the AF order and the spin-triplet superconducting order vanish.The difference between the critical temperature of superconducting orderTΔand the superfluid weightTsis different from the gap between pseudogap phase and superconducting phase,the critical temperature of superconducting orderTΔincreases with hole doping level in the lightly doped region.The critical temperature of the superconducting state with only spinsinglet pairs decreases with the hole doping level, as well as the critical temperature of superfluid weight.It is notable that afterδ=0.6, the spin-singlet superconducting order still exists but cannot support superfluid weight andTsgoes to zero in this doped region.

Fig.4.The critical temperature of AF order TN, superconducting order TΔ and superfluid weight Ts versus hole-doping δ with parameter J=0.5t.
The original research motivation of flat-band SC is to get a higher temperature superconductor.We compare the superconducting orders and their superfluid weight as a function of temperature in the flat band system to the normal dispersed system in Fig.5.We addt′=0.2tbetween inter-chain hoping energy to get a normal dispersed system in the hole-doped case.It is clearly shown that the flat band enhances the critical temperature of the superconducting state,and simultaneously the superfluid weight in the flat band system is bigger than the corresponding ones in the normal dispersed system with higher critical temperature.

Fig.5.The superconducting orders as a function of temperature with δ =0.125,J=0.5t in the flat band system(full line)compared to the corresponding ones in the normal dispersed system (dotted line).The righthand subgraph is the superfluid weight with the change of temperature.
4.Conclusions
We study the coexistence of AFM and unconventional SC on the Creutz lattice under the strong electron-electron correlation limit using the renormalized mean-field theory.An extraordinary quantum thermodynamic state with both AFM and unconventional SC is present, which also includes both the spin-singlet and spin-triplet pairs.The staggered spin-triplet pairs are induced by both the spin-singlet pairs and AFM,but AFM and the spin-triplet pairs need strong effective AF interaction to support them.If the AF coupling interactionJgoes beyond a quantum critical point,then AFM and the spin-triplet pairs both emerge with the spin-singlet SC.The flat band extends the hole-doped region of AFM and SC,the spin-singlet SC is found in most regions of the hole-doping case.The critical temperature of the AF order, superconducting order,and superfluid weight versus hole doping level show that although electrons go to preformed pairs,a real superconducting state leads to the Meissner effect and dissipationless transport emerges at the lower temperature.It is natural to consider that are there other non-uniform charge-ordered states in this flat band system, such as a charge density wave or pair density wave with another momentumQ.We will study the coexistence of charge density wave,spin density wave and pair density wave,and novel SC in the doped flat-band system in future work.
Acknowledgements
Project supported by the Natural Science Basic Research Program of Shaanxi (Program Nos.2023KJXX-064 and 2021JQ-748), the National Natural Science Foundation of China (Grant Nos.11804213 and 12174238), and Scientific Research Foundation of Shaanxi University of Technology(Grant No.SLGRCQD2006).
- Chinese Physics B的其它文章
- A multilayer network diffusion-based model for reviewer recommendation
- Speed limit effect during lane change in a two-lane lattice model under V2X environment
- Dynamics of information diffusion and disease transmission in time-varying multiplex networks with asymmetric activity levels
- Modeling the performance of perovskite solar cells with inserting porous insulating alumina nanoplates
- Logical stochastic resonance in a cross-bifurcation non-smooth system
- Experimental investigation of omnidirectional multiphysics bilayer invisibility cloak with anisotropic geometry