具有多孔介质细胞扩散和矩阵敏感性的二维 趋化Navier-Stokes系统的全局有界性
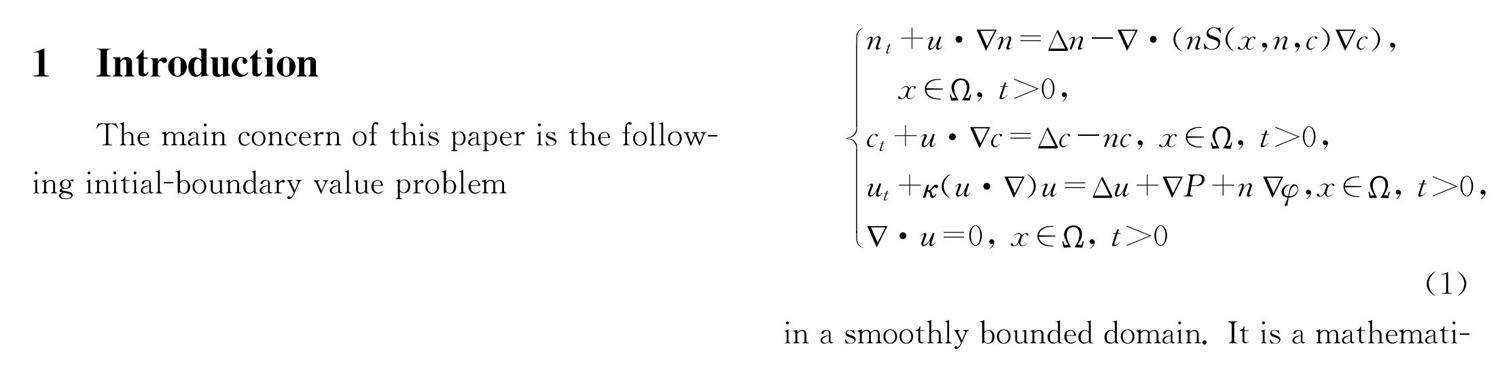


摘要:本文研究了具有多孔介质细胞扩散和矩阵敏感性的趋化-流体耦合模型的初边值问题弱解的全局有界性. 在二维有界区域上, 本文首先构造了问题对应的正则化系统,建立系统经典解的全局存在性,然后借助能量估计建立了解的有界性,最后对正则化系统取极限得到了原问题弱解的整体存在性.所得结果推广了 Tao 和 Winkler 的相应结果.
关键词:趋化Navier-Stokes系统; 多孔介質; 矩阵敏感度; 全局有界
中图分类号: O175.29 文献标识码:A DOI:10.19907/j.0490-6756.2023.051003
收稿日期: 2022-10-09
基金项目: 四川省应用基础研究计划项目(2020YJ0264)
作者简介: 何肖肖(1998-), 女, 四川南充人, 硕士研究生, 主要研究领域为偏微分方程. E-mail:xiao_x_he@163.com
Global boundedness of a 2D chemotaxis Navier-Stokes system with porous medium cell diffusion and matrix sensitivity
HE Xiao-Xiao
(School of Mathematics, UESTC, Chengdu 611731, China)
In this paper, the global boundedness of weak solutions for the initial-boundary value problem of a chemotaxis-fluid coupling model with porous medium cell diffusion and matrix sensitivity is considered. Firstly, a regularized system is constructed for the problem, and the global classical solvability of the regularized system is established. Then the boundedness of the solutions is obtained with the help of some energy estimates. Finally, the global existence of the weak solutions of the original problem is obtained by taking limit in the regularized system. The obtained results generalize the corresponding results of Tao and Winkler.
Chemotaxis-Navier-Stokes system; Porous medium cell diffusion; Matrix sensitivity; Global boundedness

