Intrinsic kinetics of catalytic hydrogenation of 2-nitro-4-acetylamino anisole to 2-amino-4-acetylamino anisole over Raney nickel catalyst

Xiangyang Cui, Xin Zhang, Baoju Wang,2,, Yuqi Sun, Haikui Zou, Guangwen Chu, Yong Luo,,Jianfeng Chen

1 State Key Laboratory of Organic-Inorganic Composites, Research Center of the Ministry of Education for High Gravity Engineering and Technology, Beijing University of Chemical Technology, Beijing 100029, China

2 School of Chemistry and Chemical Engineering, Shandong University of Technology, Zibo 255000, China

Keywords:Intrinsic kinetics 2-nitro-4-acetylamino anisole Catalytic hydrogenation Raney nickel catalyst

ABSTRACT The catalytic hydrogenation of 2-nitro-4-acetylamino anisole (NMA) is a less-polluting and efficient method to produce 2-amino-4-acetamino anisole (AMA).However, the kinetics of catalytic hydrogenation of NMA to AMA remains obscure.In this work, the kinetic models including power-law model and Langmuir-Hinshelwood-Hougen-Watson (LHHW) model of NMA hydrogenation to AMA catalyzed by Raney nickel catalyst were investigated.All experiments were carried out under the elimination of mass transfer resistance within the temperature range of 70-100 °C and the hydrogen pressure of 0.8-1.5 MPa.The reaction was found to follow 0.52-order kinetics with respect to the NMA concentration and 1.10-order kinetics in terms of hydrogen pressure.Based on the LHHW model, the dual-site dissociation adsorption of hydrogen was analyzed to be the rate determining step.The research of intrinsic kinetics of NMA to AMA provides the guidance for the reactor design and inspires the catalyst modification.

1.Introduction

The 2-amino-4-acetamino-anisole (AMA), a kind of aromatic amines, is an important intermediate of azo disperse dyes [1-3].The traditional method for AMA production was the chemical reduction of 2-nitro-4-acetylamino anisole (NMA) with iron powder under acidic conditions.But this process produced a large amount of waste water due to the use of iron powder, resulting in serious environmental pollution[4].The catalytic hydrogenation of NMA to AMA (Fig.1) was generally researched due to its less waste water and high purity of product[5-8].The intrinsic kinetics is of great significance for guiding reactor design and process optimization.However, the intrinsic kinetics for the catalytic hydrogenation of NMA to AMA remains obscure.

The researches of intrinsic kinetics of hydrogenation of aromatic nitro compounds were widely studied based on the noble[9,10] and non-noble [11-14] metal catalysts.The Raney nickel was the most effective non-noble catalyst for the production of aromatic amines [15-18].Bawane et al.[19] and Patil et al.[20]found that the hydrogen pressure generally followed first-order kinetics in the catalytic hydrogenation of aromatic nitro compounds catalyzed by the Raney nickel catalyst.The reaction orders in terms of the aromatic nitro compounds with diverse substituent groups were different in catalytic hydrogenation.For example,the p-nitrophenol and o-nitro-cardanol followed first-order kinetics,and the dinitrotoluene followed zero-order kinetics in the corresponding hydrogenation reactions [19-21].The rate-controlling step was also different in the catalytic hydrogenation of aromatic nitro compounds.For example,the o-nitro-cardanol hydrogenation followed a surface reaction controlling mechanism, and the dissociative adsorption of hydrogen was the rate-controlling step in the p-nitrophenol hydrogenation[19,20].In a word,changing the substituent group of aromatic nitro compounds would lead to different results of the kinetic characteristics (such as the reaction orders of reactants, the adsorption mechanism of reactants, and rate-controlling step).Therefore, the research of intrinsic kinetics of NMA to AMA was of great significance due to the different substituent group with other aromatic nitro compounds.

For NMA hydrogenation system, the intrinsic kinetics was rarely reported.Yang et al.[22] investigated the intrinsic kinetics of NMA hydrogenation using self-prepared Cu-Ni bimetallic catalyst.The mechanism model was not established in their work.In this work, the intrinsic kinetics of NMA to AMA catalyzed by the Raney nickel catalyst was studied in detail.Before the kinetic experiments, the influence of mass transfer resistance was eliminated to ensure the experimental data being the region of intrinsic controlling.The effects of temperature and hydrogen pressure on the NMA hydrogenation rate were investigated to obtain the basic data for establishing models.Then, the power-law model and the mechanism model of intrinsic kinetic rate equation were developed by fitting the experimental data.Finally,the consistency test was conducted to verify the established model.

2.Materials and Methods

2.1.Materials

The NMA were provided by Zhejiang Dibang Chemical Co., Ltd.The AMA (＞98.00%(mass)) was purchased from Beijing MREDA Technology Co., Ltd.The methanol (99.50%(mass)) was purchased from Shanghai Macklin Biochemical Technology Co., Ltd.Both hydrogen and nitrogen with high purity (99.99%(vol)) were purchased from the Beijing Air Liquide Gas Co., Ltd.More material details are shown in Table 1.

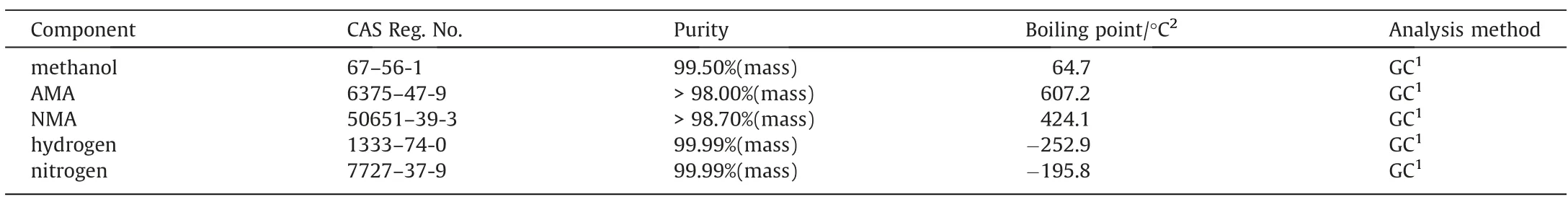
Table 1 Chemical compounds information

2.2.Experimental setup

The catalytic hydrogenation of NMA was carried out in a magnetic stirring reactor (STR) with a volume of 150 ml.The experimental apparatus was depicted in Fig.2.The pressure inside the STR was kept constant by controlling the intake valve.The temperature and pressure can be monitored in real-time.The pressure safety valve was used to prevent overpressure of system.

Fig.2.Experimental apparatus for NMA catalytic hydrogenation.(1—N2 cylinder;2—H2 cylinder; 3—gas pressure reducing valve; 4—gas inlet valve; 5—pressure gauge;6—thermocouple;7—pressure safety valve;8—gas outlet valve;9—sampling connection; 10—reactor).

2.3.Experimental procedure

The NMA, methanol, and Raney nickel catalyst were mixed in the beaker and was dissolved by ultrasound for 5 min.The uniformly dispersed solution was transferred into the STR, and the system was quickly assembled and sealed.In order to remove the air in the system, the reactor was purged with nitrogen for three times.Next, the reactor was heated by the electric heating jacket.When the temperature stabilized to the required temperature,the hydrogen was introduced into the reactor until it reached the required pressure.The reaction time was started at the time of turn on the stirring.During the reaction, 1.5 ml of solution would be taken out from the system each 10 min.An operation of filtration by filter membrane was processed to remove the insoluble impurities.The sample was analyzed by gas chromatography(Shimadzu 2014C) with a flame ionization detector.

2.4.Analysis and catalyst characterization

The concentrations of reactants and products were measured by gas chromatography.The parameters of gas chromatograph were set as follows: 280 °C of the chamber temperature; 200 °C of the column temperature; 300 °C of the detector temperature.The Hp-5 was selected as the chromatographic column.The residence time of sample during test was 12 min.

Before the catalyst characterization, the passivation of Raney nickel catalyst was conducted at 150 °C in the tube furnace with the flow of 99%(vol) nitrogen and 1% (vol) oxygen for 10 h due to the facile self-combustion property of Raney nickel.The surface morphology of the catalyst was investigated by a scanning electron microscopy (SEM, Gemini SEM300, ZEISS) to measure the particle size distribution and average particle size.The N2adsorptiondesorption was processed in a BETA201B analyzer to obtain the specific surface area, pore size, and pore volume.

The polynomial equation fitting the relationship between NMA concentration and reaction time under different experimental conditions is as follows [23]:

The NMA reaction rate is calculated by the following equation:

The NMA conversion rate is expressed as follows:

3.Results and Discussion

The catalytic hydrogenation of NMA is a typical gas-liquid-solid reaction.In order to obtain the intrinsic kinetic equation,the mass transfer resistance in the system should firstly be eliminated.

3.1.Elimination of external diffusion

The increment of stirring speed was used to eliminate the external diffusion [24].As shown in Fig.3, the NMA conversion increased with the increment of stirring speed from 400 to 1000 r·min-1.The NMA conversion did not increase significantly when the stirring speed reached up to 800 r·min-1, illustrating that the external mass transfer resistance was eliminated.At 1200 r min-1,the decrease of conversion may be ascribed to the change of flow pattern caused by the increase of stirring speed.The higher stirring speed would aggravate the bubble coagulation in the liquid phase,affect the gas-liquid mass transfer performance, and thus lead to the reduction of the conversion [25].Therefore, the stirring speed of 1000 r·min-1was conducted in the following kinetics experiments to ensure the elimination of external diffusion.

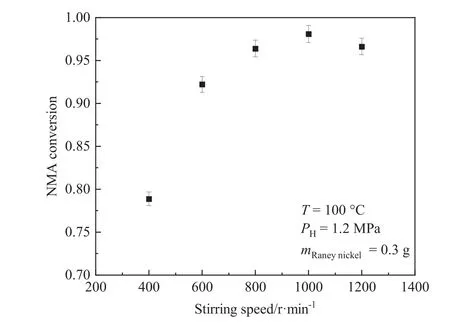
Fig.3.Effect of the stirring speed on the conversion of NMA.(Temperature:100°C;Hydrogen pressure: 1.2 MPa; Catalyst dosage (wet mass basis): 0.3 g; Reaction time: 1.5 h).

3.2.Elimination of internal diffusion

The internal mass transfer refers to the diffusion of reactants from the catalyst surface to the active sites.This process was affected by the particle size,pore size,and the specific surface area.Before the intrinsic kinetic experiment, it is necessary to ensure that the diffusion resistance inside the catalyst is eliminated completely.The influence of internal diffusion can be ignored if the particle size was less than 45 μm [20].The Raney nickel catalyst used in this paper was same as that used by Zhang et al.[26] in our previous study of p-nitroanisole hydrogenation.Combined with the characterization in Fig.4, the average particle diameter of Raney nickel catalyst was about 12 μm (much less than 45 μm), illustrating the elimination of internal diffusion.The pore parameters and specific surface area were characterized by the N2adsorption/desorption.In our previous study, the N2adsorption/desorption isotherm was accorded with the characteristics of type IV, indicating that Raney nickel catalyst possessed micropore or mesoporous structure [27-29].The BET surface area of the Raney nickel catalyst was 43.80 m2·g-1.The average pore size was 3.54 nm, yet the resistance caused by molecular diffusion within the catalyst can be ignored [30].

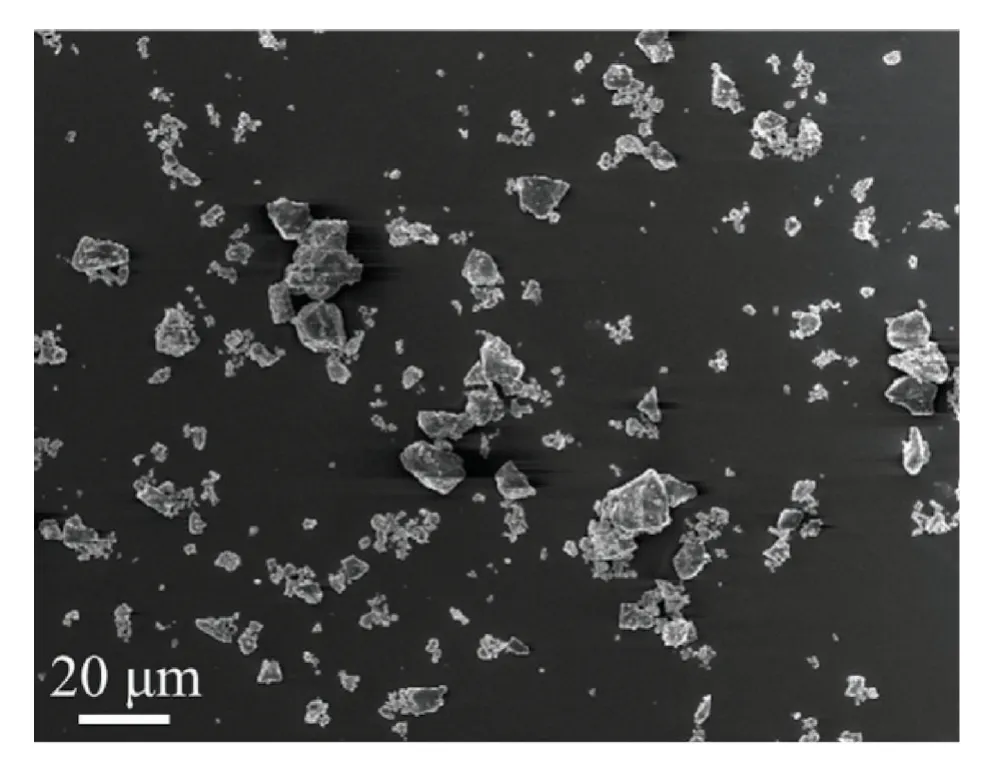
Fig.4.SEM image of Raney nickel catalyst.

In addition,the Weisz-Prater criterion can also be used to determine whether internal diffusion is eliminated or not.Based on the Weisz-Prater criterion [31], the internal mass transfer resistance can be ignored if

where i is NMA or H2.r is reaction rate, L stands for characteristic length of catalyst particle, Ciis molarity for the ith species, and Deiis the effective diffusion rate for the ith species.The effective diffusion coefficients (Dei) of hydrogen in liquid phase can be estimated by Wilke-Chang equation [32].The equation is as follows:

The equilibrium concentration of hydrogen in methanol (CH2)was calculated by the formula given by Pinter et al.[33].The relevant formulas are as follows:

In this work, the maximum value ofcalculated from all experimental groups was 1.77×10-4,much less than 1,satisfying the Weisz-Prater criterion.It demonstrated again that the internal mass transfer resistance of the catalyst could be ignored.

3.3.Intrinsic kinetics

3.3.1.Experiments

The hydrogen pressure is an essential factor to determine the intrinsic kinetic equation.As shown in Fig.5,the hydrogen pressure varied from 0.5 to 1.5 MPa,greatly affecting the NMA conversion and the reaction rate.The initial reaction rate at 1.5 MPa was 5.52×10-3mol·L-1·min-1, whereas it was 1.73 × 10-3mol·L-1·min-1at 0.5 MPa.Increasing the hydrogen pressure can obviously speed up the hydrogenation reaction rate.

Fig.5.Effect of hydrogen pressure on concentration of NMA(Temperature:100°C;Stirring speed: 1000 r·min-1; Catalyst dosage (wet mass basis): 0.3 g).

The reaction temperature could affect the molecular motion rate in the catalytic reaction.The effect of reaction temperature on the NMA concentration was studied.As shown in Fig.6,the initial reaction rate obviously improved with the increment of the temperature.The NMA conversion was less than 75%at 70°C after 1.5 h, while it reached up to 98% at 100 °C.With the increase of temperature, the effective intermolecular collision efficiency of reactants improved, benefiting to increase the reaction rate.

Fig.6.Effect of temperature on concentration of NMA (Catalyst dosage (wet mass basis): 0.3 g; Hydrogen pressure: 1.2 MPa; Stirring speed: 1000 r·min-1).

3.3.2.Power-law model

The power-law kinetic model is widely used for the reactor design and the optimization of process parameters, such as pressure, temperature and feed concentration.It can be obtained from the linear fitting of experimental data.The reaction rate of NMA is as follows:

where α and β are the reaction order of NMA concentration and hydrogen pressure, k is rate constant of reaction, respectively.In the kinetic equation, the intermediates generated in the reactionwere not considered because the intermediates can be fast converted to AMA [20,34,35].According to the Arrhenius equation,the reaction rate constant (k) and the temperature (T) satisfied the following relationship:

Substituting Eq.(10) into Eq.(9), and then processing the linearization:

The reaction orders were obtained by the linear fitting of experimental data (Fig.7).The result showed that the reaction order of hydrogen pressure was 1.10, and the reaction order of NMA concentration was 0.52.It was proved that the dependence of reaction rate on hydrogen pressure was greater than substrate concentration, which was similar with the finding of Bawane and Patil’s reports [19-20].The preexponential factor (k0) and activation energy (Ea) were obtained in the linear fitting of initial reaction rates under different temperatures.The preexponential factor(k0) was 12.58, and the activation energy (Ea) was 22.59 kJ·mol-1.

Fig.7.Relationship between ln (-rNMA) and T-1 fitted by the power-law model.

According to the above analysis for regression,the kinetic equation of hydrogenation reaction is as follow:

In order to verify the accuracy of the predicted kinetic equation,the calculated concentrations of NMA were compared with the experimental data.As shown in Fig.8,the established kinetic equation can well predict the concentration of NMA in the hydrogenation reaction.

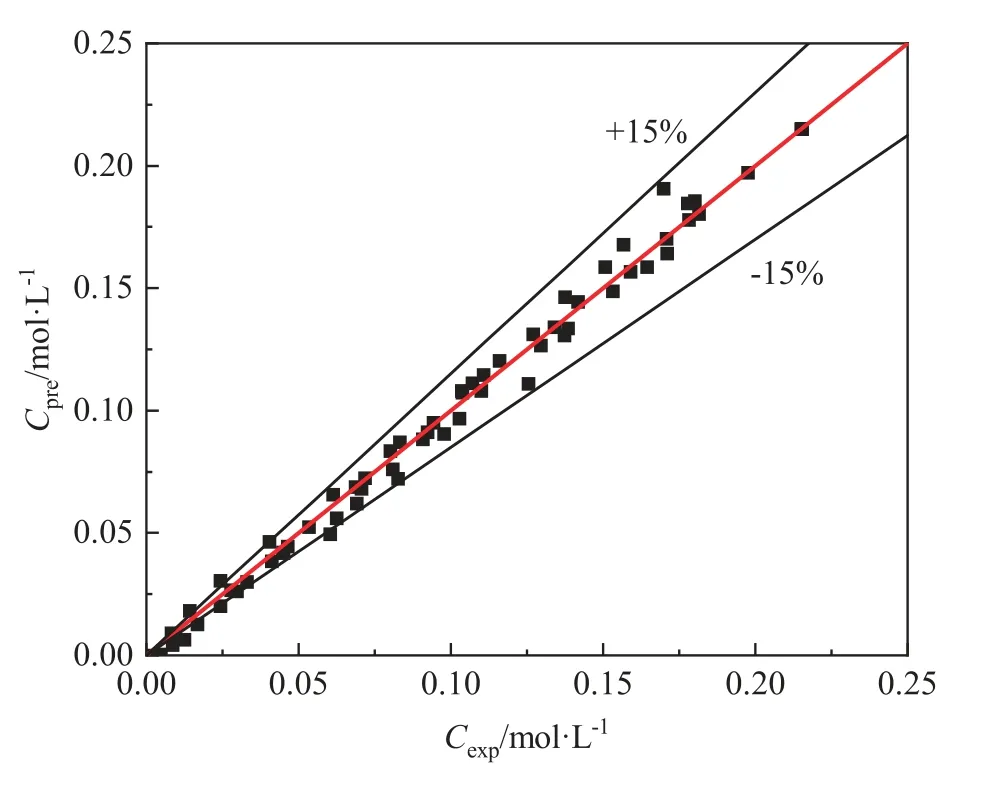
Fig.8.Comparison of the experimental Cexp and the predicted Cpre of NMA fitted by the power-law model.

3.3.3.Mechanism model

The mechanism model was further studied to reveal the ratecontrolling step and the adsorption mechanism of catalytic hydrogenation of NMA to AMA.The Langmuir-Hinshelwood-Hougen-W atson (LHHW) model and the Eley-Rideal (ER) model were most commonly used mechanism models [36,37].The LHHW model was widely applied to describe the catalytic hydrogenation of aromatic nitro compounds [20,23,24,38,39].The model satisfies the following three assumptions: (i) all the reactants are adsorbed and obey the Langmuir adsorption; (ii) the reaction process includes three steps: adsorption of reactants, surface reaction on the adsorption sites, and desorption of products; (iii) one of the steps is considered as the rate-determining step, while others are in equilibrium.In addition, the dissociation and adsorption of diatomic molecules (such as hydrogen) and the single or dual-site adsorption of reactants should also be considered.The ER model can also be used to describe the kinetic characteristics of a reaction system containing two different reactants.It assumes that only a kind of the reactants is adsorbed on the catalyst and the other reacts with the absorbed species [40].

Based on the dissociation adsorption of hydrogen molecules on Raney nickel catalyst [41], the detailed deduction process was showed in the Supplementary Material.The possible mechanism models were displayed in Table 2.

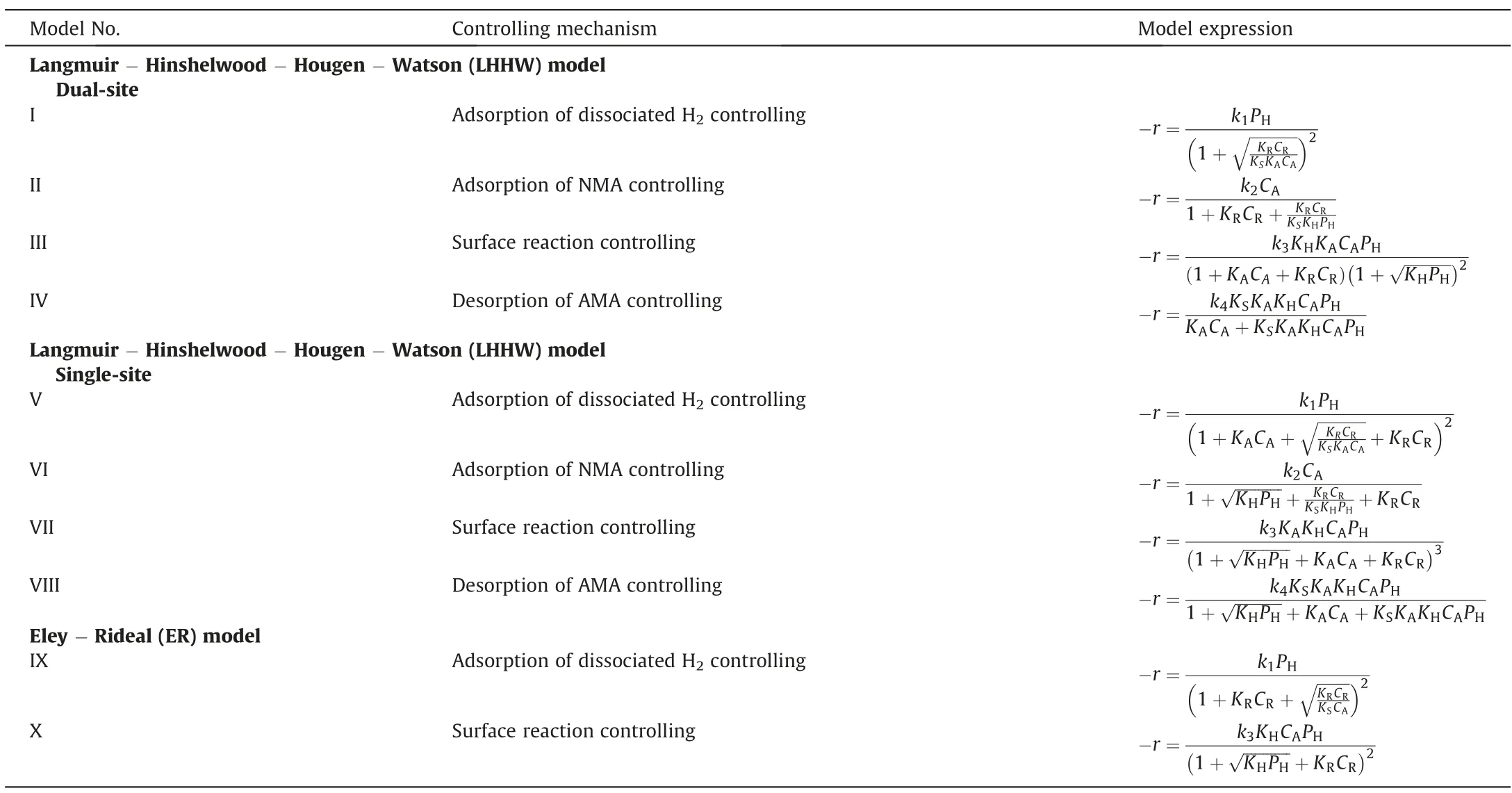
Table 2 Plausible ER and LHHW Models for the hydrogenation of NMA

In order to obtain the suitable mechanism model, the Gauss-Newton iterative method was employed to analyze the experimental data by nonlinear regression.In MATLAB, the Nlinfit function was used to modify the regression coefficient continuously.When the residual sum of squares of the original model met the requirements, the calculation was stopped.Then the reaction rate constant (k) and adsorption equilibrium constant (K) were obtained.The residual sum of squares is calculated as follows [42]:

The possible models were filtered according to the following regulations [43]:

Regulation 1: The rate constant is positive.(k ＞0)

Regulation 2: The intrinsic reaction activation energy is positive.(Ea＞0)

Regulation 3:The adsorption equilibrium constant of each substance is positive.(Ki＞0)

The result showed that three models (Models I, V and VIII) in Table 2 met the requirements.The rate constant (k) of Models V and VIII did not increase monotonically with the increment of temperature,violating the rules of Arrhenius equation.Compared with other models,model I is more reasonable.The parameters of Model I are listed in Table 3.Fig.9 shows the temperature dependence of the reaction rate constant(k)in Model I to calculate the activation energy(Ea).And the calculated Eafrom Model I was 21.94 kJ·mol-1,and was a little lower than that from the power-law model,which may result from the substitution of hydrogen adsorption rate for the overall reaction rate.This is consistent with the ratedetermining steps of nitro-hydrogenation mechanism models in many literatures.For example, hydrogenation of p-nitroanisole,p-nitrophenol, and 2,4-dinitrotoluene was controlled by hydrogen dissociation and adsorption [10,19,26].

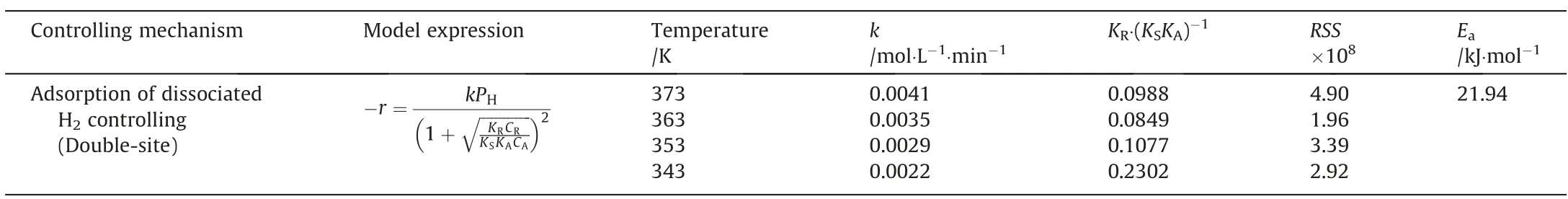
Table 3 Values of parameters of Model I

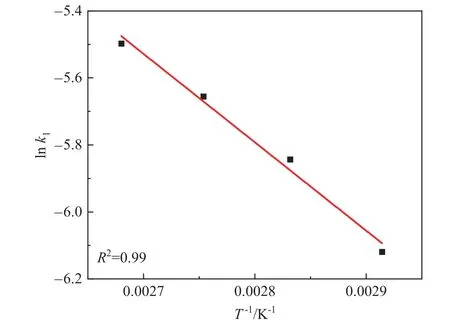
Fig.9.Relationship between lnk1 and T-1 fitted by the Model I based on LHHW.

A further comparison of the experimental and predicted intrinsic rate data(Model I)is shown in Fig.10.The results show that the intrinsic rate of NMA hydrogenation can be well predicted by the Model I.

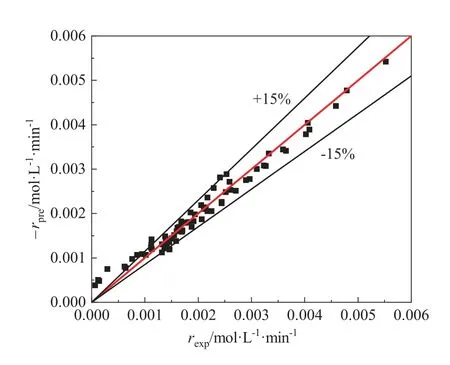
Fig.10.Comparison of the experimental reaction rate-rexp and the predicted-rpre of NMA fitted by the Model I.

4.Conclusions

The effects of reaction conditions such as temperature and hydrogen pressure on the catalytic hydrogenation rate of NMA have been investigated with Raney nickel catalyst under the premise of eliminating mass transfer resistance.The intrinsic kinetics equations for the catalytic hydrogenation of NMA were established by the power-law model and LHHW model.By fitting the experimental data using the power-law model,the reaction was found to follow 0.52-order kinetics in terms of the NMA concentration and 1.10-order kinetics with respect to the hydrogen pressure, respectively.The mechanism model based on LHHW showed that the reactants adsorbed at two sites,and the reaction was controlled by molecular dissociation adsorption of hydrogen.The activation energy of the surface reaction was determined to be 22.59 kJ·mol-1fitted by the power-law model and 21.94 kJ·mol-1fitted by the LHHW model.The calculated NMA conversions from power-law model and LHHW model were in good agreement with the measured values.The obtained kinetics data can be used to understand the reaction process and guide the reactor design and catalyst modification.

Declaration of Competing Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgements

This work was supported by the National Natural Science Foundation of China (22022802 and 22288102).

Supplementary Material

Supplementary data to this article can be found online at https://doi.org/10.1016/j.cjche.2023.06.013.

Nomenclature

Subscripts