Discussion of 90° stopband in low-energy superconducting linear accelerators

Xin-Miao Wan• Xue-Jiang Pu • Zhi-Qiang Ren • Wei-Hong Huang •Yu-Fei Yang • Zhi-Hui Li

Abstract Superconducting linear accelerators (SCL) have a high acceleration gradient and are capable of operating in a high-duty factor mode.For high-power and high-intensity SCL, the design of beam dynamics generally follows the principle that the zero-current periodic phase advance (σ0)of each degree of freedom is less than 90° to avoid envelope instability caused by space charge.However,this principle is obtained under the condition of a completely periodic focusing channel,and it is ambiguous for pseudoperiodic structures, such as linear accelerators. Although transverse beam dynamics without acceleration have been studied by other researchers,it appears that there are some connections between pure 2D and 3D beam dynamics.Based on these two points, five focusing schemes for the solenoid and quadrupole doublet channels were designed to simulate the beam behavior with non-constant σ0. Among them, the four schemes follow the characteristics of variation in the zero-current longitudinal phase advance (σ0l)under a constant acceleration gradient and synchronous phase. The zero-current transverse phase advance (σ0t) is consistent with σ0l,based on the equipartition requirement.The initial σ0t was set to 120°,110°,100°,and 90°,and was then gradually decreased to approximately 40°at the end of the channel.The last scheme maintains the maximum σ0t of 88° by reducing the acceleration gradient of the corresponding cavities, until the point at which σ0t equals 88° with a normal gradient. Using the stopbands obtained from the linearized envelope equations and multiparticle particle-in-cell (PIC) simulations, the transport properties of both continuous and 3D-bunched beams with the acceleration of the five focusing schemes were studied. It was found that for a CW beam, when tune depression ＞0.7,σ0t can break through 90°when the beams were transported in both solenoid and quadrupole doublet periodic focusing channels. When tune depression ＜0.7, the conclusions were different. For the solenoid focusing system, σ0t can partially break through 90°, and the beam quality is not significantly affected. For the quadrupole doublet focusing system, a partial breakthrough of 90° has a greater impact on the beam quality. The same conclusions were obtained for a bunched beam with acceleration.

Keywords Proton beam ∙Superconducting linear accelerators ∙Envelope instability ∙Periodic focusing structure ∙Resonance

1 Introduction

Linear accelerators (linacs) have been investigated for several applications[1].Since the successful first operation of a particle accelerator at the beginning of the last century,remarkable advances have been made in the physics and technology of linacs [2]. Theoretical and experimental studies on the stability of high-intensity beams in linacs began in the 1960s. Most of these theoretical studies have been based on two-dimensional transport models without acceleration[3].The associated strong space-charge effects present challenges in the transport of high-intensity beams[4]. One of the conclusions of these studies is that when transverse phase advance per period σ0tis greater than 90°,the space charge force may drive envelope instability and degrade the beam quality [5, 6]. To avoid envelope instability in high-intensity linacs, one of the rules that most designers follow is to maintain σ0t＜90° [7]. When the phase advance is near 90°, fourth-order resonance and envelope instability occur[8].The first experimental study of the fourth-order resonance in an operational high-intensity accelerator was undertaken at GSI UNILAC,which confirmed the emittance degradation [9]. For Kapchinskij-Vladmirskij (K-V) beams, using perturbation analysis of the linearized Vlasov–Poisson equations, stopbands of instability can be obtained [5]. Stopbands are defined as regions in which any eigenvalue lies outside the complex unit cycle. In stopbands, the emittance increases exponentially in a nonzero state. Higher-order and lower-order stopbands may coincide, but instability typically decreases with increasing order [10]. Meanwhile, the special case of the ‘‘second-order even’’ mode is known as the envelope instability [11]. Envelope instability is characterized by parametric and confluent resonance. Parametric resonance represents the resonance between the focusing lattices and one of the envelope oscillatory modes that occurs only in the solenoid channel. When parametric resonance occurs,only one of the breathing and quadrupole modes excites resonance with the beam core. This is also called halfinteger resonance because it represents the envelope eigenmode that oscillates at half the frequency of the periodically varying focusing (including space charge).Confluent resonance represents the resonance between both envelope oscillation modes and lattices,which occurs only in the quadrupole channel [12].

The space charge force not only causes envelope instability, but may also cause coupling of the particle motions and emittance exchange in different directions[13]. Therefore, Jameson introduced the equipartition concept into the beam dynamics design of linear accelerators [9, 14]. By properly setting the longitudinal and transverse focusing strengths, the oscillation energies of different degrees of freedom were balanced,and there was no free energy for resonance to occur.For the equipartition design, the motions of the particles in the transverse and longitudinal directions are decoupled. Therefore, the transverse and longitudinal motions of the superconducting linear accelerator (SCL) based on equipartition design can be studied separately. However, σ0tand σ0lmaintain the same trend because of the requirement for equipartition.

SCL can provide high-power beams and have many applications in various fields. It has negligible wall power losses, which makes it possible to operate in the high-duty factor mode with a higher accelerating gradient. The SCL is typically composed of different acceleration sections,and each section is composed of identical cells [15]. The significant difference between SCL and periodic focusing channels is that SCL have radio-frequency (RF) cavities.The RF cavity can not only accelerate beams, but can also provide focusing in the longitudinal direction and defocusing in the transverse direction to the particles[16].One of the most important outcomes for the SCL is the pseudoperiodic focusing structure, which may lead to different dynamics.When the acceleration gradient and synchronous phase are constant, the square of the focusing strength is inversely proportional to the 3D power of the βγ of particles [17]

Thus, the maximum σ0loccurs at the beginning of each section and decreases as the beam energy increases with the SCL. However, cavities at the beginning of each section must work under a reduced acceleration gradient because of the principle that σ0＜90°. This significantly reduces the acceleration efficiency.

The remainder of this paper is as follows.In Sect.2,five different focusing schemes are designed and presented. In Sect. 3, the types and characteristics of periodic focusing channels and an approach for calculating the stopbands are introduced in detail. In Sect. 4, the beam dynamics of five different focusing schemes are shown. Finally, the effects of σ0lexceeding 90° in a real SCL section are studied and verified. The conclusions and discussion are presented in Sect. 6.

2 Different focusing schemes

To study the transverse beam dynamics when σ0partially exceeds 90°, five different focusing schemes were designed to simulate σ0t. The maximum σ0tof the beam was designed to be 120° (①), 110° (②), 100° (③), and 90° (④).The purpose was to simulate the trend of σ0tunder different acceleration gradients in the presence of an acceleration field. A higher acceleration gradient corresponds to a larger σ0t. The first scheme corresponds to the highest acceleration gradient and the fourth scheme corresponds to the lowest acceleration gradient. To avoid excessive sensitivity of the beam to the focusing parameters, for every focusing scheme, the minimum value of σ0twas generally set to 40° after the beam passes through 80 focusing periods.Therefore,we designed focusing schemes based on a logistic function. This function is more suitable for the changes in σ0t. In addition, for the case in which the maximal σ0twas 120°,a control scheme was set to avoid envelope instability on the beam.The maximal σ0tof this control scheme was 88° (⑤), where envelope instability does not occur. This can be controlled by reducing the acceleration gradient of the corresponding cavity. Once σ0tof ①was less than 90°, ⑤and ①remain within the same gradient. The common logistic function is given by

In the design functions, x represents the period number,and y represents the value of σ0t.The specific parameters of the functions are listed in Table 1. Additionally, σ0tof the five schemes are shown in Fig. 2.

3 The focusing channels and stopbands

3.1 Periodic focusing channels

According to the transverse motion equation, the RF electromagnetic field has an evident defocusing effect and axial symmetry at the low-energy end of the linear accelerator.Considering that the beam has a strong space-charge effect at the low-energy end, a relatively compact transverse focusing lattice is required to maintain an appropriate beam size within our design. Magnetic quadrupoles, solenoids, and axisymmetric electrostatic lattices were chosen by designers for beam transport systems at low-energy ends[18]. In this study, a solenoid, which has axial symmetry,and a quadrupole doublet, which is non-axisymmetric,were chosen as the focusing elements. The specific parameters of the focusing elements used for the following discussion are shown in Fig. 1. For the solenoid periodic focusing system, the κ(s) is given by [12]

where G is the quadrupole linear strength gradient.

The distribution of beam particles in phase space plays a significant role because it is directly related to the space charge in high-intensity proton accelerators. The K-V distribution is an ellipse with uniform density in any projected phase plane, which is beneficial for calculating the space charge force. This is because the space charge force is a linear defocusing force that can be compensated for by an external field. Therefore, the K-V distribution is most commonly used for researching space charges in simulations.

From the research of Sacher, the exact form of the distribution hardly works on the linear part of the self-field,while the RMS size of the distribution plays an important role. The envelope equations can be used directly in simulations when there is little variability in the distribution during beam transport. Simultaneously, the results of previous research have shown that beams with different distributions but with the same beam emittance terms can be equivalently considered [19]. Generally, it is justified to use the results from the K-V distribution for the other beam distributions.

When particles are randomly generated in the K-V transverse ellipsoid,the envelopes where X(s)and Y(s)are in the two transverse directions of the periodic focusing channels are described by [12]

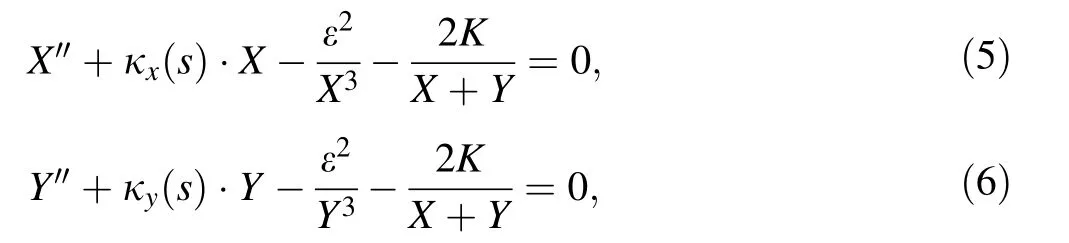
where K = 2eI/4πε0mc3β3γ3is the generalized provenance.Meanwhile, κx(s ) and κy(s ) represent the transverse focusing functions, and ε=εx=εyduring beam transport.

3.2 Calculating the stopbands

The mismatch between the periodic focusing channels and particles can cause envelope resonance. This is an important factor in space-charge-dominated beams. At present,there are several methods for calculating envelope instability stopbands caused by resonance. First, using the theory established by Struckmeier and Reiser, it is solved by linearizing differential equations with disturbance terms[11].Second,the perturbation theory based on the Chernin matrix can also obtain a complete set of second-orderinstabilities, including even and odd modes. The sum effects of the resonance on the beam were also analyzed[20]. Finally, perturbation theory based on the Vlasov–Poisson equations introduced by Hofmann provides an approach for calculating the stopbands of higher-order perturbations [5]. Because the perturbation theory established by Struckmeier and Reiser is relatively mature,widely used, and highly reliable, envelope instability stopbands are calculated based on this theory.

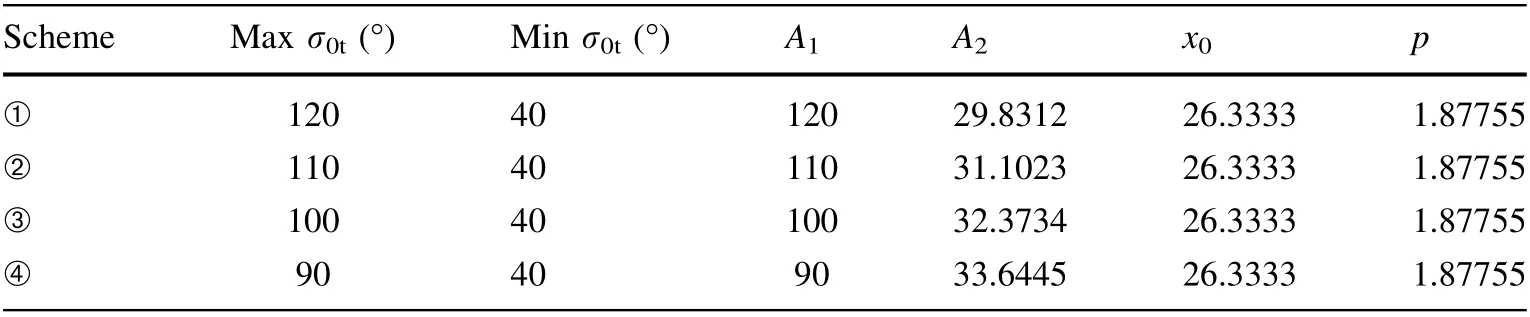
Table 1 The specific parameters of logistic functions for σ0t

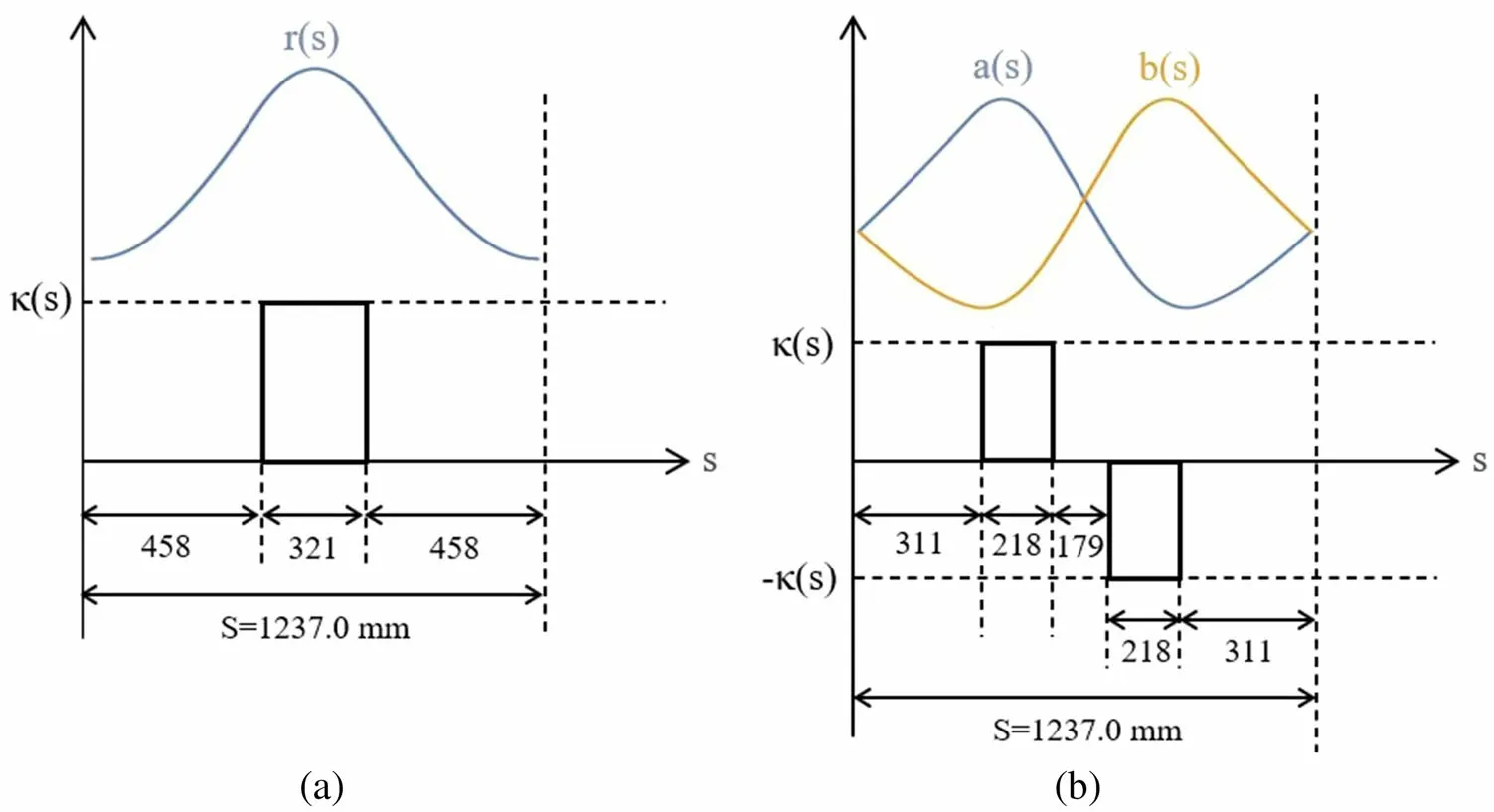
Fig. 1 Periodic focusing elements and the corresponding matched beam envelopes.a Solenoid, b quadrupole doublet. The length of each element is 1237.0 mm

With z=(x,x/,y,y/), an ensemble of phase space trajectories is represented which satisfies Z (s+S)=M (s )Z (s ) in a periodic dynamics system. It is useful to define M(s)as the transfer matrix,where M (s )=M (s+S)is also satisfied in a periodic dynamics system. Overall, λ denotes the eigenvalues of the transfer matrix that can be greater than 1 or less than 1.If |λ |=1,the system is stable;otherwise, the system is unstable. Meanwhile, λ satisfies λ= |λ |eiφ,where φ represents the eigenphase Arg[M(s)]of the transfer matrix. Additionally, φ connects with the envelope modes [21]. Finally, by converting φ to σ, a stopband with a specific σ0tcan be obtained under a certain focusing structure.

4 Simulation results of beam evolution under different focusing schemes

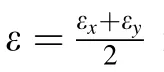
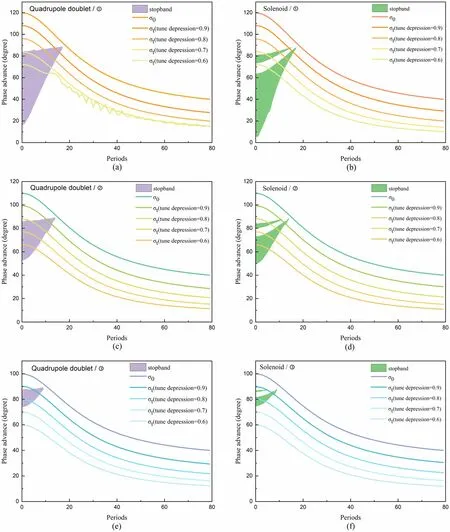
Fig.2 (Color online)The stopbands correspond to different focusing schemes and different periodic channels.Each picture shows the change of beam σt under different tune depressions

It is clear that there are significant differences in ε between the quadrupole doublet and solenoid focusing channels. For the quadrupole doublet channels, when tune depression 0.7 and 0.6, the ε of focusing scheme ①increased significantly, while the beam designed by other focusing schemes exhibited no such behavior. For the solenoid channels, the tune depression and focusing schemes seem to have no significant effect on the beam quality.This shows that the beam quality can be improved using a periodic focusing solenoid channel.

Fig. 3 (Color online) The ε when tune depression was equal to 1.0,0.9, 0.8, 0.7, and 0.6 for different focusing schemes, respectively.a shows the results when the beams are transported in periodic focusing quadrupole doublet channels, and b shows the results when beams are transported in a periodic focusing solenoid channel

This can be explained as follows. It can be seen from Fig. 2 that the reduction speed of σ is consistent with σ0t;beams transported in the solenoid channel take less time to cross the stopbands under the same focusing scheme and tune depression.With the enhancement of the space charge effect and the increase in the maximum value of σ0t, this phenomenon becomes more obvious. In stopbands, the beams are affected by envelope instability, which deteriorates the beam quality. Moreover, most of the beams transported in the solenoid channels do not enter the stopbands at the beginning. This implies that the beam is less affected by the initial state.

Second, from previous studies by other researchers,envelope instability is caused by linearized perturbed oscillations of the breathing mode and quadrupole mode.The perturbations of the beam envelope are in phase when the breathing mode occurs, whereas out-of-phase perturbations of the beam exist when the quadrupole mode occurs. Parametric resonance can occur only in the breathing mode or quadrupole mode. Certainly, there is another type of resonance, called confluent resonance.Confluent resonance occurs when the two modes occur simultaneously. Confluent resonance means that the resonance effect is between the two envelope modes and focusing lattice. In a solenoid focusing channel, only one breathing or quadrupole mode can occur. This also means that,for a beam propagating in a solenoid,only parametric resonances cause instability.Meanwhile,the decoupling of the breathing and quadrupole modes means that the stopbands of parametric resonance for the two modes do not overlap. In contrast, in a quadrupole doublet focusing channel, only confluent resonance occurs, which involves locking of the breathing and quadrupole modes. The breathing and quadrupole modes are closely related and cannot be strictly separated [25]. If one mode is activated,the other is activated, which can result in a larger emittance. Third, compared to a solenoid channel, in a quadrupole doublet channel, the high-order stopbands overlap more with the envelope instability stopband, which can lead to an increase in emittance.

From Fig. 3a, it can be seen that when tune depression equals 0.7 or 0.6, the ε corresponding to focus scheme ①reaches a maximum, and when tune depression equals 0.6,ε has exceeded 10% of the initial emittance. To analyze this, particle distribution diagrams when beams were transported in the quadrupole doublet focusing channel with different tuning depressions are shown in Fig. 4.Simultaneously, relevant information about the beam transported in the solenoid focusing channel when the tune depression is equal to 0.6 is shown in Fig. 5,corresponding to focusing scheme ①.

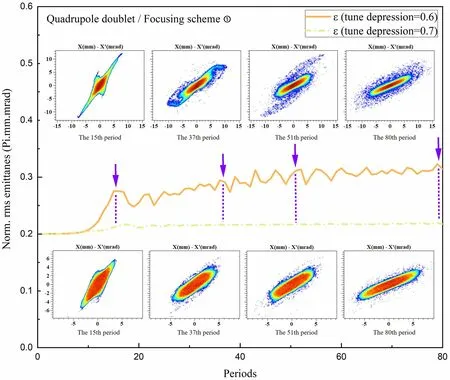
Fig.4 (Color online)The ε and phase space distribution evolution along a quadrupole doublet channel under the focusing scheme ①when tune depression is equal to 0.6 and 0.7, respectively. Here, the particle distribution diagrams of the 15th, 37th, 51st, and 80th periods are shown

From Fig. 4,it is clear that there is a four-fold structure and envelope instability structure in the phase space distribution of the particles,and there is an obvious growth in ε when the tune depression is equal to 0.6.The appearance of these structures is mainly due to the fourth-order resonance and envelope instability. Along with the beam envelope transport,envelope instability is excited from the mismatch generated by the fourth-order resonance [26].Moreover, the fourth-order resonance appears earlier than the envelope instability. This also means that the fourthorder resonance stopband has some overlapping regions with an envelope instability stopband. Furthermore, as shown in Fig. 2a, the beam envelope enters the envelope instability stopband for a relatively long time in the beginning when the tune depression is equal to 0.7 and 0.6,respectively. Hence, it is easy to observe an unstable structure in the phase–space distribution of the particles.In addition,as shown in Fig. 2,with a tune depression equal to 0.6,the beam takes more time to cross through the stopband. It seems that the stopband has a longer time to attract the beam owing to the space-charge effect. This further increased the emittance. As for the case in which the tune depression was equal to 0.7,in the first 20 periods,the distribution of particles in the phase space shows a four-fold structure.Hence, there is also a small increase in the emittance. The fourth-order resonance of the particle does not cause instability in the beam envelope, and the stopband has no obvious attraction to the beam.Finally,we analyzed the dynamics of the beam in the solenoid channel,as shown in Fig. 5. It is clear that the emittance does not exhibit a significant growth, regardless of the value of the tune depression and the scheme of focusing. Only the distribution of the particles in the phase space was elongated.

5 Simulation results of beam evolution with acceleration

The above simulation results are all obtained using pure 2D transverse dynamics, which do not consider acceleration. The beam dynamics when σ0tlocally exceeds 90°under acceleration are ambiguous. In this section, we consider 3D beam dynamics and simulate the evolution of the beam under acceleration. To explore this, we assumed two periodic acceleration channels, where each period element contains, for example, focusing lattices and acceleration structures, as shown schematically in Fig. 6.The length of each periodic element was 1236.0 mm,which is similar to that of the periodic element shown in Fig. 1.

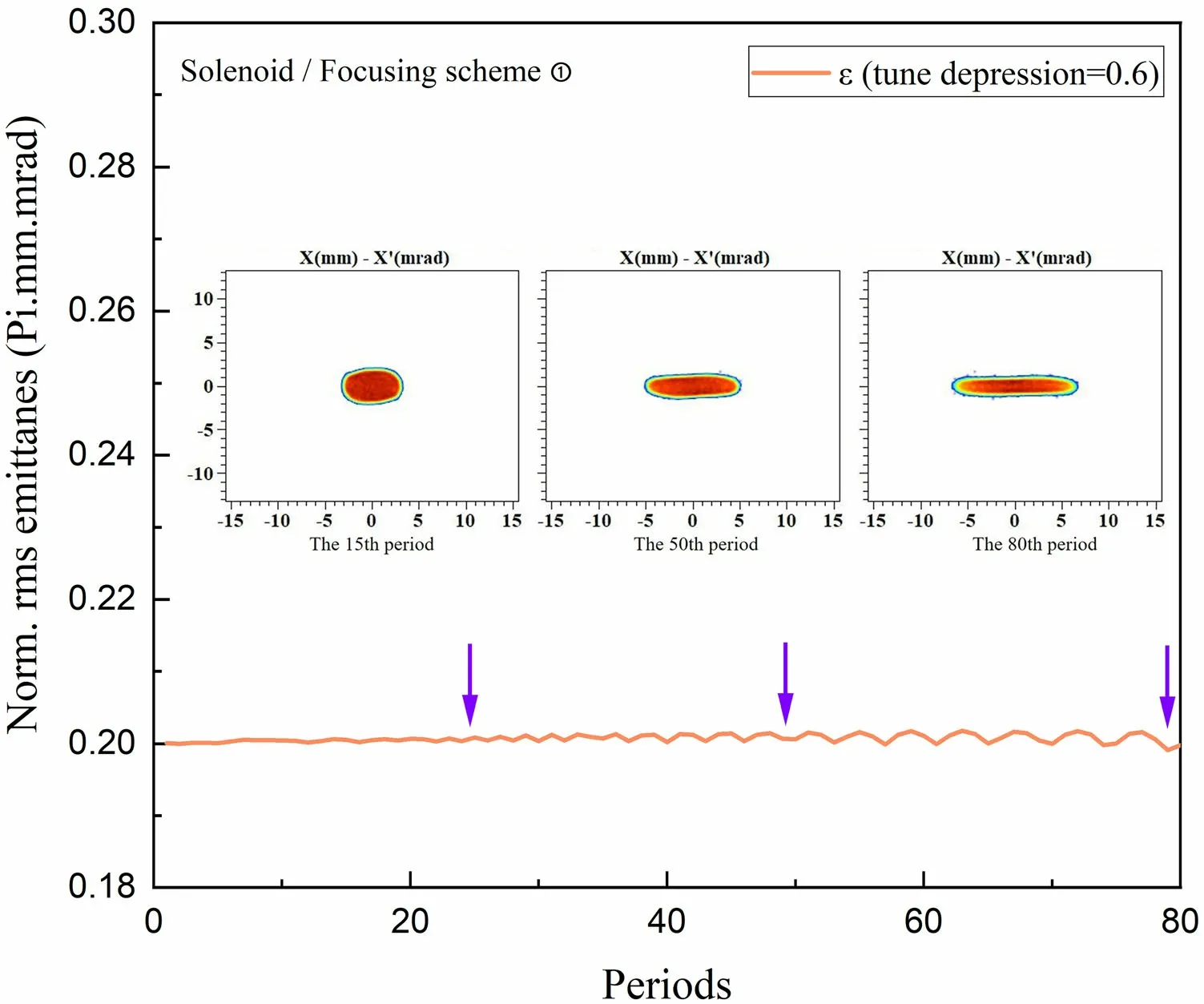
Fig.5 (Color online)The ε and phase space distribution evolution along a solenoid channel under the focusing scheme ①when tune depression is equal to 0.6.Here,the particle distribution diagrams of the 15th, 50th, and 80th periods are shown

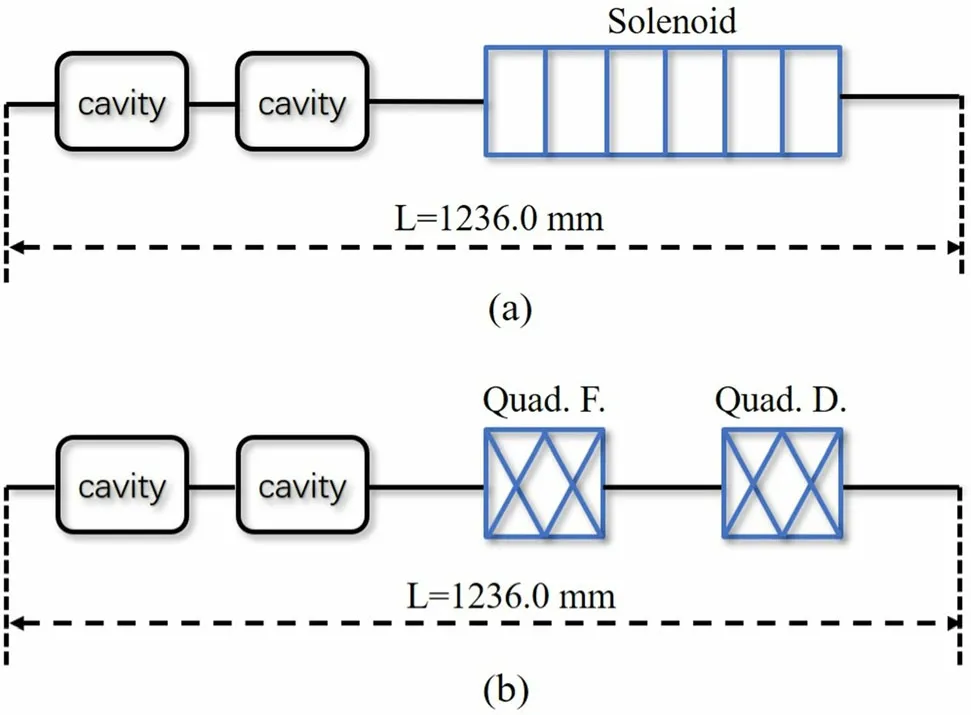
Fig. 6 Schematic plots of two periodic acceleration channels.a Shows the periodic element when beams are focused by solenoid lattice, and b shows periodic element when beams are focused by quadrupole lattice. The length of each cavity was 200.0 mm

From Fig. 8, we can see that ε increased as the tune depression decreased (the current intensity increased),regardless of which periodic element was used. For the element that provides focusing strength by the quadrupole lattice, ε exhibits a large increase when tune depression was equal to 0.6. There are two reasons for the increase in ε:one is the influence of the space charge, and the other is the nonlinear influence of the accelerating field. Under the action of a non-linear force,particles with larger amplitude will feel a much stronger force,which makes them deviate from the law of harmonic oscillatory motion that leads to an increase in beam emittance. As the beams are focused by the solenoid lattices, ε increases slightly.

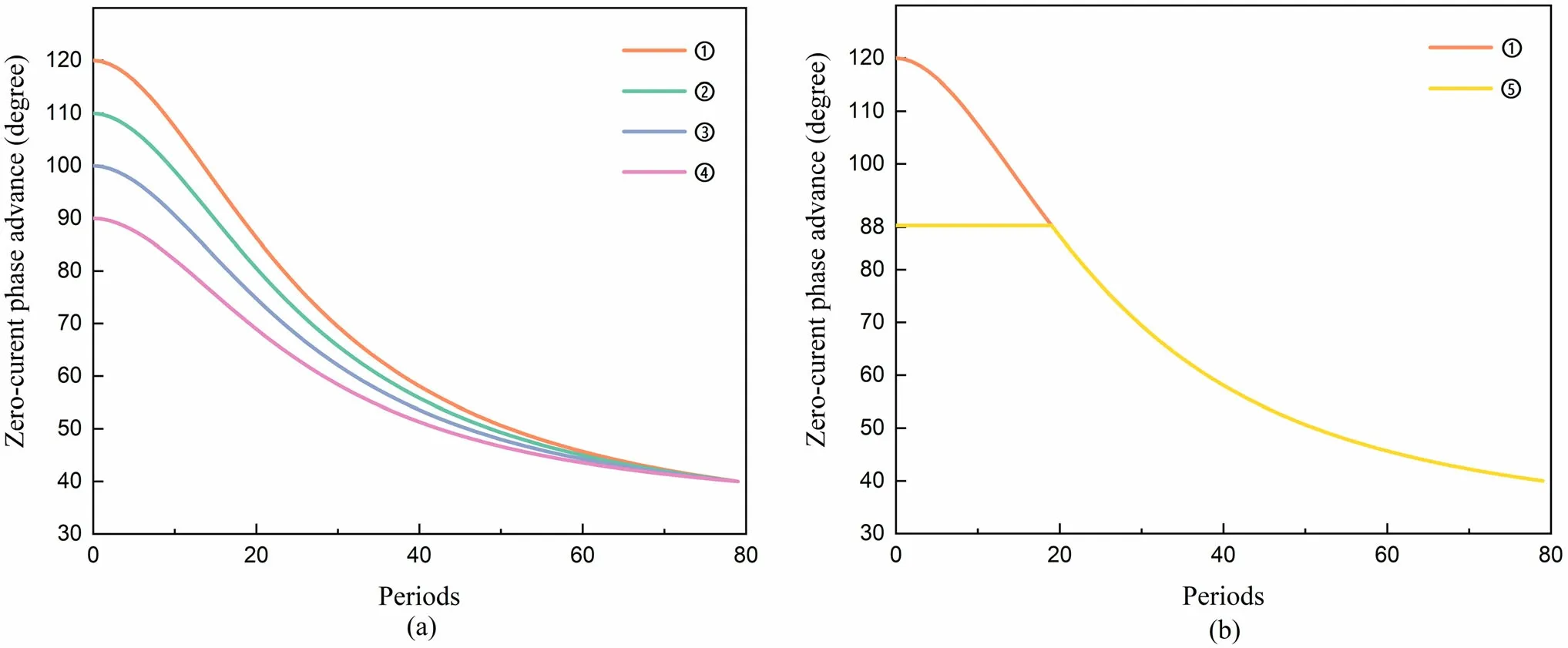
Fig. 7 (Color online) σ0t for the five schemes. a Shows the focusing schemes ①–④and b shows the focusing schemes ①and ⑤

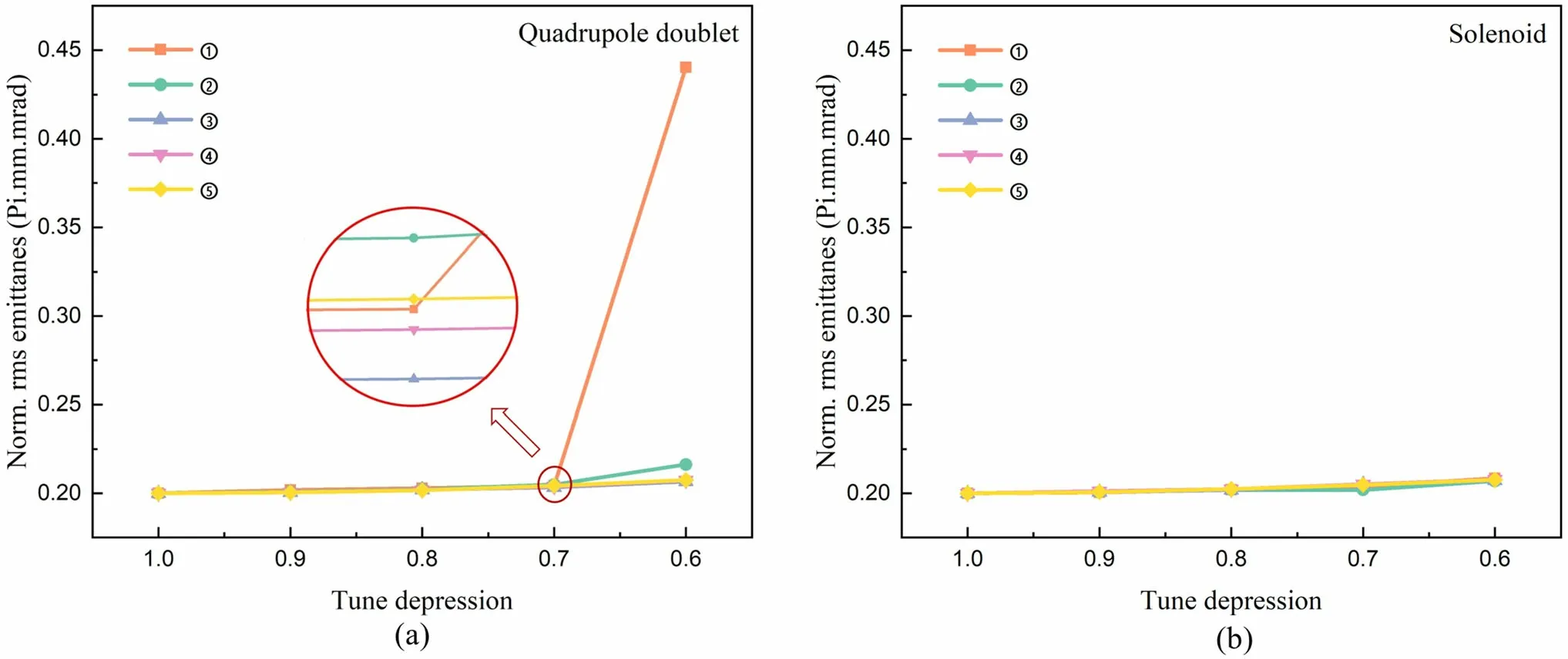
Fig. 8 (Color online) ε during beam transport. a Shows ε when beams were focused by quadrupole lattices and b shows ε when beams were focused by solenoid lattices. Different serial numbers correspond to different focusing schemes in Table 1

Compared to the results obtained by the 2D simulation,ε is mostly consistent. This means that the results obtained by the 2D simulation can be extended to 3D simulations.However, there are some differences. When the tune depression was equal to 0.6, ε was larger under focusing scheme ①. Based on this phenomenon, we separately analyzed the beam evolution under focusing schemes ①and ②. Figure 9 shows the corresponding particle distribution diagrams when the beam passed through the 9th,24th,and 80th periods.When σ0twas changed according to focusing scheme ①, the distribution of particles in the phase space had a typical four-fold structure, and filamentation occurs,but the beam core maintained a four-fold structure within a certain number of periods. Finally, the bunch was transported in the acceleration channel in an unstable structure. Therefore, in this case, the increase in the beam emittance results from the fourth-order resonance and envelope instability. This result was consistent with our conclusions. When σ0twas changed according to focusing scheme ②, the distribution of particles in the phase space demonstrated a slight filamentation, but this stabilizes as the beam is transported.

6 Conclusion

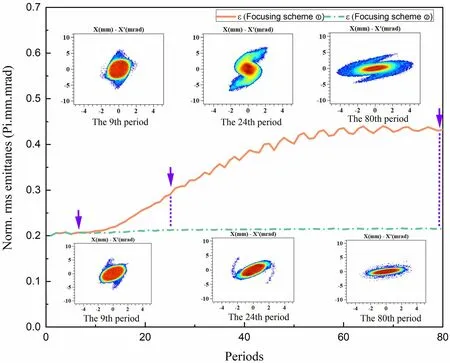
Fig. 9 (Color online) ε of focusing schemes ①and ②and the phase space distribution evolution along an acceleration channel that provides focusing strength by quadrupole doublet lattice (shown in (b) of Fig. 6)when the tune depression was equal to 0.6. Here, the particle distribution diagrams of the 9th,24th and 80th periods are shown

In this study, we analyzed the beam dynamics when σ0partially exceeded 90°. Under the condition of equipartition, we used the logistic function to fit σ0under acceleration and then calculated the stopband with a non-constant σ. From the simulation of the beam’s transverse motion, it is clear that the beam quality is affected by the focusing scheme, tune depression, and periodic focusing channel.By comparing the time at which the beam crosses the stopband and analyzing the particle distribution in the phase space, we concluded that when the tune depression was ≤0.7, the beam quality was significantly affected by the space charge.Furthermore,when σ0tpartially exceeded 90°, the emittance of the beam transported in the quadrupole doublet focusing channel increased significantly,which is inseparable from the superposition of the oscillation modes in this focusing structure. When the maximum σ0twas 120°, the distribution of the particles in the phase space exhibited an obvious fourth-fold structure over a long period of time.In addition,the main reasons for the increase in emittance are the fourth-order resonance and envelope instability. Of course, higher-order instability also affects the beam quality to a certain extent. However,for the solenoid focusing system, there was no significant increase in the emittance under the same conditions.When Tune depression was greater than 0.7,the periodic focusing structure type and focusing scheme demonstrated little effect on the beam quality. We then simulated the beam dynamics while considering acceleration. Beams are focused on solenoid lattices, which seem to have result in high beam quality.However,beams focused on quadrupole doublet lattices show different results. When the tune depression was 0.6, ε reaches its maximum value. This is mainly due to the envelope instability and nonlinear forces in the longitudinal phase space. By comparing the results obtained from the 2D simulation with those obtained in 3D,we find that they are highly consistent. Therefore, the results obtained from 2D simulations can be extended to 3D simulations.

Author ContributionsXin-Miao Wan and Zhi-Hui Li contributed to the study conception and design.Material preparation,data collection and analysis were performed by Xue-Jiang Pu,Wei-Hong Huang,Yu-Fei Yang Xin-Miao Wan and Zhi-Qiang Ren. The first draft of the manuscript was written by Xin-Miao Wan and Zhi-Hui Li.All authors commented on previous versions of the manuscript.