400～1 300 nm 波段的卤钨灯光谱辐照度衰减模型

张亚超，叶 新，夏志伟，隋 龙，方 伟

（中国科学院 长春光学精密机械与物理研究所, 吉林 长春 130033）

1 Introduction

In the 21st century, climate change has became a topic of global concern. Global climate change research has put forward unprecedented requirements for the radiation measurement uncertainty of remote sensing satellites. The ASIC3(Achieving Satellite International Corporation for Climate Change) states that the measurement uncertainty of the remote sensing satellites has to remain better than 0.3% at the reflected solar wavebands to accurately predict global climate change[1].

However, the radiometric calibration of the satellite optical remote sensors at reflected solar wavebands is usually performed by the standard halogen tungsten lamp, the solar-diffuser calibrator or vicarious calibration. At present, the minimum and maximum spectral irradiance uncertainty of standard halogen tungsten lamp is 0.47% at wavebands of 1 600 nm (k=2) and 1.16% at wavebands of 400 nm (k=2) during its lifetime[2]. Therefore, standard halogen tungsten lamps cannot satisfy a measurement uncertainty of 1% (k=2) at whole reflected solar wavebands. Moreover, radiometric calibration uncertainty is only about 5% at reflected solar wavebands by solar-diffuser calibrator and vicarious calibration[3-7]. Therefore, the current radiometric calibration methods hardly satisfy the high-precision radiometric calibration requirements in the field of climate research.

An alternative method to improve the accuracy of radiometric calibration is to trace the spectral radiance of the lamp-diffuser calibrator to the onboard space cryogenic absolute radiometer.However, it is impossible to do this at each operating waveband of the remote sensor in orbit. Therefore, it is necessary to reconstruct the spectral radiance of the entire operating waveband range from the spectral radiances of several operating wavebands. In addition, the reconstruction uncertainty must be better than 0.3% to realize the target measurement uncertainty of 1% of reflected solar spectral radiation.

At present, the spectral radiance (irradiance) of the light source is reconstructed according to its spectral radiance (irradiance) model. For example,the spectral irradiance of a halogen tungsten lamp can be reconstructed with an uncertainty of approximately 0.25% according to its spectral irradiance model[8]. However, the spectral radiance of the lamp-diffuser calibrator cannot be described by a simple but precise model, as such a model has not yet been reconstructed for the diffuser reflector.Therefore, it is difficult to reconstruct the spectral radiance of the lamp-diffuser calibrator with an uncertainty of 0.3% using the present reconstruction method.

It is well known that the degradation characteristics of the diffuser reflectors in the Moderate Resolution Imaging Spectroradiometer (MODIS) and Visible Infrared Imaging Radiometer Suite (VIIRS)can be described by an analytical model[9]. Therefore, it is reasonable to believe that the degradation characteristics of the other on-board diffuser reflectors can also be described in this way. The spectral radiance degradation characteristics of the lampdiffuser calibrator can correspondingly be described by an analytical model if the spectral irradiance degradation characteristics of the halogen tungsten lamp can be described by an analytical model. In addition, the spectral radiance degradation curve of the lamp-diffuser calibrator can be reconstructed with high accuracy according to its spectral radiance degradation model. The high-precision spectral radiance of the lamp-diffuser calibrator can subsequently be calculated by the product of the reconstructed spectral radiance degradation curve and the spectral radiance calibrated before launch.

In summary, it is necessary to research the high-precision spectral irradiance degradation model of a halogen tungsten lamp to reconstruct the spectral radiance of the on-board lamp-diffuser calibrator with an uncertainty better than 0.3%. In this paper, the following work was performed to construct the spectral irradiance degradation model of a halogen tungsten lamp from the Chinese Radiometric Benchmark of Reflected Solar Band project.First, the spectral irradiance degradation model of a halogen tungsten lamp with undetermined order was derived according to the blackbody radiation law and Weierstrass theorem in Section 2. Next, the spectral irradiance degradation curve was measured and the criterion to determine the model order was given, according to which the model order was determined in Section 3. The conclusion is presented in Section 4.

2 Derivation of spectral irradiance degradation model

Because the spectral irradiance degradation curve of a halogen tungsten lamp is the ratio of its spectral irradiances at different moments, the spectral irradiance degradation model is closely related to its spectral irradiance model. The physical model of the halogen tungsten lamp irradiance is expressed in Eq.(1):

whereB(T) is a geometrical factor of the lamp filament that takes into account the measurement distance and the dimensions of the filament; λ is the wavelength in a vacuum;Tis the temperature of the filament; εW(λ,T) is the nominal spectral emissivity of tungsten; εΔ(λ) is the spectral emissivity correction factor for the emissivity of the lamp;h¯ is the Planck constant;cis the velocity of light in a vacuum; andkis the Boltzmann constant[10]. The residual correction factor accounts for the effects of all factors in addition to the nominal spectral emissivity of tungsten. These include the transmittance of the quartz bulb, the transmittance of the filling gas,the difference in the properties of tungsten used in the lamp filament and in the nominal emissivity determination, and the light recycling effect in the coiled filament[11-12].

In this paper, the product of the geometric factorB(T), the nominal spectral emissivity of tungsten εW(λ,T), and the spectral emissivity correction factor εΔ(λ) is referred to as the spectral emissivity of the halogen tungsten lamp ε(λ,T). Therefore, the spectral irradiance of the halogen tungsten lamp can be expressed as the product of the Planck functionH(λ,T)and the spectral emissivity, as shown in Eq.(2):

If the increments of the Planck function and spectral emissivity are expressed as ΔH(λ,T) and Δε(λ,T), respectively, when the filament temperature changes fromTtoT+ΔT, the spectral irradiance degradation model of the halogen tungsten lamp can be expressed as Eq. (3):

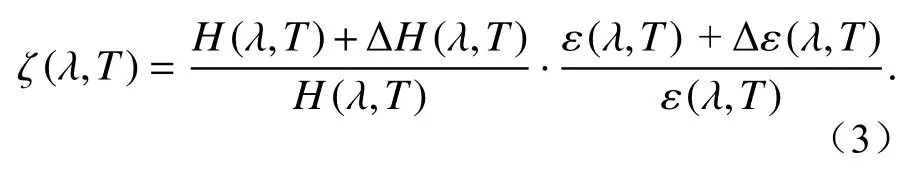
When λ is less than 1300 nm,the P(lanc)k functionH(λ,T)is reduced to Eq.(4)as exp≫1 is satisfied:

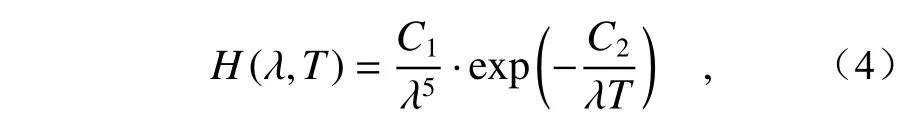
whereC1andC2are the first and second radiation constants, respectively. The increment of the Planck function can be expressed as Eq. (5) derived from Eq. (4) by the differential operation.

Because the spectral emissivity degradation model of a halogen tungsten lamp is affected by the spectral emissivity of tungsten, the spectral transmittance of the quartz lamp shell, the spectral transmittance of the halogen gas, and the filament morphology, it is difficult to achieve the analytical expression through theoretical derivation. However,any continuous function can be approximated by an algebraic polynomial function according to the Weierstrass theorem. Therefore, the spectral emissivity degradation model of a halogen tungsten lamp can be approximated by the polynomial function shown in Eq. (6):

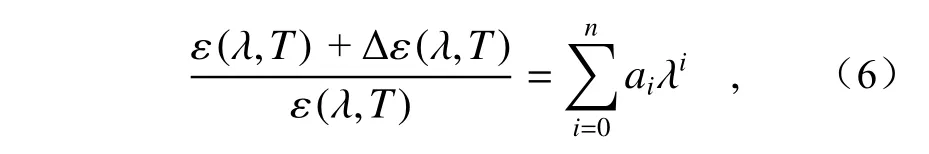
whereaiis an undetermined coefficient. Therefore,the spectral irradiance degradation model of the halogen tungsten lamp can be expressed as Eq. (7)according to Eqs.(4)-(6).

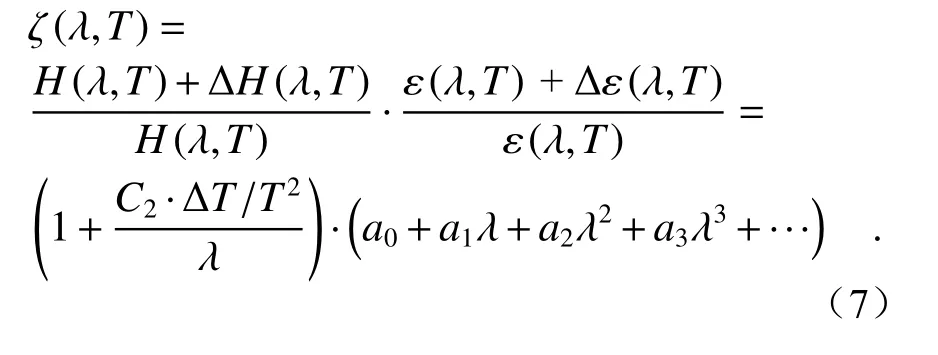
Since the temperatureTand temperature difference ΔTwere contained in the undetermined coefficients ξ0,ξ1,η1,η2,η3···, Eq.(7) can be simplified to be Eq.(8).

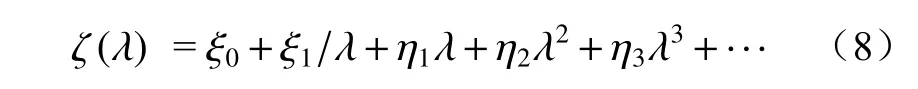
There may be several different orders of the model that satisfy the reconstruction uncertainty required by the spectral radiance of the lamp-diffuser calibrator. However, the model order should be determined as the lowest order that satisfies the spectral radiance reconstruction uncertainty of the onboard lamp-diffuser calibrator, which is referred to as the model-order-determination criterion in this study. The lowest-order model requires the fewest wavebands to reconstruct from the spectral radiance of the on-board lamp-diffuser calibrator, which incurs the lowest cost to the on-board spectral radiometric calibration. Therefore, the model order of Eq.(7) should be determined experimentally.

3 Model order determined by experiment method

3.1 Measurement of the spectral irradiance degradation curve

Because the spectral irradiance degradation characteristics of halogen tungsten lamps are closely related to their manufacturing process, it is reasonable to believe that halogen spectral irradiance degradation characteristics of the same type of the halogen tungsten lamp can be described by the same analytic model. In other words, the model order of one type of halogen tungsten lamp can be determined from the spectral irradiance degradation curves of samples. The spectral irradiance degradation curve of the halogen tungsten lamp is the ratio of its spectral irradiances at different moments, which can be expressed by Eq.(9) whereEo(λ) andEj(λ) are the initial and the spectral irradiance after thejthaging interval, respectively.

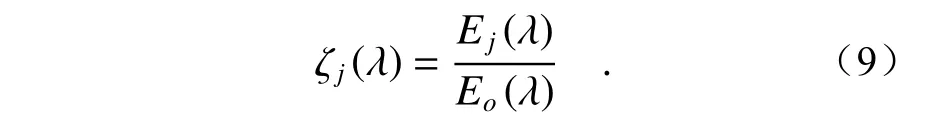
Therefore, the spectral irradiance degradation curve can be measured by the scheme shown in Fig.1, which is composed of a halogen tungsten lamp, diffuser reflection plate, and spectrometer.Two Osram 64610HLX lamps have been measured to investigate the spectral irradiance degradation model of the halogen tungsten lamp, whose rated voltage and power are 12 V and 50 V, respectively.Therefore, the operating current at which the halogen tungsten lamp operates is set to a constant 4 amperes which is the same as the operating current of the halogen tungsten lamp for on-board calibration during the test.

Fig. 1 Measurement scheme of the spectral irradiance degradation curve of the halogen tungsten lamp

Fig. 2 (a) Extra-atmospheric solar spectral irradiance and(b) spectral irradiance of tested halogen tungsten lamp

The diffuser plate was made of spectralon (one kind of PTFE supplied with Labsphere). The spectral reflectance degradation of the spectralon diffuser was caused by exposure to radiation at wavebands of 200 nm-400 nm according to J.E.Leland[13]. Fig.2 shows the spectral irradiance of the tested halogen tungsten lamp and the extra-atmospheric solar spectral irradiance calculated by MODTRAN[14], the ratio of which at wavebands of 250 nm to 400 nm was shown in Fig.3. According to Fig.3, the averaged ratio at wavebands of 250-400 nm was calculated to be about 0.86%.Moreover, the radiation of the halogen tungsten lamp at wavebands of 200-250 nm was so low that it can be neglected. Therefore, the diffuser equals to be exposure to the extra-atmospheric solar radiation for about one hour when it is irradiated by the halogen tungsten lamp for 160 hours. According to the technical guide of Labsphere, the reflectance of the diffuser decreases at wavelength of 250 nm to about 0.04%, when the irradiation is equivalent to one extra-hour of atmospheric solar irradiation. Moreover,the diffuser reflectance returned to near original values when it returned to atmospheric conditions, presumably due to oxidation and the loss of the surface contaminants that caused the discoloration[15]. Therefore, it was reasonable to infer that the reflectance degradation of the diffuser reflector was much smaller than 0.04%, which was much smaller than the degradation of the halogen tungsten lamp. That is to say that the reflectance degradation of the diffuser reflector can be neglected in researching the degradation of the halogen tungsten lamp.

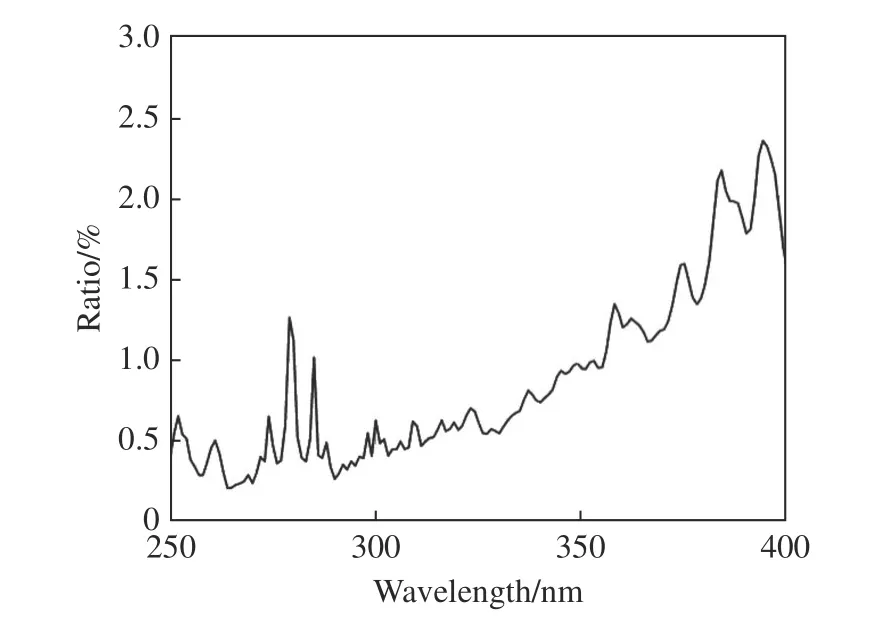
Fig. 3 Ratio of the spectral irradiance of the tested halogen tungsten lamp to the sun

The spectrometer was an HR-1024i, supplied by SVC (Spectra Vista Corporation, US), that operates at wavebands of 350-2 500 nm and has 1 024 spectral channels. The detector assembly of the HR-1024i spectrometer was composed of three linear array detectors: a 512 CCD detector for wavebands from 350-990 nm, a 256 InGaAs detector for wavebands from 990-1 900 nm and a 256 extended In-GaAs detector for wavebands from 1 900-2 500 nm. The temperature stability of the laboratory and the three detectors in the spectrometer were kept within ± 1°C and ± 0.1°C, respectively. Besides,the lamp, the spectrometer and the diffuser plate had never been moved or replaced during the 7-day measurement. Therefore, the responsivity of the HR-1024i spectrometer can be assumed to remain unchanged during the 7-day measurement.

Additionally, the dark current had been removed from the measuring results during the measurement, which means that the spectral irradiance decay curve only contains the random noise. The method to remove dark current from the measuring results was shown in the following. First, the dark current is measured by closing the shutter, and then,the reflected light from the diffuser is measured by opening the shutter. The dark current is removed by reducing the closed shutter measurement results from the final opened shutter measurement results.To make the two unused new lamps remain stable,they were aged for 20 hours in the same experimental setting before the measurement.

To sum up, the spectral irradiance degradation curve shown in Eq. (9) can be simplified as Eq.(10),whereDNo(λ) andDNj(λ) are the initial andjthoutput of the spectrometer with the dark current removed, respectively.

According to Eq.(10), the measurement uncertainty of the spectral irradiance degradation curve is simplified to its measurement repeatability, when the measurement setup is not touched and the dark current is removed during the measurement. The calculation of the measurement uncertainty is shown in chapter 3.2 in this paper.

To simulate the on-orbit operation, the lamp is turned on for one hour and then turned off for five minutes, and the above procedure is repeated 160 times during the measurement to ensure the lamp can satisfy the on-orbit calibration requirements.The measured spectral irradiance degradation curves of the two halogen tungsten lamps are shown in Fig.4 (Color online).

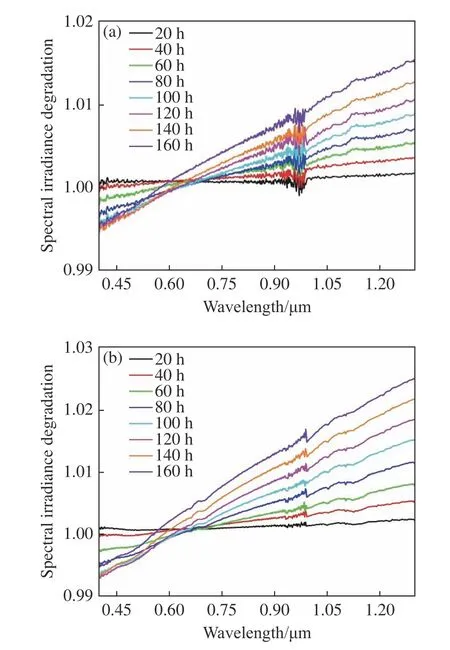
Fig. 4 Spectral irradiance degradation curves of (a) 1# and(b) 2# halogen tungsten lamps

3.2 Measurement uncertainty of the spectral irradiance degradation curve

According to Eq.(10), the measurement uncertainty of the spectral irradiance degradation curve can be expressed by Eq. (11).

Therefore, the measurement uncertainty of the spectral irradiance degradation curve is simplified to Eq. (13):

Eq.(13) shows that the uncertainty of the spectral irradiance degradation curve can be determined by the measurement repeatability ofDNthat stands for the spectral irradiance. However, the repeatability of theDNis determined by the spectral irradiance of the light source and the signal to noise ratio of the HR-1024i spectrometer, which can be calculated according to the measured spectral irradiance expressed byDN. To summarize, the measurement uncertainty of the spectral irradiance degradation curve can be calculated by the measured spectral irradiance according to Eq.(13).

The measurement uncertainty of the spectral irradiance degradation curve shown in Fig.4 was calculated and shown in Fig.5 (Color online) according to Eq.(13). The standard deviations of the measurement uncertainty of the two lamps were calculated to be approximately 0.038% according to the measurement uncertainty curves shown in Fig.5,both of which were significantly less than the spectral radiance reconstruction uncertainty of 0.3% required by the on-board lamp-diffuser calibrator.Therefore, it is reasonable to construct a spectral irradiance degradation model that satisfies the spectral radiance reconstruction uncertainty of the onboard lamp-diffuser calibrator according to the measured spectral irradiance degradation curve.

Fig. 5 Measurement uncertainty of the spectral irradiance degradation curve for (a) 1# and (b) 2# halogen tungsten lamps

3.3 Determination of the model order

The spectral irradiance degradation curves of the two lamps were fitted by Eq. (7) with the model order ranging from one to four using the leastsquares method. Fig.6 showed the measured spectral irradiance degradation curves and fitted results with the two-order model of the two halogen tungsten lamps. Because the fitting results of the models almost coincide, only the fitting results of the second-order model are shown in Fig.6 as an example. The relative standard deviations are expressed in Eq.(14):

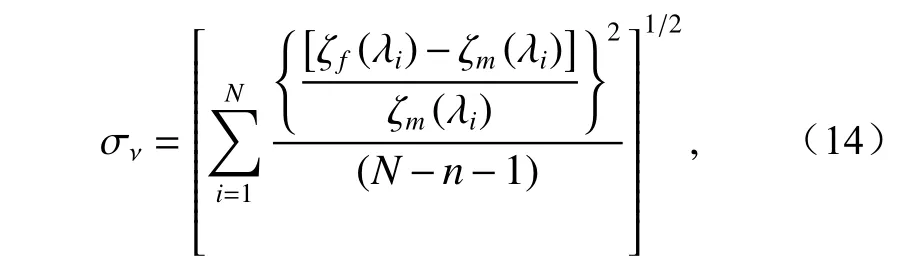
Fig. 6 Measured spectral irradiance degradation curves and fitted results with the second-order model of (a) 1#and (b) 2# halogen tungsten lamps

where ζf, ζm,Nandn+1 are the fitted data, measured data, number of measuring wavebands, and number of fitting parameters, respectively. The relative standard deviations were calculated and is shown in Fig.7 (Color online) according to Eq.(14).

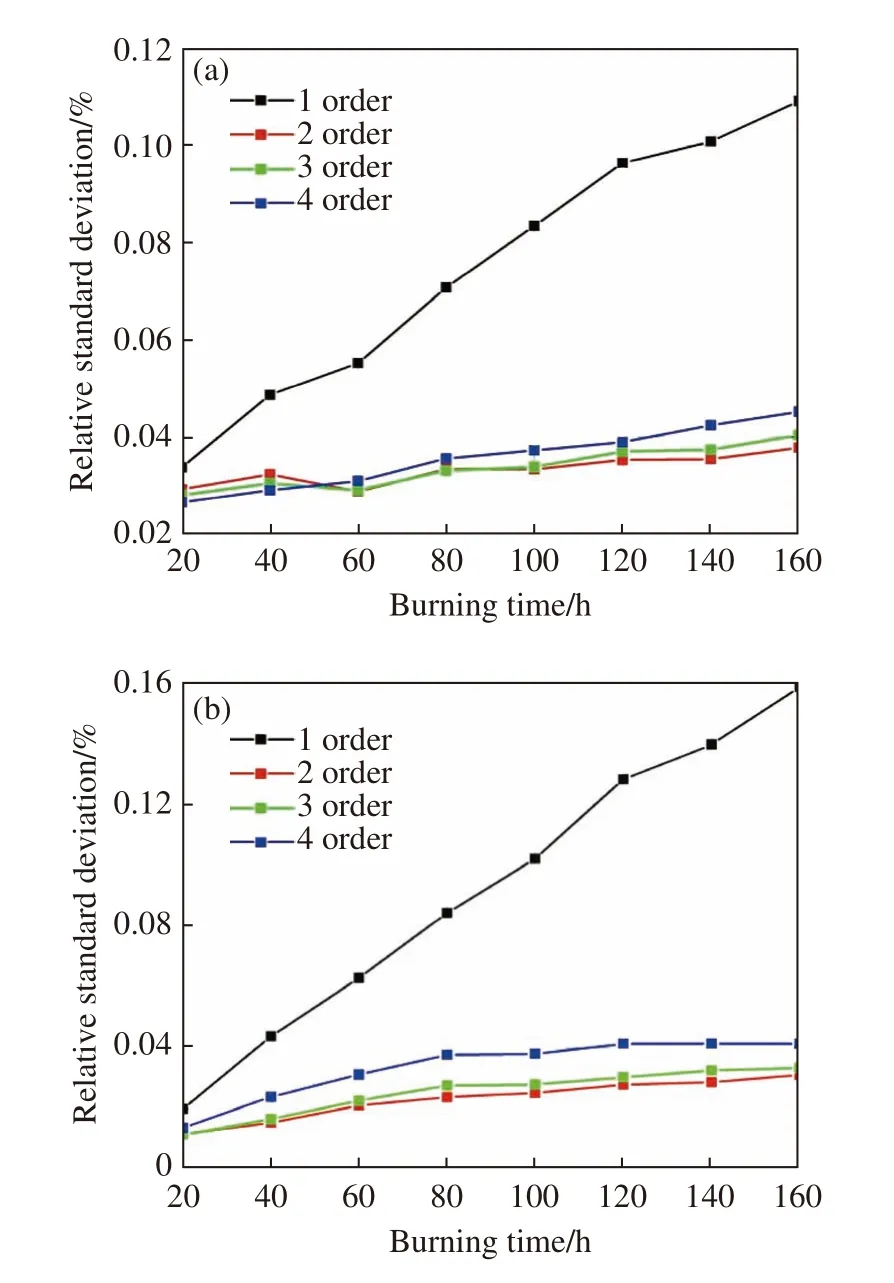
Fig. 7 Relative standard deviations of the spectral irradiance degradation curves of (a) 1# and (b) 2# halogen tungsten lamps

Fig.7(a) shows that the relative standard deviations of the first-order model increase from about 0.03% to about 0.11% and from about 0.03% to about 0.04% for the model orders from two to four during the burning time for 1# lamp. Fig.7(b) shows that the relative standard deviations of the first-order model increase from about 0.02% to about 0.16% and from about 0.02% to about 0.04% for the model orders from two to four during the burning time for 2# lamp. That is to say that the relative standard deviation of the first-order model increases much faster than the higher order model,which indicates that first-order model cannot describe the degradation characterization of the halogen tungsten lamp accurately enough during the burning life.

Moreover, Fig.7(a) and Fig.7(b) show that the relative standard deviations are better than 0.05%and very similar for the model orders from two to four during the burning time for the two lamps.Therefore, the model order of the two halogen tungsten lamps are determined to be two since it obtains the least fitting parameters in this paper.

The model precisions are estimated by relative errors between the fitted and measured spectral irradiance degradation curve. The relative errors of the two lamps are calculated to be better than 0.25% at wavebands from 400 nm to 1 300 nm as shown in Fig.8(a) (Color online) and Fig.8(b) (Color online),respectively. Therefore, the proposed spectral irradiance degradation model of halogen tungsten lamps can satisfy the spectral radiance reconstruction uncertainty of the on-board lamp-diffuser calibrator.

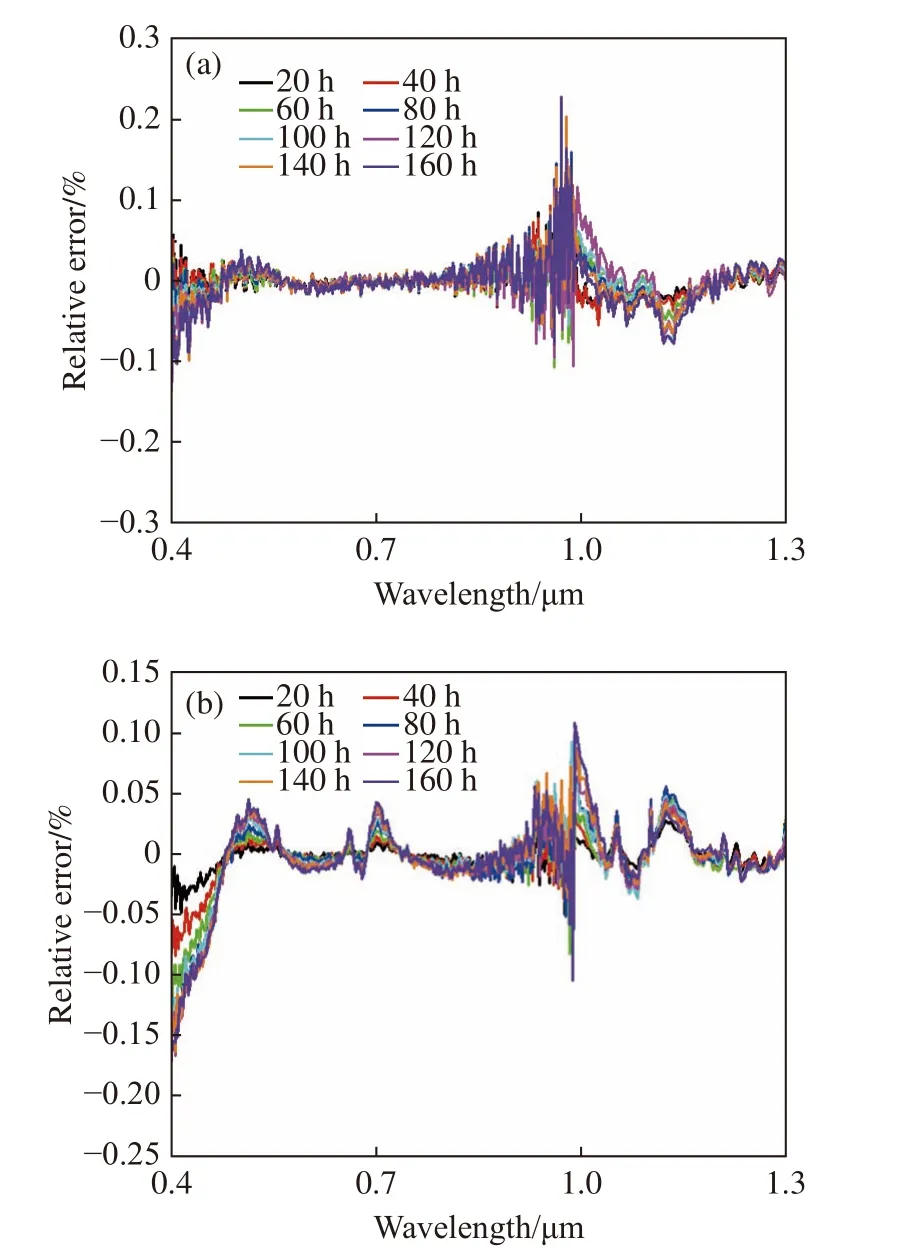
Fig. 8 Relative errors between the fitted and measured spectral irradiance degradation curve of the two halogen tungsten lamps fitted by the second-order model. (a) 1# and (b) 2# halogen tungsten lamp

Hence, the spectral irradiance degradation model of the two halogen tungsten lamps can be expressed by Eq. (15):

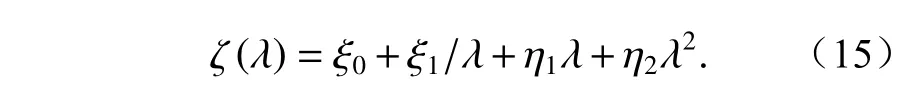
The proposed spectral irradiance degradation model may not be suitable for other types of lamps;however, the method used to construct the spectral irradiance degradation model is still valid for other types of halogen tungsten lamp.

4 Conclusions

In this paper, a hemi-empirical spectral irradiance degradation model of a halogen tungsten lamp with an undetermined model order at wavelengths from 400 nm to 1 300 nm was derived based on the blackbody radiation law and the Weierstrass theorem, and the model order was subsequently determined to be two by the proposed model-order-determination criterion. The proposed spectral irradiance degradation model may not be suitable for other types of lamps. However, the method used to construct the spectral irradiance degradation model is still valid for other types of halogen tungsten lamps.The uncertainty of the proposed model is approximately 0.25%, which satisfies the spectral radiance reconstruction uncertainty of the on-board lampdiffuser calibrator at wavelengths from 400 nm to 1 300 nm and lays a theoretical foundation to realize a measurement uncertainty of 0.3% at reflected solar wavebands. The spectral irradiance degradation model of halogen tungsten lamps at wavelengths from 1 300 nm to 2 350 nm will be researched, subsequently.

Acknowledgements

The author thanks Dr. Lei kai-chao from Chang Chun Institute of Optics, Fine Mechanics and Physics, Chinese Academy of Sciences, for his valuable suggestions and selfless help that have helped improve this manuscript substantially.