Nonlinear excitation of a geodesic acoustic mode by reversed shear Alfvén eignemodes
Yahui WANG(王亚辉),Tao WANG(王涛),2,Shizhao WEI(魏士朝) and Zhiyong QIU(仇志勇),2
1 Institute for Fusion Theory and Simulation and Department of Physics,Zhejiang University,Hangzhou 310027,People's Republic of China
2 Center for Nonlinear Plasma Science and ENEA C.R.Frascati,Frascati 65-00044,Italy
Abstract The parametric decay process of a reversed shear Alfvén eigenmeode(RSAE)into a geodesic acoustic mode and a kinetic RSAE is investigated using nonlinear gyrokinetic theory.The excitation conditions mainly require the pump RSAE amplitude to exceed a certain threshold,which could be readily satisfied in burning plasmas operated in steady-state advanced scenario.This decay process can contribute to thermal plasma heating and confinement improvement.
Keywords:reversed shear Alfvén eigenmode,geodesic acoustic mode,gyrokinetic theory,nonlinear mode coupling,burning plasmas,alpha channelling
1.Introduction
Good confinement of energetic particles(EPs),generated by fusion reaction or auxiliary heating such as neutral beam injection,is crucial to the performance of future tokamak devices such as ITER[1,2]and CFETR[3],since the EPs are needed to be sufficiently well confined to heat thermal plasmas and maintain them in optimal conditions for self-sustained fusion reactor.However,shear Alfvén wave(SAW)instabilities,including Alfvén eigenmodes[4](AEs)and EP continuum modes[5],could be resonantly excited by the free energy of the nonuniform EP distribution.As finite amplitude SAW instabilities may cause significant anomalous EP transport and performance degradation,theoretical analysis on the SAW nonlinear dynamics has been a key topic of continuous research,see[6]for a recent in-depth review.
In this work,we focus on the typical parameter regime of future burning plasmas operated at steady-state,where a significant fraction of plasma current is generated noninductively.Since the radial profile of the non-inductive(e.g.bootstrap)current generally peaks off-axis,the magnetic shear in the plasma core region is reversed in this scenario,i.e.the safety factorqprofile contains an off-axis local minimumqmin.As first identified during the advanced operation in JET[7],a specific branch of SAW,namely,the reversed shear Alfvén eigenmode(RSAE,an alternative name is Alfvén cascades)exists close to the flux surface ofqmin,and can be readily driven unstable by EPs[8-10].Considering the fact that the EP pressure gradient also peaks in the core region,one may reasonably expect that,among all the branches of the SAW instabilities,RSAEs are firstly excited as the EP pressure profile ramps up in time.Recent nonlinear simulation analyses suggest that,the core-localized RSAEs could efficiently induce convective type of EP radial transport,along with further impact on the global EP confinement property[11,12].In particular,the modulation to the EP density profile by RSAE may enhance the drive to the toroidal Alfvén eigenmodes(TAEs)[4],which generally locate in the outer-core region with finite magnetic shear[13]and are a dangerous concern for direct EP loss and damage to the plasma facing components[14].Thus,in order to predict the steady-state performance of a burning plasma device(such as ITER),it is of great importance to study the nonlinear evolution of RSAE,complementary to the research activities focusing on TAE[6].
As clarified in[12,15],the dynamics of an AE activity could be subdivided into three stages.In the ‘linear’ stage where the AE is exponentially growing,the important physics include the wave-particle linear resonance condition and forced drive of secondary modes including axisymmetric zero frequency zonal structures(ZS)[16].Wave-particle trapping takes place in the following ‘saturation’ stage[17].Note that the first two stages generally occur in extremely short timescales,which are characterized byrespectively.Here,γLis the linear AE growth rate,and is independent of the mode amplitude,is the waveparticle trapping frequency,with |δφ| being the mode amplitude.After the initial saturation stage due to wave-EP nonlinearity,wave-wave nonlinearity dominates in the longer timescale ‘saturated’ stage with a time scale ∝1/|δφ|,where the AE spectral energy could spontaneously decay into linearly stable domains[18].This nonlinear spontaneous decay process is sometimes classified into the alternative AE saturation mechanism via wave-wave nonlinear coupling[19],to be considered on the same footing with wave-EP nonlinearity.Since the RSAEs are mostly observed with quasi-static amplitudes rather than bursts,the wave-wave couplings in the‘saturated’stage have significant implication to the long timescale,global performance of fusion plasmas,where one could consider the initial state as a RSAE pump wave with a prescribed mode structure and finite amplitude for a simplified analytical study[20].Furthermore,note the lowest order RSAE dispersion relation,the wavenumber parallel to the equilibrium magnetic field,m/nthe poloidal/toroidal mode numbers,respectively,R0the major radius,vAthe Alfvén speed,and ωGthe frequency of geodesic acoustic mode(GAM,see section 3.1)[21]corresponding to geodesic curvature induced SAW continuum upshift.Consequently,the RSAE spectra are sensitive to the underlying toroidal mode numbern,and RSAEs with differentnare generally discrete eigenstates with clear frequency separations[13].Thus,besides direct coupling between RSAEs with differentngiving rise to the generation of low frequency AEs,other mechanisms usually involve the spontaneous excitation ofn=0,m≃0 ZS.Here,we have also taken into account the fact that magnetic shear vanishes aroundqmin,which makes each RSAE dominated by only one or two poloidal mode numbers[8].Indeed,it has been recently shown that a RSAE pump wave could generate both electrostatic zonal flow as well as electromagnetic zonal current via modulational instability,where the zonal current could play a potentially important role in the long timescale RSAE saturation mechanism[20].Alternatively,as we show in the present paper,if a critical amplitude threshold is exceeded,a RSAE pump wave could parametrically decay into a finite frequency GAM and a lower sideband kinetic RSAE(KRSAE).Analogous process for TAE has been recently published in[22,23],which suggests the important role played by the thermal ion βivalue.Here,β is ratio between kinetic and magnetic pressures.We consider the high βilimit in this work,so the GAM frequency is larger than the difference between pump RSAE frequency and continuum accumulation point frequency,consistent with the parameter regime of future burning plasmas as mentioned above.Here,∊0is the inverse aspect ratio.
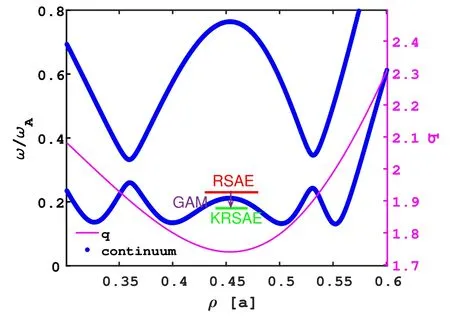
Figure 1.Cartoon of the pump RSAE decay into a GAM and a KRSAE in the high βi limit.
The physics mechanism presented in this work could have significant implication to the operation of burning plasma devices.On one hand,the GAM generated in this process,as a finite frequency branch of electrostatic ZS,could regulate drift wave turbulence[24]and improve plasma confinement.On the other hand,the GAM mainly suffers from thermal ion Landau damping in the plasma core region[25],and thus,it provides a viable route to alpha channelling[26].In fact,the present work is consistent with the recent observation of high thermal ion temperature and improved confinement regime in JET with the presence of ~MeV EPs and rich RSAE activity[27].Thus,we could reasonably expect this mechanism to be at work in future burning plasmas.
The rest of the paper is organized as follows.In section 2,the basic equations of the theoretical model are given.In section 3,the parametric decay process of the pump RSAE into a GAM and a KRSAE is investigated.Finally,conclusions and discussions are given in section 4.
2.Theoretical model
We investigate the process of a pump RSAE(Ω0≡(ω0,k0))decaying into a GAM(ΩG≡(ωG,kG))and a KRSAE(ΩK≡(ωK,kK))with the same toroidal and poloidal mode numbers as the pump RSAE in high βilimit,as shown in figure 1.For simplicity of discussion while without loss of generality,we considered the case with RSAE frequency above the local maximum of SAW continuum,which corresponds tonqmin-m<0,as discussed in[8].Extension of the present analysis to more general cases is straightforward.
For the present analysis of nonlinear interactions between RSAE and GAM,the scalar potential δφ and parallel component of vector potential δA‖are adopted as the field variables,while the parallel magnetic perturbation is negligible in consistency with the typical low thermal to magnetic pressure ratio.Furthermore,for convenience,δψ ≡ωδA‖/(ck‖)is taken as an alternative field variable for RSAE and KRSAE,where the ideal MHD limit can be achieved by taking δψ=δφ.The scalar potential perturbation contains δφ=δφ0+δφG+δφK,with the subscript 0,G and K denoting the pump RSAE,GAM and KRSAE,respectively.The frequency and wavenumber matching conditions for the resonant decay are adopted as Ω0=ΩG+ΩK.Considering single-nRSAE/KRSAE with one dominant poloidal modem,the perturbations of RSAE and KRSAE in straight-field-line(r,ϑ,φ)coordinates can be expressed as



3.Parametric decay dispersion relation
3.1.Nonlinear GAM equation

3.2.Nonlinear KRSAE equation

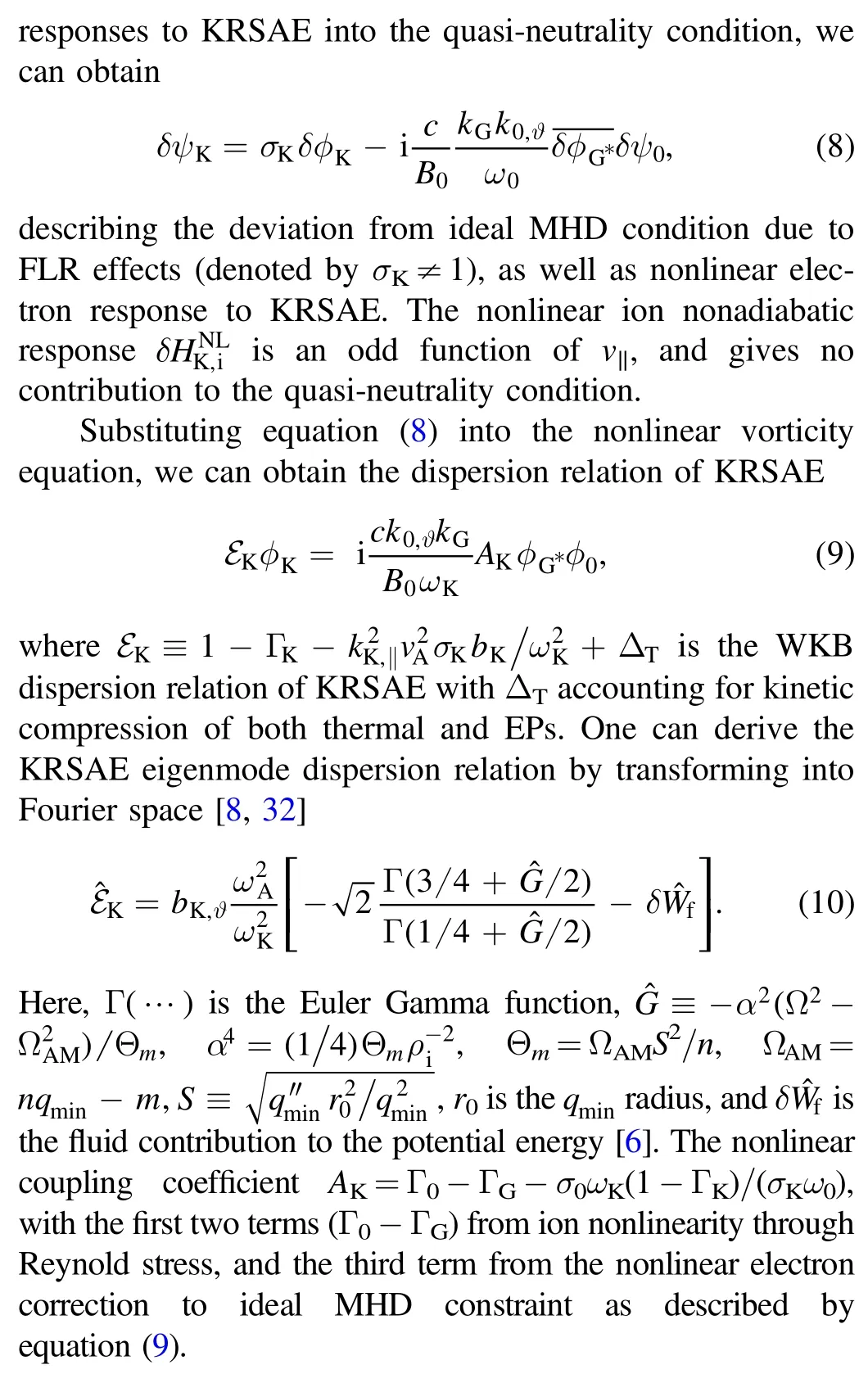
3.3.Nonlinear parametric dispersion relation



4.Conclusions and discussions
In this work,using nonlinear gyrokinetic theory,we have investigated the nonlinear decay mechanism of a pump RSAE into a GAM and a KRSAE with the same toroidal and poloidal mode numbers as the pump RSAE.This channel of RSAE spectral energy transfer is important for future fusion reactors due to the core localized fusion alpha particle distribution,which may preferentially drive RSAEs in the advanced reversed shear scenarios.The investigated parametric decay process can take place due to the dense KRSAE spectrum,which makes the frequency and wavenumber matching conditions for the parametric decay process possible.
For the process to be prevalent in fusion reactors,the threshold condition of the parametric instability isi.e.the pump RSAE drive should overcome the threshold due to KRSAE and GAM dissipation to drive the parametric decay instability,and the threshold of pump RSAE amplitude can be estimated asIn addition,the GAM and KRSAE are required to be weakly damped normal modes,and the GAM frequency should be larger than the difference between the pump RSAE frequency and the continuum accumulation point frequency,which is determined by the underlying equilibrium profiles as well as the non-perturbative EP effects[13].The corresponding criteria are estimated as,respectively,andwhich are generally satisfied in burning plasma scenarios.
Note that other mode coupling processes are also possible in fusion plasmas,e.g.a RSAE pump wave could generate zero frequency ZF via modulational instability,as investigated in[20].The relative importance of these processes in a realistic scenario requires further analyses,before quantitative estimation of RSAE saturation level and the related heating/transport can be made.However,the investigated parametric decay process can provide a novel mechanism of alpha channelling[26]that may effectively heat core thermal plasmas in the future fusion device.The pump RSAE,excited by alpha particles,transfers energy to a GAM and a KRSAE through the parametric decay process.KRSAE and GAM mainly lose energy to electrons and ions,respectively[23],leading to core localized plasma heating.The heating rate can be obtained by estimating the saturation level of KRSAE and GAM,from the fixed point solution of the coupled nonlinear equations,e.g.equations(45)-(47)of[23],and will be investigated in a separated publication.On the other hand,the nonlinearly generated GAM as a finite frequency component of zonal flow[21],can regulate other types of drift waves,including drift Alfvén waves and the associated anomalous transport,by scattering drift waves into short wavelength stable region[35],leading to cross-scale couplings[36]and improvement of the thermal plasma confinement.
As a final remark,in the parameter regimes where the KRSAE or GAM is heavily damped and becomes a virtual mode,the parametric decay instability can still occur and becomes a non-resonant decay process(also called the nonlinear Landau damping in some literatures[37]),with the decay being a result of the corresponding Landau damping of the sideband.This process can be of relevance noting that GAM could be heavily damped[25]in the center of the tokamak with relatively smallq,where RSAE tends to be localized.Extension of the present analysis to the non-resonant decay process is straightforward.
Acknowledgments
This work is supported by the National Key R&D Program of China(No.2017YFE0301900),National Natural Science Foundation of China(No.11 875 233),and Users of Excellence Program of Hefei Science Center CAS(No.2021HSC-UE016).
——王涛
 Plasma Science and Technology2022年2期
Plasma Science and Technology2022年2期
- Plasma Science and Technology的其它文章
- Application of local Monte Carlo method in neutronics calculation of EAST radial neutron camera
- Effect of gas pressure on ion energy at substrate side of Ag target radio-frequency and very-high-frequency magnetron sputtering discharge
- Numerical simulation of hydrogen arcjet thruster with coupled sheath model
- An electromagnetic wave attenuation superposition structure for thin-layer plasma
- Plasma-assisted ammonia synthesis in a packed-bed dielectric barrier discharge reactor:roles of dielectric constant and thermal conductivity of packing materials
- A fast convergence fourth-order Vlasov model for Hall thruster ionization oscillation analyses
