改进的软硬阈值法及其在地震数据降噪中的研究
刘剑 秦飞龙 成亚丽

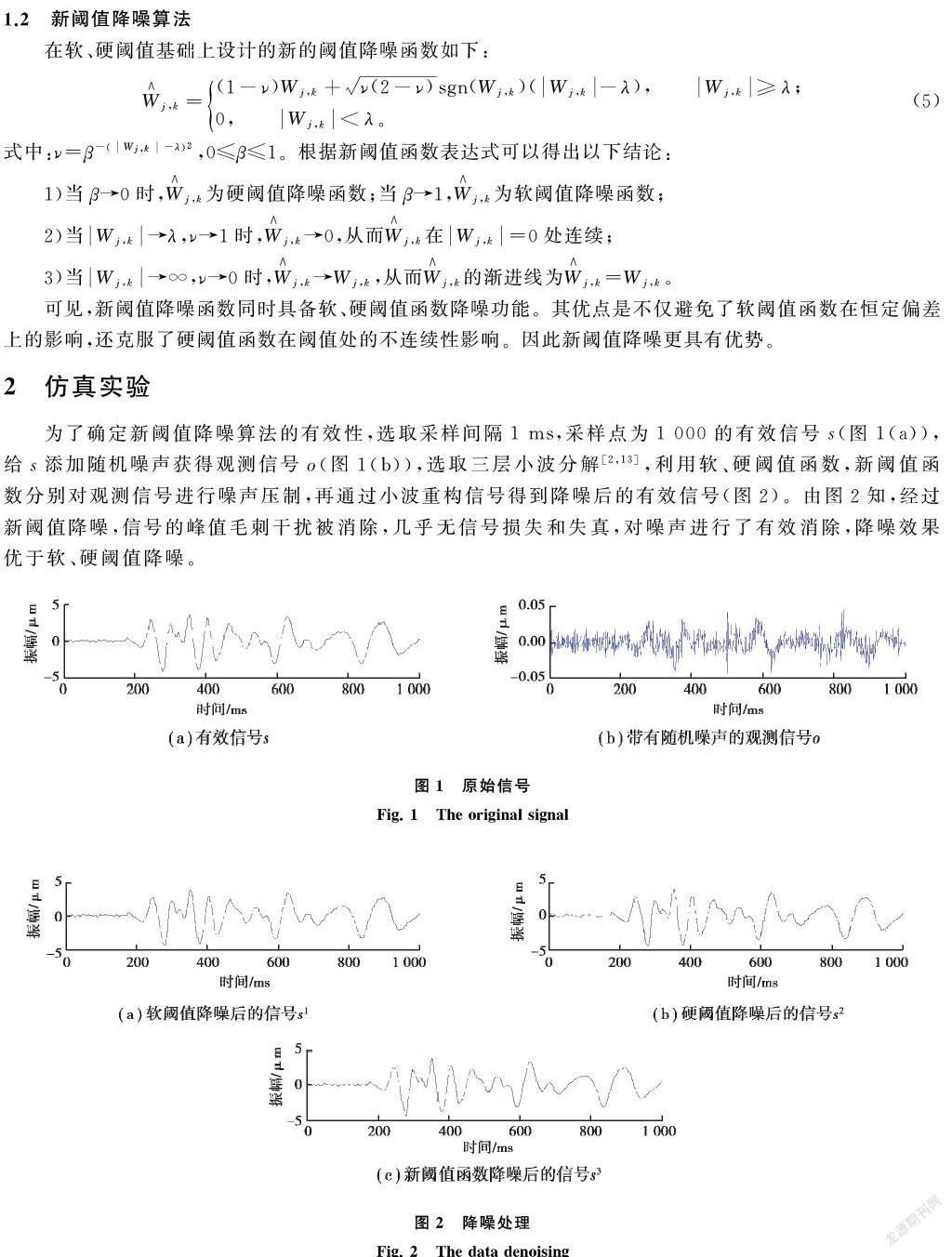


摘要:采集的野外地震数据伴随有随机噪声干扰,需要将其消除。软硬阈值法能够压制地震数据的噪声信号,但是降噪效果并不理想。因此,提出了一种改进的软硬阈值算法用于地震数据降噪。首先利用软硬阈值法原理构建了一种新的阈值降噪法,并对新算法相关特性进行了研究,通过仿真实验确定了新阈值算法的小波基为sym 3,利用均方差和信噪比对新阈值降噪法的降噪效果进行了评价。最后,将新阈值降噪法用于实际地震数据降噪,结果发现新阈值降噪法能够去除地震数据中的随机噪声,降噪效果较软硬阈值法更理想。
关键词:地震数据;小波变换;软硬阈值法;新阈值法;降噪
中图分类号:P631.443
文献标志码:A文章编号:1000-582X(2022)02-052-06
Abstract: The collected field seismic data are accompanied by random noise interference, which needs to be eliminated by wavelet threshold denoising method. However, the soft and hard thresholding methods have some shortcomings in noise reduction. In this paper, a new threshold denoising algorithm is proposed to perform seismic data denoising. Firstly, a new threshold denoising method is designed based on the principle of soft and hard threshold method, and the relevant characteristics of the new algorithm are studied. Then, the wavelet base of the new threshold algorithm is defined as sym 3, and the denoising effect of the new method is evaluated by root mean square error (RMSE) and signal-to-noise ratio (SNR) through simulation experiments. Finally, the new threshold denoising method is applied to actual seismic data denoising. The results show that the improved threshold denoising method can remove random interference in seismic data, and the denoising effect is better than that of the old soft and hard threshold method.
Keywords: seismic data; wavelet transform; soft and hard threshold method; new threshold method; denoising
地质勘查能够给社會带来重要的能源资源。然而,在野外地质勘查中,各种不可预知的因素给采集到的地震数据带来随机噪声干扰,使地震剖面分辨率不高,不利于地震数据解释,因而需要对地震数据的随机噪声进行消除[1]。为了去除随机噪声,学者们根据随机噪声与有效信号的差异,提出了一些随机噪声消除方法,如K-L变换、傅里叶变换、倾斜叠加法等。这些降噪方法需要数据的一些先验条件,但实际上地震数据受到的人为影响、机器影响、地质环境影响等干扰是无法预知的。因此,这些算法降噪效果不佳[2]。近年来,盲源分离[3]中的ICA算法[4]、FASTICA算法[5]、JADE算法[6]等也广泛应用于地震数据降噪,但是盲源分离方法要求各个信号是相互独立而且高斯信号最多为一个,彼此之间的混合不复杂[2]。地震数据埋藏较深,受到干扰影响严重,各种数据信息并非线性混合,利用盲源分离进行地震数据降噪存在缺陷。在随机噪声降噪中,小波降噪具有一定的处理优势,因为小波变换不仅具有良好的时频局部变换特征,还具有“时间频率”窗口自适应特征,号称信号降噪的“显微镜”[7]。小波变换降噪主要通过小波分解把地震数据分解到低频和高频不同尺度空间上,通过选取合理阈值方法对各尺度上的小波系数进行噪声压制,然后利用小波重构变换恢复原始信号[8]。在阈值处理中,应用最广泛的是Donoho软硬阈值降噪算法[9],然而该类阈值算法由于算法特性使降噪具有一定的缺陷性。软阈值降噪算法通过恒定的方式压缩小波系数,会丢失某些有效的高频信号;硬阈值降噪算法处理后的小波系数在阈值处不连续,给重构信号带来振荡,降噪后的信号不光滑。因此直接利用软硬阈值降噪效果不理想[10]。但是软阈值法处理后的小波系数具有连续性,硬阈值能够避免软阈值法以恒定的方式压缩小波系数的影响[10]。因此,笔者在软硬阈值降噪原理基础上,结合其降噪的优点,设计了一种改进的软硬阈值降噪方法,以弥补软硬阈值降噪的不足,提高地震数据降噪效果。
1 新阈值降噪算法
1.1 算法基础
3 实际地震数据降噪处理
将提出的新阈值函数用于实际地震数据降噪处理,数据来自中国地质调查局计划项目(1212010916040),数据样本采样间隔道号为1,采样点数为6 000,采样间隔为1 ms。为了便于显示,截取其中的1~111道地震信号数据进行降噪处理(图4(a))。由图4(a)知,原始地震数据剖面被随机噪声干扰严重,分辨率低,看不出地震数据形态,不利后期地质解释。因此,选取小波基为sym 3,3层小波分解。利用小波变换中的软、硬阈值函数对原始地震数据进行降噪,降噪后的信号分别如图4(b)(c)所示。由图4(b)(c)知,软、硬阈值函数能够去除地震数据大部分干扰噪声,地震分辨率有所改善,然而降噪效果不理想,仍然存在大量噪声影响地震剖面,分辨不高。利用所提出的新阈值函数对原始地震数据进行降噪处理,降噪后的信号结果如图4(d)所示。由图4(d)知,整个降噪后的地震剖面数据双曲线特征明显,纹理清晰,分辨率高,几乎所有干扰噪声均被移除,表明新阈值函数降噪效果比软、硬阈值函数降噪效果更理想。
4 结 论
在软、硬阈值函数降噪基础上提出了一种新阈值降噪函数,结论如下:
1)新的阈值降噪函数同时具备软、硬阈值降噪函数功能,其优点是避免了软阈值函数在恒定偏差上的影响,也避免了硬阈值函数在阈值处的不连续性影响;
2)通过仿真实验确定了新阈值函数的小波基为sym 3,信噪比(SNR)和均方根误差(RMSE)结果表明新阈值函数降噪效果更好;
3)在实际数据降噪处理中,新阈值函数能够去除地震数据的各类干扰噪声,降噪后的地震剖面分辨率高,对比软、硬阈值函数结果表明新阈值函数降噪更有效。
参考文献:
[1] 秦飞龙. 二维盲小波算法及其在金属地震数据降噪处理中的研究[D]. 成都: 成都理工大学, 2013.
Qin F L. 2D blind-wavelet algorithm and its application in the processing of de-noising on metal seismic data[D]. Chengdu: Chengdu University of Technology, 2013. (in Chinese)
[2] Qin F L, Liu J, Yan W Y. The improved ICA algorithm and its application in the seismic data denoising[J]. Journal of Chongqing University English Edition, 2018, 17(4): 162-170.
[3] 刁瑞, 吴国忱, 尚新民, 等. 地面阵列式微地震数据盲源分离去噪方法[J]. 物探与化探, 2017, 41(3): 521-526.
Diao R, Wu G C, Shang X M, et al. The blind separation denoising method for surface array micro-seismic data[J]. Geophysical and Geochemical Exploration, 2017, 41(3): 521-526. (in Chinese)
[4] Safont G, Salazar A, Vergara L, et al. Nonlinear estimators from ICA mixture models[J]. Signal Processing, 2019, 155: 281-286.
[5] Jiang S C, Lin P, Chen Y M, et al. Mixed-signal extraction and recognition of wind turbine blade multiple-area damage based on improved Fast-ICA[J]. Optik, 2019, 179: 1152-1159.
[6] Piotrowski A P, Napiorkowski J J. Step-by-step improvement of JADE and SHADE-based algorithms: success or failure?[J]. Swarm and Evolutionary Computation, 2018, 43: 88-108.
[7] 程鲁, 秦飞龙, 张津, 等. 改进的Mallat算法在金属地震数据降噪处理中的应用[J]. 桂林理工大学学报, 2017, 37(4): 602-607.
Cheng L, Qin F L, Zhang J, et al. Application of improved Mallat algorithm in noise reduction processing of metal seismic data[J]. Journal of Guilin University of Technology, 2017, 37(4): 602-607. (in Chinese)
[8] 宋立业, 周乐, 刘昕明. 基于邻域相关性多阈值新函数寻优法的小波降噪分析[J]. 信息与控制, 2019, 48(1): 57-64.
Song L Y, Zhou L, Liu X M. Wavelet denoising analysis based on an optimization method of multi-threshold new function with neighborhood correlation[J]. Information and Control, 2019, 48(1): 57-64. (in Chinese)
[9] Donoho D L, Johnstone I M. Ideal spatial adaptation by wavelet shrinkage[J]. Biometrika, 1994, 81(3): 425-455.
[10] 刘书俊, 李生林, 蒋明, 等. 一种基于加权平均的改進型小波阈值降噪算法[J]. 化工自动化及仪表, 2017, 44(3): 239-242, 318.
Liu S J, Li S L, Jiang M, et al. An improved wavelet threshold de-noising algorithm based on weighted average method[J]. Control and Instruments in Chemical Industry, 2017, 44(3): 239-242, 318. (in Chinese)
[11] Wang G X, Chen L, Guo S, et al. Application of a new wavelet threshold method in unconventional oil and gas reservoir seismic data denoising[J]. Mathematical Problems in Engineering, 2015, 2015: 1-7.
[12] Bayer F M, Kozakevicius A J, Cintra R J. An iterative wavelet threshold for signal denoising[J]. Signal Processing, 2019, 162: 10-20.
[13] Cotronei M, Rossini M, Sauer T, et al. Filters for anisotropic wavelet decompositions[J]. Journal of Computational and Applied Mathematics, 2019, 349: 316-330.
[14] 成枢, 马卫骄, 牛英杰, 等. 融合加权平均的改进小波阈值函数的测量数据处理[J]. 中国科技论文, 2018, 13(15): 1811-1816.
Cheng S, Ma W J, Niu Y J, et al. Measurement data processing of improved wavelet threshold function with weighted average[J]. China Sciencepaper, 2018, 13(15): 1811-1816. (in Chinese)
[15] 周仲礼, 秦飞龙, 夏欢欢, 等. 盲小波算法在金属矿床地震资料去噪处理中的应用[J]. 成都理工大學学报(自然科学版), 2013, 40(2): 120-124.
Zhou Z L, Qin F L, Xia H H, et al. Application of blind wavelet algorithm to de-noising of the metallic ore deposit seismic data[J]. Journal of Chengdu University of Technology(Science & Technology Edition), 2013, 40(2): 120-124. (in Chinese)
(编辑 罗 敏)

