Notch fatigue of Cu50Zr50 metallic glasses under cyclic loading:molecular dynamics simulations

Yong Yang,Hairui Li,Zailin Yang,∗,Jin Liu,Evans Kabutey Kateye and Jianwei Zhao

1 College of Aerospace and Civil Engineering,Harbin Engineering University,Harbin 150001,China

2 Key Laboratory of Advanced Material of Ship and Mechanics,Ministry of Industry and Information Technology,Harbin Engineering University,Harbin 150001,China

3 College of Materials and Textile Engineering,Jiaxing University,Jiaxing 314000,China

Abstract Molecular dynamics simulation is performed to simulate the tension–compression fatigue of notched metallic glasses(MGs),and the notch effect of MGs is explored.The notches will accelerate the accumulation of shear transition zones,leading to faster shear banding around the notches’root causing it to undergo severe plastic deformation.Furthermore,a qualitative investigation of the notched MGs demonstrates that fatigue life gradually becomes shorter with the increase in sharpness until it reaches a critical scale.The fatigue performance of blunt notches is stronger than that of sharp notches.Making the notches blunter can improve the fatigue life of MGs.

Keywords:metallic glasses,notches,fatigue life,molecular dynamics simulations

1.Introduction

In the field of materials science,research in notches is always a hot topic.It is important for the safety and reliability design of precision structural components[1–3].Metallic glasses(MGs)are widely used in microelectronic systems due to their high strength,high hardness,and good forming ability[4,5].However,in the manufacture and application of engineering materials,mechanical damage,corrosion,and other factors can cause notches and cracks[6].Therefore,exploring the notch effect of MGs has become a top priority[7–9].Numerous experiments and simulations have been performed to analyze the ductility and notch insensitivity of MGs.For example,Jang et al reported separate and distinct critical sizes for maximum strength and the brittle-to-ductile transition,thereby demonstrating that strength and ability to carry plasticity are decoupled at the nanoscale[10].Qu et al found that the tensile strength of the studied bulk MGs(BMGs)is insensitive to notches and much better than that of conventional brittle materials.Moreover,it might be possible to toughen BMGs by introducing artificial defects[11].Sha et al considered that failure mode and strength in notched MGs critically depend on the notch depth and notch sharpness[12].Pan et al reported that the anomalous inverse notch effect is caused by a transition in the failure mechanism from shear banding at the notch tip to the cavitation and the void coalescence[13,14].

At present,fruitful research results have been achieved in improving ductility.However,over 90 percent of failures are due to fatigue in practical applications[15].It is particularly important to study the fatigue properties of MGs.The failure of notched MGs is accompanied by the initiation,propagation,and arrest of the shear band(SB).The time scale and length involved in fatigue failure are small,and it is difficult to observe the deformation process in the experiment.For instance,the critical scale of the SB is about 10nm[16].Conversely,the research scope of molecular dynamics(MD)simulations can completely solve the scale problem,and can also characterize the microstructure and deformation mechanism of materials[17].

In this work,the MD method is used to investigate the fatigue response of notchedCu50Zr50MGs under tensile–compression fatigue experiments.The fatigue failure mechanism of MGs at the atomic level is analyzed and the factors affecting fatigue life are summarized.The fatigue life of MGs has a quantitative relationship with the notch sharpness.According to the fatigue response and deformation process analysis of the notched MGs under cyclic loading,the aggregation rate of the shear transition zones(STZs)is the key to determining the fatigue performance.The sample of sharp notches has a large stress concentration,which increases the growth rate of STZs,accelerates the amplitude of atomic energy changes,and shortens the fatigue life.

2.Atomistic simulations

The Large-Scale Atomic/Molecular Massive Parallel Simulator(LAMMPS)is a commonly used method for MD simulation and is often used to describe multi-scale,largescale atomic structures and mechanical properties[18,19].A small cube containing 10,000 Cu atoms is established,and the corresponding number of Cu atoms is replaced with Zr atoms in combination with the random atomic replacement,forming the initial configuration ofCu50Zr50.The interaction between atoms is described by the embedded atom method potential function[20]:

where F is called the embedding energy,which is a function of the electron density ρ,φ is a pair-potential interaction,α and β are the element types of atoms i and j.

Periodic boundary conditions are applied in all directions of the initial configuration,reducing it from 2000 K to 50 K at a cooling rate of 1011K/s[21,22].A preliminary thin film sample with a size of28 ×56 ×5.6 nm3and containing 548,000 atoms is obtained by periodic replication in the X-,Y-,and Z-directions at the corresponding proportion.After annealing the sample at 800 K for 0.5 ns[23,24],the temperature is broughtback to 50 K at the same cooling rate,and then a second relaxation is performed to eliminate the effects of multiple replications and temperature fluctuations.After the sample is constructed,a strain rate of109s-1is applied to the Y-direction for loading.The boundary conditions are reset to free boundary conditions in the X-direction and periodic boundary conditions in the Y-and Z-directions.To quantify the plastic deformation of MGs and observe the change in the notches,the color of the atom is specified according to the atomic local shear strainηMises.

whereηij(i,j=x,y,z)are the components of the Lagrangian strain matrix for the specific atoms[25,26].

3.Results and discussion

To explore the effect of notches on the fatigue performance of MGs,fatigue tests are performed on samples with different notch sharpness,and the relationship between notch sharpness,stress concentration,and fatigue life is analyzed.The constructed notched MGs sample is shown in figure 1(a).The notch radian θ is calculated as

and the main features of the notched sample are the symmetrical notch radius R and notch depth D.To eliminate the impact of notch depth on the simulation results,a constant valueD=2 nm is set.Uniaxial tensile loading is carried out on the samples with different sharpness,and an applied strain of 5.4%is employed in the fatigue tests,where the maximum stress is 96% of the ultimate tensile strength(UTS).

Tensile–compression cyclic loading is performed on samples with different sharpness.Figure 2 shows the stress versus cycle numbers curve of each sample(0 degrees,20 degrees,40 degrees,80 degrees).From each curve,it is found that after certain fatigue cycles the stress decreased obviously.After a few more cycles,the stress dropped to a stable value,i.e.at the blue arrow.The comparison results of the curves show that the stress drop position of the sample with small sharpness lags significantly.To elaborate on the curve changes,a series of snapshots of the deformation process of each sample is obtained by monitoring the atomic local shear strain during cyclic loading.The atomic local shear strain is characterized using the corresponding color[17,27].Figure 3 shows the process of the atomic structure of the unnotched sample.Shear banding is divided into four stages.A region with a large local atomic shear strain indicates a high density of STZs.In the SB initiation,the STZ density is relatively low.As the cycle numbers increase,the STZs gradually aggregate and reach a critical size.The STZs reaching the critical size inspire the SB,which propagates along the Y-direction at 45 degrees.After the SB crosses through the entire sample,it gradually thickens.The SB initiation stage of the notched sample gradually becomes shorter as the sharpness increases.Combining the stress curve change and deformation process,the stress drop corresponds to the rapid localization of plastic strain,while the SB formation corresponds to the failure of the sample.As in Sha’s simulation,the fatigue life of MGs is mostly concentrated in the SB initiation[28].

Figure 1.(a)Structuralrepresentation of the Cu50Zr50 notched MGs sample,with notch depth D=2 nm,notch radius R,and notch radian θ.(b)The fatigue test with a 5.4% maximum strain corresponding to the 96% UTS.

Figure 2.The stress versus cycle number plots for the fatigue tests with an applied strain of 5.4%,where the blue arrow corresponds to the cycle numbers of SB formation:(a)unnotched;(b)θ=20°;(c)θ=40°;(d)θ=80°.

Figure 3.A series of snapshots are captured by monitoring the deformation process withηMises:(a)unnotched;(b)θ=20°;(c)θ=40°;(d)θ=8°0.

Figure 4.The notch radian versus fatigue cycle number;the red dotted line is a fitting curve.

A series of notched samples with different radians are simulated,the failure cycles are statistically analyzed,and the curve fitting is performed for the obtained data.Figure 4 shows the fatigue life versus notch radian curve;the fatigue life of the unnotched sample is 20 cycles,while the fatigue life is correspondingly shorter with the increase in radians.When the radian exceeds 40 degrees,the fatigue life is maintained at six cycles.By combining with the atomic structure snapshot of each notched sample during deformation,the phenomenon of fatigue life reduction can be clarified.The notches accelerate the aggregation rate of STZs and,as the notch sharpness increases,the faster the aggregation rate.According to Nakai’s experimental results[29,30],notches in the material will cause stress concentration,and the notch sharpness affects the degree of stress concentration.In the simulation process,the stress concentration at the root of the sharp notch is large,resulting in a faster STZ aggregation rate,and faster formation and propagation of the SB.The fatigue life gradually becomes shorter with the increase in sharpness until it reaches a critical scale.

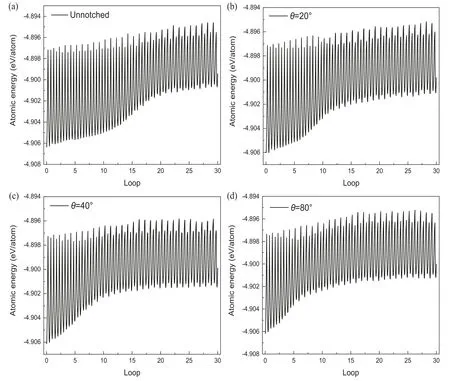
Figure 5.Atomic energy versus fatigue cycle number during fatigue tests:(a)unnotched;(b)θ=20°;(c)θ=40°;(d)θ=80°.

Figure 6.(a)The proportion of STZs versus fatigue cycle numbers;(b)the STZ’s growth rate versus fatigue cycle numbers.

Figure 5 is the atomic energy change in each notched MG sample.As the sharpness increases,the linear elastic phase gradually becomes shorter.When the radian exceeds 40 degrees,the linear elastic phase disappears.The large stress gradient causes STZs to accumulate faster,the SB forms faster to withstand plastic deformation and,at the same time,the energy storage capacity of the notched sample drops faster.There is also significant STZ activity in the thickening stage of the SB,which is mainly because the stress required to form the SB is much larger than that continuing to propagate the SB.It is manifested as the thickening of the SB and the energy circulation within a certain amplitude at this stage.

From the analysis and summary of the fatigue mechanism of the notched MGs,it is believed that the fatigue life is related to the aggregation rate of STZs.The STZs are formed by the aggregation of atoms with the large local atomic shear strain(ηMises>0.2).Statistics and analysis of the changes in the proportion of these atoms will help one to understand the intrinsic mechanism of the MGs’failure behavior.The proportion of the large shear strain atoms under each cycle is recorded,and the STZs of each sample are shown in figure 6(a).The STZ’s growth rate is obtained by taking the first-order derivative of each curve,as shown in figure 6(b).

From figure 6,it is believed that the change in the content of STZs is related to the stress concentration at the notch root.The STZs show an S-shaped growth trend,andthe differences among the samples are mainly concentrated in the SB initiation stage.From the growth rate curves of the STZs,the overall trend is growth first and then it declines.Before the peak,it is the SB initiation stage,and the sample with greater sharpness has a more obvious stress concentration,resulting in a faster STZ aggregation rate.After the peak,the SB is completely formed,the stress concentration at the notch root disappears,and the growth rate of STZs is almost the same and gradually decreases.The position of each peak point corresponds to the SB propagation stage,and the growth rate of STZs is the highest at this moment.Obvious stratification can be observed from the curve.The STZ fraction of the unnotched sample has the slowest growth rate.When the notch radian exceeds 40 degrees,this indicates that fatigue life has reached the critical value at this moment.The fatigue life of notched MGs can be predicted by the STZs’growth rate curves.

4.Conclusions

Using MD simulation,cyclic responses of notched MGs under tension–compression fatigue have been investigated,and the fatigue failure mechanism of the notched MGs has been explained.Considering the impact of the notch radian on fatigue performance,several important conclusions are as follows:

(i)According to the fatigue response of the notch radian,as the notch radian gradually increases,the fatigue life becomes shorter.When the radian exceeds 40 degrees,the fatigue life of the notched sample is maintained at six cycles.From the comparison of multiple samples,the fatigue life of notched MGs can be predicted.

(ii)The fatigue life of the blunt notched MGs is longer than that of the sharp notched MGs.The stress concentration at the root of the sharp notch is strong,which induces the faster aggregation of STZs,leads to the SB initiation,SB formation,and SB propagation,and reduces the fatigue life of MGs.Conversely,the SB formed by the blunt notch root is stable,the plastic zone of the sample is large,and the blunt notch enhances the fatigue resistance.

(iii)With the effect of cyclic stress on the notch root,the SB is formed around it to undergo plastic deformation.The formation and propagation of the SB reduce the energy storage capacity of structures,and the energy storage capacity of sharp notch samples decreases faster.The SB propagation is inhibited at the later stage,which results in the thickening of the SB.

Acknowledgments

The work is supported by the Key Laboratory of Yarn Materials Forming and Composite Processing Technology,Zhejiang Province(No.MTC2019-01),the Fundamental Research Funds for the Central Universities(No.3072020CF0202)and the Program for Innovative Research Team in China Earthquake Administration.