Simulating the Turbulent Hydrothermal Behavior of Oil/MWCNT Nanofluid in a Solar Channel Heat Exchanger Equipped with Vortex Generators

Rachid Maouedj,Younes Menni,Mustafa Inc,Yu-Ming Chu,Houari Ameur and Giulio Lorenzini

1Unit´e de Recherche en Energies Renouvelables en Milieu Saharien,URERMS,Centre de D´eveloppement des Energies Renouvelables,CDER,Adrar,01000,Algeria

2Department of Physics,Unit of Research on Materials and Renewable Energies,Faculty of Sciences,Abou Bekr Belkaid University,Tlemcen,13000,Algeria

3Department of Mathematics,Science Faculty,Firat University,Elazig,23119,Turkey

4Department of Medical Research,China Medical University Hospital,China Medical University,Taichung,40402,Taiwan

5Department of Mathematics,Huzhou University,Huzhou,313000,China

6Hunan Provincial Key Laboratory of Mathematical Modeling and Analysis in Engineering,Changsha University of Science &Technology,Changsha,410114,China

7Department of Technology,University Centre of Naama,Naama,45000,Algeria

8Department of Engineering and Architecture,University of Parma,Parco Area delle Scienze 181/A,Parma,43124,Italy

ABSTRACT Re-engineering the channel heat exchangers(CHEs)is the goal of many recent studies,due to their great importance in the scope of energy transport in various industrial and environmental fields.Changing the internal geometry of the CHEs by using extended surfaces,i.e.,VGs(vortex generators),is the most common technique to enhance the efficiency of heat exchangers.This work aims to develop a new design of solar collectors to improve the overall energy efficiency.The study presents a new channel design by introducing VGs.The FVM(finite volume method)was adopted as a numerical technique to solve the problem,with the use of Oil/MWCNT(oil/multi-walled carbon nano-tubes)nanofluid to raise the thermal conductivity of the flow field.The study is achieved for a Re number ranging from 12×103 to 27×103,while the concentration(φ)of solid particles in the fluid(Oil)is set to 4%.The computational results showed that the hydrothermal characteristics depend strongly on the flow patterns with the presence of VGs within the CHE.Increasing the Oil/MWCNT rates with the presence of VGs generates negative turbulent velocities with high amounts,which promotes the good agitation of nanofluid particles,resulting in enhanced great transfer rates.

KEYWORDS Channel heat exchanger;forced-convection;Oil/MWCNT nanofluid;CFD;vortex generators

Nomenclature

CpSpecific heat at constant pressure,J kg−1K−1

DhHydraulic diameter of exchanger,m

dfOil molecular diameter,m

dpNanoparticle diameter,m

DωCross-diffusion

eThickness of VG,m

HHeight of exchanger,m

GkKgeneration due to mean-velocity-gradients

Gωωgeneration

hxLocal convective heat transfer coefficient,W m−2K−1

kKinetic energy of turbulence,m2s−2

LLength of exchanger,m

Lin‘Inlet—attached-VG’distance,m

Ls‘Detached-VG–exit’distance,m

NuAverage Nusselt number of baffled exchanger

Nu0Average Nusselt number for smooth exchanger

PPressure,Pa

PatmAtmospheric pressure,Pa

PcPressure coefficient

PdDynamic pressure,Pa

PrNumber of Prandtl

ReNumber of Reynolds

Sk and SωSource terms

TTemperature,K

TITurbulent intensity,%

TinInlet fluid temperature,K

TwWall temperature,K

uX-velocity,m s−1

uiVelocity inxi-direction,m s−1

UinIntake velocity,m s−1

ujVelocity inxj-direction,m s−1

VAverage velocity,m s−1

vY-velocity,m s−1

YkKdissipation due to turbulence

Yωωdissipation due to turbulence

Greeks Symbols

ΓkKeffective-diffusivity

Γωωeffective-diffusivity

δijKroenecker delta

λThermal-conductivity,W m−1K−1

μDynamic viscosity,kg m−1s−1

μeffEffective viscosity,kg m−1s−1

μtTurbulent viscosity,kg m−1s−1

ρDensity,kg m−3

φSolid fraction,%

ΨStream-function,kg/s

ωSpecific dissipation rate

Subscript

atmAtmosphere

effEffective

fBass fluid

inIntake

nfNanofluid

pNanoparticle

sOutlet

tTurbulent

wWall

xLocal

1 Introduction

The insertion of turbulators and vortex generators(Ts-VGs)inside channel heat exchangers(CHEs)is well-known for its efficiency to enhance the convective heat transfer[1–7].Several studies have been reported on CHEs equipped with various types of obstacles.

Berner et al.[8]characterized the turbulent flow details throughout a channel with segmented baffles.Under laminar flow conditions,Webb et al.[9]simulated the dynamic behavior inside a duct with transverse ribs.The significant increase in transfer of heat was reached for fluids with high Pr numbers,such as fluorocarbons or water.Demartini et al.[10]mounted baffles on the surfaces of a rectangular duct to enhance the hydrothermal characteristics.Antoniou et al.[11]employed the hot wire technique to inspect the flow pattern around a prism with different aspect ratios(L/H).The increase ofL/Hyielded a reattachment of flows on the prism surface and downstream,with a reduction of turbulence scales and recirculation lengths.

By using numerical simulations and for a backward-facing step,Tsay et al.[12]obtained an enhancement by 190% inNunumber by employing a baffle.A strong dependence of the hydrothermal characteristics on the position of the baffle was also observed.Nasiruddin et al.[13]compared the efficiency of three orientations of baffles inside a tube heat exchanger.The highest performance was obtained with the 15◦-downstream inclined baffle.Under turbulent flow conditions and by numerical simulation,Yongsiri et al.[14]addressed the impact of attack angles(0 to 165◦)of detached-ribs in a channel.At highRe,the cases of 60◦and 120◦inclined ribs were the most efficient in terms of thermal transfer.However,so significant effect of the rib attack angle was observed at low Reynolds number.Ameur[15]inspected also the effect of baffle inclination inside a channel heat exchanger.

By experiments,Khan et al.[16]combined rib obstacles with inclined perforated baffles in a duct to enhance the hydrothermal behavior.Their results showed that the combination of ribs with baffle is an efficient technique in cases where the thermal transfer rate is critical to the device efficiency.Through numerical simulations and experiments,Ary et al.[17]used perforated VGs with holes of inclined-type diamond-shape in a duct.The numbers of holes had a great impact on the fluid current aspects and the local characteristics of transfer of heat.Furthermore,the efficiency of the single baffle was found to be lower than that of two baffles.Using aRenumber ranging from 2×104to 5×104,Ko et al.[18]measured the hydrothermal details within a channel provided with staggered porous obstacles.An enhancement in the heat transfer rates by 300% has been obtained in comparison with the unbaffled channel.

Under laminar flow conditions,Guerroudj et al.[19]predicted the impact of the shape of porous type blocks on the thermal transfer in a duct with the presence of simultaneous effect of buoyant and forced flows.The hydrothermal characteristics have been substantially altered by the shape of the blocks.ForRevarying from 102to 6×102,Sripattanapipat et al.[20]found an augmentation in the transfer of heat,from 200 to 680%,when using staggered diamond-shaped baffles with various inclination angles(5 to 35◦)in a horizontal channel.However,the friction loss has been increased up to 220 times over the unbaffled channel.By experiments,Abene et al.[21]inspected the efficiency of different kinds of VGs on the efficiency of an air-flow solar-channel,namely:waisted type tube(WT),waisted delta type lengthways(WDL1),ogival inclined type folded(OIF1),ogival type transverse(OT),and waisted ogival type lengthways(WOL1).The highest efficiency has been reached with the WDL1.

Bopche et al.[22]used turbulators of form of U on the surface of an absorber found in an air-flow heater.The values of Nu number as well as friction factor have been increased by about 2.82 and 3.72 times over than those for the unbaffled duct,respectively.Kumar et al.[23]determined the performance of an air-flow solar channel equipped with V-obstacles.Among the different cases inspected,the highest performance factor was around 3.14.Ameur et al.[24]reported a simulation to test the influence of a new baffle shape on the hydrodynamics and filtration of a membrane system.They examined two orientations of hemispherical baffles,namely:RO baffle for the Right Orientation and LO baffle for the Left Orientation.

For turbulent flows through CHEs,Li et al.[25]found that the multi V type baffles may provide an improvement in the thermal performance factor up to 12%.Other interesting papers are available(Tab.1),such as those of Yang et al.[26],Tongyote et al.[27],Ribeiro et al.[28],Promvonge et al.[29],Chen et al.[30],Muñoz-Cámara et al.[31],Eiamsa-ard et al.[32],Pourhedayat et al.[33],Li et al.[34,35],Abdullah et al.[36]and Mandal et al.[37].

Most of these studies have known a deterioration in their effectiveness of their engineering devices,due to their use of traditional fluids known to have low values of thermal conductivities,such as oil,ethylene glycol,water,etc.To address this constraint,researchers used the nanotechnology technique by supporting conventional fluids with nanoscale solid particles(for example,Cu,Ag,Al2O3,TiO2,Fe3O4,CuO,etc.),i.e.,forming new fluids,called nanofluids with elevated thermal conductivities.Recently,many studied have been achieved on the subject.For example,Barber et al.[38],Gupta et al.[39],Najah Al-Shamani et al.[40],Bozorgan et al.[41],Lomascolo et al.[42],Sadeghinezhad et al.[43],Mukherjee et al.[44],Babita et al.[45],Rasheed et al.[46],Yang et al.[47],Colangelo et al.[48],Ganvir et al.[49],Kasaeian et al.[50],Mohammed et al.[51],Che Sidik et al.[52],Nadooshan et al.[53],Angayarkanni et al.[54],Mahian et al.[55],Raj et al.[56],Taherian et al.[57],Zhang et al.[58],Zendehboudi et al.[59],Arshad et al.[60],Bumataria et al.[61]and Wahab et al.[62],as listed in Tab.2.

High thermal conductivity of nanoparticles yields a significant improvement of the overall performance,even with small amount of nanoparticles volume fraction.Several parameters may affect the nanofluids’ thermal conductivity such as the base-fluid nature,type,fraction,and form of the solid nano-particles.Further details may be found in the papers of Pourmehran et al.[63],Nazari et al.[64],Biglarian et al.[65],Sheikholeslami[66],Nojoomizadeh et al.[67],Nojoomizadeh et al.[68],Job et al.[69],Ashorynejet al.[70],Saba et al.[71],Ogunseye et al.[72],Bezaatpour et al.[73],Fakour et al.[74],Shahmohamadi et al.[75],Saqib et al.[76]and Siddiqui et al.[77],as reported in Tab.3.

Reinforcing thermal systems with nanofluids and porous media simultaneously is also an effective technique,as illustrated by Baghsaz et al.[78],Gholinia et al.[79],Alsabery et al.[80],Astanina et al.[81],Chamkha et al.[82],Ismael et al.[83],Mehryan et al.[84],Miroshnichenko et al.[85],Akhter et al.[86],Izadi et al.[87],Malik et al.[88]and Nithyadevi et al.[89],as tabulated in Tab.4.Other physical models under various boundary conditions can be found in Mahani et al.[90],Mehryan et al.[91,92],Ho et al.[93]and Mohammed et al.[94].

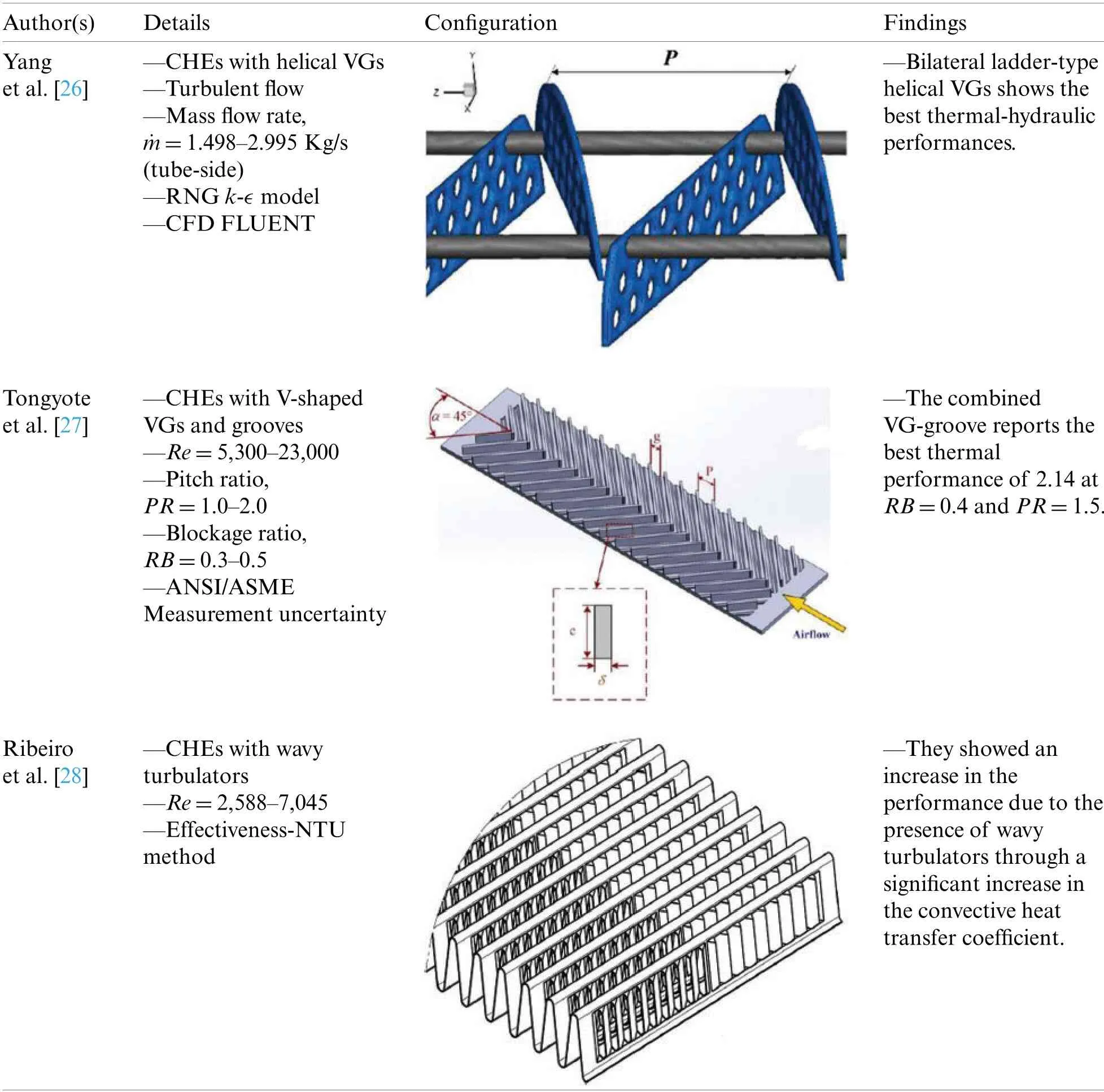
Table 1:Performance improvement of CHEs by using Ts-VGs

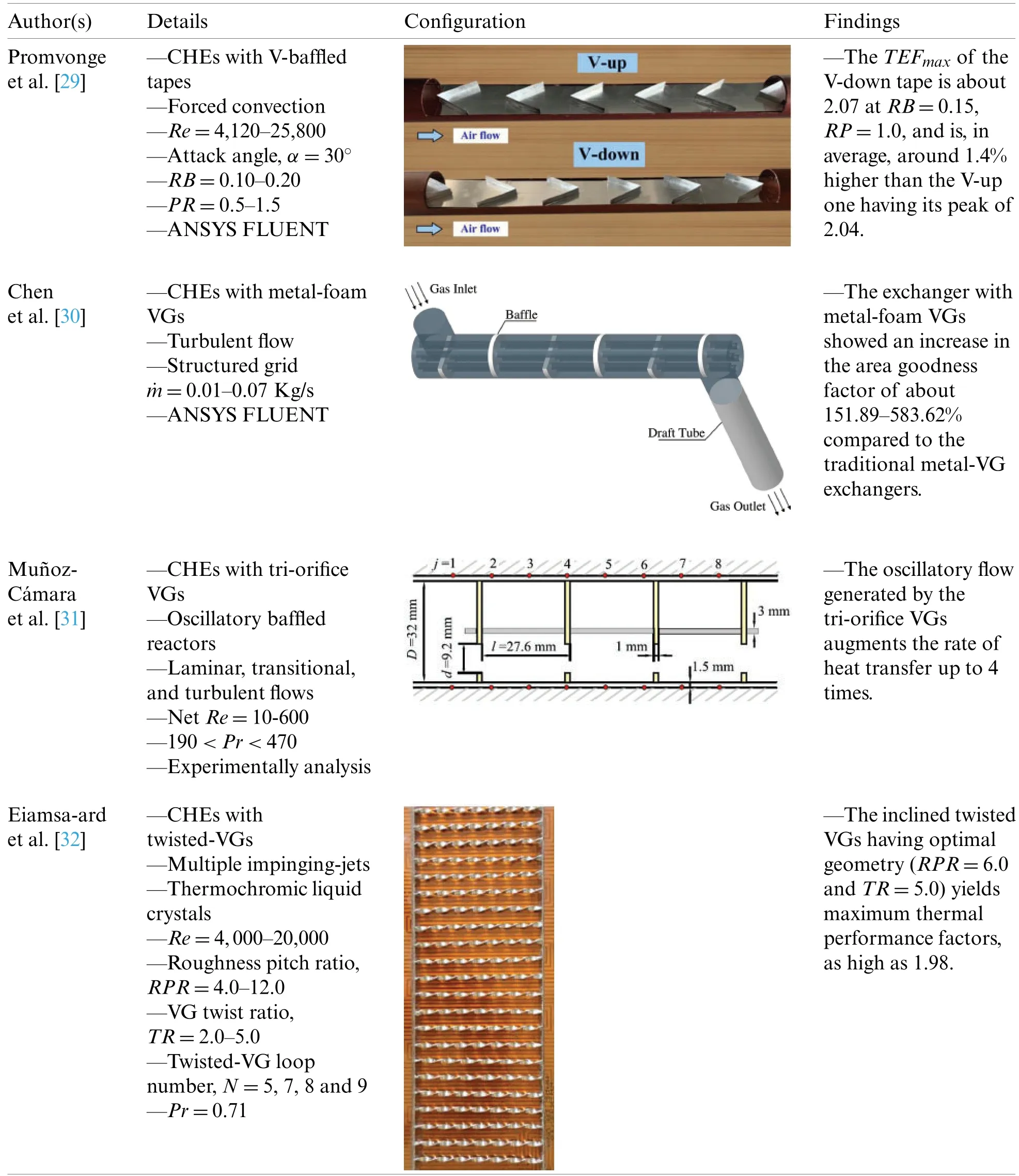
Table 1(Continued).

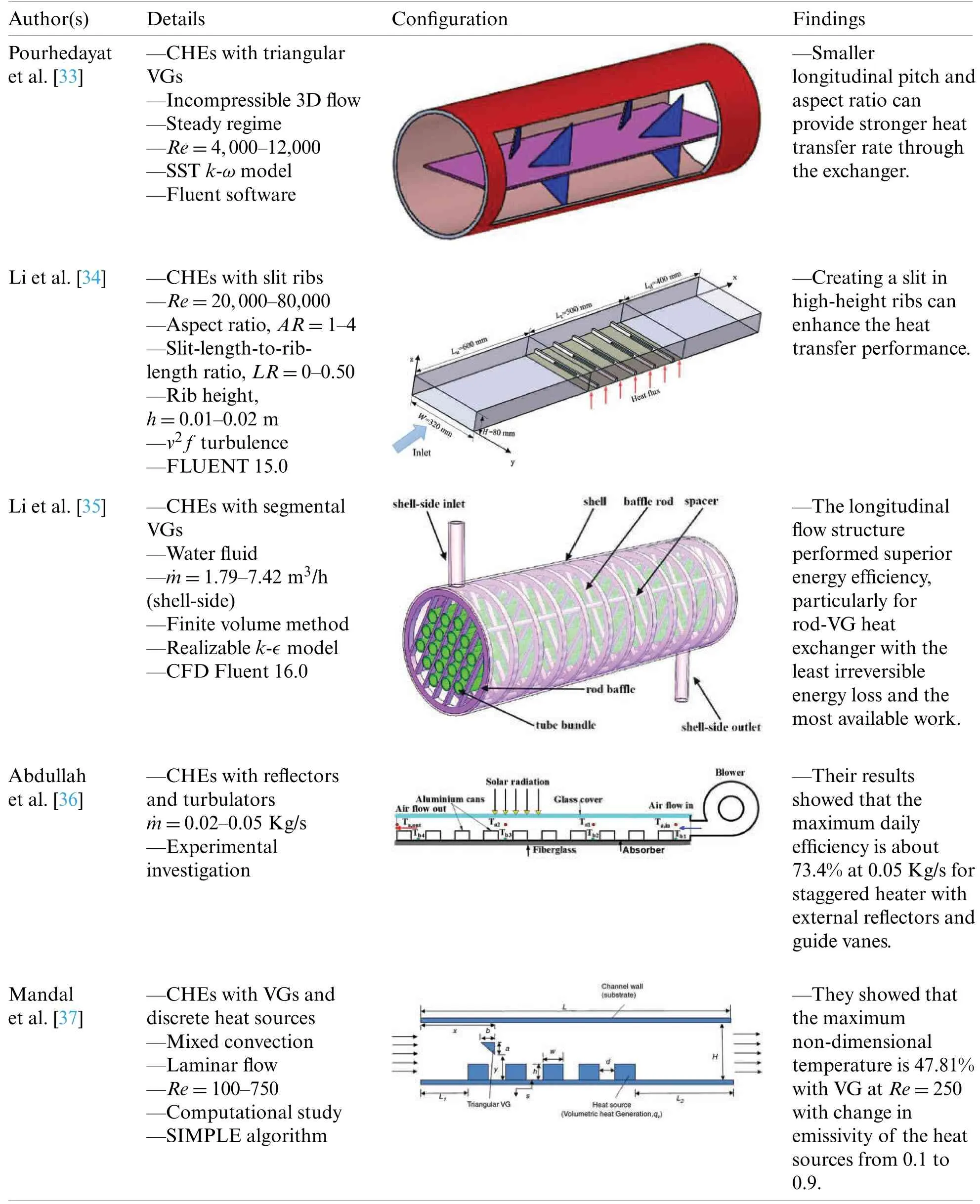
Table 1(Continued).

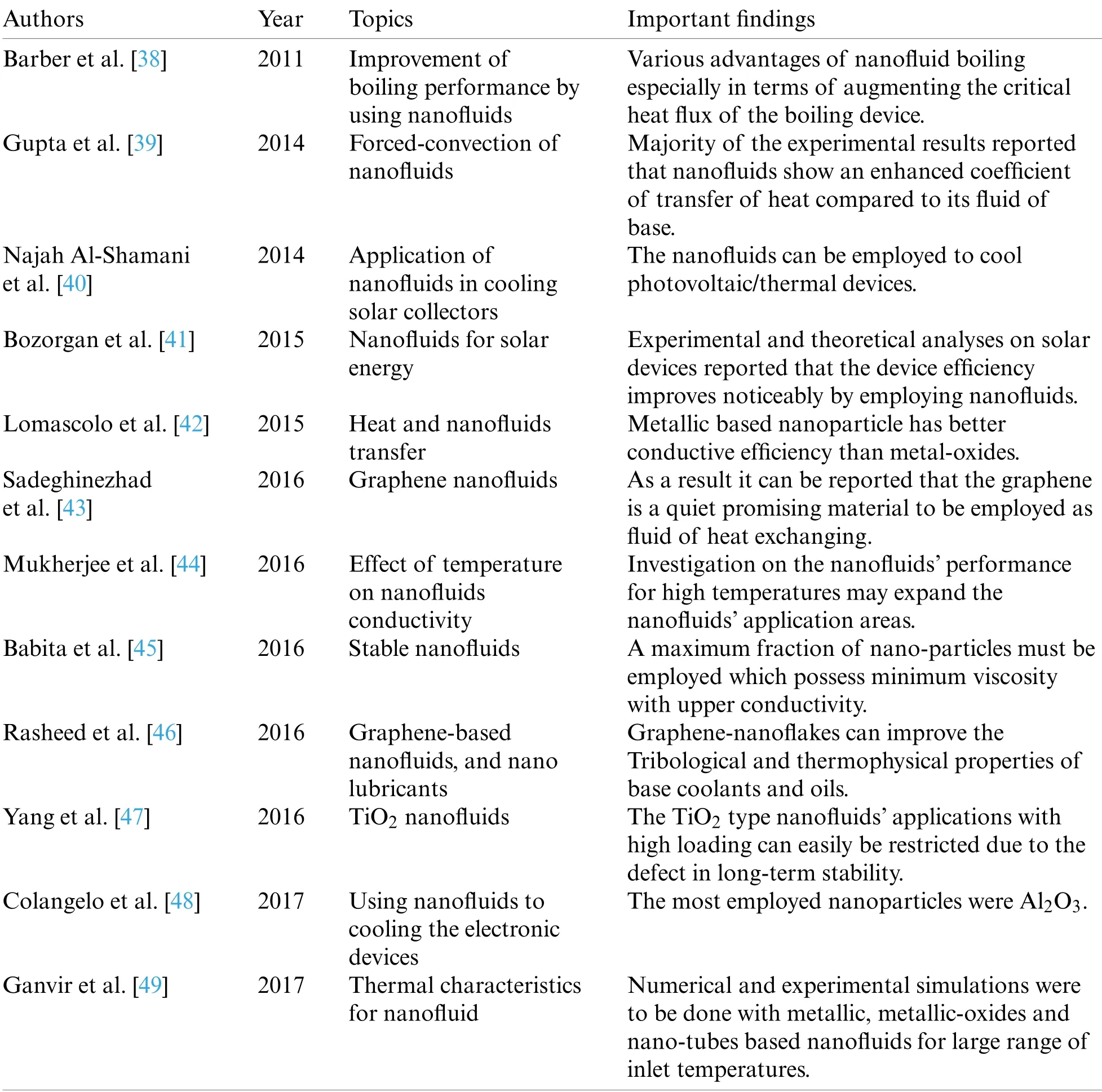
Table 2:Description of nanofluids—Review studies

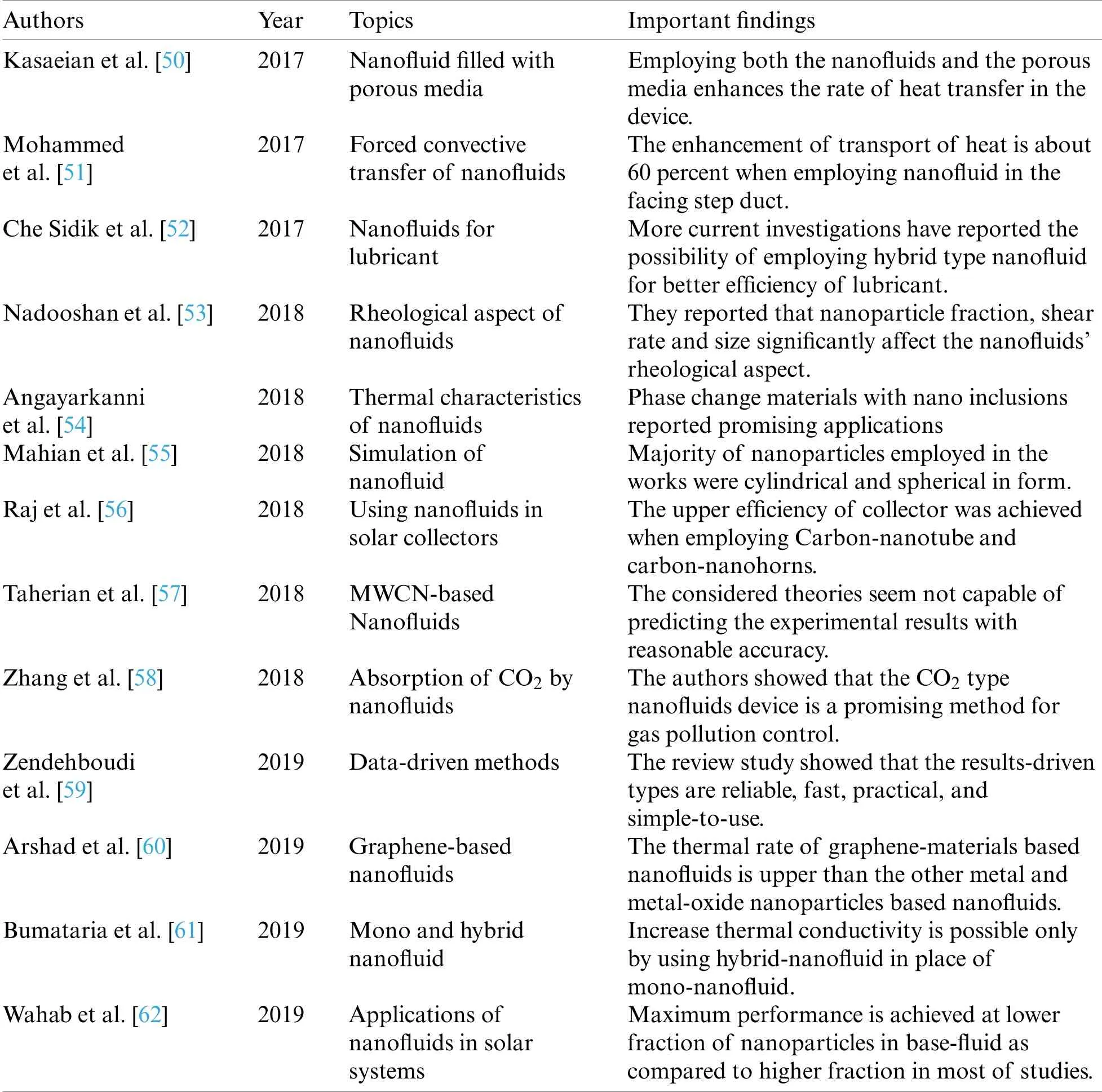
Table 2(Continued).

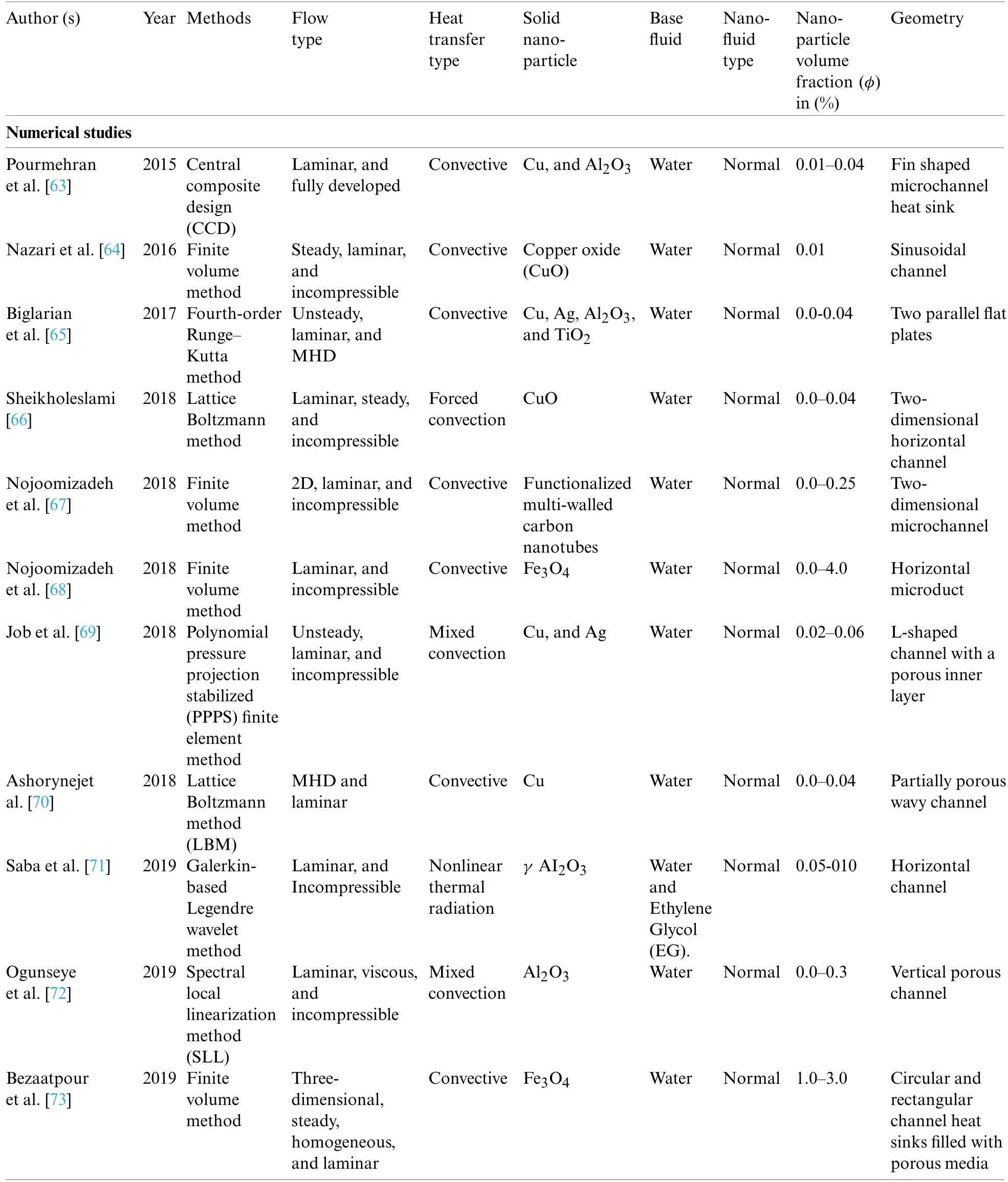
Table 3:Performance improvement of CHEs by using nanofluids

Table 3(Continued).

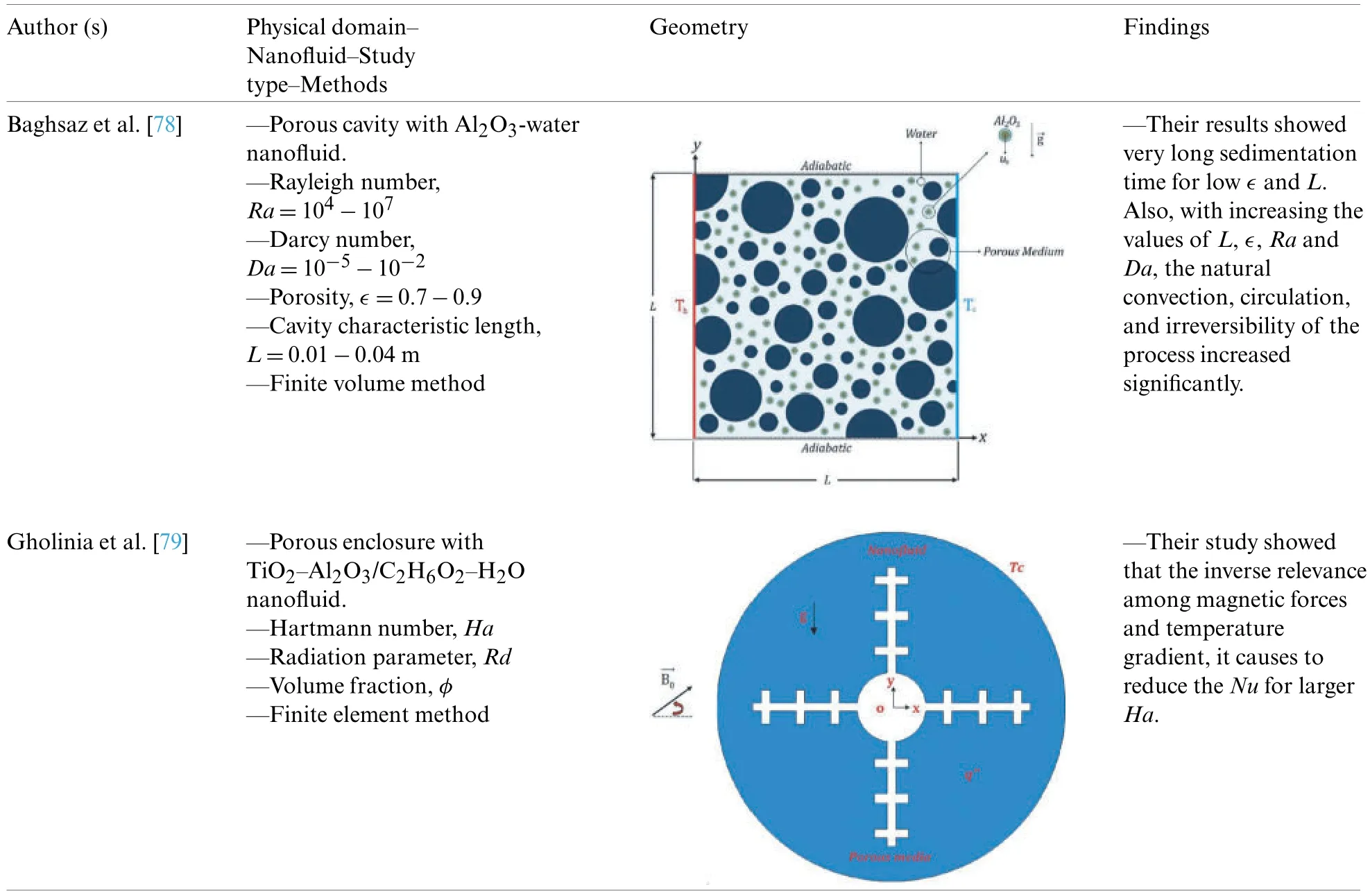
Table 4:Performance improvement of porous cavities and enclosures by using the nanofluids

Table 4(Continued).

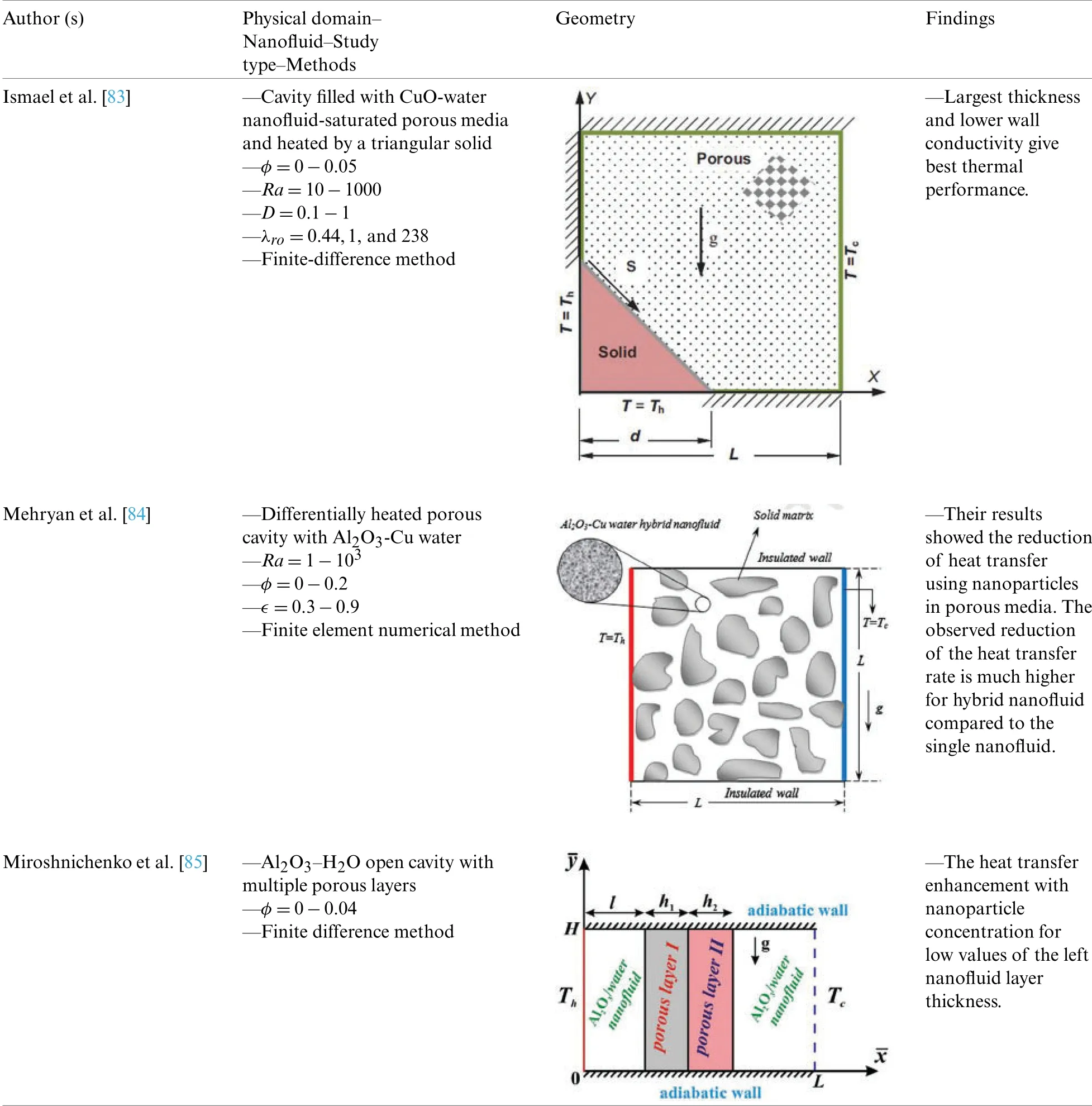
Table 4(Continued).

Table 4(Continued).

All the studies cited above have addressed the improvement of thermal and dynamic performance within channels by:

(i)increasing the heat transfer surfaces by adding transverse/longitudinal fins,

(ii)increasing the turbulence level by adding baffle plates,i.e.,VGs,or

(iii)by treating the nature of the fluid by inserting nano-sized solid particles ‘called:nanofluids.’

The majority of these studies used only one technique:insert the obstacles with the classic working fluid,or use nanofluids in the absence of obstacles.Therefore,and in this study,the two methods(baffling and nanofluid techniques)are adopted simultaneously by inserting VGs to improve the dynamic aspect of the flow,while the oil/multi-walled carbon nano-tubes(Oil/MWCNT)is used to promote a good heat transfer.

2 Mathematical Modeling

2.1 Physical Domain

This new study relies on a new model of CHE to update those in the solar receptors,Fig.1.The CHE is a horizontal and rectangular duct.The upper surface of the CHE subjected to a constant temperature(Tw= 375 K[13]),while the bottom surface is thermally insulated.Two baffles(VGs)are attached to the top and lower walls of the CHE.Both baffles are located at the distance,Lin,from the CHE inlet.The space between the tips of the upper and lower VGs is 0.45×H,which represents the length of the exit section.A third VG is added at the distance,Ls,behind the upper and the lower VGs.The 3rd VG is centered between the top and lower surfaces of the CHE.All dimensional parameters of the CHE are summarized in Fig.1.

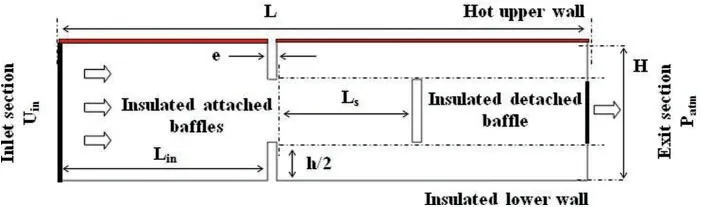
Figure 1:Computational domain.(L = 0.554 m, H = 0.146 m, Lin = 0.218 m, Ls = 0.142 m,e=0.01 m,and h=0.08 m[10])

The working fluid is an oil-based nanofluid,dispersed by nano-sized solid particles of Multi-Walled Carbon Nano-Tubes(MWCNT),with a volume fraction(φ)of 4%.The thermo-physical properties of the oil base-fluid(oil molecular diameter,df=2◦A)[95],carbon-nanotubes’nanoparticles[95],and Oil/MWCNT nanofluid(φ=0.04)[96]are presented in Tab.5[96].The Reynolds number is changed from 1.2×104to 2.7×104.

2.2 Physical Model

The hydrothermal field inside the CHE atφ=0.04 is subjected to some conditions,the most important of which are:(i)The forced-convection heat transfer and flow are two-dimensional;(ii)The stream is steady,turbulent,Newtonian[96],and incompressible;(iii)The nanofluid is homogeneous[96]and single-phase[96];(iv)The condition of no-slip boundaries is applied to the solid surfaces[10];and(v)The transfer of heat by radiation is neglected.

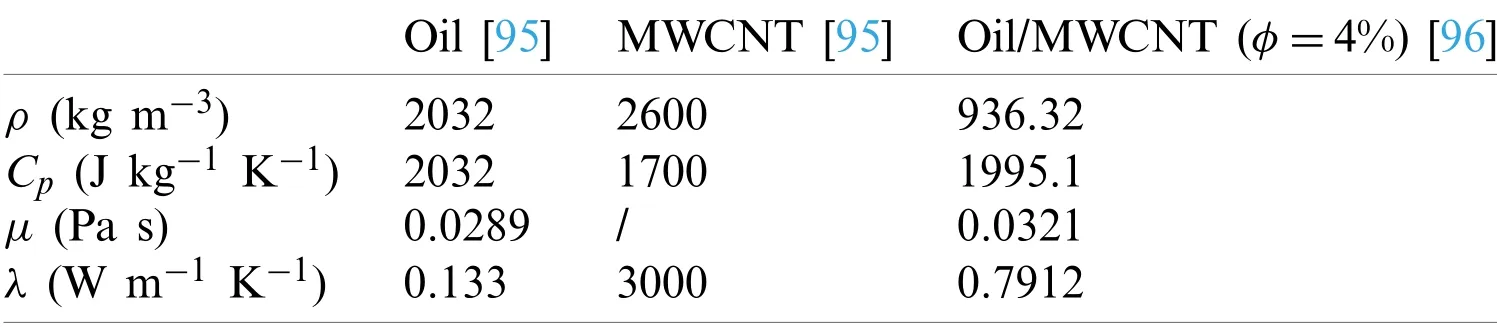
Table 5:Thermo-physical properties of 298 K used materials[96]

2.3 Boundary Conditions

In this simulation,the top wall of the CHE(0 ≤x≤LinandLin+e≤x≤L,y=H/2)is considered under the constant surface-temperature condition(Tw)of 375 K[13],and the opposite wall(0 ≤x≤L,y=−H/2)is considered thermally insulated.The T-VGs as well as the right walls of the channel also are considered as adiabatic.For the section of the channel entrance(x=0,−H/2 ≤y≤H/2),the profile of velocity is uniform[10,13](u=Uin,v=0),while the condition of pressure-outlet(P=Patm)[10]is applied at the exit section(x=L,−H/2+h/2 ≤y≤H/2−h/2).The fluidTin,i.e.,at the inlet,is 298 K[96].

2.4 Governing Equations

According to the hydrothermal conditions reported above,the present simulated physical model is governed by[97]:

The continuity:

The momentum:

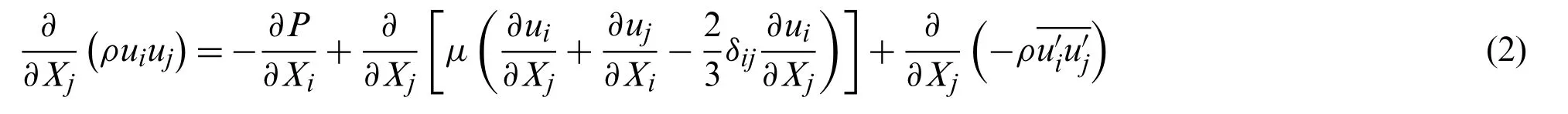
The energy:

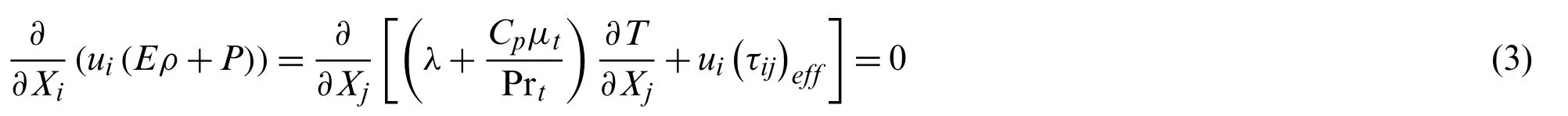
with,

•Total energy(E):

•Deviated stress tensor(τij eff):

The SSTk-ωmodel is used to model the turbulence in the case of the simulated configuration.Its turbulent kinetic energy,kis defined as follow[13,96]:

While,its specific dissipation rate,ωis given as follow:

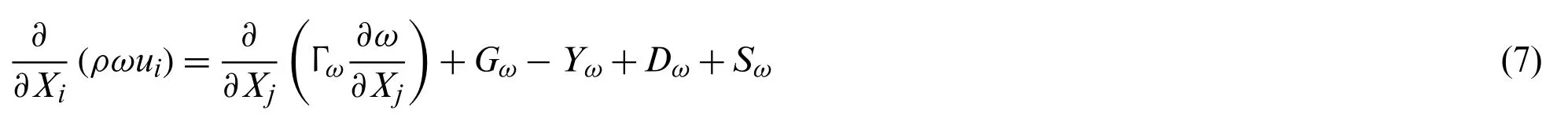
The thermo-physical properties of the nanofluid can be defined as follows[96]:

Density(ρnf):

Heat capacitance(ρ Cp)nf:

Thermal conductivity(λnf):

Effective dynamic viscosity(μnf):

p,fandnfare the nanoparticle,base fluid,and nanofluid,respectively.

Reynolds number(Re):

whereDhis thehydraulic diametercalculated as:

The heat transfer in terms of local Nusselt number(Nux)is defined by:

3 Used Resolution Technique

3.1 Numerical Solution(NS)

The study was realized by using ANSYS Fluent.The computer tool Gambit was employed to mesh the computational domain.The governing equations were discretized by FV(finite volume)technique[98].The SIMPLE-type algorithm was employed for the coupling ‘pressurevelocity’[98]and the Quick-scheme was applied to the interpolations[99],while a second-order upwind scheme[99]was employed for the pressure terms.The under-relaxation factor was varied between 0.3 and 1.0 to verify the update of the predicted results at each iteration[13].The residual target was fixed to 10−9for the convergence criterion.The numerical simulations were realized on a PC-i5 with a CPU frequency of 2.5 Go and a RAM of 6 Go.A typical model of running time(CPU time)for the calculation of a case is about 180–240 min.

3.2 Grid Independence

Rectangular mesh elements were constructed for the computational domain.The mesh density was increased near the CHE walls to capture the strong flow field gradients in these regions.The grid independence tests were conducted by changing the mesh nodes’density,from 35 to 145 along with the CHE height and from 95 to 370 along with the length.Forφ=0.04 andRe=1.2×104,the optimum values of the dynamic pressure(Pd)and the axial velocity(u)are plotted in Fig.2 versus the mesh density.The variation inPdanduvalues is found to be less than 1% when the number of cells has been changed from(245×95)to(370×145).Thus,the increase in the density of cells beyond this value is not needed.Considering the results precision,the mesh of(245×95)cells with high node concentration near the solid limits was selected for the next calculation.

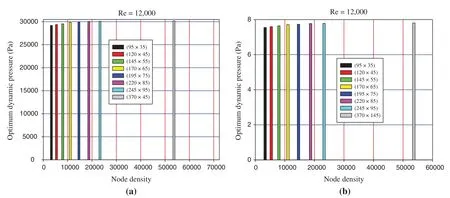
Figure 2:Effect of mesh node density on the NS in terms of(a)Pd and(b)u for Re=1.2×104

3.3 Model Validation

The simulated results are verified against the experimental and CFD results of Demartini et al.[10].For this reason,the same physical model and flow characteristics are considered[10]:(i)2D horizontal rectangular channel with 0.554 m of length,0.146 m of height,and 0.167 m of hydraulic diameter;(ii)Two upper and lower VGs with 0.08 m of height,0.01 m of thickness and 0.142 m of separation distance;(iii)Air fluid;and(iv)Re=8.73×104.

Figs.3a and 3b present the results of the pressure coefficient(Pc)and the axial velocity(u)forUin=7.8 m/s,respectively.The values ofPcanduare,respectively,calculated along with the CHE depth at the positionsx=0.223 m andx=0.525 m from the exchanger inlet.We note that the air is employed as a working fluid for this validation.As remarked,the comparison illustrates a good agreement.After checking the validity of our predicted results,the same CFD technique was used for the new model that contains:

Firstly,using the same CHE and its dimensions(L,HandDh)mentioned by Demartini et al.[10];

Secondly,use three VGs instead of two baffle plates[10],but with the same dimensions(Lin,Lsande)received from the same source[10];

Thirdly,adopt a new fluid,which is Oil/MWCNT nanofluid[96],instead of the traditional gaseous fluid(air)[10].

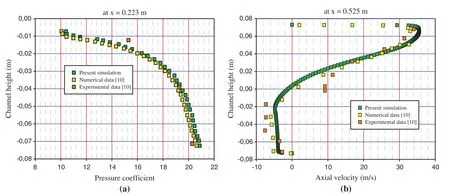
Figure 3:Validation of results in terms of(a) Pc and(b) u for Uin=7.8 m/s

4 Results and Discussion

This research is an important numerical simulation in the context of solar channel heat exchangers.Therefore,visualization of the flow distribution should be determined,indicating possible recycling phenomena,and highlighting regions with high temperature gradients.

4.1 Dynamic Pressure and Stream-Function Fields

Fig.4a shows thePdfield inside the CHE for a variableRevalue.ThePdvalues are very high across the first gap,between the upper edges of the top and bottom attached baffles,as well as through the upper and lower gaps next to the top and bottom walls of the channel,due to the existence of the detached third baffle.ThePdvalues are also high across the channel outlet,due to its smallness.Whereas,Pdvalues are low in the vicinity of the walls of the channel,from its inlet to the left sides of the attached baffles.ThePdvalues are also reduced on the rear sides of the three baffles and next to the upper and lower edges of the channel outlet.

From this same figure,it is very clear that thePdvalues would greatly improve ifRevalues improved from 1.2×104to 2.7×104.So,there is a direct correlation betweenRevalues andPdvalues.As theRenumber increases,thePdimproves.

Fig.4b shows the stream-function(ψ)field distribution whenRe=1.2×104to 2.7×104.From the figure,the nanofluid current enters from a large area,of a height ofH,located inx=0.The current is disturbed immediately after the inlet of the CHE,as it approaches the VGs.The current disturbance in this area results in the appearance of two recycling cells.The first cell of recirculation is located at the top of the channel,upstream of the upper VG,while the second cell is presented at the bottom,before the lower VG.These regions have lowPdvalues as reported in Fig.4a.The main current flows through the first gap,between the upper edges of the upper and lower VGs,towards the turbulator-type detached baffle.The presence of VGs leads to the formation of two recycling cells,next to their right sides,due to the decrease inPdvalues in their back regions.The presence of the turbulator-type third baffle in the CHE center allows the main current to be divided into two streams,heading towards the upper and lower gaps,next to the channel surfaces,to the exit section with the formation of two adjacent recycling cells on the back region of the same turbulator,due to a decrease inPdvalues.As shown in Fig.4b,the current disturbance augments as the number ofReincreases.

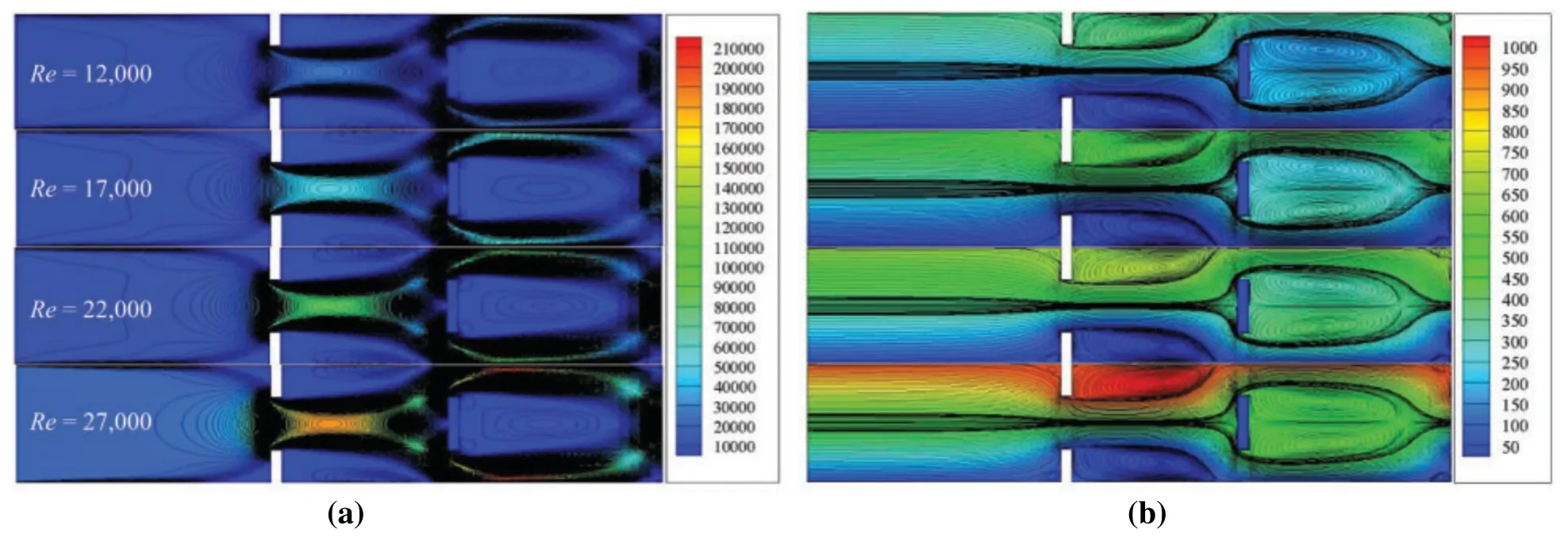
Figure 4:Hydrodynamic fields in terms of(a) Pd(in Pa)and(b) ψ(in Kg s−1)

4.2 Axial and Transverse Velocity Fields

From Fig.5a,the axial velocity(u)has negative values in the areas in front and back of the VGs,behind the turbulator,as well as next to the top and bottom edges of CHE outlet.Negativeuvalues are evidence of adverse currents called recycling cells.These cells have length,height,volume,strength,and speed to rotate in the reverse direction of the mainstream.However,theuvalues increase across the three gaps,due to the presence of T-VGs in the fluid circulation vein and severe current deviations on their left sides.It is very clear that largeuvalues are located near the CHE walls,behind the turbulator.Theuvalues enhance asRevalues improve.All geometrical dimensions of the recycling cells as well as their strength improve in the case of the wideRerange.

There is negative transverse velocity(v)values on the upper front edge of the upper VG,as well as on the lower front edge of the turbulator,while there are very highvvalues on the upper front edges of the bottom VG and the turbulator,see Fig.5b.As expected,the high number ofRecreates strong current deviations on the left sides of the T-VGs,towards the top and bottom of the CHE and thus,thevincreases in both directions,direct towards the top of the channel,and negative towards the its bottom.

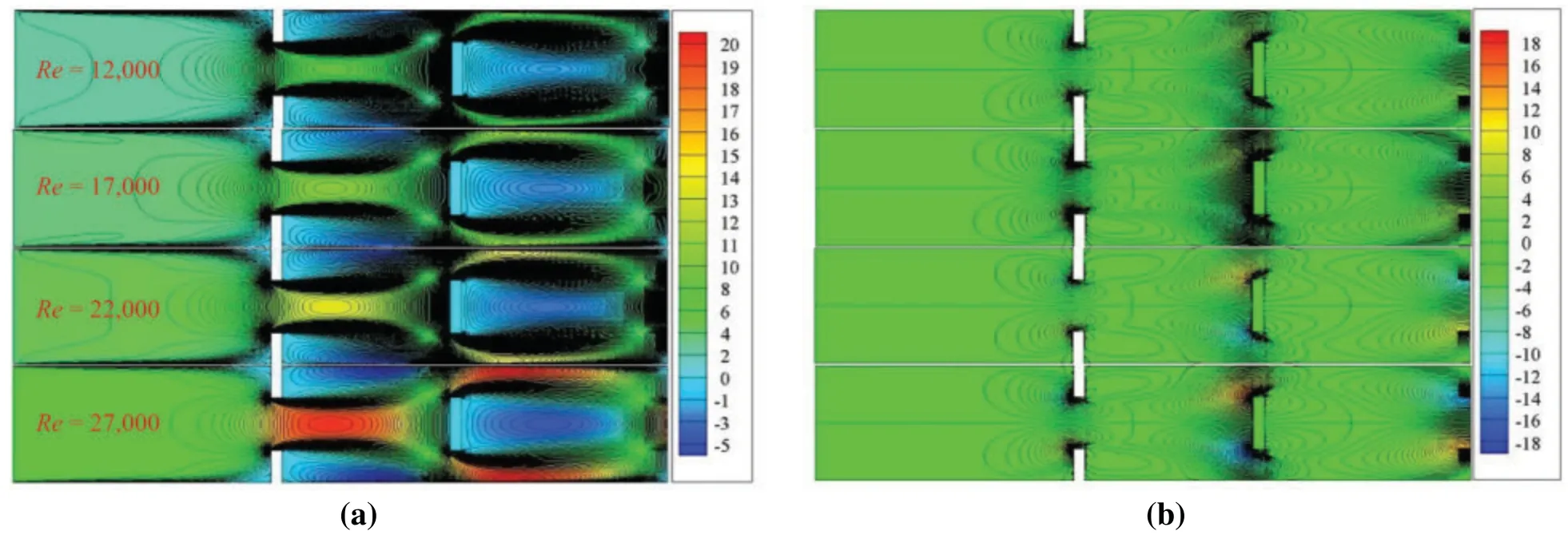
Figure 5:Hydrodynamic fields in terms of(a) u(in m s−1)and(b) v(in m s−1)

4.3 Mean Velocity and Kinetic Energy Fields

Mean velocity(V)values are low on the left and right sides of the VGs,on the rear side of the Turbulator,and next to the top and bottom edges of the outlet.In the region between the upper edges of the VGs,Vvalues rise as a result of decreases in the Oil/MWCNT circulation vein,and current flows through this gap under highPd.The current acceleration decreases as it approaches the front side of the turbulator,while accelerating through the upper and lower gaps adjacent to its edges,due to severe current deviations in these areas.TheVreaches its maximum values on the right side of the turbulator,near the top and bottom CHE surfaces.The nanofluid flows under highPdand very quickly to the CHE outlet in the middle,see Fig.6a.In addition,it is very clear from this figure that the change in Re number greatly affects the V of nanofluid flow where there is a direct correlation between the increase in the Re and the rise in V values.As the number ofReincreases,the nanofluid deviation force increases on the left sides of the T-VGs,towards the gaps,up to the CHE outlet.

Figure 6:Hydrodynamic fields in terms of(a) V(in m s−1)and(b) K(in m2 s−2)

From Fig.6b,it is evident that there are high turbulent kinetic energy(K)values on the upper front edges of the VGs,on the left side of the turbulator as well as on its upper and lower edges.In addition,Kvalues are high at the outlet.However,Kenergy decreases on the rest of the CHE areas,in front and behind the VGs and on the back of the turbulator as well as next to the top and bottom edges of the outlet.From the figure,it turns out that theKenergy of the Oil/MWCNT nanofluid current is influenced by the change in the value of theRenumber.TheKenergy gets better as the number of Reynolds improves.The turbulent kinetic energy values are very important for 2.7×104compared to 2.2×104,1.7×104and 1.2×104.

4.4 Turbulent Fields of Viscosity and Intensity

In Fig.7a,turbulent viscosity(μt)values are very high compared tokvalues.Theμtvalues are high across the three gaps and on the front ends of the T-VGs.Theμtvalues also rise behind the turbulator to the CHE exit.Conversely,μtvalues are very low near the solid boundaries of the CHE and the T-VGs.Theμtviscosity is directly proportional to the values of theRenumber as it reaches its maximum values in the exchanger outlet area,as reported in Fig.7a.

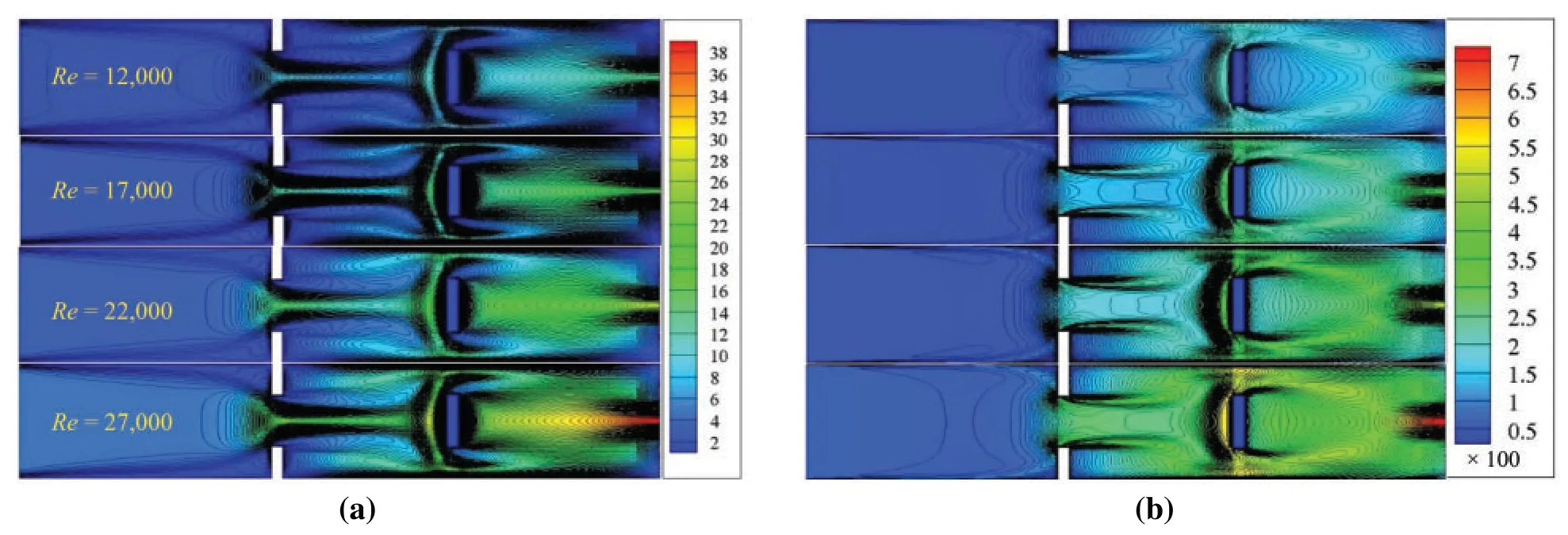
Figure 7:Hydrodynamic fields in terms of(a) μt(in kg m−1 s−1)and(b) TI(in %)

Fig.7b shows the contour plot of the turbulence intensity(TI)field.For the minimumRenumber,i.e.,1.2×104,theTIis low in areas adjacent to the right sides of the VGs,ranging from 30% to 50%.TheTIalso decreases on the rear side of the turbulator,estimated at 60%.TheTIhas low values at the exchanger entrance,estimated at 10%.This decrease continues until the first gap between the upper and lower edges of the VGs.There are also low values ofTInear the edges of the exit,estimated at 60%.TheTIrises to 80% next to the upper and lower walls of the exchanger to the front left sides of the VGs,reaching 120% near its edges.TheTIis also higher on the front side of the turbulator and next to its edges,estimated at 160%.In addition,there are high values ofTIaway from the rear side of the 3rd obstacle,ranging from 100% to 120%.TheTIreaches its maximum values at the exchanger outlet,which is estimated at 240%,24 times greater than that at the inlet.WhenRenumber changes from 1.2×104to 2.7×104,theTIincreases from 240% to 700% at the exit section.So,there is a direct correlation between the values ofTIand theRevalues.

4.5 Dynamic Pressure Profiles in Different Axial Sections

Computational data report very low profiles ofPdnext to the T-VGs.In the areas behind the T-VGs,zones of vortices with very low curves ofPdare presented.In the areas situated between the upper edges of the VGs,thePdis augmented.

Due to the variations in the streamlines reported by the T-VGs,the highest profiles ofPdappear downstream of the turbulator,near the top and bottom CHE surfaces,with a process of acceleration that found just after the third VG.Also,there is a direct correlation betweenRevalues andPdvalues in all sections examined as shown in Fig.8.

4.6 Normalized Axial Velocity Profiles in Different Axial Sections

Fig.9a shows the normalized axial velocity(u/Uin)profiles upstream of the top and bottom VGs,atx=0.19 m.ForRe=1.2×104,theu/Uinprofiles are very high next to the center of the gap formed between the edges of these VGs and very low near the top and bottom CHE surfaces.

A comparison of curves ofu/Uinbetween the VGs’top edges,atx=0.223 m,for the sameRevalue is given in Fig.9b.Theu/Uinprofiles at this location are flat.The values ofu/Uinare high in the middle of the gap,while decreasing next to the top edges of the turbulator.

Fig.9c reports the profile plot ofu/Uindownstream of the VGs,atx=0.26 m.ForRe=1.2×104,theu/Uinvalues are negative in the upper and lower sections of the exchanger,due to the presence of recycling cells on the back sides of the VGs.In the middle,there is a significant rise inu/Uinvalues,due to the rapid flow of the nanofluid current through the first gap under high pressure,due to the presence of these VGs.

Theu/Uinprofiles for the positionx=0.33 m,measured downstream of the inlet section,are shown in Fig.9d.There is a decrease in the highu/Uinvalues compared to the previous location,i.e.,atx=0.26 m in Fig.9c,due to the current approaching the turbulator,while the negative values ofu/Uinindicate the extension of the recycling cells located behind the VGs.

Fig.9e plots the distribution ofu/Uinprofiles in the positionx=0.375 m,starting from the tip of the turbulator to the opposite walls.ForRe=1.2×104,it is very clear that theu/Uinvalues are very high across the top and lower gaps,especially next to the upper and bottom edges of the turbulator.

Fig.9f shows theu/Uinprofiles at a station given byx=0.4 m,0.02 m after the turbulator.The low speed values are indicative of a large recycling zone on the right side of the turbulator,due to a decrease inPdvalues in this region.Theu/Uinvalues rise significantly near the top and lower surfaces of the exchanger,due to the rapid flow through the top and lower gaps in the presence of a highPd.

Figs.9a–9f also show the variation ofu/Uinprofiles with flow rate,i.e.,Re=1.2×104,1.7×104,2.2×104and 2.7×104,in different axial stations from the exchanger,i.e.,x=0.19,0.223,0.26,0.33,0.375,and 0.4 m,respectively.When the numberRerises from 1.2×104to 2.7×104,theu/Uinvalues go up considerably allowing rapid flow through the gaps while improving the size and strength of the rotating cells on the rear sides of the T-VGs.

4.7 Isotherms

Fig.10 shows the thermal field for a constantRevalue of 12,000.The nanofluid temperature(Tnf)is high in areas with low speeds;next to the top VG,due to the recycling cells in these CHE regions.However,theTnfgradients are improved in high-velocity areas;in the area opposite the detached.

Figure 8:Effect of Re on the Pd curves in different axial sections

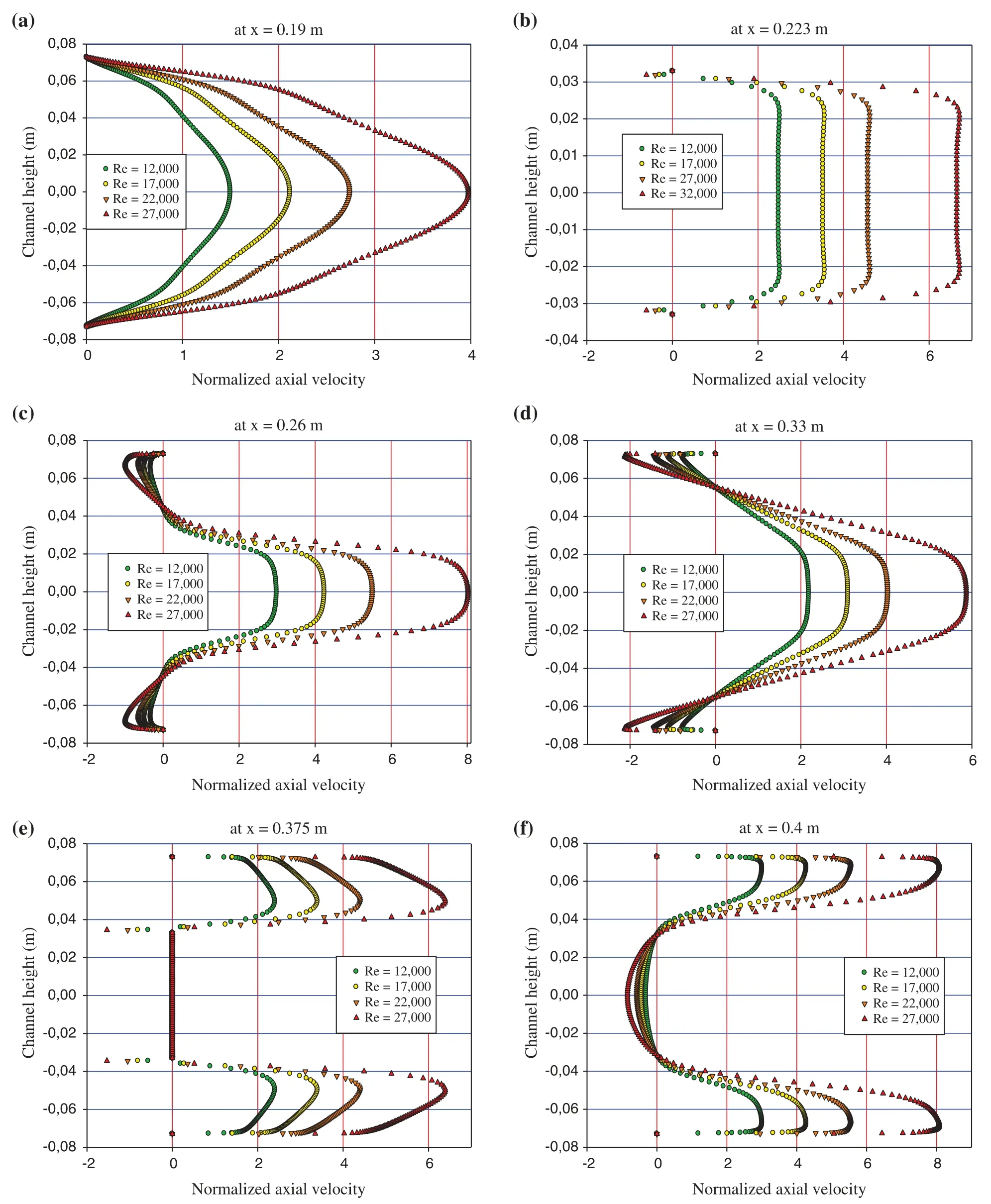
Figure 9:Effect of Re on the u/Uin curves in different axial sections

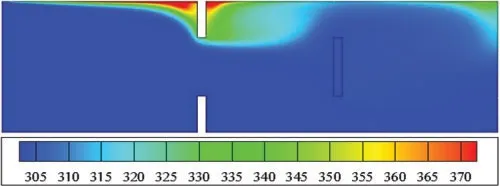
Figure 10: Tnf contour plot for Re=12,000

4.8 Temperature Profiles in Various Axial Sections

Fig.11a represents theTnfchanges of the field along the vertical position,located atx=0.19 m from the exchanger inlet,i.e.,28 mm before the left side of the VGs.It is very clear that theTnfatRe=12,000 is variable from 298 to 330 K in the upper part of the CHE,due to the presence of convective heat transfer from the hot top surface to the nanofluid,while its temperature is constant in the lower part of the exchanger and estimated at 298 K,due to the lack of heat transfer in this area,due to the absence of hot surfaces.

Fig.11b shows the temperature curve of the nanofluid current on the line extending between the upper edges of the VGs,in the axial positionx=0.223 m of the inlet of the exchanger,meaning 331 mm before its exit.From the figure,theTnfis elevated next to the upper edge of the top VG,up to 301 K atRe=12,000,whereas,its temperature decreases as the fluid moves away from the hot surface where it reaches 298 K next to the top edge of the bottom VG.So theTnfvaries from 298 to 301 K between the edges of the VGs.

Fig.11c shows theTnfvariations on the back region of the VGs,at the transverse positionx=0.26 m from the exchanger inlet,32 mm after the right sides of the VGs,110 mm before the left side of the turbulator,and 294 mm before the CHE outlet.TheTnfvalues are constant in the lower part of the exchanger,298 K(forRe=12,000),because there are no hot surfaces in this region of the CHE and therefore,there is no heat transfer.However,theTnfis variable in the upper region of the exchanger where there is a large recycling cell in this area.TheTnfvalue ranges between 301 and 319 K in the recycling zone between the tip of the top VG and the hot wall of the exchanger.TheTnfdrops from 319 to 298 K in the area between the top edge of the upper VG and the bottom of the CHE.

At the transverse positionx=0.33 m behind the inlet section of the exchanger,102 mm from the right sides of the VGs,40 and 224 mm before the front side of the third VG(or turbulator)and the outlet section of the CHE,respectively,and according to Fig.11d,theTnfis improved next to the hot surface of the exchanger where it ranges between 298 and 305 K atRe=12,000.This indicates the presence of heat transfer in the region.

At the exchanger outlet,through the positionx=L,and based on Fig.11e,the temperature of the nanofluid is varied from 298.059 K,at the bottom of the outlet section due to the absence of hot spaces in the lower part of the CHE and therefore there is no heat transfer in this area,to 299.6 K at the top of this same exit section,due to the presence of a hot surface in the upper part of the exchanger and,it is very clear that the maximumTnfdrops from 299.6 to 298.69 K ifRevalues rise from 1.2×104to 2.7×104.

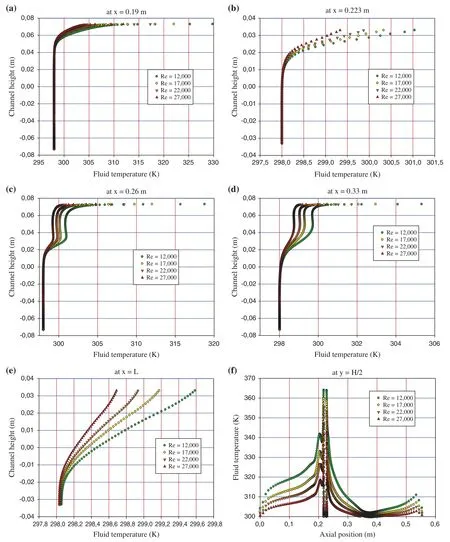
Figure 11:Effect of Re on the Tnf curves in different CHE stations

Fig.11f examines theTnfdistribution along the top horizontal station,i.e.,y=H/2.In the figure,two stations have low temperature gradients.The first station is situated next to the upper VG,due to the lowVandPdon its front and rear sides.The second station is located above the exchanger exit section.All of these regions have poor heat transfer.The high gradients of temperature are located next to the top edge of the turbulator,due to the strong current flow and its extreme deviation on the front side of the third obstacle towards the top and lower parts of the CHE.Thus,the thermal gradient rises in the stations with highVandPdvalues.Also,the thermal gradient is proportional to the values of theRenumber,along the hot top surface of the exchanger,as also reported in Fig.11.

4.9 Heat Transfer Rate

The heat transfer rate of the hot wall,0 ≤x≤LinandLin+e≤x≤L,y=H/2,is calculated in terms of the normalized local Nusselt number(Nux/Nu0)and for different values of flow rate(12,000 ≤Re≤27,000).TheNuxnumber is given by Eq.(14),while theNu0number represents the Nusselt number for the smooth channel under the same flow conditions.Three different phases can be distinguished(Fig.12).The first phase has a decrease in theNux/Nu0values,on the front of the upper VG,as the flow is deflected downward towards the gap situated between the two attached VGs.The second phase indicates the absence of the heat transfer in the attachment position of the insulated upper VG.The third phase shows an increase in the heat transfer values in the vicinity of the detached VG.In general,the heat exchange values are maximum for areas with high flow velocities,i.e.,with high temperature gradients,and which are located near the upper surface of the third VG,while the smallNux/Nu0values are situated near the front and back sides of the top VG.The figure also shows an enhanced heat transfer in the baffled exchanger compared to the smooth exchanger for allRevalues used.Finally,the heat transfer rate is further improved when the flow rate goes from 12,000 to 27,000.

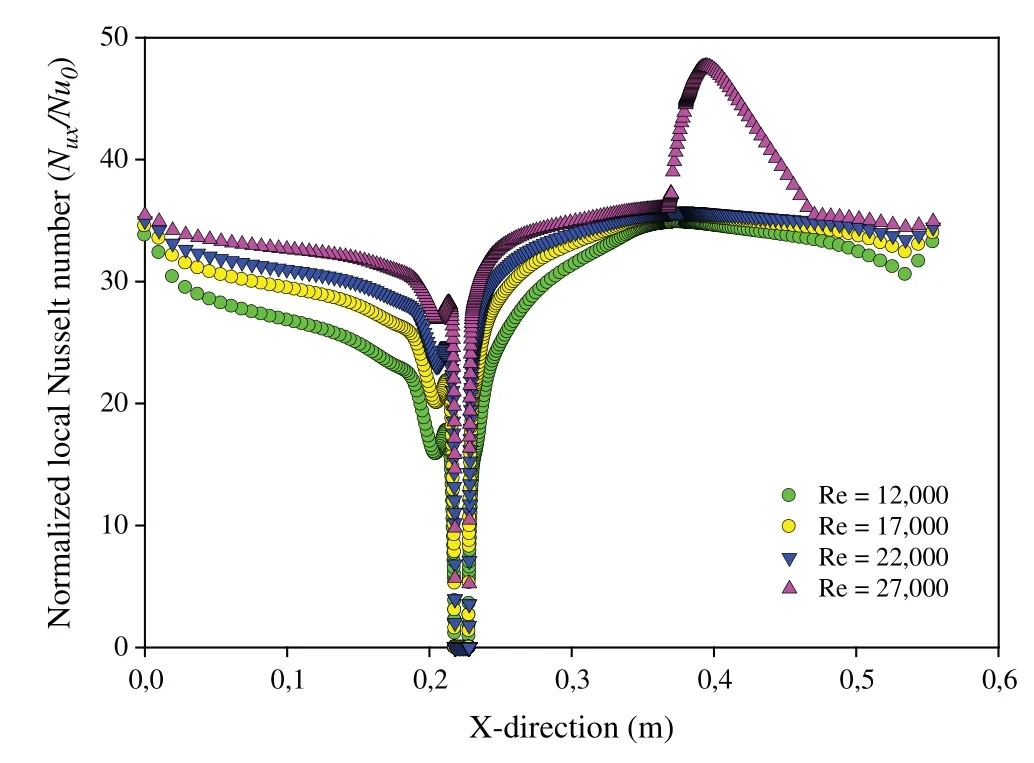
Figure 12:Variation of(Nux/Nu0)with Re number

5 Conclusion

This paper aimed to develop a new design of SCHEs to improve their overall energy efficiency.The study simulated a new channel configuration by introducing VGs.The FVM was adopted as a computation method to solve the problem,with the use of Oil/MWCNT to raise the thermal conductivity of the flow field.This is an appreciated effort in the field of numerical analysis of convective heat transfer in a channel with T-VGs.Comprehensive literature review in the introduction part and detailed analysis of the fluid flow and energy was highly appreciated.The computational results showed that the hydrothermal characteristics depend strongly on the flow patterns with the presence of VGs within the SCHE.Increasing the Oil/MWCNT rates with the presence of VGs generate negative turbulent velocities with high amounts,which promotes the good agitation of nanofluid particles,resulting thus in enhanced great transfer rates.This selected heat exchanger can be applicable in various sectors such as heating and cooling of houses,drying agricultural food materials,and water desalination process.

For future developments,here are some research perspectives to be considered:

(i)Further investigations are needed to precise the best location of the Ts-VGs.The geometrical parameters of the vortex generator should be optimized,such as thickness,width,inclination,arrangement,etc.

(ii)To eliminate the lower thermal transfer regions that are developed behind the VGs,the introduction of holes in the walls of the obstacles may overcome this issue.When using the perforation technique,several parameters should also be optimized,like the number of pores,their shape and dimensions,and the porous medium permeability.

(iii)The 3D computations may allow further knowledge for the entire volume of the solar collector.

Acknowledgement:The authors would like to thank the General Directorate of Scientific Research and Technological Development(DGRSDT)of the Ministry of Higher Education and Scientific Research in Algeria,which sponsored this work.

Funding Statement:The work was supported by the Natural Science Foundation of China(Grant Nos.61673169,11301127,11701176,11626101,11601485).

Conflicts of Interest:The author(s)declared no potential conflicts of interest with respect to the research,authorship and publication of this article.