Ellipticity-dependent ionization yield for noble atoms∗

Hristina Delibaˇsi´c and Violeta Petrovi´c

Faculty of Science,University of Kragujevac,Radoja Domanovi´ca 12,34000 Kragujevac,Serbia

Keywords: elliptical polarization,ponderomotive potential,Stark effect,Gaussian laser pulse

1. Introduction

Photoionization is a fundamental physical process that occurs when an atom or molecule absorbs light of sufficient energy to cause an electron to leave it and create a positive ion. Consequently, there has been intensive research activity in both experimental and theoretical physics[1,2]to understand the detailed photoionization dynamics of atoms and molecules that are exposed to an external laser field. As a result, many theories were defined as the theoretical framework of these processes.[3,4]

The first theory was developed by Keldysh who, in order to describe the ionization dynamics of atoms by intense electromagnetic fields,introduced a quasistatic tunneling picture in his paper in 1965.[3]Following the calculation of the tunneling ionization rate for the ground state of hydrogen in a static electric field by Landau.[5]Keldysh extended the theory to the ionization by strong electromagnetic fields. In the original derivation,he introduced the dimensionless parameter(known as Keldysh),which is defined as the ratio between the frequency of laser light ω and the frequency of electron tunneling through the potential barrier formed by Coulomb potential and electric fieldwhere Ipis the unperturbed ionization potential and F is the amplitude of the electric field. Here and throughout the paper,all equations are given in atomic units(a.u.,e=me=¯h=1)[6]unless otherwise stated.

As one of its central results,Keldysh theory showed that multiphoton ionization and electron tunneling are,in fact,two pathways that dominate photoionization in the strong-field regime. The ionization process in which tunneling or multiphoton regime takes place is determined by the Keldysh parameter γ. For γ ≫1 multiphoton ionization is the dominant process, while for γ ≪1, the tunneling is the dominant process. Yudin and Ivanov,[7]and Ivanov et al.[8]suggested that the intermediate range of the Keldysh parameter, γ ∼1, corresponds to the nonadiabatic tunneling regime. Additionally,according to Reiss[9]the regime when γ ∼1 at λ =800 nm ionization in a strong laser field can be successfully described as a tunneling process.

Over the last few years, the behavior of atoms in elliptically polarized laser fields has attracted increasing attention and still involves a wide range of topics to be studied.[10]To shed more light on atomic ionization in an elliptically polarized field, it is necessary to extend the quasistatic tunneling theory. During the last decade,when the extension of the quasistatic tunneling picture is appropriate and which kind of extensions are valid have been extensively studied.[11,12]

The theoretical approach to the tunneling problem is based on a single-active-electron approximation which is based on the assumption that only one electron is involved in the ionization process. The Ammosov-Delone-Krainov(ADK) theory[4]is one of the most widely used theories in this area, which has been experimentally verified many times.[13,14]The physical idea of this theory is based on the assumption that ionization occurs within a period of only a fraction of an optical cycle so that the laser field can be regarded as quasistatic.

Due to its simplicity,the ADK theory is commonly used in practical applications even in the intermediate range of the Keldysh parameter γ. According to this theory, the exponential growth of ionization rate,W ∝exp■2(2Ip)3/2/3F■, is determined primarily by the field strength F and the ionization potential Ip, and, therefore, here we focus on the ionization rate behavior when the ionization potential is modified by the ponderomotive potential[15]and the Stark shift.[16]The main goal of this paper is to investigate the applicability of the ADK theory by implying a nonadiabatic effect during a tunneling ionization process for an elliptical polarization with γ ∼1.To achieve this,we perform a comparative study between experimental data[17]and modified ionization yield for the case of singly ionized noble-gas atoms. Furthermore, to eliminate disagreement between theory and experiment, we consider a Gaussian-shaped laser pulse[18]and analyze the behavior of the ionization yield.

This paper is structured as follows. An introduction part is followed by Section 2,theoretical framework,where we define an expression for the ionization yield for elliptical polarization of the laser field with corrected ionization potential and temporal profile.In Section 3,results and discussion,we apply the obtained formula on noble-gas atoms and compare them with experimental data. Our conclusions are summarized in Section 4.

2. Theoretical framework

In the tunneling regime, for a linearly polarized laser field, the ADK ionization rate is characterized by the following expression:[19,20]

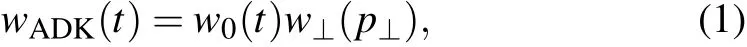
where the first term

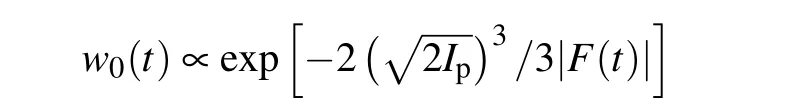
relates to the instantaneous laser field|F(t)|,while the second term

depends on the initial transverse momentum p⊥. Both terms of w0(t)and w⊥(p⊥)are related to the ionization potential Ip.We have chosen the dependence of the ionization rate on the transverse momentum spread along the field direction because even for small ellipticity, the shape of the transverse momentum distribution changes notably.[21]Recently, Arissian and his coworkers[22]showed consistency in the transverse momenta with the ADK theory.

The electric field of the ionizing laser pulse in the (x,y)plane can be described within the dipole approximation as[23]

where ε is the ellipticity of the laser radiation and f(t)is the pulse envelope, f(t)=sin2(ωt/N)(N is the number of optical cycles in the pulse)with a maximum of f(0)=1. The value of ellipticity varies in the range 0 ≤ ε ≤1;and for ε =0,the wave is linearly polarized, while for ε =±1, it is circularly polarized.

The non-adiabatic theory was initially developed based on Perelomov, Popov, and Terent’ev (PPT) theory.[24]Based on this theory, approaching the nonadiabatic regime with the intermediate range of the Keldysh parameter γ ∼1, Mur et al.[25]and, more recently, Geng et al.[26]proposed the presence of a transverse momentum in elliptically polarized laser field as: p⊥=εF[(sinh(τ0)/τ0)-1]/ω.[26]Here, τ0represents the complex time which is determined from the following equation: τ0=-iωt0, where t0is the purely imaginary time.[26]In the nonadiabatic tunneling regime irradiated with femtosecond laser pulses,this inline equation can be rewritten as an asymptotic transverse momentum p⊥∼ εF/ω.[27]This can be achieved by using the imaginary time method[28,29]and definition of the hyperbolic sinus function sinh(ix) =i sin(x),[30]as well as small angle approximation sin(x)≈x,x ≪1.[30]Inserting an asymptotic transverse momentum p⊥,and Eq.(2)into Eq.(1)and using some simple mathematical manipulations,wADK(t)is then given by[23]

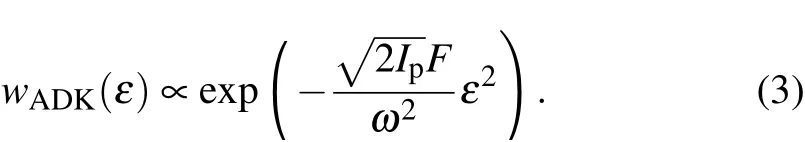
Experiments have clearly shown that the results of this equation are in very good agreement with the experimental findings.[31]Equation (3) explicitly indicates that the ionization rate wADK(ε) decreases exponentially as the strength of the laser field F, ellipticity ε, and ionization potential Ipincrease. The fact that wADK(ε)depends sensitively on the ionization potential Ipmotivates us to check how modification of the ionization potential changes the ionization rate.

The intense laser field influences the electron’s binding potential,perturbs it and makes it higher than the unperturbed value. There are at least two reasons for this increase: the ponderomotive potential and the Stark effect.[31]The ponderomotive potential is represented as the average oscillation kinetic energy of a free electron in the electric field of the laser with strength F, and for an elliptically polarized laser field it is given by the formula: Up=[F2(1+ε2)]/4ω2.[32]The ponderomotive potential causes a shift of atomic energies to the continuum. Thus, the resulting ionization potential is given as a sum of unperturbed and ponderomotive potential,Ip→Ip+Up.[33]Also,the energy levels of an atom are altered in the laser field and this effect is known as the Stark effect.This displacement of the energy level is determined by the expression, Ist=αpF2/2+γhF4/24,[16]where αpis the dipole polarizability and γhis the dipole hyperpolarizability. The val-ues of polarizability αpand hyperpolarizability γhfor different atoms and ions can be found in Refs.[16],[34],and[35].

Having both effects in mind, we can write the corrected ionization potentialin the following form:[36]

To analyze how the ionization rate wADK(ε) is affected by the corrected ionization potential,we substitute the unperturbed ionization potential Ipwith the shifted one,correct the effective ionization potentialin Eq.(3)and obtain the following expression:

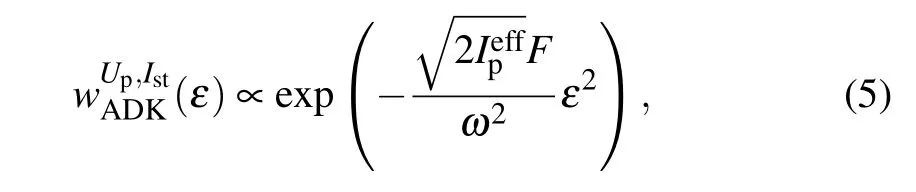
One of the most important points about the ionization rate is the laser beam shape because no matter how fast the ionization process occurs,it is dependent on the laser field strength.In theoretical studies, the main purpose of changing parameters such as envelope f(t), amplitude F, and frequency of the laser field ω,is to examine how they influence the ionization rate.[37]On the other hand,the purpose of beam shaping in the experimental environment is to wipe off fluorescence around the laser beam, decrease pulse distortion, and fabricate all kinds of figures.[38]Additionally,the change of a beam shape in the experimental environment may provide evidence for explaining a future theory.There are many different shapes and here we want to discuss how the choice of some particular shape influences the rate.

We consider the case of a Gaussian shape which resembles an experimental laser pulse in a reasonable manner. The temporal distribution of this laser beam shape can be represented in the following form:[18]

where t0=Nπ/ω (N denotes the number of optical cycles in the pulse)and σ2=t20/4ln(20). The modulation of the generally assumed laser beam shape F with the Gaussian shaped laser beam FG(t) in Eq. (5) allows us to compare our results with experimental data.[17]We incorporate the laser beam shape FG(t)in the formula for the ionization rate wADK,corr(ε)and obtain

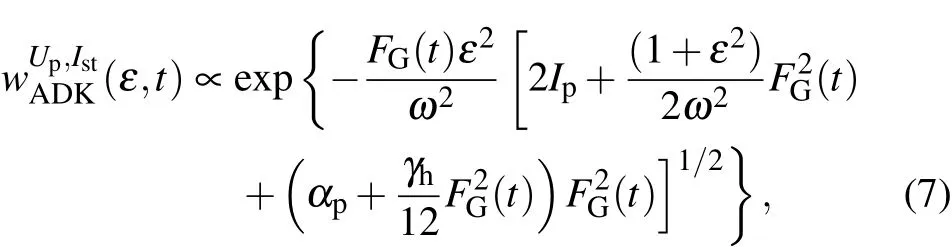
where the part with temporal distribution of this laser beam shape FG(t) can be expanded into a power series (exp[-x]≈1-x+···),and written in the following form:

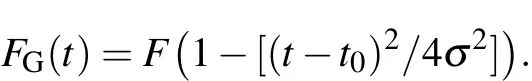
The fact that the ion yield is more often measured in experiments motivates us to calculate the appropriate ionization yield based on the obtained rate(Eq.(7)). To achieve this,we use the following expression:[39]

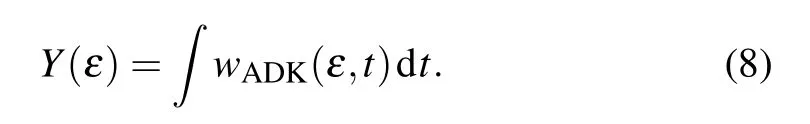
First,we calculate the ionization yield based on Eq.(8)without any corrections of the ionization potential. Substituting Eq.(5)into Eq.(8)provides the following equation:

To solve the integral,we use the Gauss error function:[40]

As expected,the integral in Eq.(9)simplifies and the final result is

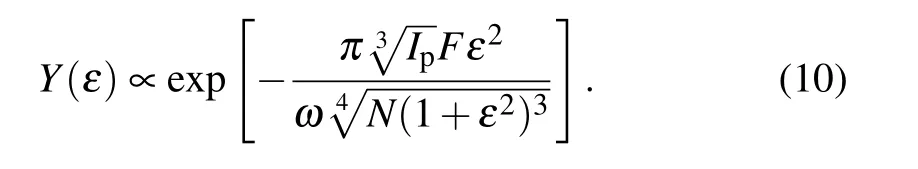
Next, we substitute Eq. (4) into Eq. (8) to determine the ionization yield YUp(ε), when the ponderomotive potential Upis included. Now,the resulting yield is given by

We repeat the procedure and additionally use a Gamma function,,arriving at the following result:

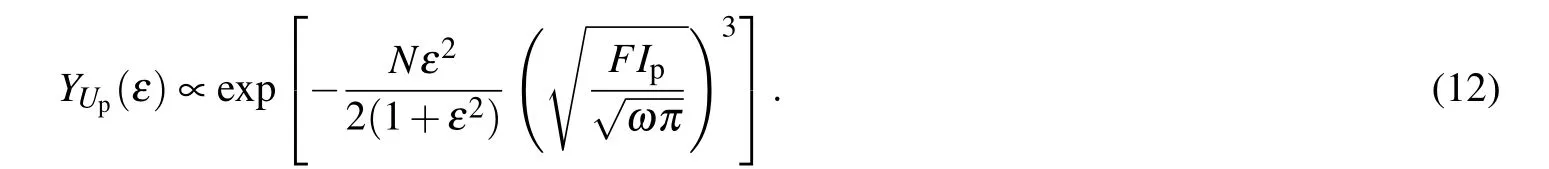
Finally, we calculate the ionization yield YUp,Ist(ε) when the resulting ionization potential Ieffpis fully corrected (based on Eq.(7))

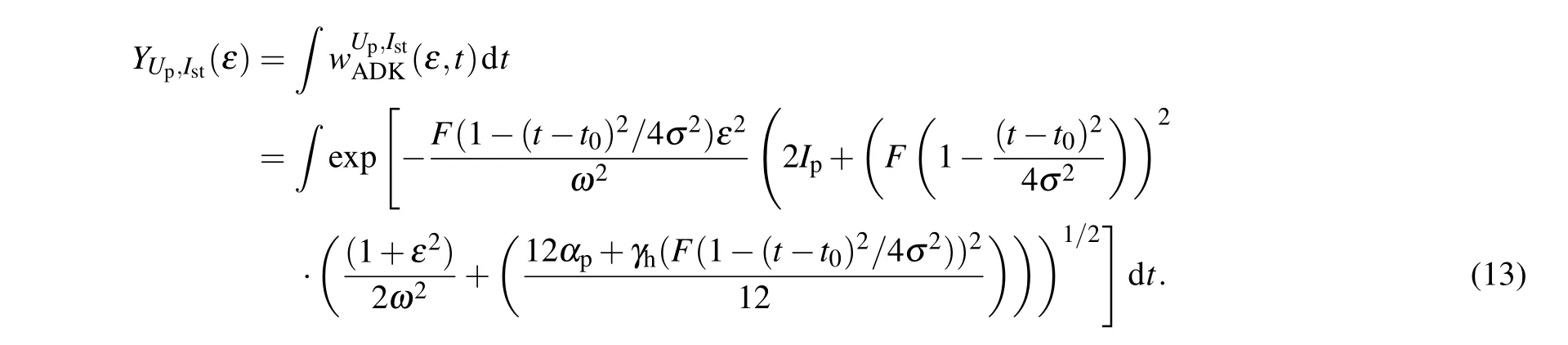
The calculation of the temporal integral in Eq. (13) is performed in a similar manner. Consequently,we obtain

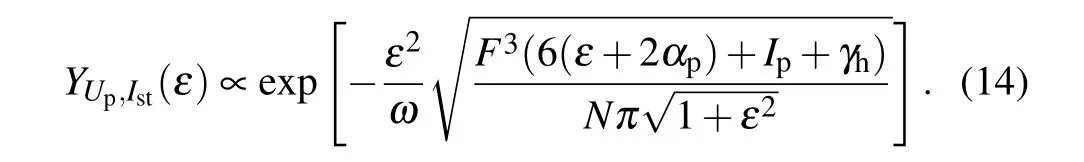
Equations (10), (12), and (14) present the formulas for the ionization yield. Regarding the initial formula(Eq.(1))it can be seen that the exponential dependence is kept,but the timedependent laser field FG(t) and corrected ionization potentialprovide us an additional possibility to analyze the behavior of the ionization yield for an elliptical field polarization.Our theoretical analysis shows that Y(ε),YUp(ε),and YUp,Ist(ε)are very sensitive to the change of frequency ω and laser field strength F. A minimal change of these parameters strongly affects the ionization yield.

3. Results and discussion

In this section,the results of a theoretical investigation of the modified ionization yield have been presented and compared with experimental results (taken from Ref. [17]). We consider the cases of singly ionized noble atoms, argon (Ar),krypton(Kr),and xenon(Xe),which are the most commonly used targets in strong-field studies. This is accomplished by considering a λ =800 nm elliptically polarized laser pulse.The number of optical cycles in the pulse is chosen to match the experiment[17]and it is fixed to the value N =33 with a duration of τ =30 fs. The field intensities I have been varied within the range of 0.8×1014W/cm2-3.0×1014W/cm2.These parameters limit the value of Keldysh parameter in the range which is characteristic for tunnel ionization. The ellipticity varies in the range of ε(0,1). We assume the Gaussian beam profile with a step by step,included fully corrected ionization potential. The effect of the magnetic component can be neglected in the considered intensity range.[41]

We plot the ionization yield as a function of ellipticity ε(two-dimensional(2D)graph),and as a function of both ellipticity ε and field intensity I (three-dimensional (3D) graph).To analyze the influence of the ponderomotive and Stark shift effects on the ionization yield, we include them sequentially(step by step)based on Eqs.(10),(12),and(14). In Fig.1,for Xe atom,we display a comparative review of the yields Y(ε),YUp(ε), and YUp,Ist(ε) with the unperturbated, with the ponderomotive, and fully corrected ionization potential, respectively.

Fig. 1. Comparative review of the ionization yield Y(ε), YUp(ε), and YUp,Ist(ε)for Xe atom as a function of ellipticity ε(0,1),when the laser intensity:(a)is fixed to the value I=1.1×1014 W/cm2,and(b)varies within the range I=0.8×1014 W/cm2-3.0×1014 W/cm2.

At first glance,it is noticeable that all curves from Fig.1 approach to the ellipticity axis with similar asymptotic slopes.The curve which includes the influence of the ponderomotive potential (the dashed line in Fig. 1(a)) has almost the same“flow”as the curve with uncorrected ionization potential Y(ε)(the solid line in Fig.1(a)).Our results suggest that for ε ＞0.4,inclusion of the mentioned effect causes a decrease in yield and also a shift through the lower ellipticities. This is completely in accordance with the fact that the influence of the ponderomotive potential grows in a nearly circularly polarized laser field.[41]Meanwhile, as the ellipticity increases, significant deviation of the curve with the fully corrected ionization potential YUp,Ist(ε) occurs (the dotted line in Fig. 1(a)). One can observe that this curve is shifted vertically downward for laser ellipticity below 0.2. This shift could be due to the effect of the influence of the incorporated corrected ionization potential, which is completely in accordance with theoretical predictions.[42]Now,it can be noted that the ground state shift caused by the ponderomotive and the Stark effects cannot be neglected.We find that the shape of the curves is in accordance with Refs.[17]and[43].

To obtain a more complete analysis, in Fig. 2 we give a comparative review of the ionization yield without any correction Y(ε), with included correction of the ponderomotive potential YUp(ε),and with fully corrected ionization potential YUp,Ist(ε), for Ar, Kr, and Xe atoms. We plot the ionization yield based on Eqs.(10),(12),and(14)as a function of ellipticity ε.

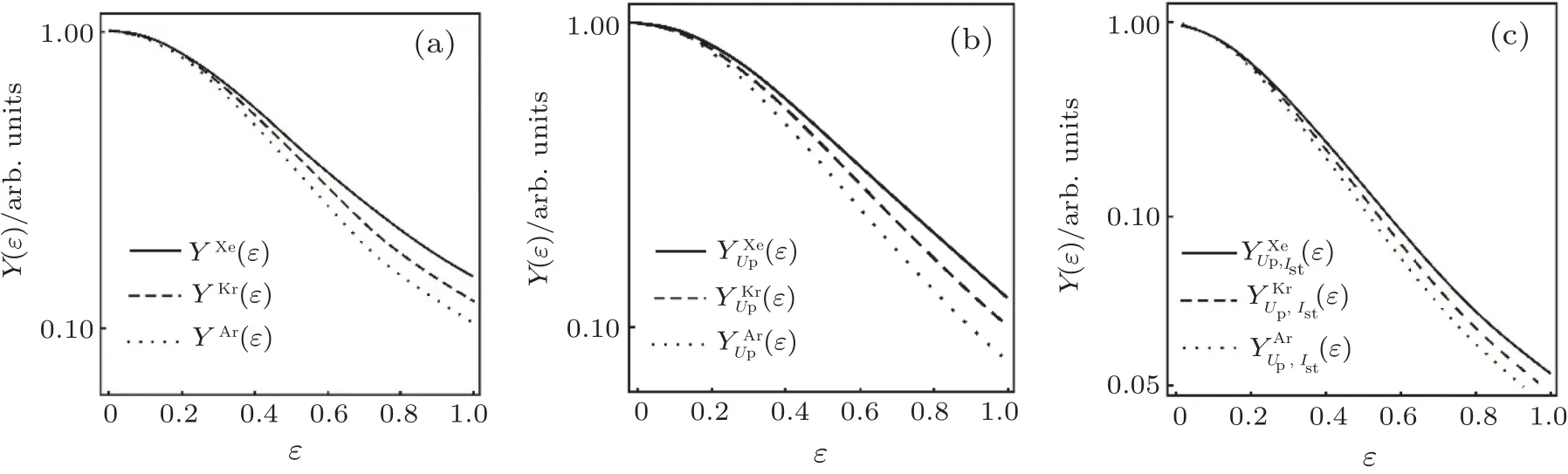
Fig.2. Comparative review of the ionization yield as a function of ellipticity ε(0,1)of: (a)Y(ε),(b)YUp(ε),and(c)YUp,Ist(ε),when the laser intensity is fxied to the value: I=3×1014 W/cm2. The following notation is used for all the three panels: solid line is for Xe atom,dashed line is for Kr atom,and dotted line is for Ar atom.

From Fig.2,it can be seen that the ionization yield curves decrease from Xeto Ar atoms. This order is completely expected since based on Eqs.(10),(12),and(14),the ionization yield decreases exponentially(for fixed values of the field intensity) much more quickly for an atom with higher ionization potential Ip,dipole polarizability αp,and dipole hyperpolarizability γh. It should be noted that the values of the unperturbed ionization potential Ipof Ar atom (Ip=0.579 a.u.)and Xe atom (Ip=0.445 a.u.) differ by more than an order(0.134 a.u.),while for the Kr atom this parameter value lies in between Ar and Xe atoms(Ip=0.514 a.u.). Additionally,the dipole polarizability αpand hyperpolarizability γhhave different values for the corresponding noble atoms: αp∼11 and γh∼1170 for Ar atom, and αp∼16.8 and γh∼2600 for Kr atom, and αp∼27.1 and γh∼6888 and for Xe atom.[40]A closer inspection of our results shows that for a fixed laser intensity, the ellipticity dependence of ionization yields is more pronounced for atoms with a higher ionization potential Ip,dipole polarizability αp,and dipole hyperpolarizability γh,which agrees with experimental and theoretical findings.[44,45]Consequently, it appears natural that the Ar ionization yield curves are more strongly influenced than Kr and Xe ionization yield curves.

Some experimental results[17,46,47]are available for noble gases and our theoretically obtained results can be compared with them.

A comparison between experimental data (taken from Ref. [17]) and the analytically obtained curve given by Eqs. (10) and (14) for the case of Ar and Xe atoms is shown in Fig.3. The intensity and laser frequency used in the figure are chosen for the purpose of comparison with the experiment given in Ref.[17]. It is found that as the ellipticity increases,the ratio of both ionization yield curves Y(ε) and YUp,Ist(ε)decreases rapidly and this feature is in good agreement with Refs. [46]and [47]. The case of ε =0 corresponds to linear polarization and for this value,the experimentally obtained ionization yield Y(ε)exptis nearly the same as the theoretically obtained Y(ε)and YUp,Ist(ε). In some definite range of ε, the ionization yield curves decrease monotonically until a minimum is obtained when ε =1.0,which corresponds to circular polarization.

Based on Figs.3(a)and 3(b),it is obvious that for ε ＞0.2,the ionization yield curve which corresponds to the case of unperturbated ionization potential Y(ε)deviates slightly but noticeably from the experimentally measured ion yield Y(ε)exptand the yield with perturbated ionization potential YUp,Ist(ε).As seen in Fig. 3, a good agreement between the experimental measurements Y(ε)exptand our calculations YUp,Ist(ε) can be accomplished. This result indicates that the ionization potential corrected by the ponderomotive and Stark shift clearly plays a role in achieving better agreement with experimental results. Similar conclusions regarding the comparison between experiments and theories can be drawn for other noble gases and different values of the laser field intensity I.

Fig.3. Ionization yields as a function of ellipticity ε(0,1)for: (a)Ar atom,when the laser intensity is fixed to the value: I=0.8×1014 W/cm2;(b)Xe atom,when the laser intensity is fixed to the value: I=0.5×1014 W/cm2.

4. Conclusion and perspectives

In summary, we have theoretically investigated the photoionization of noble-gas atoms in an elliptically polarized laser field by including the perturbated ionization potential.We observed the ionization yield and compared it with the experimental findings. Our results show that the ionization yields decrease as the ellipticity increases, and the drop becomes more dramatic for an atom with a higher ionization potential Ip, dipole polarizability αp, and dipole hyperpolarizability γh. As demonstrated by our work,the inclusion of the perturbated ionization potential in the standard ADK formula improves agreement with experimental results. To conclude,the presented theory provides an accurate and efficient theoretical model for calculating the ionization yields of noble atoms.The described model can be extended to other atoms with ease and the work is in progress,which can further test the validity of the presented model.