基于时间-空间谱配法的分数阶微分方程的一种解法

王龙,赵丹



基于时间-空间谱配法的分数阶微分方程的一种解法

王龙,赵丹

四川职业技术学院, 四川 遂宁 629000

随着分数阶微分方程的应用领域越来越广泛，相应的理论研究也变得更加重要。本文针对时间分数阶的经典微分方程，提出一种加入空间谱配的解法。通过对时间分数阶经典微分方程的推导，得出等价的微分方程并获取空间配置点，然后应用高斯积分公式转变空间，求出转换方程的积分项。数值验算结果表明：采用时间-空间谱配法得出的精确解与数值解吻合程度较好，基本能满足分数阶微分方程高精度近似解的要求。

分数阶微分方程; 时间-空间谱配法; 精确解; 数值解

分数阶微分方程已经有300多年历史，但一直以来关于它的研究只停留在纯数学领域。近三十年以来，分数阶微分方程开始被广泛应用于光学、生物学、热学、流变学、金融学和物理学等领域，受到了人们的关注。关于分数阶微分方程的精确解求得非常不容易，在实际应用中一般只需要精度较高的近似解即可，因此高精度近似解的求得具有较大的实用价值。Amele等人提出了分数阶微分方程的一种扩散形式，主要是在时空方向上形成一阶差分，但需要具备稳定条件[1]。Temirkhan等人对基于时间分数阶的扩散方程进行求解，该方法收敛性的推导主要基于先验误差的估算[2]。Ghomanjani通过有限元对时间与空间分数阶的微分方程进行求解[3]。Mohammed等人使用有限差分法对时间分数阶微分方程进行求解，然后给出了方程误差的估计[4]。本文在时间分数阶经典微分方程的基础上加入了空间谱配，通过时间-空间谱配法研究了分数阶微分方程的一种解法。

1 时间分数阶的经典微分方程

在上式中，(,)代表粒子的概率密度函数，该粒子的位置是，时间是。代表粒子质量。1代表粒子与环境的摩擦系数。1为扩散函数。

时间分数阶外场存在反常扩散，为了描述该情景，需要考虑用以下的时间分数阶微分方程：

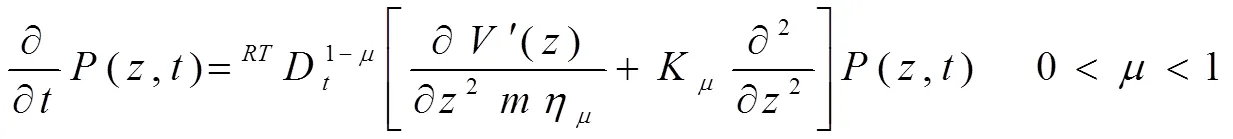
满足以上方程的初始条件：(,0)=()，<<。满足以上方程的边界条件：(,)=1()，(,)=2()，0<<。

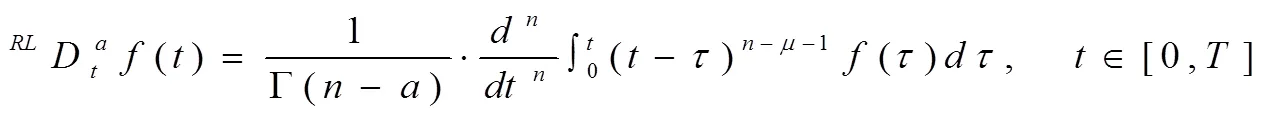
在上式中，代表正整数，而且0≤-1<≤。

2 基于时间-空间谱配法的分数阶微分方程求解过程

2.1 求解过程

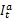
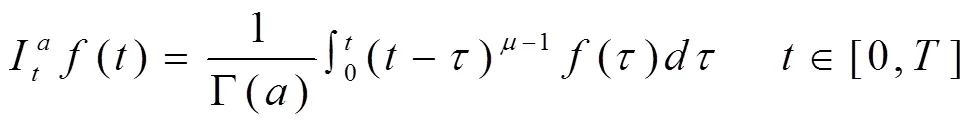
在上式中，代表正整数，且0≤-1<≤。

由于IµtDµtZ()=()-(0)，上式也可以用以下方程表示：

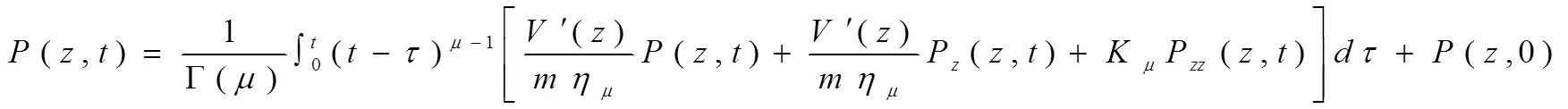
(,)相应初始条件：(,0)=()，<<；边界条件：(,)=1()，(,)=2()，0<<。为了使正交多项式，进行以下变量转换：

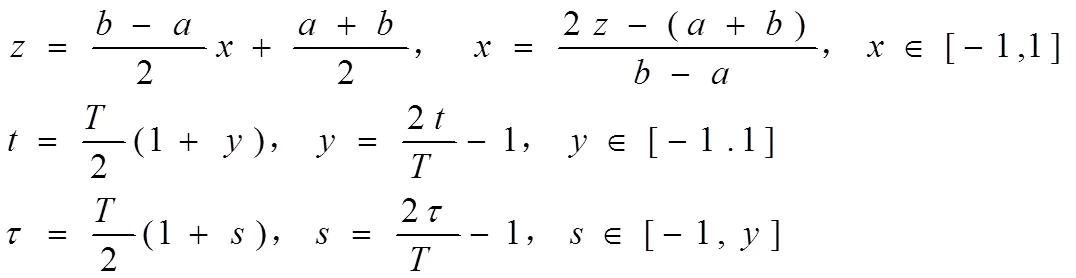
为更好地应用高斯积分公式，需要作出空间的线性改变，从空间[-1,y]转变到空间[-1,1]：

最后配置空间，在[-1,1]中获取配置点{x}，使式（12）在配置点成立，得出下式：

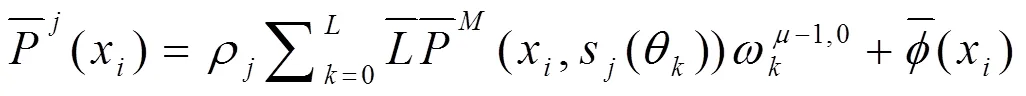
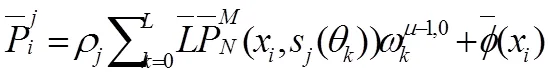
2.2 数值验算

为验证本文提出的基于时间-空间谱配法的分数阶微分方程求解有效性，采用以下算例。

例1：计算分数阶微分方程数值解与精确解的吻合情况。设方程如下：

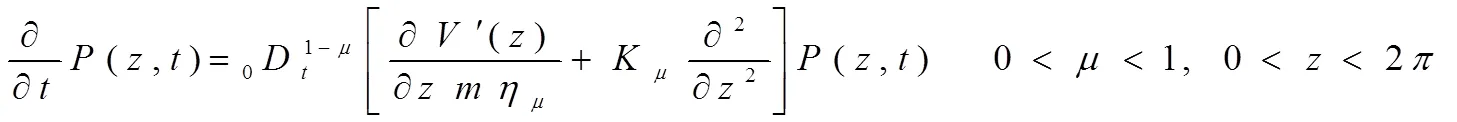
当例1中的=0.9，精确解与数值解情况如图1所示。当=0.8，=1或者=0.9202，精确解与数值解比较如图2所示。根据以上数值验算，采用时间-空间谱配法得出的精确解与数值解吻合程度较好，基本能满足分数阶微分方程高精度近似解的要求。

图 1=0.9时的精确解与数值解

Fig.1 Exact and numerical solutions for=0.9

图 2 左图=1、右图z=0.9202，精确解与数值解的比较

3 讨论

随着分数阶微分方程的使用领域日益扩大，关于方程解的研究也越来越多，大多数研究都是围绕方程解的唯一性和存在性而展开的。对于线性分数阶微分方程的求解，Hamani等提出要借助特殊函数工具进行方程解的探索[5]。对于非线性分数阶微分方程的求解，Doan等认为需要将原方程转化为等价的微积分方程，在适当空间中使用不动点定理[6]。Hassan等围绕时间分数阶的微积分方程求解过程，认为要适当考虑函数空间的问题，这有利于提高数值解的精确性[7]。相对来说，分数阶微分方程的理论研究滞后于实践应用，但随着学者们的广泛重视，在理论研究方面将会有较大的进展。

4 结论

针对分数阶微分方程高精度近似解求得问题，提出一种基于时间-空间谱配法的解法，使时间分数阶的经典微分方程能够在空间方向的配置点成立，形成全离散格式，但要满足配置点的要求。通过数值验算，求得的数值解较好地接近精确解。本文解法的优势在于能够通过较少的配置点得出精度较高的数值解，在节省储存空间的同时，还能求解较为复杂的分数阶微分方程。

[1] Taieb A, Dahmani Z. A new problem of singular fractional differential equations[J]. Journal of Dynamical Systems and Geometric Theories, 2016,14(2):165-187

[2] Aleroev T, Kekharsaeva E. Boundary value problems for differential equations with fractional derivatives[J]. Integral Transforms and Special Functions, 2017,28(12):900-908

[3] Ghomanjani F. A new approach for solving fractional differential-algebraic equations[J]. Journal of Taibah University for Science, 2017,11(6):1158-1164

[4] ALHorani M, Khalil R. Total fractional differentials with applications to exact fractional differential equations[J]. International Journal of Computer Mathematics, 2018,95(6):1444-1452

[5] Hamani S, Hammou A, Henderson J. Impulsive fractional differential equations involving the hadamard fractional derivative[J]. Communications on applied nonlinear analysis, 2017,24(3):48-58

[6] Doan TS, Huong PT, Kloeden PE,. Asymptotic separation between solutions of Caputo fractional stochastic differential equations[J]. Stochastic Analysis and Applications, 2018,36(4):654-664

[7] Hassan K, Muhammad AS, Tauseef MD,Numerical solutions to systems of fractional Fredholm Integro differential equations, using Chebyshev wavelet method[J]. Journal of Taibah University for Science, 2018,12(5):584-591

A Solution of Fractional Differential Equations on Time-space Spectrum Allocation Method

WANG Long, ZHAO Dan

629000,

With the application of fractional differential equation more and more widely, the corresponding theoretical research has become more important. In this paper, we propose a solution to the classical differential equation of fractional order in time by adding spatial spectral assignment. By deducing the classical differential equation of time fractional order, the equivalent differential equation is obtained and the spatial collocation points are obtained. Then the integral terms of the transformation equation are obtained by applying the Gauss integral formula to transform the space. The numerical results show that the exact solution obtained by the time-space spectrum matching method is in good agreement with the numerical solution, and can basically meet the requirements of high-precision approximate solution of fractional differential equation.

Fractional differential equations; Time-space spectrum allocation method; exact solutions; numerical solutions

O29

A

1000-2324(2019)01-0142-03

10.3969/j.issn.1000-2324.2019.01.032

2018-02-10

2018-04-20

四川省教育厅一般基金项目:偏微分方程图像分割算法研究(15ZB0358)

王龙(1983-),男,硕士,副教授,主要研究方向为偏微分方程，数学建模，应用数学. E-mail:wanglongzhujun@163.com