基于LQ优化的航空交通管理

杨守剑

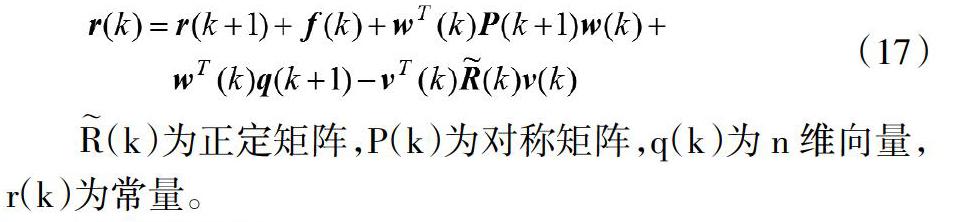
摘 要：随着航空交通流量的增长，通过对交通流进行的改进，后与元胞理论结合，改进了一维元胞传输。接着，再应用线性二次型（Linear-Quadratic）最优控制的理论对空中流量模型进行分析，实现对某区域的流量进行预测。最后，利用Matlab对系统进行了仿真。模型在仿真过程中考虑了扰动输入，流量更新的随机性，对不同的流动比例系数下，获得的控制序列与反馈增益进行了比较。结果显示某个空域范围的交通流量得到了改善。

关键词：空中交通管理；最优控制；线性二次型

中图分类号：F562.1 文献标志码：A 文章编号：2095-2945（2018）35-0189-02

Abstract： With the increase of air traffic flow， the one-dimensional cellular transmission is improved by improving the traffic flow and combining with the cellular theory. Then， the Linear-Quadratic optimal control theory is applied to analyze the air flow model to predict the flow in a certain area. Finally， the system is simulated by Matlab. In the process of simulation， the randomness of disturbance input and flow update are considered， and the control sequence and feedback gain are compared under different flow ratio coefficients. The results show that the traffic flow in a certain airspace is improved.

Keywords： air traffic management； optimal control； linear quadratic form

近年隨着航空交通流量不断增加，航空流量控制问题引起的延误也不断增长。故对航空交通流量的研究变得很重要。在交通流研究领域， 现在广泛存在的研究方法有跟驰模型、连续模型、分子动力论方法和元胞自动机方法等[1]。1955年，Whitham和Lighthill首先提出交通流动力学理论[2]，同时Richards提出相对独立理论[3]，这三个人提出的理论被称为LWR理论。本文试着对LWR理论分析改善，建立改进的一维元胞模型。

3 系统仿真

选定某空域，其初始条件为：航空交通流平均速度是v=250节，物理尺寸为L=70海里。于是时间步长？子=0.25小时（设此时间为时间单元），即基础处理时间单元是0.25小时。

根据对模型初始状态的设定，利用MATLAB语言[4]对模型进行仿真， 结果为：未引入控制向量的流量的20次采样总数为182，引入控制向量的流量的20次采样总数为241，使流量总数降低了24.5%。做到了对飞行流量的初步控制。

4 结束语

经过LQ最优控制后，模型中的元胞单元中的流量有所减少。验证了本文提出的理论能有效提高了效率，从而使空中交通运行更顺畅。

参考文献：

[1]顾国庆，戴世强. 交通流动力学[J].自然杂志，2003，9：198-203.

[2]Lighthill M H， Whitham G B. On kinematics wave： Ⅱ A theory of traffic flow on long crowed roads[M]. Proc R Soc London， Ser A， 1955，22：317-345.

[3]Richards P I. Shock waves on the highway[M]. Opns Res， 1956，4：42-51.

[4]朱衡君.MATLAB语言及实践教程[M].北京：清华大学出版社，北京交通大学出版社，2004.