厄米-高斯光束在饱和非线性介质中的传输特性

姜其畅，苏艳丽，聂合贤，马紫微，李永宏

(运城学院 物理与电子工程系，运城 044000)

厄米-高斯光束在饱和非线性介质中的传输特性

姜其畅，苏艳丽，聂合贤，马紫微，李永宏

(运城学院 物理与电子工程系，运城 044000)

为了研究厄米-高斯光束在光折变饱和非线性介质中的传输特性，采用有限差分方法数值求解了光波演化方程,理论分析了厄米-高斯光束的传输特性。结果表明，1维1阶、2阶和3阶厄米-高斯光束在光折变非线性介质中传输时，在合适的非线性条件下，均可以形成呼吸模式的孤子；随着非线性的加大，厄米-高斯光束的光场分量之间的相互分离趋势将逐渐变弱，同时，每个光场分量的振幅起伏效应会更加明显；改变厄米-高斯光束的入射位置、入射角度对其传输特性没有影响；2维厄米-高斯光束的传输特性和1维情况是类似的。厄米-高斯光束的这些特性在光开关领域有一定的应用前景。

非线性光学；厄米-高斯光束；光折变效应；空间孤子

引 言

1998年，CASPERSON和TOVAR给出了近轴近似直角坐标下亥姆霍兹方程的一类特解，即所谓厄米-正弦类-高斯光束，它是具有广泛代表性意义的一类光束[1-5]。厄米-高斯光束被认为是厄米-正弦类-高斯光束的特例。目前，人们对厄米-高斯光束的研究重要集中在两个方面，一方面是研究厄米-高斯光束对各种介质圆柱、介质球的散射问题[6-8];另一方面是研究厄米-高斯光束在大气湍流[9-11]和非线性介质[12-15]中的传输问题。近几年，人们报道了厄米-高斯光束在强非局域非线性介质中的传输特性,发现厄米-高斯光束可以在一定条件下形成厄米-高斯孤子，由于厄米多项式的调制作用，厄米-高斯孤子相比于传统的基模高斯孤子[16-17]表现出更丰富的传输特性，但是关于厄米-高斯光束在光折变饱和非线性介质中的传输特性还少有相关报道。本文中借助光波演化的非线性薛定谔方程，数值研究了厄米-高斯光束在光折变饱和非线性介质中的传输特性。

1 基本理论

考虑厄米-高斯光束在光折变晶体中沿z轴传输，其偏振沿x方向；假定光束只在x方向衍射，晶体光轴和外加电场均沿x方向。为了便于数值分析，取归一化的坐标参量s=x/x0，ξ=z/(kx02)。其中，x0是任意空间宽度，k=k0ne=(2π/λ0)ne，λ0是自由空间波长，ne是未受扰动的非寻常光折射率。光波传输的归一化方程可以表示为:

式中，U(s,ξ)是归一化光波包络，非线性系数β=(k0x0)2(ne4r33/2)E0，r33是电光系数，E0是外加电场强度。入射面处的厄米-高斯光束可以表示为:

式中，Hn是厄米多项式，n表示厄米多项式的阶数。如果x0=w0=50μm(w0表示高斯光束的光斑大小)，λ0=0.5μm，相应的高斯光束的瑞利距离ZR=πw02/λ0=15.7mm。下面基于(1)式和(2)式数值分析各阶厄米-高斯光束的传输特性。

2 数值结果

当厄米多项式H0=1时，(2)式即退化为基模高斯光束的表达式。如果外加电场为零，则非线性参量β=0，可得到图1a所示结果，图中横纵坐标均是归一化的无量纲坐标。ξ=1对应实际传输距离大约是70mm，可以看到超过瑞利距离(约ξ=0.2)，由于光束的衍射效应，高斯光束的光斑能量逐渐弥散。为了抑制光束的这种衍射效应，增加外加电场强度，当非线性系数β=5.5，基本可以抑制光束的衍射，形成呼吸模式的高斯孤子，为了更清楚看到其呼吸模式，可以将传输距离增加到ξ=6，如图1b和图1c所示。

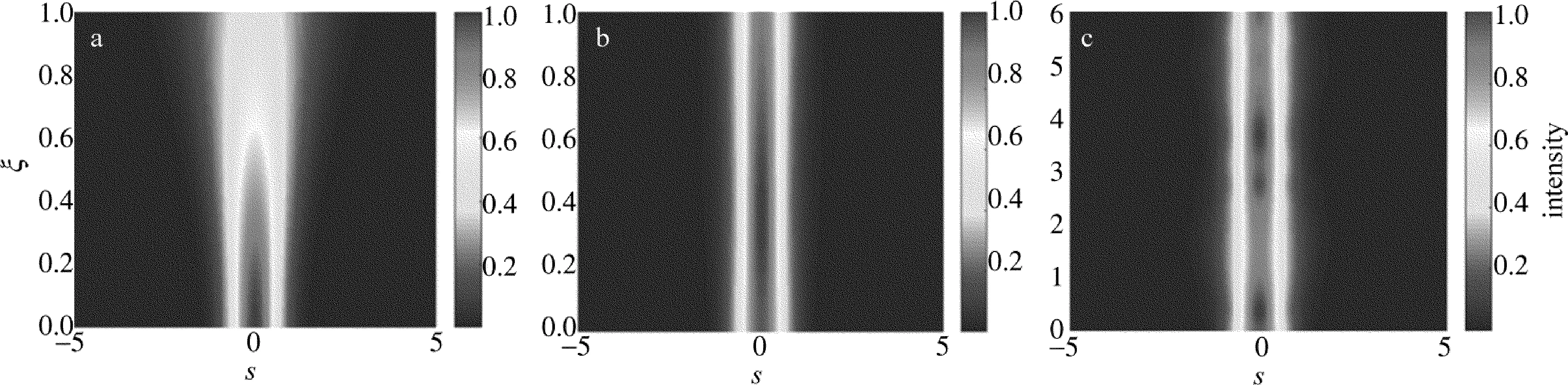
Fig.1 Natural diffraction and soliton propagation of fundamental-mode Gaussian beama—β=0 b—β=5.5 c—β=5.5

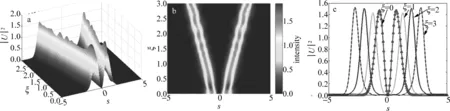
Fig.2 Propagation of first-order Hermite-Gaussian beam

如果加大非线性即加大外加电场，两个光场分量之间的分离距离可以明显减小，但是相应的每个光场分量的起伏效应会显著加大，如图3所示。通过改变外加电场的大小可以灵活调节两个光场分量之间的距离，从而可以控制出射面某一点处光信号的有无，这在光开关领域有一定的应用前景。

同样，当厄米多项式取H2=8s2-2时，(2)式就是2阶厄米-高斯光束的表达式。此时，在初始入射面，2阶厄米-高斯光束呈现对称的3个光场分量，取合适的外加电场强度如β=15，同样可以形成呼吸模式的孤子，中间光场分量直线传输，两侧的光场分量彼此分离，而且分离的距离随着传输距离的加大而增加；当外加电场强度加大时，比如β=25，两侧光场分量之间的分离距离明显减小，但是3个光场分量振幅的起伏效应会更加明显(见图4)。

Fig.3 Propagation of the first-order Hermite-Gaussian beam at β=40

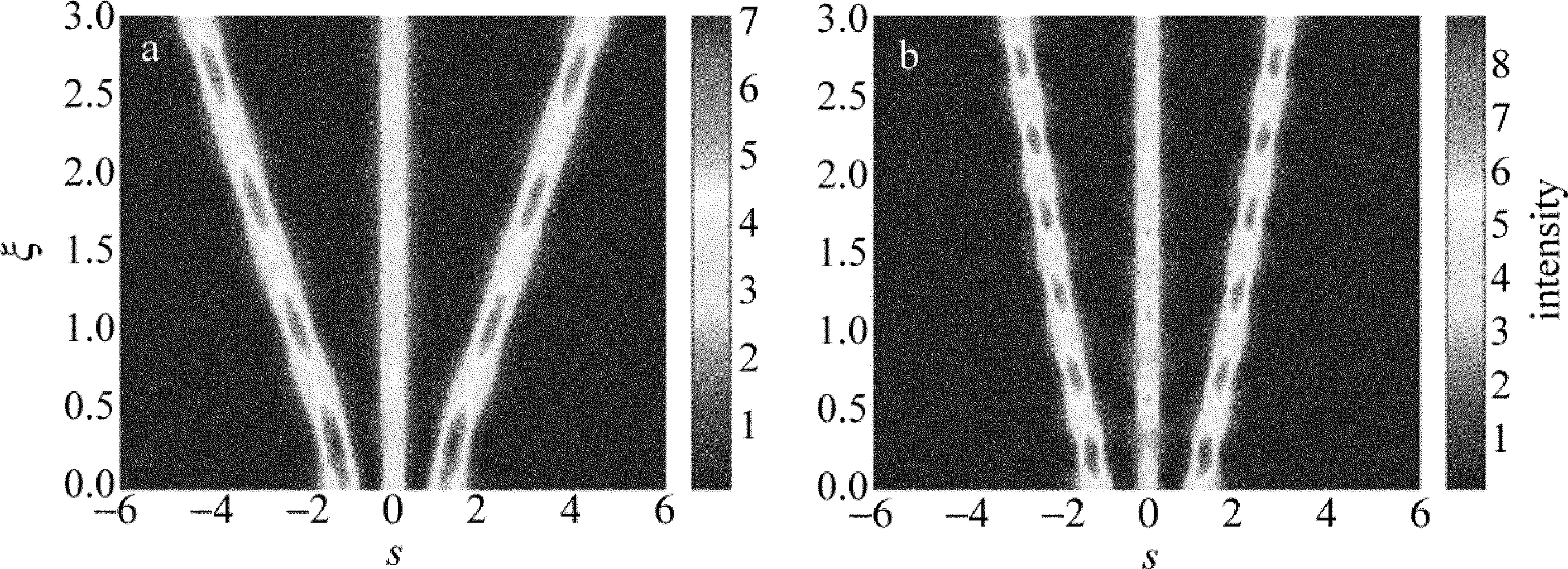
Fig.4 Propagation of the second-order Hermite-Gaussian beam under different nonlinear conditions

Fig.5 Propagation of the third-order Hermite-Gaussian beam under different conditions

光波传输的归一化方程(1)式在2维情况时可以表示为[15]:

式中，sx=x/x0，sy=y/x0，分别是x方向和y方向的归一化坐标。其它参量的意义和(1)式相同。这里以2阶厄米-高斯光束为例，2维厄米-高斯光束表示为U(sx,sy,0)=(8sx2-2)(8sy2-2)exp(-sx2-sy2)，同样取非线性参量β=15，2维厄米-高斯光束在ξ=0，ξ=1.5和ξ=3处的横截面强度分布如图6所示。可以看到，在合适非线性条件下，2阶厄米-高斯光束的每一个光场分量都以呼吸模式的孤子形式传输，而且随着传输距离的加大，各个光场分量之间的距离会逐渐增加。

Fig.6 Propagation of 2-D second-order Hermite-Gaussian beam

3 结 论

分析了1阶、2阶和3阶厄米-高斯光束在光折变饱和非线性介质中的传输特性，在合适的外加电场强度条件下，厄米-高斯光束的各个光场分量都可以形成呼吸模式的孤子，各个光场分量之间的分离距离可以由外加电场强度灵活操控。厄米-高斯光束的这些特性在光开关领域有一定的应用前景。

[1] CASPERSON L W, TOVAR A A. Hermite-sinusoidal-Gaussian beams in complex optical systems [J]. Journal of the Optical Society of America, 1998, A15(4):954-961.

[2] TOVAR A A, CASPERSON L W. Production and propagation of Hermite-sinusoidal-Gaussian laser beams [J]. Journal of the Optical Society of America, 1998, A15(9):2425-2432.

[3] ZHAO D, MAO H, LIU H. Propagation of off-axial Hermite cosh-Gaussian laser beams [J]. Journal of Optics, 2004, A6(1):77-83.

[4] YU S, GUO H, FU X,etal. Propagation properties of elegant Hermite-cosh-Gaussian laser beams [J]. Optics Communications, 2002, 204(1/6):59-66.

[5] ZHAO Q, HAO H Y, FAN H Y,etal. Focusing characteristics of partially coherent cosh-Gaussian beams propagating through turbulent atmosphere [J]. Laser Technology, 2016, 40(5):750-755 (in Chinese).

[6] YOKOTA M, KUDOU T, FUKUMITSU O. High-frequency scattering of a Hermite-Gaussian beam by a perfectly conducting cylinder [J]. Electronics Letters, 1987, 23(4):174-175.

[7] YOKOTA M, HE S, TAKENAKA T. Scattering of a Hermite-Gaussian beam field by a chiral sphere [J]. Journal of Optical Society American, 2001, A18(7):1681-1689.

[8] QU T, WU Zh S, SHANG Q Ch,etal. Far-field scattering of a chiral sphere located in a Hermite-Gaussian beam [J]. Acta Optica Sinica, 2016, 36(4):0429001 (in Chinese).

[9] JI X L, CHEN X W, LÜ B D. Spreading and directionality of partially coherent Hermite-Gaussian beams propagating through atmospheric turbulence [J]. Journal of the Optical Society of America, 2008, A25(1):21-28.

[10] JI X L, LI X Q. Effective radius of curvature of partially coherent Hermite-Gaussian beams propagating through atmospheric turbulence [J]. Journal of Optics, 2010, 12(3):035403.

[11] HUANG Y P, GAO Z H, WANG F H,etal. The effective radius of curvature of partially coherent Hermite-Gaussian linear array beams passing through non-Kolmogorov turbulence [J]. Optics Communications, 2014, 315(19):130-137.

[12] DENG D, ZHAO X, GUO Q,etal. Hermite-Gaussian breathers and solitons in strongly nonlocal nonlinear media [J]. Journal of the Optical Society of America, 2007, B24(9):2537-2544.

[13] WANG Q, LI J Z. Elliptic Hermite-Gaussian soliton in anisotropic strong nonlocal media [J]. Optics Communications, 2016, 359:31-37.

[14] ZHONG L H, YANG J, REN Z M,etal. Hermite-Gaussian stationary solutions in strongly nonlocal nonlinear optical media [J]. Optics Communications, 2017, 383:274-280.

[15] LI Sh H, YANG Zh J, LU D Q,etal. Numerical study of Hermite-Gaussian beams in nonlocal thermal media [J]. Acta Physica Sinica, 2011, 60(2):024214 (in Chinese).

[16] KANG J, TANG Y L, LI D Y,etal. Propagation characteristics of Gaussian beam in logarithmically nonlinear media [J]. Laser Technology, 2000, 24(2):118-121 (in Chinese).

[17] SU Y L, JIANG Q C, JI X M,etal. The temperature properties of matching Gaussian beam in biased two-photon centrosymmetric paraelectric photorefractive crystals [J]. Optics and Quantum Electronics, 2012, 44(14):649-655.

PropagationcharacteristicsofHermite-Gaussianbeaminsaturablenonlinearmedia

JIANGQichang,SUYanli,NIEHexian,MAZiwei,LIYonghong

(Department of Physics and Electronic Engineering, Yuncheng University, Yuncheng 044000, China)

In order to study propagation properties of Hermite-Gaussian beams in photorefractive saturable nonlinear media, finite difference method was used to solve the evolution equation of light wave numerically and analyze the propagation properties of Hermite-Gaussian beams theoretically. The results show that, under suitable nonlinear conditions, 1-D Hermite-Gaussian beams of 1-order, 2-order and 3-order can form the solitons in respiratory mode during the propagation in photorefractive nonlinear media. With the increase of nonlinearity, the separation tendency among light field components of Hermite-Gaussian beams would become weaker. At the same time, the amplitude fluctuation effect of each light field component would be more obvious. The changes of incident position and incident angle of Hermite-Gaussian beams have no influence on its propagation characteristics. The transmission characteristics of 2-D Hermite-Gaussian beams are similar to those of 1-D. These properties of Hermite-Gaussian beams have certain application prospects in the field of optical switching.

nonlinear optics; Hermite-Gaussian beam; photorefractive effect; spatial solitons

1001-3806(2018)01-0141-04

山西省自然科学基金资助项目(2011011003-2)

姜其畅(1980-),男，博士，副教授，主要从事非线性光学和光场调控方面的研究。

E-mail:jiangsir009@163.com

2017-02-13；

2017-03-17

O437.5

A

10.7510/jgjs.issn.1001-3806.2018.01.028