Thermal-structural analysis of regenerativelycooled thrust chamber wall in reusable LOX/Methane rocket engines
Jiawen SONG,Bing SUN
School of Astronautics,Beihang University,Beijing 100083,China
Thermal-structural analysis of regenerativelycooled thrust chamber wall in reusable LOX/Methane rocket engines
Jiawen SONG,Bing SUN*
School of Astronautics,Beihang University,Beijing 100083,China
Available online 6 May 2017
*Corresponding author.
E-mail address:sunbing@buaa.edu.cn(B.SUN).
Peer review under responsibility of Editorial Committee of CJA.
Production and hosting by Elsevier
http://dx.doi.org/10.1016/j.cja.2017.04.007
1000-9361©2017 Production and hosting by Elsevier Ltd.on behalf of Chinese Society of Aeronautics and Astronautics.
This is an open access article under the CC BY-NC-ND license(http://creativecommons.org/licenses/by-nc-nd/4.0/).AbstractTo predict the thermal and structural responses of the thrust chamber wall under cyclic work,a 3-D fluid-structural coupling computational methodology is developed.The thermal and mechanical loads are determined by a validated 3-D finite volume fluid-thermal coupling computational method.With the specif i ed loads,the nonlinear thermal-structural finite element analysis is applied to obtaining the 3-D thermal and structural responses.The Chaboche nonlinear kinematic hardening model calibrated by experimental data is adopted to predict the cyclic plastic behavior of the inner wall.The methodology is further applied to the thrust chamber of LOX/Methane rocket engines.The results show that both the maximum temperature at hot run phase and the maximum circumferential residual strain of the inner wall appear at the convergent part of the chamber.Structural analysis for multiple work cycles reveals that the failure of the inner wall may be controlled by the low-cycle fatigue when the Chaboche model parameter γ3=0,and the damage caused by the thermal-mechanical ratcheting of the inner wall cannot be ignored when γ3> 0.The results of sensitivity analysis indicate that mechanical loads have a strong influence on the strains in the inner wall.
©2017 Production and hosting by Elsevier Ltd.on behalf of Chinese Society of Aeronautics and Astronautics.This is an open access article under the CC BY-NC-ND license(http://creativecommons.org/licenses/by-nc-nd/4.0/).
Rocket engine;
Thrust chamber;
Regenerative cooling;
Heat transfer;
Mechanical load;
Cyclic plasticity;
Ratcheting
1.Introduction
LOX/Methane Liquid Rocket Engines(LRE)has aroused the interests of many countries in the past several decades.1–5Methane is a hydrocarbon which has many advantages,including low density,non-toxic,and easy to be produced.6The propellant combination of oxygen and methane can provide high performance,high reliability,and low cost.Thus,LOX/Methane engines are considered as one of the candidates for Reusable Launch Vehicle(RLV)propulsion system.7RLV is the focus within the aerospace community in recent decades due to the significant military and civil value.As one of the critical assemblies of LRE,the thrust chamber wall is subjected to huge heat flux from the combustion of propellants,especially in the throat region.In high-thrust LREs such as the Space Shuttle Main Engine(SSME),the pressure of the combustion chamber could exceed 20 MPa,and the heat flux in the throat region is around 160 MW/m2.No material could sustain the huge heat flux without melting.To protect the thrust chamber wall,sophisticated cooling methods must be applied.Regenerative cooling achieved by flowing a liquid propellant into suitable cooling channels machined in the thrust chamber wall is one of the most effective cooling methods.Copper or copperbased alloys are often used as the inner liner of thrust chambers in modern high-thrust LRE due to their high thermal conductivity,which could improve the cooling efficiency of coolants.Meanwhile,such materials own sufficient mechanical strength to support thermal and mechanical loads in thrust chambers.8
The typical cooling channels of regeneratively-cooled thrust chambers are milled in the copper liner and closed out by a copper barrier through an electroforming process,followed by a nickel jacket over the copper barrier.During the operation of LRE,the thrust chamber wall is subjected to the thermal load induced by huge temperature difference across the thrust chamber wall and the mechanical load caused by the pressure difference between the coolant-side wall and the hot-gas-side wall.The stress of the copper liner would be beyond the elastic limit,and severe inelastic strains accumulate in the inner wall.After some cycles,the ‘doghouse” shape failure of cooling channels characterized by the thinning and bulging of the inner wall would occur.9The potential failure modes are creep rupture,low-cycle thermal fatigue and thermal-mechanical ratcheting.8The thermal-structural analysis of the thrust chamber wall is significant for the design of LRE.Much work has been published regarding this urgent problem.To perform the thermal-structural analysis of the thrust chamber wall,both the material properties of the inner liner and outer jacket must be available.Since 1970s,NASA has carried out studies on the candidate materials for the regeneratively-cooled thrust chamber wall including NARLoy-Z,Amzirc,Oxygen-Free High thermal Conductivity(OFHC)copper and electroformed nickel to obtain their physical and mechanical properties.10–12Miller13performed a plane strain finite element analysis for the throat section of thrust chambers to identify the strain range of the thrust chamber wall under cyclic work.The low-cycle fatigue life of the thrust chamber wall was estimated based on isothermal fatigue test data.Hannum et al.14attempted to correlate the predicted life with experimental value,and the predicted lives are much longer than experimentally determined lives.They suggested that the transient feature of heat transfer must be considered,but the comprehensive transient thermal analysis of thrust chambers was limited at that time.Arya and Arnold15applied a viscoplastic model to estimating the deformation behavior of an experimental thrust chamber.The results qualitatively replicated the ‘doghouse” shape failure of the thrust chamber wall.Yang et al.16performed a detailed structural analysis of the thrust chamber wall phase by phase.The numerical results revealed that the startup and shutdown phases have a great effect on the stress and strain evolution.However,in Refs.13–16only 2-D analyses on the specific cross section of the thrust chamber wall were performed,and their time-dependent thermal and mechanical loads have to be obtained in aid of a series of costly experiments.
Armstrong and Brogren17made a comparison between 3-D and 2-D plan-train thermal-structural analysis.The authors concluded that although 2-D model can predict the thermal response at the throat section,only 3-D model could accurately determine the effective strain range of the thrust chamber wall.However,they used relatively coarse mesh in their analyses and admitted that the accuracy of 3-D model was affected by the adopted mesh size.Riccius et al.18conducted a 3-D coupled thermal-structural finite element analysis of a thrust chamber of LRE during a full work cycle.They suggested that the predicted life based on 3-D analysis is longer than that based on 2-D analysis because 2-D analysis neglected axial strain,which resulted in the increase of the deviatoric part of the stress tensor.Cheng et al.19used the 3-D fluid-thermal coupling method and the 3-D thermal-structural coupling analysis technique to investigate the deformation behavior of the nozzle wall.However,the thrust chamber wall was not included in their model.As they indicate,the load conditions of the thrust chamber wall are quite different from those of nozzle wall.
Most studies reviewed above have been carried out for thermal-structural analysis of LOX/LH2 thrust chamber wall,but little work has been published on that of LOX/Methane thrust chamber wall,especially for multiple work cycles.The situation is understandable due to the reusability of SSME,which is a LOX/LH2 rocket engine.On the other hand,LOX/Methane engines have never been used for a practical flight in the world.
To provide a reference for the design of reusable thrust chambers,and further promote the practical use of LOX/Methane engines,the thermal and structural responses of the regeneratively-cooled thrust chamber wall of LOX/Methane rocket engine under cyclic work are obtained by a numerical methodology in this study.The thermal and mechanical loads are determined by a validated 3-D finite volume fluid-thermal coupling computational method.With the specif i ed loads,the nonlinear thermal-structural finite element analysis is applied to obtaining the 3-D temperature and strain characteristics of the thrust chamber wall.To capture the cyclic plastic behavior of the copper liner,the Chaboche nonlinear kinematic hardening model calibrated by experimental data is employed in finite element analysis.With the results of the first work cycle,the 3-D temperature and strain distributions of the thrust chamber wall during one cycle including pre-cooling,hot run,postcooling,and relaxation phases are presented and discussed.With the results of multiple work cycles,the strain evolution of the thrust chamber wall is analyzed in detail to reveal its ratcheting behavior.Furthermore,the influence of mechanical loads on ratcheting behavior of the inner wall is thoroughly investigated.Based on the findings,several approaches are suggested to slow down the progressive thinning and bulging of the inner wall and improve the durability of thrust chambers.
2.Computational methodology
2.1.Computational scheme
The durability of thrust chamber is mainly limited by thermal induced strains of the liner material under cyclic work.Thus,an exact thermal-structural analysis of the thrust chamber wall must be performed in the design phase of reusable LRE.As Fig.1 shows,a precise fluid-thermal calculation for the coupled flow and heat transfer characteristics in the thrust chambers provides the requisite convection and mechanical loads for the thermal-structural analysis of the thrust chamber wall.To make the computation more effective and robust for this study,several assumptions were proposed under the premise of ensuring sufficient accuracy.
(1)The creep of chamber wall materials is not considered because the temperature of the thrust chamber wall is lower than 800 K.The strain of the thrust chamber wall is small enough(<0.1)so that the geometrical nonlinearity can be ignored and engineering strain can be used in computation.This assumption can be justified by the final computational results.
(2)The flow and heat transfer of the hot gas and coolant are not influenced by the deformation of the thrust chamber wall.That means that the convection and mechanical loads of the first work cycle obtained by fluid-thermal coupling calculation can be applied in the successive cycles.
(3)The parameters of chamber wall materials are independent of damage evolution.
2.2.Fluid-thermal coupling calculation
In general,the heat transfer in thrust chambers with regenerative cooling consists of the following three processes:(1)the heat transfer between the hot gas and the hot-gas-side wall,(2)the heat conduction through the thrust chamber wall,and(3)the convective heat transfer between the coolant and coolant-side wall.To precisely obtain the temperatures in the thrust chamber wall,all the processes must be considered.In our published work,20a fluid-thermal coupling calculation method based on Finite Volume Method(FVM)with nonequilibrium chemical reaction was developed to predict the 3-D coupled heat transfer characteristics in regeneratively-cooled thrust chambers.The finite-rate chemical reaction for nonequilibrium hot-gas flow was evaluated by Eddy Dissipation Concept(EDC)model,and radiative heat transfer was solved with Discrete Ordinates(DO)model.In this method,Reynolds Stress Model(RSM)is used to close the Reynolds-averaged Navier-Stokes equations,and SIMPLE algorithm is employed to treat the pressure-velocity coupling.The coupling of the heat transfer between fluidand solid domains was achieved by a manually iterative procedure.The procedure can enforce the continuity of temperature and heat flux to maintain energy balance.A complete introduction about the fluid-thermal coupling calculation method can be found in Ref.20.
The method was validated20in comparison with experimental data.The results suggest that the method is a comprehensive and efficient method for predicting the temperatures in the thrust chamber wall.With the fluid-thermal coupling calculation method,the convection and mechanical loads on the fluid-solid interface surface can be thoroughly obtained.Therefore,the method was applied in this study to determine the loads for the thermal-structural analysis of the thrust chamber wall.
It should be noted that the transcritical flow behavior of methane in cooling channels results in dramatic changes of thermophysical properties even with small changes of temperature and pressure.To deal with it,thermophysical properties of the coolant must be accurately predicted and embedded in the FVM solver.The calculation methods in Ref.20were used to calculate the thermophysical properties of the coolant in this study,including the density,constant-pressure specific heat,viscosity,and thermal conductivity.Although those methods have been employed initially for the prediction of supercritical hydrogen,the accuracy for transcritical methane has been demonstrated in Ref.21.
2.3.Thermal-structural analysis
2.3.1.Governing equation
The transient heat conduction of the thrust chamber wall is governed by
where ρ is density,cspecific heat capacity,kthermal conductivity,Ttemperature,andttime.In this study,ρ,candkare the material properties of the thrust chamber wall,which are determined by the self of materials and vary with temperature.
For the case of classical elastic-plastic analysis,the definition of total strain given with Eq.(2)has the form of
where ε is total strain vector,εththermal strain vector,εelelastic strain vector,and εplplastic strain vector.Since thermal expansion of materials only causes normal strains,the thermal strain vector for isotropic materials is
where α is isotropic secant coefficients of thermal expansion,Tcurrent temperature,andTrefreference temperature.Elastic strain vector is related to the stress by generalized Hooke’s law:
whereDis elastic stiffness matrix,and σ stress vector.
The constitutive model for the cyclic plastic behavior of materials adopted in this study is a rate-independent version of the kinematic hardening model proposed by Chaboche.22The constitutive equations are based on a von Mises yield function,the associated flow rule,and the nonlinear kinematic hardening law.The stress level at which yielding is initiated is defined by von Mises yield criterion which is given by
whereFdenotes the yield function,sdeviatoric stress tensor,α back stress tensor,and σymaterial yield stress.In this paper,three independent back stress tensors are superposed to model the translation of yield surface.Thus,the back stress tensor is given as
The evolution of the back stress tensor for each component is given by Armstrong-Frederick kinematic hardening rule:
whereCirepresent the plastic moduli;γiare the parameters for history dependence,and γi=0 stands for the linear kinematic hardening rule;˙εpldenotes plastic strain rate;˙¯εplis the magnitude of plastic strain rate.Because the dynamic recovery term(γi˙¯εplαi)is included in the expression of back stress tensor,the ratcheting behavior could be simulated.In Eq.(7),Ciand γiare the material parameters determined by curve fitting processes based on uniaxial stress-strain experimental data.
The numerical discretization and solving of the governing equations were achieved by FEM based ANSYS Parametric Design Language(APDL).23Because of the nonlinear relationship between stressand strain,coupling with the temperature-dependent material parameters, Newton-Raphson method was used to solve nonlinear equilibrium equations.24Newton-Raphson method performed iterations at each step until convergence was achieved.
2.3.2.Material parameters
In this study,the generic copper-based alloy and electroformed nickel were chosen as the inner liner and outer jacket of thrust chamber respectively.The material parameters were carefully defined so that the model could closely match the behavior of materials.The Chaboche nonlinear kinematic hardening parameters of the inner liner such asCi(i=1,2,3)and γi(i=1,2,3)have no definite physical meaning and cannot be directly taken from experimental data.In order to determine the model parameters,a series of curve-fitting processes25were performed based on uniaxial tensile stress-strain experimental data of NARloy-Z at different operational temperatures.The experimental data were obtained from the technical report11presented by NASA.
Since the curve-fitting method is a nonlinear iterative process,initial values for all the parameters are required.In this study,the initial parameters were also preliminarily estimated26using the uniaxial stress-strain data.The fitting parameters are summarized in Table 1.All the parameters in Table 1 except γ3are least-squares best f i t values to the data.γ3is fixed as a given positive value during the curve-fitting processes because it is hard to be estimated based on uniaxial tensile experiment.Since γ3is closely related to the ratcheting strain rate,it is best determined by stress-controlled ratcheting experimental data.However,these experimental data of NARloy-Z are almost unavailable in published work.In consideration of the normal range scope of γ3,the adopted value represents a medium rate of ratcheting strain accumulation under stress-controlled cyclic loading.
With the fitted parameters,the Chaboche model is assessed here by comparison between uniaxial tensile simulation results and corresponding experimental data.11As Fig.2 shows,the stress-strain curves predicted by Chaboche model agree well with the experimental data at different operational temperatures.The comparison demonstrates that the Chaboche model parameters in Table 1 are accurate and reliable.The Chaboche model parameters at the temperature not explicitly given in Table 1 were defined by piecewise linear interpolation.Other material parameters including density,specific heat capacity,thermal conductivity,isotropic secant coefficients of thermal expansion,elastic modulus,and Poisson’s ratio were directly obtained from the experimental results in Ref.11.
2.3.3.Grid generation and boundary conditions
The analysis was carried out for a typical full-scale experimental thrust chamber of LOX/Methane rocket engine,which is designed to achieve longer service life.Fig.3 shows the schematic of the thrust chamber with regenerative cooling.The thrust chamber wall is cooled by cryogenic methane which flow adversely as hot gas flow.There are 360 optimized cooling channels milled axial in the copper liner.Because the geometry of the thrust chamber is axisymmetric in the circumferential direction,the computation was conducted for only 0.5°by employing the symmetry boundary conditions.The diameter of the throat is 210 mm,while the diameter of the cylindrical part of the chamber is 340 mm.The lengths from the throat to the face plate and chamber outlet are 380 mm and 220 mm respectively.The chamber contraction ratio is 2.62,while the expansion ratio is 4.75.Calculations were performed by applying the overall oxidizer-to-fuel mixture ratio O/F=3.5 and the pressure of the combustion chamber is 10.1 MPa.The width and height of the cooling channel in the throat region are 0.87 mm and 4 mm respectively.At coolant inlet,mass flow rate is 27 kg/s,static temperature is 120 K,and static pressure is 17 MPa.A close-up shot of computational grid is shown in Fig.4.The 3-D twenty-node solid element which can be used for both thermal analysis and structural analysis was generated in computational domain.The reason why the higher order element was adopted in this study is that it can ensure accuracy with a relatively coarse mesh.

Table 1 Chaboche nonlinear kinematic hardening parameters for inner liner.
(1)Boundary conditions for transient thermal analysis
The work cycle of reusable LRE consists of four basis phases:pre-cooling,hot run,post-cooling and relaxation.The initial temperature of the thrust chamber wall was set equal to room temperature.The schematic of boundary conditions is shown in Fig.4.For the symmetric boundary conditions,the derivation of temperatures in the normal direction of the boundary was set to zero.The convective boundary conditions were applied at the fluid-solid coupled wall surface.In Fig.4,Tis bulk temperature of the fluidandhis convective film coefficients,pis the pressure.The subscript ‘hot’, ‘cool’and ‘out’represent hot-gas-side,coolant-side,and out-side respectively.
The specific values of boundary conditions for transient thermal analysis are summarized in Table 2 phase by phase.Both the values ofToutandhoutremained constant during work cycle considering natural convection between out-side wall surface and air.For hot run phase,the convective loads on the coupled wall surfaces were obtained from the numerical results of fluid-thermal coupling calculation.The values of the uniform convective loads on the coupled wall surfaces remained constant during the other phases.In fact,most researchers16,19,27,28determine the boundary condition of thermal analysis in the same way because the distributions of the thermal boundaries are hard to be comprehensively obtained by experiments or steady CFD simulations.It should be noted that the adoptedhcoolat pre-cooling and post-cooling phases was 120000 W/(m2·K)which is an estimated average value for the coolant-side wall according to the studies by Riccius et al.27,28In their studies on Vulcain engine,an available convective film coefficients of the coolant(liquid hydrogen)at precooling and post-cooling phases was 220000 W/(m2·K).With that value,the convective film coefficients of the methane at pre-cooling and post-cooling phases in this study were deduced by Dittus-Boelter equation,29which is given by
whereNuis the Nusselt number,Rethe Reynolds number,andPrthe Prandtl number.Let us suppose that the mass flow rate of the coolant is equal to that in Ref.27.With this equation,we thus derived
where μ is dynamic viscosity,Lthe wetted perimeter of the cross section,andDhydraulic diameter.The subscript ‘1’and ‘2’represent methane and hydrogen respectively.All the thermophysical properties of methane and hydrogen at corresponding pressures and temperatures were taken from NIST Standard Reference Database,30as summarized in Table 3.The deduction reveals that the cooling capacity of methane is much lower than that of hydrogen.Meanwhile,all the convective film coefficients of natural convection in this study were assumed to be 10 W/(m2·K)which was an estimate value.However,these values are consistent with the actual situation that the convective film coefficients of natural convection should be much smaller than those of coolant convection.
(2)Boundary conditions for nonlinear structural analysis
For the symmetric boundary conditions,the displacements in the normal direction of the boundary were set to zero.The pressure distributions of the fluid-solid coupled wall obtained by fluid-thermal coupling calculations were applied as the mechanical loads at hot run phase.For the other phases,the values of the uniform mechanical loads at the hot-gas-side wall were assumed to be normal atmospheric pressure,while the values at the coolant-side wall were 0.5 MPa.The temperatures in the thrust chamber wall obtained by transient thermal analysis for each time step were applied as the thermal body loads.
3.Results and discussion
3.1.Finite element model validation
To demonstrate that the results of thermal-structural analysis were independent of the adopted finite element(FE)model,a validation was carried out by comparison of the stressstrain responses of the identical location predicted with various grid levels and load substeps.Table 4 summarizes the number of substeps during one cycle and the spacing of nodes in the radial,circumferential,and axial directions for various FE models.Model 1,Model 3,and Model 5 have different grid levels with the same substep arrangement.Model 2,Model 3,and Model 4 have the same grid level but different substep arrangements.
Fig.5 present the stress-strain responses during one work cycle for various grid levels and various substep arrangements respectively.As Fig.5(a)shows,discrepancies could be observed between the solutions with Model 1 and Model 3,especially in the upper left part of the loop.There is a high level of agreement between the solutions with Model 3 and Model 5,although the nodes of Model 5 are much more than those of Model 3.As Fig.5(b)shows,large discrepancies exist between Model 2 and Model 3 at the transition from elastic to plastic deformation.However,the stress-strain curve predicted by Model 3 is only slightly inconsistent with that predicted by Model 4.As discussed above,the comparison verifies that the solutions with Model 3 are independent of the selected grid and substep arrangement.Therefore,the results with Model 3 are adopted in the following research.
3.2.Analysis for the first work cycle
3.2.1.Transient thermal analysis
Fig.6 illustrates the temperature distribution of the thrust chamber wall at the end of hot run phase.It should be noted that the hot gas flows in the+zdirection.The inner wall in the throat region and the upstream area of the throat are under the extreme high temperatures due to the enhanced convection between the hot-gas-side wall and the rapidly accelerating hot gas.Owing to the influence of the Dean vortices in the throat region,20the maximum temperature of the inner wall appears at the convergent part of the chamber.Nevertheless,thetemperatures in the inner wall are well below the limit of copper alloy because of the effective cooling of cryogenic methane in cooling channels.The maximum temperature(785 K)seems lower than that of an expandable chamber,but it is appropriate to prolong the life of a reusable chamber.The temperatures in the outer jacket remain relatively low because most of the heat from the combustion is absorbed by the coolant.These results suggest that the regenerative cooling introduces the huge temperature difference across the thrust chamber wall in hot run phase.
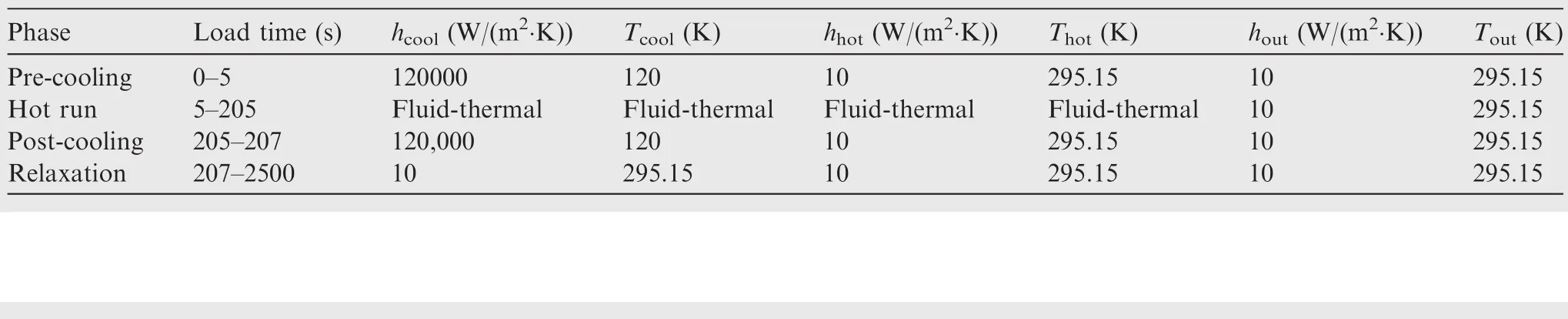
Table 2 Boundary conditions for thermal analysis.

Table 3 Thermophysical properties of coolants at pre-cooling and post-cooling phases.

Table 4 Various finite element models for validation.
The transient responses of the temperatures for the various points(see Fig.4)at the throat section are shown in Fig.7.For the full cycle,the temperature variation ranges of the points in the inner wall(C,DandE)are much wider than those of the points in the outer jacket(AandB).The temperatures of PointsC,DandEhave mutations at the beginning of hot run phase because the convective boundary condition of the hot-gas-side wall is applied at this moment.Since these points are located below the cooling channel,at or near the hot-gasside wall,the temperature responses of these points are much enlarged.Meanwhile,the temperature changes of the points in the outer jacket lag behind those of the points in the inner wall,especially at the beginning of pre-cooling and hot run phases.The finding reveals that the transient effect is obvious in the heat transfer of the thrust chamber wall because the thermal conductivity of the nickel jacket is far smaller than that of the copper liner.It can also be observed that the temperature difference between the inner wall and outer jacket reaches the maximum at the end of hot run phase.However,the temperatures of all points decline during post-cooling phase until the temperatures in the thrust chamber wall are almost uniform.
3.2.2.Structural analysis for the first work cycle
Fig.8 depicts the predicted total circumferential mechanical strain distributions of the thrust chamber wall at the end of hot run and relaxation phases respectively.As shown in Fig.8(a),large compressive strains appear in the inner wall.The results can be explained by the huge temperature difference observed in Figs.6 and 7.The temperatures in the inner wall are much higher than those in the outer jacket,and the inner wall tends to expand compared to the outer jacket.Hence,the relatively stiff and thick nickel jacket imposes compressive stresses to constrain the thermal deformation of the inner wall.The maximum compressive strain at hot run phase is 0.01384,which is significantly smaller than 0.1.Thus,this information can clearly demonstrate that the small strain assumption is appropriate for the first work cycle.Because the coolant supply is cut off at relaxation phase,the temperatures in the thrust chamber wall gradually return to the initial value.The absolute values of total circumferential mechanical strains significantly reduce at the end of the first cycle(see Fig.8(b)).However,for the throat region and the upstream area of the throat,the residual strains in the center of the inner wall ligament remain relatively large values.
The maximum circumferential residual strain of the inner wall also appears at the convergent part of the chamber,which is consistent with the maximum temperature in Fig.6.Fig.9 displays the circumferential stress–mechanical strain response of maximum residual strain point during the first work cycle.For pre-cooling,hot run,and post-cooling phases,this point experiences a load reversal during one of these phases.This is because the temperature difference between the inner wall and outer jacket increases first,and then decreases during one of these phases.More specifically,the temperatures in the inner wall change much quickly than those in the outer jacket at the transition to the next phase.However,at the latter part of the next phase,the temperatures in the inner wall remain unchanged while those in the outer jacket continue to change slowly(see Fig.7).This finding confirms that the temperature transient effect is the strong factor affecting the stressstrain responses in the inner wall.For pre-cooling phase,only a small plastic strain is developed at this point.A huge temperature gradient brings about a large compressive plastic strain at this point during the hot run phase.However,because the temperatures in the entire chamber wall go down to about 120 K,the temperature gradients in the thrust chamber wall almost disappear at the end of post-cooling phase,which results in the decrease and reversal of the compressive strain.Although the tensile strain progressively decreases during relaxation phase,a significant tensile strain accumulates at this point when the first cycle ends.
Over the last few decades,2-D plane strain finite element analysis for the throat section has been widely performed to obtain the structural responses in the thrust chamber wall.However,the results of 3-D thermal-structural analysis in this study indicate that the maximum circumferential residual strain of the inner wall does not occur at the throat section.To accurately predict the failure of the inner wall,it is quite necessary to perform a reliable 3-D thermal-structural analysis.
3.3.Structural analysis for multiple work cycles
For reusable LRE,the thermal-mechanical ratcheting of the inner wall is the essential reason for the failure of thrust chambers under cyclic work.To investigate the ratcheting behavior of the inner wall,the 3-D thermal-structural analysis of the thrust chamber wall for 20 work cycles was carried out.Fig.10 shows the variations of circumferential residual strain with cycle number for the different γ3. γ3=0, γ3=5 and γ3=10 represent cyclically hardening material,cyclically stable material with relatively low ratcheting strain rate,and cyclically stable material with relatively high ratcheting strain rate respectively.
It can be seen from Fig.10 that the variations of circumferential residual strains are nonlinear in the first few cycles.The residual strains for the different γ3are almost identical at the end of the first work cycle.However,the accumulated residual strains for the different γ3are not equal since the second work cycle.In Eq.(6),α1,α2and α3are respectively used for describing the nonlinear behavior when small,medium,and large plastic strains occur.This means that γ1,γ2and γ3sequentially control the growth rate of the residual strains when the plastic strain increases.Because the order of magnitude of γidecreases asiincreases(see Table 1),the growth rate of the residual strains decreases and the discrepancies between the residual strains for different γ3increase when the work cycle proceeds.For γ3=0,the growth rate of the residual strains gradually falls to nearly zero.This is because the dynamic recovery term of α3is eliminated.Consequently,linear kinematic hardening occurs and ratcheting is suppressed when plastic strains are large enough.For γ3=5 and γ3=10,the growth rates of the residual strains decline since the first work cycle,until they remain constant greater than zero.Because the influence of the dynamic recovery term is strengthened as γ3increases,γ3=10 ultimately has much higher growth rates of the residual strains than γ3=5.Ultimately,the residual strain at the 20th work cycle for γ3=10 is about 0.03448,which means that the small strain assumption is also reasonable for the multiple work cycles in this study.
The data reported here suggest that the values of γ3seriously affect the simulated ratcheting behavior of the inner wall.To accurately predict the lives of thrust chambers in engineering practice,the values of γ3over a wide temperature range for the specific material of the inner wall must be first determined by numerous stress-controlled ratcheting experimental data.For the inner wall of cyclically hardening materials(γ3=0),the ratcheting strains have plateaued after several cycles.Thus,the failure of the thrust chamber may be dominated by the low-cycle fatigue of the inner wall.For the inner wall of cyclically stable materials(γ3> 0),the ratcheting strains steadily increase as the work cycle proceeds.As the general material of the inner liner for high pressure thrust chambers of reusable LRE,the copper or the copper-based alloy usually has a value of γ3greater than zero.Therefore,the damage caused by the thermal-mechanical ratcheting of the inner wall cannot be ignored.In fact,the local bulging and thinning of the inner wall observed in experiment9are the typical features of the thermal-mechanical ratcheting.31
3.4.Sensitivity analysis of mechanical loads
As mentioned above,the thermal-mechanical ratcheting results in the thinning and bulging of the inner wall.The effect of mechanical loads on this cyclic plastic behavior of the inner wall was discussed in detail to provide substantial advices for the design of reusable LRE to prolong the lives of thrust chambers in industry.For the reusable thrust chamber,the mechanical loads consist of the pressure of the hot gas during hot run phase and the pressure of the coolant during pre-cooling,hot run,and post-cooling phases.Table 5 presents the scheme for comparison,in which four cases with different pressures of the hot gas and coolant were calculated.The material parameters,grid arrangements,and boundary conditions of Case 0 are identical to those described in Section 2.Compared to Case 0,Case 1 does not consider the pressures of the hot gas and coolant during hot run phases,and Case 2 and Case 3 have much greater pressures of the coolant during precooling and post-cooling phases respectively.
Fig.11 shows the comparison of the circumferential stress–mechanical strain curves of maximum residual strain points during the first work cycle.Compared to other cases,Case 1 has a much greater compressive strain at the end of hot run phase,leading to a much smaller residual tensile strain at the end of the first work cycle.The tensile strains at the end of the pre-cooling phase of Case 2 and at the end of the postcooling phase of Case 3 are greater than those of Case 0.Correspondingly,the residual tensile strains of Case 2 and Case 3 are greater than that of Case 0.These results can be explained by assuming that the pressure difference between the coolant and the hot gas always tends to bend the inner wall,leading to a circumferential tensile strain at maximum residual strain point.It can be concluded that mechanical loads have a strong influence on the circumferential strain of the inner wall when large plastic strains are produced,especially during hot run and post-cooling phases.Fig.12 shows the variations of circumferential residual strains as a function of cycle number for all cases.As the work cycle proceeds,the differences of residual strains among these cases are getting greater because of the stable accumulation of residual strains after several cycles.
These findings suggest that the ratcheting of the inner wall under cyclic work may slow down through the reasonable choices of coolant pressures during each phase.The specific approaches are described as follows:
(1)Choose a relatively low pressure at the coolant inlet during hot run phase on the premise of meeting the requirement of the pressure downstream cooling channels.
(2)Reduce the coolant pressure during pre-cooling and
post-cooling phases as far as possible.

Table 5 Various arrangements of mechanical loads for comparison.
4.Conclusions
(1)A 3-D fluid-structural coupling computational methodology is developed in this paper to obtain the thermal and structural responses of the thrust chamber wall under cyclic work.The Chaboche nonlinear kinematic hardening model is adopted in this methodology to predict the cyclic plastic behavior of the inner wall,and the stress-strain curves obtained by Chaboche model conform closely to experimental data from literature.
(2)The presented methodology reveals that both the maximum temperature at hot run phase and the maximum circumferential residual strain of the inner wall appear at the convergent part of the chamber because of Dean vortices in the throat region.However,these features cannot be thoroughly obtained by traditional 2-D plane strain finite element analysis.
(3)The value of γ3of Chaboche model has a significant effect on the predicted ratcheting behavior of the inner wall.For the inner wall with γ3=0,the failure of the thrust chamber may be controlled by the low-cycle fatigue.For the inner wall with γ3> 0,the damage caused by the thermal-mechanical ratcheting of the inner wall is not ignorable.
(4)The results of sensitivity analysis of mechanical loads indicate that they have a strong influence on the circumferential strain of the inner wall,especially during hot run and post-cooling phases.To improve the durability of inner wall,the coolant pressures during each phase should be as low as possible to slow down the ratcheting of the inner wall under cyclic work.
(5)However,we recognize that γ3of Chaboche model in this paper was not strictly determined by experimental data and was assumed to be independent of the operational temperature.Quantitatively predicting the lives of thrust chambers based on the strain data with exact γ3merits further study.
1.Klepikov IA,Katorgin BI,Chvanov VK.The new generation of rocket engines,operating by ecologically safe propellant ‘liquid oxygen and liquef i ed natural gas(methane)”.Acta Astronaut1997;41(4–10):209–17.
2.Crocker AM,Peery SD.System sensitivity studies of a LOX/methane expander cycle rocket engine.Reston:AIAA;1998.Report No.:AIAA-1998-3674.
3.Pempie P,Froehlich T,Vernin H.LOX/methane and LOX/kerosene high thrust engine trade-off.Reston:AIAA;2001.Report No.:AIAA-2001-3542.
4.Brown CD.Conceptual investigations for a methane-fueled expander rocket engine.Reston:AIAA;2004.Report No.:AIAA-2004-4210.
5.Burkhardt H,Sippel M,Herbertz A,Klevanski J.Kerosene vs.methane:A propellant tradeoff for reusable liquid booster stages.J Spacecr Rockets2004;41(5):762–9.
6.Neill T,Judd D,Veith E,Rousar D.Practical uses of liquid methane in rocket engine applications.Acta Astronaut2009;65(5–6):696–705.
7.Baiocco P,Bonnal Ch.Technology demonstration for reusable launchers.Acta Astronaut2016;120:43–58.
8.Janovsky RS,Arya VK,Kazaroff JM,Halford GR.Structurally compliant rocket engine combustion chamber—Experimental and analytical validation.J Spacecr Rockets1995;32(4):645–52.
9.Quentmeyer RJ.Experimental fatigue life investigation of cylindrical thrust chambers.Reston:AIAA;1977.Report No.:AIAA-1977-0893.
10.Conway JB,Stentz RH,Berling JT.High-temperature,low-cycle fatigue of copper-base alloys for rocket nozzles.Part 1:Data summary for materials tested in prior programs.Washington,D.C.:NASA;1975.Report No.:NASA-CR-134908.
11.Esposito JJ,Zabora RF.Thrust chamber life prediction.Volume 1:Mechanical and physical properties of high performance rocket nozzle materials.Washington,D.C.:NASA;1975.Report No.:NASA-CR-134908.
12.de Groh III HC,Ellis DL,Loewenthal WS.Comparison of GRCop-84 to other high thermal conductive Cu alloys.Washington,D.C.:NASA;2007.Report No.:NASA/TM-2007-214663.
13.Miller RW.Low-cycle fatigue analysis of a cooled copper combustion chamber.Reston:AIAA;1974.Report No.:AIAA-1974-1079.
14.Hannum NP,Kasper HJ,Pavli AJ.Experimental and theoretical investigation of fatigue life in reusable rocket thrust chambers.Reston:AIAA;1976.Report No.:AIAA-1976-685.
15.Arya VK,Arnold SM.Viscoplastic analysis of an experimental cylindrical thrust chamber liner.AIAA J1992;30(3):781–9.
16.Yang JH,Chen T,Jin P,Cai GB.Influence of the startup and shutdown phases on the viscoplastic structural analysis of the thrust chamber wall.Aerosp Sci Technol2014;34:84–91.
17.Armstrong WH,Brogren EW.Three dimensional thrust chamber life prediction.Washington,D.C.:NASA;1976.Report No.:NASA-CR-134979.
18.Riccius JR,Zametaev EB,Haidn OJ.Comparison of 2D and 3D structural FE-Analyses of LRE combustion chamber walls.Reston:AIAA;2006.Report No.:AIAA-2006-4365.
19.Cheng C,Wang YB,Liu Y,Liu DW,Lu XY.Thermal-structural response and low-cycle fatigue damage of channel wall nozzle.Chin J Aeronaut2013;26(6):1449–58.
20.Kang YD,Sun B.Numerical simulation of liquid rocket engine thrust chamber regenerative cooling.J Thermophys Heat Transf2011;25(1):155–64.
21.Song J,Sun B.Coupled numerical simulation of combustion and regenerative cooling in LOX/Methane rocket engines.Appl Therm Eng2016;106:762–73.
22.Chaboche JL.Constitutive equations for cyclic plasticity and cyclic viscoplasticity.Int J Plast1989;5(3):247–302.
23.ANSYS Inc.ANSYS theory reference release 14.5.Canonsburg(PA):ANSYS Inc.;2012.
24.BatheKJ.Finite element procedures.2nd ed.Englewood Cliffs:Prentice Hall;2014.p.755–9.
25.Bari S,Hassan T.Anatomy of coupled constitutive models for ratcheting simulation.Int J Plast2000;16(3–4):381–409.
26.Rezaiee-Pajand M,Sinaie S.On the calibration of the Chaboche hardening model and a modified hardening rule for uniaxial ratcheting prediction.Int J Solids Struct2009;46(16):3009–17.
27.Riccius JR,Zametaev EB.Stationary and transient thermal analyses of cryogenic liquid rocket combustion chamber walls.Reston:AIAA;2002.Report No.:AIAA-2002-3694.
28.Riccius JR,Oskar H,Zametaev EB.Influence of time dependent effects on the estimated life time of liquid rocket combustion chamber walls.Reston:AIAA;2004.Report No.:AIAA-2004-3670.
29.Incropera FP,Dewitt DP,Bergman TL,Lavine AS.Fundamentals of heat and mass transfer.6th ed.Hoboken(NJ):John Wileyamp;Sons;2006.p.514–21.
30.Lemmon EW,Mclinden MO,Friend DG.Thermophysical properties of fluid systems.In:Linstrom PJ,Mallard WG,editors.NIST chemistry webbook,NIST Standard Reference Database number 69.Gaithersburg(MD):National Institute of Standards and Technology;2017.
31.Popp M,Schmidt G.Rocket engine combustion chamber design concepts for enhanced life.Reston:AIAA;1996.Report No.:AIAA-1996-3303.
29 March 2016;revised 7 February 2017;accepted 12 March 2017
 CHINESE JOURNAL OF AERONAUTICS2017年3期
CHINESE JOURNAL OF AERONAUTICS2017年3期
- CHINESE JOURNAL OF AERONAUTICS的其它文章
- Review on signal-by-wire and power-by-wire actuation for more electric aircraft
- Real-time solution of nonlinear potential flow equations for lifting rotors
- Suggestion for aircraft flying qualities requirements of a short-range air combat mission
- A high-order model of rotating stall in axial compressors with inlet distortion
- Experimental and numerical study of tip injection in a subsonic axial flow compressor
- Dynamic behavior of aero-engine rotor with fusing design suffering blade of f
