含时驱动的Dicke模型的混沌特性∗

刘妮梁九卿

(山西大学理论物理研究所,太原 030006)

含时驱动的Dicke模型的混沌特性∗

刘妮†梁九卿

(山西大学理论物理研究所,太原 030006)

(2017年1月21日收到;2017年3月28日收到修改稿)

依据Dicke量子相变首次被观测的实验装置,我们通过调节抽运激光强度来实现原子-场集体耦合强度的单频非绝热调制,即实现含时驱动的Dicke模型.当驱动的耦合强度为零时,系统回退到标准的Dicke模型.从刻画的庞加莱截面图观测到:在临近相变点附近系统由经典规则轨道向混沌变化,超辐射区对应着相空间的完全混沌;当静态耦合和驱动耦合同时存在时,系统显示出丰富的混沌运动.通过调节振荡频率,系统可在正常相区间从经典规则轨道变到混沌再变到经典规则轨道.

单频非绝热调制,Dicke模型,量子混沌,庞加莱截面图

1 引言

很多量子系统都存在量子混沌,量子混沌是一种普遍存在的现象,它与相变、纠缠、隧穿等物理现象存在很多关联.经典哈密顿系统是否可积与混沌直接相关.把经典力学中的可积性推广到量子力学对量子混沌的研究是非常有益的.现在人们经常使用的量子可积性定义都是经典可积性的推广.三十多年前,科学家已经预测到标准Dicke模型存在正常相到超辐射相的量子相变[1−3].同时,Dicke模型的量子混沌特性也早有研究[4−8],但是真正将其混沌特性与量子相变连接在一起的却是从文献[9]开始的.文献[9]指出:标准Dicke模型会经历从准可积到量子混沌的变化,而且这种变化是由与之完全对应的量子相变引起的.我们考虑一组四能级原子自发地与横向抽运激光驱动的单模腔场进行相互作用的系统.最近,实验上已在玻色-爱因斯坦凝聚(BEC)与高精细光腔组合的实验中实现了相似的装置,而且在该装置中观测到了从正常相到超辐射相的Dicke量子相变[10].该装置的一个直观优点是:通过操纵抽运激光强度来实现可调谐的集体原子场耦合强度.本文的主要想法是:在实现的Dicke模型中引入含时依赖的原子场集体耦合项.我们发现:当含时系统回退到标准Dicke模型时,其量子混沌特性与前人的结果完全符合,在临界相变点附近发生从规则周期轨道到完全混沌的变化.当我们引入原子场集体耦合强度的单频非绝热调制项后,系统的混沌特性变得非常复杂.为了更好地理解静态耦合(∆g=0)和驱动耦合(∆=0),以及弱驱动(如:∆g=0.2)和强驱动(如:∆g=0.6)对含时Dicke系统混沌特性的影响,本文给出了一系列参数下系统的庞加莱截面图,为研究含时Dicke系统提供了一种新的研究方法和研究手段.结果发现只有系统整体稳定,局部不稳定才会出现混沌.

2 含时驱动的Dicke模型

2.1 理论模型

Dicke模型描述单个二能级原子系综集体耦合到一个单模量子化电磁场的情形,当原子-场集体耦合强度超过某个临界值时系统会经历一个零温量子相变,发生从正常相到超辐射相的量子相变.该相变已于2010年由Baumann等首次在实验上观测到[10].这种集体量子现象所引起的超辐射-正常量子相变在原子物理和量子光学中被广泛研究.此外量子混沌、基态纠缠、临界行为等与量子相变相关的领域也为大家所关注.依据Baumann等[10]提出的实验装置(即一组Rb87的四能级超冷原子与单模光场发生集体相互作用,外加横向抽运激光),每个原子有两个稳定的基态,分别记为|0,0⟩和|±kpmk⟩,它们通过两束拉曼通道耦合.在大失谐极限下,原子的激发态能被绝热消除而成为有效的二能级系统[11−13],运用集体自旋算符Si(i=±,z)来表示,这样我们实现了Dicke类型的哈密顿量(~=1):

其中,N是原子数;有效腔频ω=ωc−ωp,而ωc是腔频,ωp是横向抽运激光频率;有效原子频率ω0=2ωr,而原子的反冲能量ωr=k2/2m;集体耦合强度,而ξ是抽运拉比频率;a†和a代表光子的产生和湮没算符;集体原子算符Si(i=±,z)满足SU(2)角动量关系.

实验上通过改变抽运激光强度来调节横向抽运激光频率ωp,进而调节集体原子-场耦合强度.那么将原子-场耦合强度调制成g(t)=g+∆g cos(ηt)这样的形式,我们将得到含时驱动的Dicke模型[14,15],即

哈密顿量(2)与哈密顿量(1)具有相同的形式,只是原子-场耦合强度g被取代为g(t).g(t)的最大值和最小值分别为g+∆g和g−∆g.在后面我们会发现:规则轨道到量子混沌的临界点并非仅仅由g+∆g和g−∆g体现,这是不同于标准Dicke模型的混沌特性的.在热力学极限,N→∞,无驱动的Dicke模型(∆g=0)在临界耦合时显示一个量子相变,此时基态从无激发的正常相变到对称破缺的超辐射相.在超辐射相区,场和原子集体都具有了宏观占据.同时混沌特性也正是在相变点处从规则轨道变化到完全的混沌.我们通过调查无驱动下Dicke模型的庞加莱截面图来开始我们的分析.

我们先使用Holstein-Primako ff变换[16,17],即用单一玻色模取代自旋算符的操作:

标准Dicke模型可以演化出许多不同的半经典模型[5],而且也发展了许多不同的研究方法.广泛讨论的方法是Hartree-Fork-type的近似,它是先得到系统的海森伯运动方程,并用期待值取代方程中的算符[4].它们被看作经典变量,并且得到它们的一系列非线性运动方程.依据这些运动方程可以得到一定参数区的经典混沌.尽管如此,在一定程度上,上面的方法并不能完全满足仅依赖于原子数N变化的运动.Furuya等[5]研究了类似于Dicke模型的一个经典模型,通过在光子和原子相干态基失构成的态下评定系统的期待值,得到其半经典哈密顿量,并且展示出系统出现混沌.尽管文献中的模型与我们的无驱动Dicke模型非常相似,但其并未完备地讨论模型的混沌特性.而混沌特性是反映系统诸如量子相变、稳定性等基态特性至关重要的手段或特征.

2.2 运动方程

通过引入两玻色模的坐标和位置算符关系,即两维谐振子算符与坐标和位置的关系

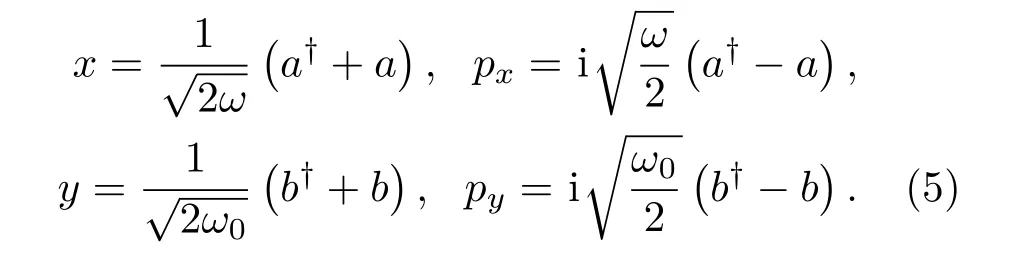
将(5)式经过整理得到

将(6)式坐标-动量表示代入哈密顿量(4),得到如下形式的系统经典哈密顿量:

为了分析有限粒子数N的半经典系统的行为,我们从Hsc的偏导数给出了哈密顿量的运动方程:

3 系统的混沌特性

原则上由经典哈密顿量和运动方程就可以研究系统的混沌特性.我们在初始条件和各种变化参数下数值地调研了半经典系统的哈密顿量运动方程.为了分析系统的运动轨迹,我们使用四维相空间的庞加莱截面图来刻画.我们定义截面图的表面为x=0,px由E(t)确定.作为例证,图1给出了某参数下的无驱动Dicke系统的(y,py)平面的庞加莱截面图.

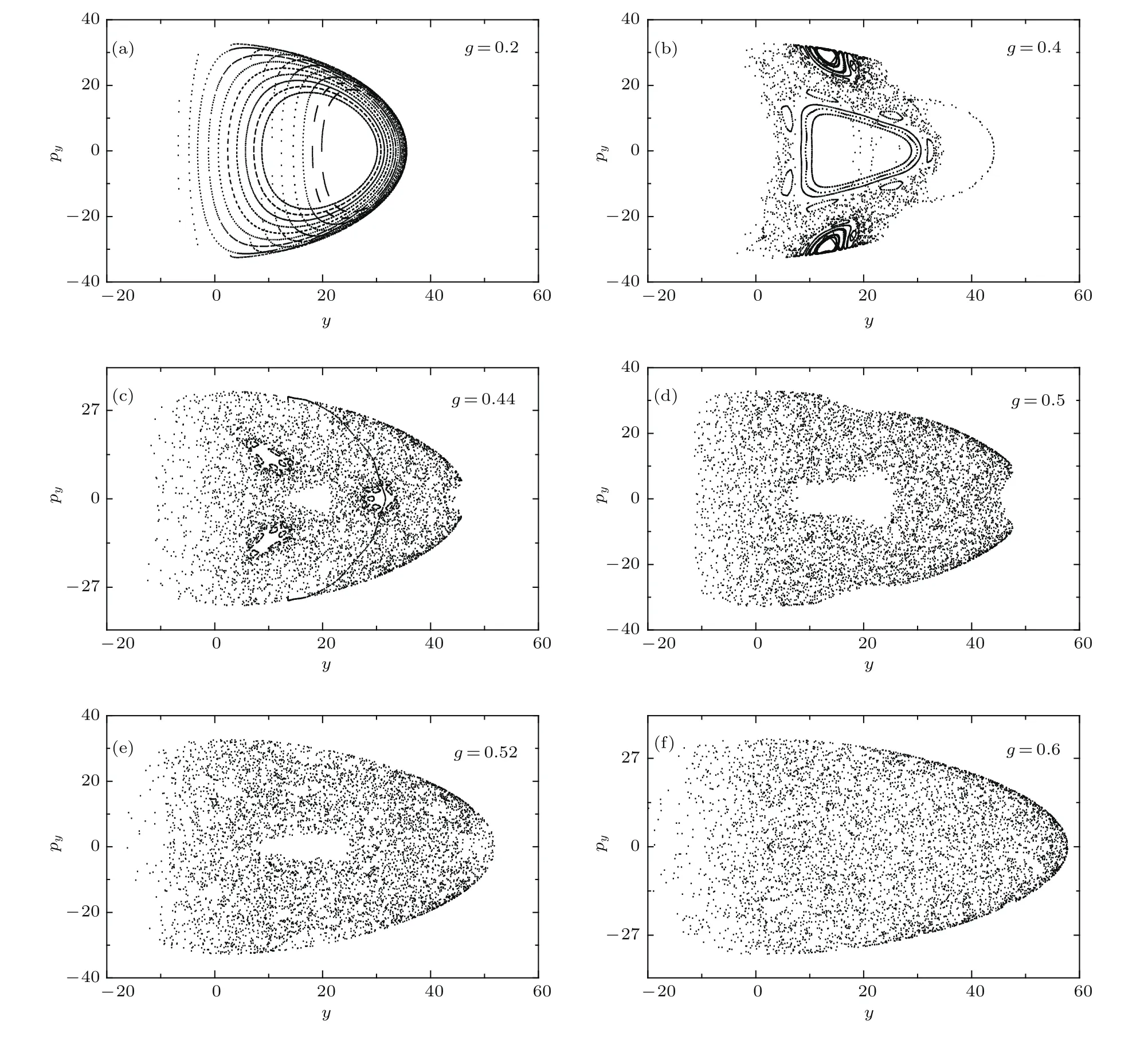
图1 无驱动Dicke模型随参数变化的庞加莱截面图,其中初始能量E=20,原子数N=1000,哈密顿量在共振情形ω=ω0=1Fig.1.The Poincaré sections of the undriven Dicke model in the resonance case of ω=ω0=1.The plotted parameters are given by the initial energy E=20 and the atomic number N=1000.

图1清晰地描述出:在原子-场耦合强度g很小,即g 6 0.4(如图1(a),(b))时,庞加莱截面图是由一系列规则、周期或准周期轨道组成;接近临界原子-场耦合强度,即g=0.44,g=0.50(如图1(c),(d))时,我们看到周期轨道特征的改变,并且出现许多混沌轨迹,即软混沌;增加原子-场耦合强度更导致残余的周期轨道解体,并且整个相空间变成混沌区,即硬混沌,如原子-场耦合强度略微超过临界值gc=0.50,即g=0.52,g=0.60(如图1(e),(f)).该图结果再一次验证了文献[9]中图14的结果,也表明无论从理论计算还是数值模拟,无驱动结果回退的正确性,这也保证了我们后面给出驱动Dicke模型结论的可靠性.最重要的是,图1表明:对于标准Dicke模型,量子相变点是系统由规则轨道向混沌转变的指标.

图2清晰地展示出,当静态耦合不存在时,系统是没有混沌特性的.驱动耦合的调节本质上并没有改变系统的物理特性.该组图告诉我们,此时的原子-场耦合强度本质上是由余旋函数∆g cos(Ωt)调节的振荡形式,且原子-场耦合强度取最大值g(t)max=0,0.4,0.6,0.8时,并没有显示出规则轨道或混沌图像.可见,混沌图像是与我们的静态耦合g息息相关的.

图2 静态耦合为零(g=0),驱动耦合变化(∆=0)时Dicke模型的庞加莱截面图,其中初始能量E=20,原子数N=1000,振荡频率Ω=10,且哈密顿量在共振情形ω=ω0=1Fig.2.The Poincaré sections of Dicke model as the function of the driven coupling strength∆g with the static coupling g=0 in the resonance case of ω=ω0=1.The plotted parameters are given by the initial energy E=20,the atomic number N=1000,and the oscillation frequency Ω=10.

图4主要展示的是不同静态耦合和驱动耦合下系统的庞加莱截面图.由图4(a)可以看出,当静态耦合g=0.2gc=0.5时,若驱动耦合∆g=0,系统显示的是完全混沌图像,参看图1(f);若调节驱动耦合∆g=0.2,系统仍然显示的是完全混沌图像.可见,静态耦合的大小对系统是规则轨道还是混沌起决定作用,而驱动耦合只是一定程度上起调节或者振荡作用.

图3 静态耦合和驱动耦合为定值(g=∆g=0.2)时含时Dicke模型随振荡频率变化的庞加莱截面图,其中初始能量E=20,原子数N=1000,振荡频率Ω=0.02,0.2,2,5,10,200,且哈密顿量是在共振情形ω=ω0=1Fig.3.The Poincaré sections of the driven Dicke model with time-dependent as a function of the oscillation frequency Ω with the given coupling g=∆g=0.2 in the resonance case of ω=ω0=1.The plotted parameters are given by the initial energy E=20 and the atomic number N=1000.

图4 静态耦合和驱动耦合影响下含时Dicke模型的庞加莱截面图,其中初始能量E=20,原子数N=1000,振荡频率Ω=10,且哈密顿量是在共振情形ω=ω0=1Fig.4.The Poincaré sections of the driven Dicke model with time-dependent as the function of the static and driven coupling strengths in the resonance case of ω=ω0=1.The plotted parameters are given by the initial energy E=20,the atomic number N=1000 and the oscillation frequency Ω=10.

图5 给定的静态耦合(g=0.6)下变化的驱动耦合对含时Dicke模型庞加莱截面图的影响,其中初始能量E=20,原子数N=1000,振荡频率Ω=10,且哈密顿量是共振情形ω=ω0=1Fig.5.The Poincaré sections of the driven Dicke model with time-dependent as a function of the driven coupling strength with the given static coupling strength g=0.6 in the resonance case of ω=ω0=1.The plotted parameters are given by the initial energy E=20,the atomic number N=1000 and the oscillation frequency Ω=10.

为了更好地说明驱动耦合∆g对系统是否出现混沌的调节作用,图5给出了静态耦合为g=0.6时,不同驱动耦合下系统的庞加莱截面图.图5(a)说明当静态耦合g=0.6>gc=0.5时,调节驱动耦合∆g=0.2系统显示的是完全混沌图像,与无驱动情形完全一致,参看图1(f);若调节驱动耦合∆g=2.0,系统仍然显示的是完全混沌图像,但在中间区域出现一个很小的半月形区域,如图5(b);随着驱动耦合∆g增大,在整个混沌空间内,中间区域越来越大,且越来越趋于规则图像,参见图5(c),(d);当驱动耦合∆g增大至5.0时,整个区间变成规则图像,系统显示的是规则周期轨道.可见,静态耦合g可以调节出混沌特性,但同时驱动耦合∆g也可以使系统显示混沌特性向规则周期轨道转变.由此看来,含时系统的混沌特性要复杂于理想系统,而且含时规律的不同直接影响混沌图.

4 结论

本文考虑了包含单频非绝热调制的原子-场耦合强度的Dicke模型的混沌特性.在经典系统从周期轨道到混沌变化可以反映出系统的量子特性,尤其量子相变点是我们分析规则轨道和混沌转变的一个关键位置.如果仅有驱动耦合系统是不会出现混沌图像的,仅是振荡形式的余弦函数的体现;如果静态耦合强度和驱动耦合强度共存时,系统的基态特性主要由静态耦合决定,但驱动耦合在一定程度上可以调节系统的轨道.当系统的静态耦合强度大于临界耦合强度时,庞加莱截面图显示系统是完全混沌图像,但强的驱动耦合也可以调节出规则轨道特性;当系统的静态耦合强度小于临界耦合强度时,通过调节驱动耦合强度和振荡频率,系统也能显示出混沌图像.

[1]Hepp K,Lieb E H 1973 Ann.Phys.76 360

[2]Hioes F T 1973 Phys.Rev.A 8 1440

[3]Liu N,Lian J L,Ma J,Xiao L T,Chen G,Liang J Q,Jia S T 2011 Phys.Rev.A 83 033601

[4]Graham R,Höhnerbach M 1984 Phys.Lett.A 101 61

[5]Furuya K,Nemes M C,Pellegrino G Q 1998 Phys.Rev.Lett.80 5524

[6]Song L J,Yan D,Gai Y J,Wang Y B 2011 Acta Phys.Sin.60 020302(in Chinese)[宋立军,严冬,盖永杰,王玉波2011物理学报60 020302]

[7]Song L J,Yan D,Gai Y J,Wang Y B 2010 Acta Phys.Sin.59 3695(in Chinese)[宋立军,严冬,盖永杰,王玉波2010物理学报59 3695]

[8]Zhao W L,Wang J Z,Dou F Q 2012 Acta Phys.Sin.61 240302(in Chinese)[赵文垒,王建忠,豆福全2012物理学报61 240302]

[9]Emary C,Brandes T 2003 Phys.Rev.E 67 066203

[10]Baumann K,Guerlin C,Brennecke F,Esslinger T 2010 Nature 464 1301

[11]Yu L X,Fan J T,Zhu S Q,Chen G,Jia S T,Nori F 2014 Phys.Rev.A 89 023838

[12]Fan J T,Yang Z W,Zhang Y W,Ma J,Chen G,Jia S T 2014 Phys.Rev.A 89 023812

[13]Zhao X Q,Liu N,Liang J Q 2014 Phys.Rev.A 90 023622

[14]Bastidas V M,Emary C,Regler B,Brandes T 2012 Phys.Rev.Lett.108 043003

[15]Liu N,Li J D,Liang J Q 2013 Phys.Rev.A 87 053623

[16]Holstein T,Primako ffH 1949 Phys.Rev.A 58 1098

[17]Hillery M,Mlodinow L D 1984 Phys.Rev.A 31 797

PACS:05.45.Mt,42.50.Pq,73.43.NqDOI:10.7498/aps.66.110502

Chaos propeties of the time-dependent driven Dicke model∗

Liu Ni†Liang Jiu-Qing
(Institute of Theoretical Physics,Shanxi University,Taiyuan 030006,China)

21 January 2017;revised manuscript

28 March 2017)

Now,many di ff erent approaches have been presented to study the di ff erent semi-classical models derived from the Dicke Hamiltonian,which re fl ect a fact that the quantum-mechanical spin possesses no direct classical analog.The Hartree-Fock-type approximation is one of the widely used approaches,with which we derive the Heisenberg equations of motion for the system and replace the operators in these equations with the corresponding expectation values.In the present paper,we investigate the role of quantum phase transition in determining the chaotic property of the timedependent driven Dicke model.The semi-classical Hamiltonian is derived by evaluating the expectation value of the Dicke Hamiltonian in a state,which is a product state of photonic and atomic coherent states.Based on the inverse of the relations between the position-momentum representation and the Bosonic creation-annihilation operators,the Hamiltonian is rewritten in the position-momentum representation and it undergoes a spontaneous symmetry-breaking phase transition,which is directly analogous to the quantum phase transition of the quantum system.In order to depict the Poincaré sections,which are used to analyze the trajectories through the four-dimensional phase space,we give the equations of motion of system from the derivatives of the semi-classical Hamiltonian for a variety of di ff erent parameters and initial conditions.According to the Dicke quantum phase transition observed from the experimental setup,we study the e ff ect of a monochromatic non-adiabatic modulation of the atom- fi eld coupling in Dicke model(i.e.,the driven Dicke model)on the system chaos by adjusting the pump laser intensity.The change from periodic track to chaotic fi gure re fl ects the quantum properties of the system,especially the quantum phase transition point,which is a key position for people to analyse the shift from periodic orbit to chaos.In an undriven case,the system reduces to the standard Dicke model.We discover from the Poincaré sections that the system undergoes a change from the classical periodic orbit to a number of chaotic trajectories and in the superradiant phase area,the whole phase space is completely chaotic.When the static and driving coupling both exist,the system shows rich chaotic motion.The ground state properties are mainly determined by the static coupling,while the orbit of the system is adjusted by the driving coupling.If the static coupling is greater than the critical coupling,the system displays completely chaotic images in the Poincaré sections,and the periodic orbits in the chaos can also be adjusted by the strong driving coupling.While the static coupling is less than the critical coupling,the system can also show the chaotic images by adjusting the driving coupling strength and oscillation frequency.Moreover,by tuning the oscillation frequency,the Poincaré sections may change from the classical orbits to the chaos,and back to the classical orbits in the normal phase of the system.

a monochromatic non-adiabatic modulation,Dicke model,quantum chaos,the Poincaré section

10.7498/aps.66.110502

∗国家自然科学基金(批准号:11404198,11275118,91430109)、山西省高等学校科技创新项目(批准号:2014102)、山西大学科研启动金(批准号:011151801004)和国家基础科学人才培养基金(批准号:J1103210)资助的课题.

†通信作者.E-mail:317446484@qq.com

©2017中国物理学会Chinese Physical Society

http://wulixb.iphy.ac.cn

*Project supported by the National Natural Science Foundation of China(Grant Nos.11404198,11275118,91430109),the Scienti fi c and Technological Innovation Program of Higher Education Institutions in Shanxi Province(STIP),China(Grant No.2014102),the Launch of the Scienti fi c Research of Shanxi University,China(Grant No.011151801004),and the National Fundamental Training,China(Grant No.J1103210).

†Corresponding author.E-mail:317446484@qq.com