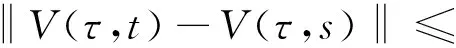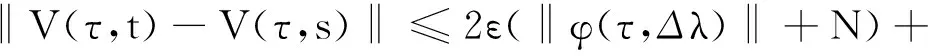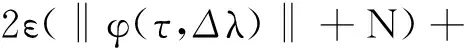Measure Functional Differential Equations with Infinite Delay: Differentiability of Solutions with Respect to Initial Conditions

LI Baolin, WANG Baodi

(College of Mathematics and Statistics, Northwest Normal University, Lanzhou 730070, Gansu)

Measure Functional Differential Equations with Infinite Delay: Differentiability of Solutions with Respect to Initial Conditions

LI Baolin, WANG Baodi

(CollegeofMathematicsandStatistics,NorthwestNormalUniversity,Lanzhou730070,Gansu)

In this paper, we consider a measure functional differential equation with infinite delay,which can be changed into a generalized ordinary differential equation. By differentiability of solutions with respect to initial condition for the generalized ODE, we obtain the differentiability for the measure functional differential equation.

measure functional differential equation; differentiability of solutions; Kurzweil integral; generalized ordinary differential equation

1 Introduction

There are many sources that describe the differentiability of solutions with respect to initial conditions for ordinary differential equations, such as [1-2]. From [3], we can see the description of a similar type for ordinary differential equations, and for dynamic equations on time scales. Similar work as [3] was also carried out in [4]. In this paper, we consider the measure differential equations.

When a system described by ordinary differential equation

(1)

is acted upon by perturbation, the resultant perturbed system is generally given by ordinary differential equation of the form dx/dt=f(t,x)+G(t,x), where we assume the perturbation termG(t,x) is well-behaved, i.e.,G(t,x) is continuous or integrable and as such the state of the system changes continuously with respect to time. However, in some system, the perturbations are impulsive, so we cannot expect the perturbation is always well-behaved. Therefore, the following equation

(2)

was defined in [5], whereDudenotes the distributional derivative of functionu. Ifuis a function of bounded variation,Ducan be identified with a Stieltjes measure, it will suddenly change the state of the system at a discontinuity ofu. In [5], equations of the form (2) are called measure differential equations, also a special case of the equation (2). Inspired by [5], the authors of [6] have generalized a very useful functional differential equation as following

(3)

wherextrepresents the restriction of the functionx(·) (x(·) denotes a solution of equation (2)) means a function of bounded variation whose distributional derivativeDxsatisfies the equation (2) on the interval [m(t),n(t)],mandnbeing functions with the propertym(t)≤n(t)≤t.

Moreover, in [7], an important theorem was proved. The main contents are as following：

x(·) is a solution of (2) through (t0,x0) on an intervalI, with left end pointt0, if and only ifx(·) satisfies the following equations

Authors of [8] especially proved the following measure functional differential equation with infinite delay

(4)

is equivalent to the generalized ordinary differential equation under some conditions. Also, equations (4) is the integral form of the following measure equation

Dx=G(s,xs)dg(s)，

whereg(s) is a nondecreasing function, and the integral on the right-hand side of (4) is the Kurzweil-Stieltjes integral.

In this paper, we shall consider differentiability of initial value problem for measure differential equations

(5)

wherexis an unknown function with values inRnandthesymbolxsdenotesthefunctionxs(τ)=x(s+τ)definedon(-∞,0],whichcorrespondingtothelengthofthedelay, f:P×[t0,t0+σ]→Rnis a function satisfies the following conditions (A)-(C):

(B)ThereexistsafunctionM:[t0,t0+σ] →R+,whichisKurzweil-Stieltjiesintegrablewithrespecttog,suchthat

wheneverx∈Oand[a,b]⊆[t0,to+σ].

(C)ThereexistsafunctionL :[t0,to+σ] →R+,which is Kurzweil-Stieltjies integrable with respect tog, such that

wheneverx,y∈Oand [a,b]⊆[t0,to+σ].(we assume that the integral on the right-hand side exists).

Andg:[t0,t0+σ]→Risanondecreasingfunction, P={xt:x∈O,t∈[t0, t0+σ]}⊂ H0,H0⊂ G((-∞,0],Rn) is a Banach space equipped with a norm denoted by ‖·‖. We assumeH0satisfies the following conditions (H1)-(H6):

(H1)H0is complete.

(H2) Ifx∈H0andt<0, thenxt∈H0.

(H3) There exists a locally bounded functionk1:(-∞,0]→R+suchthatifx∈H0andt≤0,then‖x(t)‖≤k1(t)‖x‖.

(H4)Thereexistsafunctionk2: (0,∞) →[1,∞)suchthatifσ > 0andx∈H0isafunctionwhosesupportiscontainedin[-σ,0],then

(H5) There exists a locally bounded functionk3:(-∞,0]→R+suchthatifx∈H0andt≤0,then

(H6)Ifx∈H0,thenthefunctiont |→‖xt‖isregulatedon(-∞,0].

t0∈R,σ>0,O⊂Ht0+σis a space satisfying conditions 1)-6) of Lemma 2.7.G((-∞,0],Rn)denotesthesetofallregulatedfunctionsf:(-∞,0]→Rn.

Our main result is to derive the differentiability of solutions with respect to initial conditions for measure function differential equations with infinite delay.

2 Preliminaries

We start this section with a short summary of Kurzweil integral.

Letδ:[a,b]→R+beafunction,andτbeapartitionofinterval[a,b]withdivisionpointsa=α0≤α1≤…≤αk=b.Thetagsτi∈[αi-1,αi]iscalledδ-fineif[αi-1,αi]⊂[τi-δ(τi),τi+δ(τi)],i=1,2,…,k.

Definition2.1[2]Amatrix-valuedfunctionF:[a,b]×[a,b]→Rn×mis called Kurzweil integrable on [a,b], if there is a matrixI∈Rn×msuchthatforeveryε>0,thereisagaugeδon[a,b]suchthat

AnimportantspecialcaseistheKurzweil-Stieltjesintegralofafunctionf:[a,b]→Rnwith respect to a functiong:[a,b]→R, which corresponds to the choice

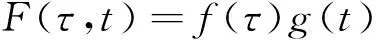
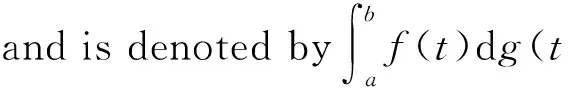
Definition 2.2[1]G⊂Rn× R,(x,t)∈G, a functionx:[a,b]→Bis called a solution of the generalized ordinary differential equation

(7)

whenever

Definition 2.3[8]LetXbe a Banach space. Consider a setO⊂X. A functionF:O×[t0,t0+σ] →Xbelongs to the classF(O × [t0,t0+σ] ,h,k),ifthefollowingconditionsaresatisfied:

(F1)Thereexistsanondecreasingfunctionh:[t0,t0+σ]→R such thatF:O×[t0,t0+σ] →Xsatisfies

for everyx∈Oands1,s2∈[t0,t0+σ],

(F2) There exists a nondecreasing functionk:[t0,t0+σ]→RsuchthatF:O×[t0,t0+σ] →Xsatisfies

(9)

foreveryx,y∈Oands1,s2∈[t0,t0+σ],

Lemma 2.2[2]LetU:[a,b]×[a,b]→Rn×nbeaKurzweilintegrablefunction,assumethereexistsapairoffunctionsf:[a,b]→Rnandg:[a,b]→Rsuchthatfisregulated, gisnondecreasing,and

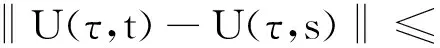
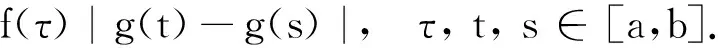
(10)

Then

Lemma2.3[9]AssumethatU:[a,b]×[a,b]→Rn×mis Kurzweil integrable andu:[a,b]→Rn×misitsprimitive,i.e.,

IfUisregulatedinthesecondvariable,thenuisregulatedandsatisfies

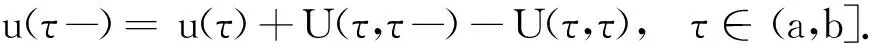
Moreover,ifthereexistsanondecreasingfunctionh:[a,b]→R such that

then

Lemma 2.4[9]Leth:[a,b]→[0,+∞) be a nondecreasing left-continuous function,k>0,l≥0. If thatψ:[a,b]→[0,+∞) is bounded and satisfies

thenψ(ξ)≤kel(h(ξ)-h(a))for everyξ∈[a,b].

Lemma 2.5[2]Assume thatF:[a,b]×[a,b]→Rn×nsatisfies(8).Lety,z :[a,b]→Rnbe a pair of functions such that

Then,zis regulated on [a,b].

Lemma 2.6[2]Assume thatF:[a,b]×[a,b]→Rn×nisKurzweilintegrableandsatisfies(8)withaleft-continuousfunctionh.Thenforeveryz0∈Rn, the initial value problem

(12)

has a unique solutionz:[a,b]→Rn.

Toestablishthecorrespondencebetweenmeasurefunctionaldifferentialequationsandgeneralizedordinarydifferentialequations,wealsoneedasuitablespaceHaofregulatedfunctionsdefinedon(-∞,a],wherea∈R, the next lemma shows that the spacesHainherit all important properties ofH0.

Lemma 2.7[8]IfH0⊂G((-∞,0],Rn)isaspacesatisfyingconditions1)-6),thenthefollowingstatementsaretrueforeverya∈R:

1)Hais complete; 2) Ifx∈Haandt≤a, thenxt∈H0; 3) Ift≤aandx∈Ha, then ‖x(t)‖≤k1(t-a)‖x‖; 4) Ifσ> 0 andx∈Ha+σis a function whose support is contained in [a,a+σ], then

5) Ifx∈Ha+σandt≤a+σ, then ‖xt‖≤k3(t-a-σ)‖x‖; 6) Ifx∈Ha+σ, then the functiont|→‖xt‖is regulated on (-∞,a+σ].

Theorem 2.8[8]Assume thatOis a subset ofHt0+σhaving the prolongation property fort≥t0,P={xt:x∈O,t∈[t0,t0+σ]},Ø∈P,g:[t0,t0+σ]→Risanondecreasingfunction, f:P×[t0,t0+σ]→Rnsatisfies conditions (A), (B), (C), andF:O×[t0,t0+σ]→G((-∞,t0+σ],Rn)givenby(13)hasvaluesinHa+σ.Ify∈Oisasolutionofthemeasurefunctionaldifferentialequation

then the functionx:[t0,t0+σ]→Ogiven by

is a solution of the generalized ordinary differential equation

Wherextakes values inO, andF:O×[t0,t0+σ]→G((-∞,t0+σ],Rn)isgivenby

(13)

for everyx∈Oandt∈[t0,t0+σ].

Proof The statement follows easily from Theorem 3.6 in [8]

3 Main result

Now, we discuss the differentiability theorem of solutions with respect to initial conditions for equation (5).

Theorem 3.1 Letf:P×[t0,t0+σ]→RnbeacontinuousfunctionwhosederivativefxexistsandiscontinuousonP×[t0,t0+σ],andsatisfiestheaforementionedconditions(A)-(C),whereP={xt:x∈O, t∈[t0, t0+σ]}⊂ H0,andH0⊂ G((-∞,0],Rn) be a Banach space satisfying the aforementioned conditions (H1)-(H6),t0∈{R},σ>0, O⊂ Ht0+σ.Ifg : [t0,t0+σ]→R is a nondecreasing function andλ0∈Rl,σ>0,Λ={λ∈Rl; ‖λ-λ0‖<σ},x0:Λ→O× [t0,t0+σ] for everyλ∈Λ, the initial value problem of the measure functional differential equations with infinite delay (5) is equivalent to the initial value problem

(14)

then (14) has a solution defined on [t0,t0+σ]. Letx(t,λ) be the value of that solution att∈[t0,t0+σ].

Moreover, let the following conditions be satisfied:

1) For every fixedt∈[t0,t0+σ], the functionx|→F(x,t) is continuously differentiable onO× [t0,t0+σ].

2) The functionx0is differentiable atλ0.

Then the functionλ|→x(t,λ) is differentiable atλ0, uniformly for allt∈[t0,t0+σ]. Moreover, its derivativeZ(t)=xλ(t,λ0),t∈[t0,t0+σ] is the unique solution of the generalized differential equation

(15)

Proof Our proof is based on the idea from [2].

According to the assumptions, there exist positive constantsA1,A2such that

for everyx,y∈O,t∈[t0,t0+σ], andt0≤t1

for everyx∈O, the fourth statement of Lemma 2.7 implies

where

by the fifth statement of Lemma 2.7. The last expression is smaller than or equal to

where

i.e.,Fx∈F(O × [t0,t0+σ],h,k).

BecauseofO × [t0,t0+σ]isclosed,accordingtothemean-valuetheoremforvectorvaluedfunctionandFx∈F(O× [t0,t0+σ],h,k)

(16)

By the assumptions, we have

According to the Lemma 2.3, every solutionxis a regulated and left-continuous function on [t0,t0+σ]. If Δλ∈Rlissuchthat‖Δλ‖<σ,then

where

By(16),weobtain

andbyusingLemma2.2,foreverys∈[t0,t0+σ],weobtain

Consequently,byusingLemma2.4,wehave

SowecanseethatwhenΔλ→0, x(s,λ0+Δλ)→x(s,λ0)uniformlyforalls∈[t0,t0+σ].

LetW(τ,t)=Fx(x(τ,λ0),t).BecauseFx∈F(O× [t0,t0+σ] ,h,k),W(τ,t) satisfies (16), by Lemma 2.6, (15) has a unique solutionZ:[t0,t0+σ]→Rn× n.ByusingLemma2.5,thesolutionisregulated.SothereexistsaconstantN>0suchthat‖Z(t)‖≤N,t∈[t0,t0+σ].ForeveryΔλ∈Rlsuch that ‖Δλ‖<σ, let

Next, we will prove that if Δλ→0, thenφ(r,Δλ)→0 uniformly forr∈[t0,t0+σ].

Letε>0 be given, there exists aδ>0 such that if Δλ∈Rland‖Δλ‖<σ,then

and

It is obvious that

where

Thus,

Because of the functionx|→F(x,t) is continuously differentiable onO×[t0,t0+σ] and the definition of theφ(r,Δλ), so for any givenε>0,t,s∈[t0,t0+σ], we have

and thus (usingFx∈F(O × [t0,t0+σ] ,h,k) )

Consequently

Finally,Gronwall’sinequalityleadstotheestimate

Sinceε→0+,wehavethatifΔλ→0,thenφ(r,Δλ)→0uniformlyforanyr∈[t0,t0+σ].

[1] KEllEY W G, PETERSON A C. The Theory of Differential Equations[M]. 2nd ed. New York:Springer-Verlag,2010.

[3] LAKSHMIKANTHAM V, BAINOV D D, SIMEONOV P S. Theory of Impulsive Differential Equations[M]. Singapore:World Scientific,1989.

[4] HILSHCER R, ZEIDAN V, KRATZ W. Differentiation of solutions of dynamic equations on time scales with respect to parameters[J]. Adv Dyn Syst Appl,2009,4(1):35-54.

[5] SCHMAEDEKE W W. Optimal control theory for nonlinear vector differential equations containing measures[J]. SIAM Control,1965,3(2):231-280.

[6] DAS P C, SHARMA R R. On optimal comtrols for measure delay-differential equations[J]. SIAM Control,1971,9(1):43-61.

[7] PURNA C D, RISHI R S. Existence and stability of measure differential equations[J]. Czechoslovak Math J,1972,22(97):145-158.

[12] VERHUST F. Nonlinear Differential Equations and Dynamical Systems[M]. 2nd ed. New York:Springer-Verlag,2000.

[13] KURZWEIL J. Generalized ordinary differential equations and continuous dependence on a parameter[J]. Czechoslovak Math,1957,82(7):418-449.

[14] 朱雯雯,徐有基. 带非线性边界条件的一阶微分方程多个正解的存在性[J]. 四川师范大学学报(自然科学版),2016,39(2):226-230.

[15] KURZWEIL J. Generalized ordinary differential equations[J]. Czechoslovak Math J,1958,83(8):360-389.

无限滞后测度泛函微分方程的解关于初值条件的可微性

李宝麟, 王保弟

(西北师范大学 数学与统计学院, 甘肃 兰州 730070)

利用广义常微分方程的解关于初值条件的可微性,考虑可以转化为广义常微分方程的无限时滞测度泛函微分方程，得到这类方程的解关于初值条件的可微性.

测度泛函微分方程; 解的可微性; Kurzweil 积分; 广义常微分方程

O175.12

A

1001-8395(2017)01-0061-07

2016-07-01

国家自然科学基金(11061031)

李宝麟(1963—)男，教授，主要从事常微分方程和拓扑动力系统的研究,E-mail:libl@nwnu.edu.cn

Foundation Items:This work is supported by National Natural Science Foundation of China (No.11061031)

10.3969/j.issn.1001-8395.2017.01.010

(编辑 陶志宁)

Received date:2016-07-01

2010 MSC：26A39; 30G30; 34A20; 34G10