Dynamics of Tripartite Entanglement and Intramolecular Energy in Symmetric Trimer Molecule
Di Yi,Xing-lin Jian,Xi-wen HouDepartment of Physics,Huazhong Normal University,Wuhan 430079,China(Dated:Received on July 13,2015;Accepted on August 22,2015)
Dynamics of Tripartite Entanglement and Intramolecular Energy in Symmetric Trimer Molecule
Di Yi,Xing-lin Jian,Xi-wen Hou∗
Department of Physics,Huazhong Normal University,Wuhan 430079,China
(Dated:Received on July 13,2015;Accepted on August 22,2015)
The dynamics of tripartite entanglement and intramolecular energy for one harmonic-and two anharmonic-vibrational modes in a symmetric trimer molecule is studied for various initial states,where the entanglement is quantified in terms of concurrence and the interacting energy among three modes is calculated to establish a link between entanglement and energy.It is shown that the concurrence and the interacting energy behave dominantly positive correlation for the localized state in the anharmonic-vibrational mode,while they are dominantly anti-correlated for the localized state in the harmonic-vibrational mode.The relation between bipartite entanglement and the energy in a subsystem is discussed as well.Those are useful for quantum computing and quantum information in high dimensional states prepared in polyatomic molecules.
Vibrational analyses,Tripartite entanglement,Quantum energy
I.INTRODUCTION
In recent years,considerable effort has been devoted to studying the dynamics of entanglement and energy in a composite system due to its important role in quantum information science.For example,the entanglement indicated by the von Neuamnn entropy[1],the linear entropy[2],the relative entropy[3],and so forth is intensively and extensively characterized for bipartite pure states[4].The dynamics of energy in two anharmonic oscillators is investigated in terms of classical and quantum mechanics[5],where explicit conditions are presented for the complete energy exchange via 1∶1 or 1∶2 resonance between two modes.Moreover,the connection between entanglement entropy and energy transfer is analyzed for integrable[6]and nonintegrable[7]systems.Recently,such connection has been further considered for various quantum states in small polyatomic molecules[8,9].It has been shown that entropy and energy can display dynamical correlation under suitable conditions[8,9].Additionally,the dynamics[10]and the control[11]of entanglement have been studied for other systems.Those investigations[5-11]are,however,concentrated on a bipartite system.It is thus interesting and necessary to explore the dynamical correlation between entanglement and energy in a multipartite system.
We investigate the dynamics of tripartite entanglement and intramolecular energy for vibrationally localized states in a symmetric trimer molecule[12],where the entanglement is measured by concurrence[13],and the intramolecular energy is given by the interacting energy among three vibrational modes in this molecule. Consequently,the relation between both quantities can be established for those states.What is more,the concurrence for a pure state is quantified in terms of quantum entropies of subsystems,which will be defined below.Such entropies not only present the information of individual subsystem,but also indicate the amount of bipartite entanglement between this subsystem and the remains of subsystems in the case of multipartite pure sates.That inspires us to compare the dynamics of bipartite entanglement with that of the energy in a subsystem.Hamiltonian are given for three vibrational modes in a symmetric trimer molecule in which the definitions of the concurrence and the interacting energy are presented.The time evolution of both quantities for various initial states with the localized excitations in single vibrational mode is discussed with the dislocalized excitations in all three vibrational modes.
II.MODEL AND DEFINITION

The Hamiltonian is given as[12]∶where β is the coupling inside the dimer(anharmonicvibrational modes 1 and 2)and γ is the coupling between the dimer and harmonic-vibrational mode 3. This Hamiltonian conserves the total boson numberand thus we can diagonalizeEq.(1)in the basis of eigenfunctions of N.The eigenfunctions are products of the number states|n〉of each degree of freedom,|n1〉⊗|n2〉⊗|n3〉=|n1,n2,n3〉,where niis the number of bosons in mode i.Moreover,the Hamiltonian is symmetric under permutation between modes 1 and 2.
The trimer model and its variants not only have been used for the description of vibrational spectra[14],but also have been extensively investigated for classical and quantum properties in a triple-well condensate.In particular,Flach and his coworkers have investigated quantum dynamics of localized excitations in a symmetric trimer molecule,showing that local excitations can be strongly localized forever,similar to their classical counterparts[12,15].Moreover,the dynamics of particle numbers in variants of such trimer model has been characterized in terms of quantum and semi-classical mechanics[16,17].Those studies are,however,limited to the dynamics of oscillations[16-18]in particle numbers for specific states.To the best of our knowledge,the dynamics of tripartite entanglement and the interacting energy is little known for a high-dimensional state in those models.
With regard to several measures of tripartite entanglement,we choose concurrence[13]to quantify tripartite entanglement in the system of interest for its wide applicability as well as its efficient simulation for pure states in an arbitrary tripartite system[13].In particular,the concurrence pure state|ψ〉is expressed in terms of the linear entropy of subsystems,which is defined as[13]∶
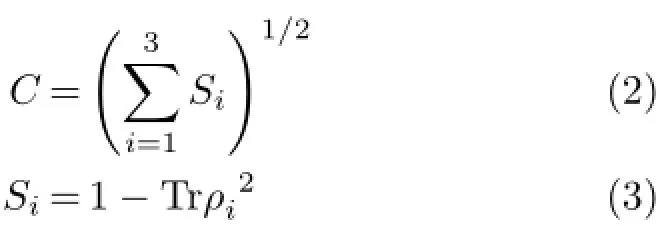
where Siis the linear entropy of subsystem i(i=1,2,and 3)and ρiis its density matrix,which is obtained via reducing other two subsystems from full density matrix
For a mixed state ρ the concurrence is given by∶

with the infimum being determined among all sets of probabilities pkand pure states∑〉,such thatBecause such an optimization is very difficult to solve exactly[13],the simulation of such concurrence for mixed states will have to wait for the development in the theory of both optimization and entanglement for multipartite systems.Nevertheless,our calculations that will be presented for high dimensional states can be regarded as an alternative with potential connections to actual experiments,other than the systems more usually used in the field of tripartite entanglement in three qubits[19].
In order to explore the dynamics of intramolecular energy,we are able to study the dynamics of population of an initial excitation[20],or directly investigate the time evolution of energy in every vibrational mode.For the energy of a vibrational subsystem we would like to inspect the mean value of the relevant restriction of the free Hamiltonian,
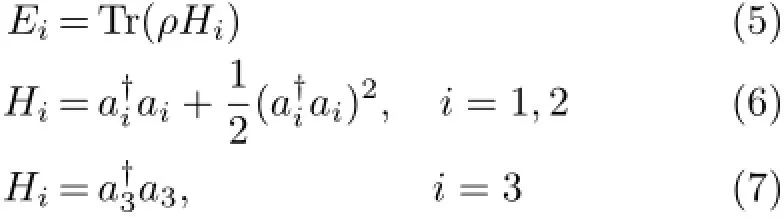
Therefore,of particular interest is the interacting energy,given as∶

where H is the total Hamiltonian given by Eq.(1).In what follows,we shall examine the dynamics of the interacting energy and the concurrence for several initial states in the trimer.Moreover,we shall discuss the dynamics of bipartite entanglement and the energy in the related subsystem.
III.DYNAMICS OF TRIPARTITE ENTANGLEMENT AND INTERACTING ENERGY
Once the model and the definitions of concurrence and energy are presented,we are able to inspect the time evolution of both quantities for an initial state. Of particular interest is the initial state taken to be the localized state with excitations in single mode and no excitations in other two modes.Therefore,we have two kinds of localized excitations in modes 1 and 3,respectively.Moreover,the molecule prepared in the localized state is more suitable for quantum computation[8],where the bipartite entanglement has been discussed.It is thus of interest to study the behaviors of tripartite entanglement for two kinds of localized states in the trimer.Additionally,we shall consider the results for a typical dislocalized state with the equal or nearly equal excitations in every mode.It is straightforward to discuss the dynamics of the concurrence and the interacting energy for other initial states.
For an initial state|ψ(0)〉and|ψ(t)〉evolves in time under the action of Hamiltonian Eq.(1)as∶

andtherelateddensitymatrixisavailableviaHence,we can examine the time evolution of the concurrence and the interacting energy,provided that the model parameters in Hamiltonian Eq.(1)are known.In the following numerical simulations,we take model parameters to be β=2.0 and γ=1.0,as done by Pinto and Flach[12].

FIG.1 Concurrence C(t)(solid line)and interacting energy E(t)(dashed line)for different initial states(a)|10,0,0〉,(b)|15,0,0〉,(c)|0,0,10〉,(d)|0,0,15〉,(e)|3,3,4〉,and(f)|5,5,5〉,where four former states are the localized states in modes 1 and 3,respectively,two latter states are the dislocalized states,and the energy is dimensionless and scaled.
Figure 1 shows concurrence C(t)and interacting energy E(t)in the trimer for different initial states |10,0,0〉,|15,0,0〉,|0,0,10〉,|0,0,15〉,|3,3,4〉,and |5,5,5〉,where two former pairs are the localized states in modes 1 and 3,respectively,the latter is the dislocalized state,and the energy is scaled.Some observations in Fig.1 are in order.
First,we consider the increasing rates of both quantities in the early-time evolution.For the same excitation,the increasing rate of the concurrence in the localized state in mode 1 is larger than that in the localized state in mode 3,while it is the largest in the dislocalized state |5,5,5〉(Fig.1(f)).As soon as a non-correlated state starts to evolve,the correlated states have contributed to the concurrence due to the interactions among three modes.That implies that the concurrence of the dislocalized state can be used to indicate chaos and decoherence in a multipartite system,as demonstrated in a bipartite system[21],which deserves future work. Moreover,for those three kinds of initial states the increasing rate of the concurrence increases with higher excitations.Remarkably,the increasing rate of the interacting energy is positive for the localized states in mode 1,while that is negative for the localized states in mode 3 and the dislocalized states,suggesting that the initial energy in mode 3 is transferred into the energy in other two modes via interactions.
Second,we discuss the difference in both quantities among those initial states.The time-evolution of both quantities can exhibit the irregular or quasi-periodic behavior that depends on an initial state,where the amplitude of the concurrence is the largest for the localized state,whereas that is the smallest for the dislocalized states.Moreover,both quantities for the localized state with high excitations in mode 1 can reveal more nicely quasi-periodic.However,the oscillation of the concurrence is more faster than that of the interaction energy. That can be regarded as an extension of previous work on the dynamical difference in bipartite entanglement for various pure states in small molecules[8,9].
Third,we examine the dynamical correlation of both quantities.We can observe that there are two kinds of correlations between the entanglement and the energy in Fig.1.The first kind of correlation means that both quantities rise and lower together as time evolves,while the second means that one quantity increases at the same time as another decreases or vice versa.It is seen that the concurrence and the interacting energy are dominant-positively correlated for the localized states in mode 1,while both quantities are dominantly anticorrelated for the localized states in mode 3.Such a positive-or anti-correlation indicates that the interacting energy can offer the information of tripartite entanglement.In particular,the energy and the concurrence have nearly the same step(see Fig.1(b)).However,the positive-and anti-correlated behaviors between both quantities become irregular for the dislocalized states,shown in Fig.1(e)and(f).That represents the first step forward to establish the relations of physical quantities in the trimer from quantum information theory and molecular physics.
Finally,it would be remarked that more calculationswe made for other initial states and model parameters show that the dominantly positive-or anti-correlations remain for the localized states in both modes 1 and 3. In particular,the higher the initial excitations are,the more distinct the positive-(anti-)correlation is for the localized states in mode 1(3)in the model with strong couplings.

FIG.2 Linear entropy Si(t)(solid line)and energy Ei(t)(dashed line)in different modes(a)and(b)i=1,(b)and(c)i=2,and(e)and(f)i=3 for two different initial states(a,c,e)|10,0,0〉and(b,d,f)|15,0,0〉with initial excitations in mode 1,where the interacting energy is scaled,and the initial energy is removed.
IV.DYNAMICS OF BIPARTITE ENTANGLEMENT AND ENERGY
The possibility of bipartitioning a multipartite system allows us to discuss the relationship between bipartite entanglement and energy.Actually,we have calculated the linear entropies of subsystems in the trimer. Those entropies describe the information of individual subsystem and also present the content of bipartite entanglement between this subsystem and other two subsystems in the case of pure states.It is thus of importance to study the dynamics of bipartite entanglement and the energy in the subsystem of interest.It should be mentioned that it has been analyzed the dynamics of bipartite entanglement in the special case of the trimer with parameter γ=0[22].However,the dynamical correlation between bipartite entanglement and energy for high dimensional pure states has not yet been considered in a tripartite system.
Figure 2 shows the dynamics of Siand Eiin different mode i,i=1,2,and 3 for two different initial state |10,0,0〉and|15,0,0〉with initial excitations in mode 1,where the interacting energy is scaled with different magnitudes,and the initial energy is removed.Comparing the left column with the right column in Fig.2,one can note the increasing rate of entropy in the earlytime evolution enhances as the initial excitation in mode 1 is higher,whereas the maximal entropy of bipartite entanglement decreases,and the entropy together with the rate in mode 1 is maximal.That suggests that bipartite entanglement in the tripartite system strongly depends on how to divide this system into two partitions,and initial excitations in one mode are helpful to the bipartite entanglement between this mode and remains.The increasing rate of energy in modes 2 and 3 in the early-time evolution behaves as the same as the corresponding entropy.However,the increasing rate of the energy in the mode 1 is negative,which implies that the energy in this mode is transferred into that in other two modes via the interactions among three modes.Moreover,entropy and energy in modes 2 and 3 are dominant-positively correlated,whereas the entropy in mode 1 is dominant anti-correlation with the energy in this mode.Such positive-or anti-correlation between entanglement entropy and energy is more distinct as the localized excitation in mode 1 is higher,which implies that the energy can offer the information of bipartite entanglement.
Figure 3 is similar to Fig.2,but here is for two different initial states|0,0,10〉and|0,0,15〉with initial excitations in mode 3.Note that for such states S2=S1and E2=E1due to the symmetry between mode 1 and 2.Contrary to the initial excitation in mode 1,the maximal entropy of bipartite entanglement increases as the initial excitation grows in mode 3.Moreover,the entropy in mode 1 is dominantly positive correlation withthe energy in this mode,while the entropy in mode 3 displays dominant anti-correlation with the energy in mode 3.However,the bipartite entanglement entropy is more irregular than the energy,which comes from their definitions.

FIG.3 Linear entropy Si(t)(solid line)and energy Ei(t)(dashed line)for two different localized states(a)and(c)|0,0,10〉and(b)and(d)|0,0,15〉with initial excitations in mode 3.
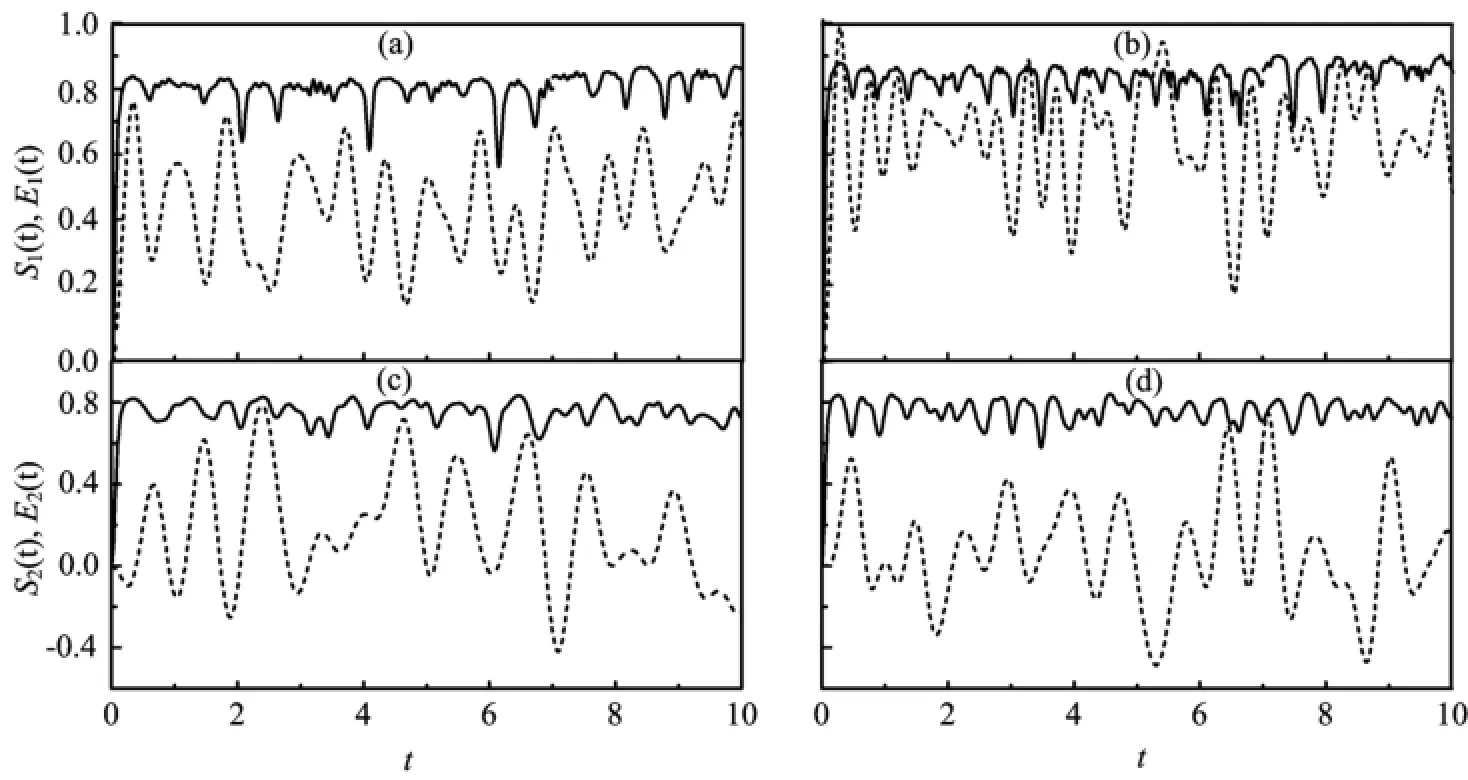
FIG.4 Linear entropy Si(t)(solid line)and energy Ei(t)(dashed line)for two typical dislocalized states(a)and(c)|3,3,4〉and(b)and(d)|5,5,5〉.
Figure 4 shows the same as in Fig.3,but here is for two typical dislocalized states(a,c)|3,3,4〉and(b,d)|5,5,5〉,and S2=S1and E2=E1since the model is invariant under permutation of modes 1 and 2.For the given total quantum number,the increasing rate of entropy in the early-time evolution for the dislocalized state is larger than that for the localized state,where such rate and the maximal entropy of bipartite entanglement increase with the increase of the total quantum number.However,the oscillations of entropy and energy are more irregular since the dislocalized state starts to interact with more states as soon as the time evolves. Differently from the localized state,the positive-or anti-correlation between entropy and energy occurs irregularly in turn for the dislocalized states|3,3,4〉and |5,5,5〉.
At last,we would like to state that more simulations we carried out for other initial states,and model parameters demonstrate that the above conclusions can be applied.Moreover,the positive-or anti-correlation between the bipartite entanglement entropy and the energy in a subsystem is more dominant for the localized state with higher excitations in individual mode in the trimer model with strong interactions.
V.CONCLUSION AND DISCUSSION
We have studied the dynamical properties of quantum entanglement and intramolecular energy for thelocalized state in an important trimer model.We have considered the tripartite and the bipartite entanglement measured in terms of concurrence[13]and the linear entropy[2],respectively,both of which are widely used in the theory of quantum information.The interacting energy and the energy in a subsystem are calculated for the construction of a possible connection between entanglement and energy.It is shown that the concurrence and the interacting energy are dominant-positively correlated for the localized states in mode 1,while they are dominantly anti-correlated for such states in mode 3.Moreover,as the trimer is bipartitioned,the linear entropy in an excited mode is dominantly positive correlation with the energy in this mode,while the linear entropy reveals dominant anticorrelation with the energy in other modes without any excitation.The positive-or anti-correlation between entanglement and energy is more dominant for the localized state with higher excitations in individual mode in the trimer model with strong interactions.However,such correlation happens irregularly in turn for the disloclized states.We believe that the correlated dynamics is useful for the understanding of the complicated entanglement for high dimensional states in tripartite molecules from bipartite entanglement and intramolecular energy.
It is worthwhile to employ those properties of bipartite and tripartite entanglement for molecular quantum computing[23].It is possible to discuss the dynamics of quantum correlation and coherence[24]in polyatomic molecules.
VI.ACKNOWLEDGMENTS
This work was supported by the National Natural Science Foundation of China(No.11174099).
[1]C.H.Bennett,H.J.Bernstein,S.Popescu,and B. Schumacher,Phys.Rev.A 53,2046(1996).
[2]J.I.Kim,M.C.Nemes,A.F.R.Toledo Piza,and H. E.Borges,Phys.Rev.Lett.77,207(1996).
[3]V.Vedral,M.B.Plenio,M.A.Rippin,and P.L. Knight,Phys.Rev.Lett.78,2275(1997).
[4]R.Horodecki,P.Horodecki,M.Horodecki,and K. Horodecki,Rev.Mod.Phys.81,865(2009).
[5](a)G.Kopidakis,S.Aubry,and G.P.Tsironis,Phys. Rev.Lett.87,165501(2001).(b)P.Maniadis and S.Aubry,Physica D 202,200(2005).
[6]D.Cavalcanti,J.G.Oliveira,J.G.P.de Faria,M. O.Terra Cunha,and M.F.Santos,Phys.Rev.A 74,042328(2006).
[7](a)X.W.Hou,M.F.Wan,and Z.Q.Ma,Eur.Phys. J.D 62,279(2011).(b)L.Zhai,Y.Zheng,and S.Ding,Chin.Phys.B 21,070503(2012).
[8](a)L.Zhai and Y.Zheng,Phys.Rev.A 88,012504(2013).(b)X.Meng and Y.Zheng,J.Phys.B 47,065501(2014).
[9](a)X.W.Hou,J.H.Chen,M.F.Wan,and Z.Q.Ma,J.Phys.A 42,075301(2009).(b)Z.Yang,X.Shen,and X.Hou,Chin.J.Chem.Phys. 28,295(2015).
[10](a)Z.G.Li,S.M.Fei,Z.D.Wang,and W.M.Liu,Phys.Rev.A 79,024303(2009).(b)X.Y.Hu,H.Fan,D.L.Zhou,and W.M.Liu,Phys. Rev.A 85,032102(2012).
[11]A.Abliz,H.J.Gao,X.C.Xie,Y.S.Wu,and W.M. Liu,Phys.Rev.A 74,052105(2006).
[12]R.A.Pinto and S.Flach,Phys.Rev.A 73,022717(2006).
[13](a)A.R.R.Carvalho,F.Mintert,and A.Buchleitner,Phys.Rev.Lett 93,230501(2004).(b)X.H.Gao,S.M.Fei,and K.Wu,Phys.Rev.A 74,050303(R)(2006).
[14](a)Q.S.Zhu,Chin.J.Chem.Phys.6,441(1993).(b)G.Wu,C.Zhang,and Z.Liu,Chin.J.Chem.Phys. 17,263(2004).(c)A.W.Liu,S.W.Hu,Y.Ding,and Q.S.Zhu,Chin. Phys.14,472(2005).
[15](a)S.Flach and V.Fleurov,J.Phys.:Condens.Matter 9,7039(1997).(b)S.Flach,V.Fleurov,and A.A.Ovchinnikov,Phys. Rev.B 63,094304(2001).
[16]V.Penna,Phys.Rev.E 87,052909(2013).
[17](a)T.F.Viscondi and K.Furuya,J.Phys.A 44,175301(2011).(b)N.J.Hui,L.H.Lu,X.Q.Xu,and Y.Q.Li,J. Phys.B 46,145305(2013).
[18]S.Rong,Q.Xie,and W.Hai,Phys.Lett.A 379,1881(2015).
[19](a)F.Anz´a,B.Militello,and A.Messina,J.Phys.B 43,205501(2009).(b)Z.G.Li,S.M.Fei,S.Albeverio,and W.M.Liu,Phys.Rev.A 80,034301(2009).(c)J.H.Huang,L.G.Wang,and S.Y.Zhu,Phys.Rev. A 81,064304(2010).
[20]M.Quack,Ann.Rev.Phys.Chem.41,839(1990).
[21]J.Gong and P.Brumer,Phys.Rev.A 68,022101(2003).
[22](a)X.W.Hou,J.H.Chen,and B.Hu,Phys.Rev.A 71,034302(2005).(b)Y.Liu,Y.Zheng,W.Ren,and S.Ding,Phys.Rev. A 78,032523(2008).(c)J.L.Zhang and J.M.Liu,Commun.Theor.Phys. 60,210(2013).
[23]L.K.McKemmish,R.H.McKenzie,N.S.Hush,and J.R.Reimers,J.Chem.Phys.135,244110(2011).
[24](a)Y.J.Li and J.M.Liu,Acta Phys.Sin.63,200302(2014).(b)X.W.Hou,Z.P.Huang,and S.Chen,Eur.Phys. J.D 68,87(2014).(c)L.H.Shao,Z.Xi,H.Fan,and Y.Li,Phys.Rev.A 91,042120(2015).
∗Author to whom correspondence should be addressed.E-mail:xwhou@phy.ccnu.edu.cn
 CHINESE JOURNAL OF CHEMICAL PHYSICS2016年2期
CHINESE JOURNAL OF CHEMICAL PHYSICS2016年2期
- CHINESE JOURNAL OF CHEMICAL PHYSICS的其它文章
- Raman Spectra of Liquid Nitromethane under Singly Shocked Conditions
- Tunneling Electron Induced Fluorescence from Single Porphyrin Molecules Decoupled by Striped-Phase Octanethiol Self-assembled Monolayer
- Ion Product of Pure Water Characterized by Physics-Based Water Model
- Laser Linewidth and Spectral Resolution in Infrared Scanning Sum Frequency Generation Vibrational Spectroscopy System
- Performances of Five Representative Force Fields on Gaseous Amino Acids with Different Termini
- Ab initio Study on Formation Mechanism of Spiro-Si-Heterocyclic Ring Compound Involving Ge from H2Ge=Si:and Formaldehyde
