Flight Dynamic Analysis of Hypersonic Vehicle Considering Liquid-Solid Coupling

Xu Xiaodong（徐晓东），Huang Yimin（黄一敏），Cai Xiaobin（蔡晓斌）

1.College of Automation Engineering，Nanjing University of Aeronautics and Astronautic，Nanjing 210016，P.R.China；

2.Research Institute of UAV，Nanjing University of Aeronautics and Astronautic，Nanjing 210016，P.R.China；

3.95835 PLA Troops，Malan 841700，P.R.China

Flight Dynamic Analysis of Hypersonic Vehicle Considering Liquid-Solid Coupling

Xu Xiaodong（徐晓东）1，2*，Huang Yimin（黄一敏）1，Cai Xiaobin（蔡晓斌）3

1.College of Automation Engineering，Nanjing University of Aeronautics and Astronautic，Nanjing 210016，P.R.China；

2.Research Institute of UAV，Nanjing University of Aeronautics and Astronautic，Nanjing 210016，P.R.China；

3.95835 PLA Troops，Malan 841700，P.R.China

With the liquid propellant making up 60%—70%of the takeoff weight of the hypersonic vehicle，the dynamic load caused by great propellant sloshing interacts with the flexible structure of the aircraft.Therefore，the dynamic model displays characteristics of strong coupling with structure/control and nonlinearity.Based on the sloshing mass dynamic simplified as a spring-mass-damping model，a rigid-flexible-sloshing model is constructed. Moreover，the effect on the dynamic performance of the coupled model is analyzed with changing frequency and damping.The results show that propellant sloshing dynamics significantly affects the rigid body motion modes，especially flexible mode and short mode.The right half plane pole（RHP）moves far from the imaginary axis with the consumption of the propellant.The flexible mode attenuates with the increase of the sloshing damping，and the coupling becomes stronger when sloshing frequency is close to the short mode frequency or the flexible frequency of the beam.

hypersonic vehicles；liquid propellant sloshing；coupling model；structure vibration

0 Introduction

Air-breathing hypersonic vehicle（AHV）such as X51 using the scramjet engine or a combination of multi-engines is a promising and costefficient technology for launching low-earth-orbit satellites and providing rapid global-response capabilities，which has received much attention especially in commercial and military fields.It has also become a key issue in the next generation of hypersonic space transportation［1-2］.

The hypersonic vehicle is normally considered as the lifting-body configuration.The design is such that the forward fuselage of aircraft creates a bow shock that acts as a compression system for the scramjet engine.The structural modes of this kind of vehicles also play an important role.Besides，the pressure on the fore body produces lift and a nose-up pitching moment.Downstream of the scramjet engine，the aircraft is shaped such that there is an external expansion of the exhaust gases［3］.The pressure on the aft body is a function of the pressure of the exhaust gas as it exits the scramjet engine and this pressure contributes to the lift of the vehicle.The vibrating of fuselage effectively changes the pressure distribution over the aircraft，and then brings additional perturbations with thrust，lift，drag，and pitching moment as well.Therefore，the dynamics of airbreathing hypersonic vehicles include great coupling between the scramjet propulsion and flight dynamics，in addition to the interactions between flexible and rigid modes.

Studies have shown that the hypersonic vehi-cle generally uses a combination of rockets and scramjet.The liquid propellant carried by the AHV makes up 60%—70%of the total weight［4］.Elight maneuvering causes great liquid propellant sloshing and induces hydraulic load，which has more impact on the structure and creates larger disturbance to the control system with the increasing proportion of the propellant to the total mass of the vehicle.On the one hand，a great deal of liquid propellant brings a large disturbing force and moment，which，once outside the limit that the control system can adjust，will cause the instability of flight；on the other hand，the hypersonic aircraft configuration like″waverider″with large slenderness ratio has a small mass coefficient of the fuselage structure and lower frequency of structure simultaneously［5］.When the vehicle vibration frequency is close to the liquid propellant sloshing frequency in the tank，the coupling effect may produce the fluid solid coupling vibration（such as POGO vibration）and then affect aircraft stability.Therefore it is necessary to establish a hypersonic flight dynamics model of multi physical field coupling，taking into account the effect of deformation and the dynamic coupling effect of the propellant mass distribution，consumption and periodic motion on the control system.

In recent years，a large amount of theoretical work has been carried out in this field to meet modern requirements for elaborate AHV model oriented to control system design.A comprehensive analytical model of the longitudinal dynamics of an AHV with an emphasis on dynamic/structure/propulsion was proposed［5］.The linearized aircraft dynamics is found to be unstable and exhibits non-minimum phase behavior.The two-dimensional simplified motion model with the propulsion coupling is constructed and based on the analysis of the scramjet，hypersonic aircraft short period mode and aero-servo-elasticity effect.The control-oriented model with the coupling with rigid/flexible mode was established and the influence of the coupling on the nonlinear control system was analyzed［6-7］.Liu established a parameterized model of hypersonic vehicles，which took into account the multidisciplinary integration including aerodynamic，propulsion and structure［8］.Bolender and Doman analyzed the influence of aerodynamic heating on the bending stiffness and elastic modulus affected by the aerodynamic heating effect［9-10］.But these studies do not consider the coupling effects of dynamic behavior of liquid propellant sloshing on hypersonic vehicle structure and the stability of flight.

An elaborate rigid/flexible/liquid/control dynamic model is built in this paper，which considers the periodic motion of liquid propellant.Then the vibration modes are compared and analyzed based on an equivalent model，an elastic beam model involving propellant sloshing.Einally the impact of propellant mass and the changes in the damping and frequency of propellant vibration on hypersonic dynamic stability is analyzed.

1 Structure Characteristics of Hypersonic Vehicle

The present paper takes an AHV as the research object，the geometric configuration of which is shown in Eig.1，where Lfis the length of fore-body，Lnthe nominal nacelle length，and Lathe length of aft-body.Other parameters are listed in Ref.［3］.

Eig.1 Hypersonic vehicle structure model

The geometric configuration is a typical representative of AHV:the scramjet engine is arranged in the lower abdomen of body.The fore body，as the inlet compression section，can make the gas experience multi-stage pre-compression. After the compression，inlet flow density and pressure can be increased；the lower wall of the aft body，as the external expansion section of thenozzle，can improve aerodynamic lift and propulsive efficiency without inducing drag.Due to the use of″waverider″aerodynamic characteristics of the aircraft body，the cross section from the nose to the middle of the fuselage gradually increases and that from the middle to the rear of the fuselage gradually decreases.Therefore the section moment of inertia changes gradually and the effect on body flexible modes should not be neglected. According to the description in Ref.［11］，mission equipment layout of AHV is shown in Eig.2.

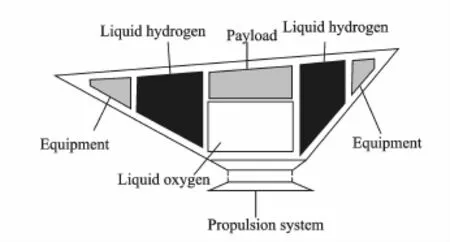
Eig.2 Notional internal volume layout

Different from the hypothesis in Ref.［11］，AHV fuselage structure will be equivalent to the beam with variable rectangle hollow section，the principal inertia axis of which is taken as the centroid to y.Based on the integrated design strategy of structure/thrust，the layout of the structure is as follows:the propulsion system is placed at the middle of the vehicle，and the head and rear parts serve as the additional structure of the compression and expansion sections of the propulsion system，respectively.So the section stiffness EI gradually changes in the longitudinal direction，as shown in Eig.3.

Eig.3 Change of cross-section EI along longitudinal

The main parameters run as follows

The additional aerodynamic and structural parameters were described in Ref.［11］.The vehicle body was simplified as a cantilever beam with mass center fixed.

2 Nonlinear Coupling Model of Hypersonic Vehicle

Eor liquid propellant dynamics，usually only the lower order is considered and the sloshing mass of the second order is only 3%of that of the first order.Also in the case of higher orders，the dynamics inside the liquid will produce turbulent disturbances and thus damping surge.Therefore the orders above the second can be ignored［12］. Assuming the liquid propellant is ideal incompressible fluid，equivalent mechanical model is generally used to analyze the dynamic behavior of liquid propellant.The model has the same kinetic behavior as the corresponding order sloshing.The aim of this paper is to analyze the effects of propellant dynamics on the structure and control system based on spring-mass-damping equivalent model.

Two kinds of strategy have been adopted to introduce the dynamic characteristics of liquid sloshing into elastic structure:（1）The propellant and flexible aircraft are modeled independently. Then the parameters of the sloshing dynamics，such as stiffness，damping and inertia are derived and coupled to a system model in the generalized coordinates，which is solved simultaneously.This approach is complex and can leak items easily；（2）In the analysis of the elastic deformation of the vehicle vibration，the influence of the characteristics of propellant sloshing is introduced to the model of flexible structure.Therefore，the deformation of elastic beam vibration with multiple sloshing mass distribution on the structure is calculated.In this paper，the conclusion is based on the second way and the vibration mode of elasticbeam containing sloshing mass is calculated with finite element method（EEM）.The AHV equivalent model with liquid sloshing is described in Eig.4，where m1，m2are the equivalent mass coefficients；k1，k2the equivalent stiffness coefficients，and c1，c2the equivalent damping coefficients，respectively.

Eig.4 AHV with liquid sloshing and its equivalent mechanical model

The continuous system of the equivalent free beam structure corresponding with the mass，the section moment of inertia and the elastic modules is divided into 500 beam elements along the longitudinal direction.The liquid propellant is simplified as concentrated mass with shaking，and the establishment of spring element，mass element and damping element is deduced.The inertial force and aerodynamic force are put on the unit as the load，and then the element equilibrium equation represented by nodal displacement is obtained.

where m is the mass matrix，c the damping matrix，k the stiffness matrix，δthe displacement matrix，and F the load matrix.

Through coordinate transformation，the local differential equations of unit are assembled into an equilibrium equation under the global coordinate and the global variable matrixes are capitalized

The generalized eigenvalue problem is solved with the calculus of variations for energy and the natural frequency and modal shape of the sloshing mass free beam are obtained.Eig.5 shows the first three-order modes of the two beams，with one characterized by even distribution without coupling and the other containing sloshing mass coupling.

Eig.5 Comparison of the first three mode shape

The first three modes are compared in the two cases:even mass distribution［11］and equivalent elastic beam as mass-spring model，as shown in Table 1.The first three-order vibration frequency of equivalent elastic beam including liquid sloshing increases by about 16%and is closely related to the mass distribution.The elaborate AHV model must consider the liquid sloshing because the sloshing changes the mode of vibration of the elastic beam and thus indirectly affects the vehicle dynamics.It is necessary to establish a nonlinear dynamic model coupled with rigid/flexible body-liquid-control，which can describe the complex dynamic behavior of hypersonic airplane system.

Table 1 Comparison of frequencies of the first three modes

The analysis is based on the following hypotheses:

（1）The frequency of rigid body moving in a wide range rarely couples with elastic beam，and the effect is mainly imposed through the interaction between structure deformations and that between loads.

（2）The tank of propellant is rigid and propellant sloshing does not couple with the rigid tank.The dynamic load derived from propellant sloshing acts on the aircraft structure.

With the aircraft flexible mode and propellant sloshing coupling aerodynamic taken into account，the hypersonic aircraft flight dynamics model is expressed by Eq.（3）

Andδ（x-xi）is Dirac function，which is satisfied for

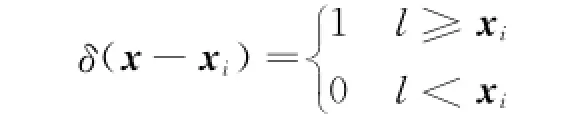
The aerodynamic force，moment and thrust were calculated with the fitted model given in Ref.［13］，which used the oblique shock theory based on the Rayleigh flow principle to estimate the hypersonic scramjet engine thrust.And the lift，drag and pitch moment were obtained with the Prandtl-Meyer expansion theory.The calculation formula is as follows

The above modeling process shows that，when the AHV states change，the additional item of inertia force caused by sloshing is added to the flexible model，which reflects the dynamic characteristics of elastic aircraft more accurately.

3 Analysis of Hypersonic Nonlinear Dynamics Model

To study the open-loop dynamics，the vehicle is trimmed in level flight at Mach 8 and altitude of 25.9 km.The balance states and dynamic characteristics are analyzed with the nonlinear dynamics model with the assumption that the mass of the rigid beam is distributed evenly and that equivalent elastic beam contains liquid sloshing mass. u=［δe，φ］Tis the control input，whereδeis elevator angle andφequivalent fuel ratio.The output variable y=［V，γ］Tis the speed and flight path angle.By trimming the open-loop transfer function，poles and zeros are calculated and shown in Table 2.

The analysis of Table 2 shows:in contrast with the rigid body model，in the elastic coupling model three elastic vibration equations are added and it has nine eigenvalues representing highly modal，phugoid period mode，short period modeand aeroelastic modes.Time-varying state of aircraft movement is the superposition of all the modes.Table 3 gives the corresponding transfer function zeros and there is a non minimum phase zero inherent in the dynamic system.

Table 2 Open-loop poles at Mach 8 in 25.9 km level flight

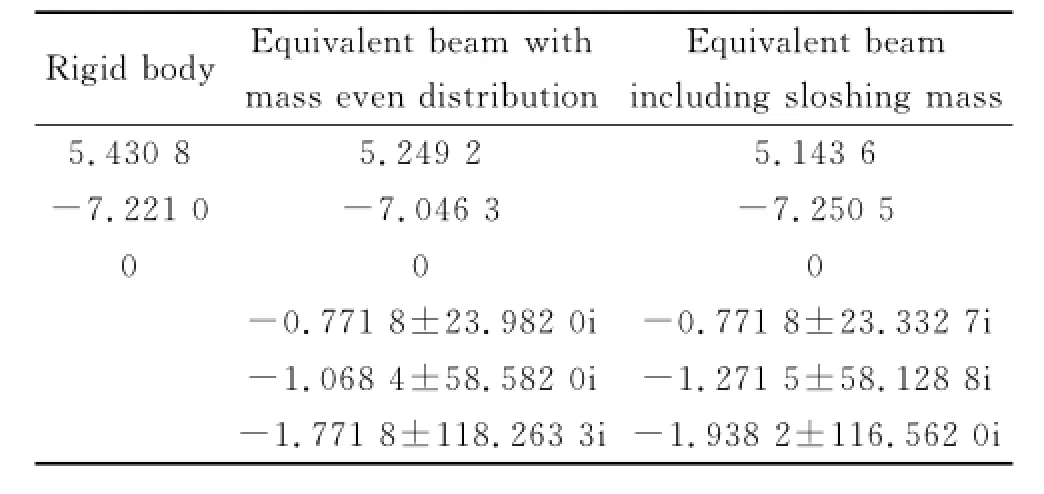
Table 3 Open-loop zeros at Mach 8 in 25.9 km level flight

The static stability and dynamic characteristics of a vehicle are determined by the zeros/poles′position of closed-loop system.If the liquid propellant sloshing is considered，the dynamic characteristics，especially the minimum phase of hypersonic vehicle system have changed.Through poles comparison，the poles of long period mode and the unstable poles of short period mode are increasing sequentially for rigid beam，even mass equivalent beam and the sloshing mass equivalent beam.Also the pole of the height modal moves close to the imaginary axis gradually and the stabilization time of height modal increases.In order to improve the maneuverability of the aircraft，it is necessary to relax static stability so that the vehicle has the right half plane poles（RHPs），whose position determines the lower limit of the bandwidth of the closed-loop control system.If the liquid sloshing effect is taken into consideration，the frequencies of the first to the third order of the aeroelastic modal decrease.The elastic vibration and rigid motion are coupled greatly. When the aeroelastic vibration frequency decreases，it narrows the bandwidth of the closed loop control system and may result in the bandwidth overlapping between structure and actuator，thus leading to control system instability.In addition，as the effect of liquid sloshing is concerned，the right half plane zero moves slightly towards the imaginary axis.To track the guidance signal，the control system is usually required to have cut-off frequency more than three times high as that of the guidance loop［14-17］.Therefore，the left-shifting zero of the closed-loop system reduces the frequency range，which is unfavorable to the stability of the system.

To evaluate the effect of the mass of liquid propellant consumption on the dynamic characteristics of the aircraft，the state equations are linearized with a cruise stage different separately with the fuel consumption ratio as a constant.The poles and zeros are calculated under the condition that liquid fuel consumption satisfies the linear distribution.The analytical results are shown in Eig 6.

Eig.6 Effect of poles/zeros due to liquid fuel consumption

The sloshing behavior of the liquid propellant affects the vibration modes of the elastic beam. Suppose further that 50%the liquid propellant remains，the zeros and poles are obtained by changing the damping and frequency of liquid sloshing in the finite element model.The zeros and poles of the distribution of the linear model are shown in Eigs.7，8.

Conclusions can be drawn from Eigs.6—8:

（1）With the decrease of liquid propellant mass，the modal frequency of vehicle vibration increases and the short period mode poles move slightly to the right half axis plane.The RHP zeros close to the imaginary axis move gradually away from the imaginary axis，having little effect on the stability of the open loop of the vehicle.At the same time，the RHP zeros have weaker control over the control system.All these increase the response speed of the system to a certainextent.

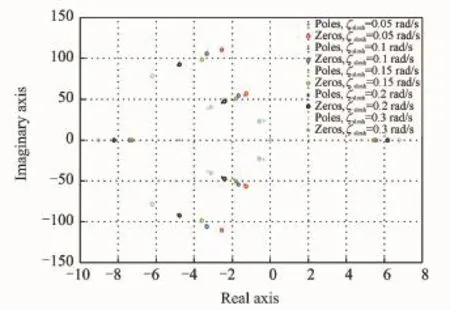
Eig.7 Effect of poles/zeros due to liquid propellant sloshing damping

Eig.8 Effect of poles/zeros due to liquid propellant sloshing frequency

（2）The influence of liquid propellant sloshing on the elastic vibration of the vehicle is analyzed.When the damping varies from 0.05 to 0.3，the zero moves away from the imaginary axis substantially.Damping increase can quickly stabilize the aircraft flexible mode and therefore reduce the coupling effect with rigid mode with limited impact on the other modes.

（3）Whenω=5 rad/s，the sloshing frequency of equivalent spring mass model is similar to that of the short period mode.The poles of short period mode move gradually close to the imaginary axis，which indictes that the coupling effects between the sloshing and the first-order short period mode.When the frequency continues to increase，the poles of short period change little.When the frequency increases toω≥20 rad/s，the poles of the first-order flexible mode move to the left and the stable time is reduced.When the right half plane zero shifts towards the imaginary axis，the bandwidth of the system is narrowed and therefore affects the stability significantly.Consequently enough attention must be paid to the bandwidth in the design of control system.

4 Conclusions

A nonlinear model involving aerodynamics，propulsion system and structural dynamics for airbreathing hypersonic vehicles has been presented，which considers variable section moment of inertia of elastic beam and liquid propellant sloshing influence.The vibration mode of equivalent elastic beam including the liquid sloshing is calculated with numerical method.The zeros and poles are compared under different conditions，and the following conclusions are drawn:

（1）Liquid propellant motion affects the dynamic characteristics of AHV short period when the sloshing frequency is lower than the natural frequency.With the reduction of the propellant and the increase of the damping of liquid propellant sloshing，the zero on the right half plane moves away from the imaginary axis gradually，but the variation is slow and the zero remains close to the imaginary axis，which limits the bandwidth of the control system.

（2）When the frequency of the propellant sloshing is close to that of the vibration，the poles of short period move towards the imaginary axis and may lead to serious instability.When the sloshing frequency increases further，the poles of the first-order elastic modal shift to the left and the stable response time is reduced.In the meantime the right half plane zero shifts close to the imaginary axis and the bandwidth of the control system is decreased.Therefore，in the conceptual design of hypersonic aircrafts great attention must be paid to the undesirable effects of the liquid propellant sloshing on the flight dynamic characteristics.

Eurther research will consider the effects of the disturbance and changes in the zeros and polescaused by the propellant sloshing in the system. A modern robust controller will be designed to meet the strict requirements for the bandwidth and stability of the AHV system.

Acknowledgement

This work was supported by the Eundamental Research Eunds for the Central Universities（No. NS2015097）.

［1］ Eiorentini L，Serrani A，Bolender M A，et al.Nonlinear robust adaptive control of flexible air-breathing hypersonic vehicles［J］.Journal of Guidance，Control，and Dynamics，2009，32（2）:401-416.

［2］ Su E L，Luo J J.Modeling and analysis of hypersonic vehicle considering variable cross-section moment of inertia and aerodynamics heating［J］.Journal of Astronautics，2012，33（6）:690-697.（in Chinese）

［3］ Bolender M A，Doman D B.Nonlinear longitudinal dynamics model of an airbreathing hypersonic vehicle［J］.Journal of Spacecraft and Rockets，2007，44（2）: 374-386.

［4］ Abramson H N.The dynamic behavior of liquids in moving containers［R］.NASA SP-106，1966.

［5］ Wu Z G，Chu L E，Yang C，et al.Study on aeroservoelasticity of hypersonic vehicle with thrust coupling［J］.Acta Aeronoutica et Astronautica Sinica，2012，33（8）:1555-1363.（in Chinese）

［6］ Sigthorsson D O.Control-oriented modeling and output feedback control of hypersonic air-breathing vehicles［D］.Ohio:The Ohio State University，2008.

［7］ Rodriguez A A，Dickeson J J，Srid-haran S，et al. Control-relevant modeling，analysis，and design for scramjet-powered hypersonic vehicles［R］.AIAA Paper 2009-7287，2009.

［8］ Xiao Dibo，Liu Yanbin，Lu Yuping，et al.Optimization on design of integrated control for hypersonic vehicles［J］.Journal of Nanjing University of Aeronautics and Astronautics，2012，45（6）:752-762.（in Chinese）

［9］ Williams T，Bolender M A，Doman D B，et al.An aero-thermal flexible mode analysis of a hypersonic vehicle［C］∥Proc of AIAA Atmospheric Elight Mechanics Conference and Exhibit.Colorado，USA: AIAA，2006:21-24.

［10］Bolender M A，Doman D B.Modeling unsteady heating effects on the structural dynamics of a hypersonic vehicle［C］∥Proc of AIAA Atmospheric Elight Mechanics Conference and Exhibit.Colorado:AIAA，2006:21-24.

［11］Li J L，Tang Z G，Eeng Z W，et al.Modeling and analysis of a hypersonic vehicle with aeroelastic effect［J］.Journal of National University of Defense Technology，2013，35（1）:7-11.（in Chinese）

［12］Xu Y W.Control system（Part 2）［M］.Beijing:Astronautics Press，1989:489-496.

［13］Xiang J W，Zeng K C，Nie L.Elastic effects on flight mechanics of waverider［J］.Journal of Beijing University of Aeronautics and Astronautics，2012，38（10）: 1306-1310.（in Chinese）

［14］Yang Jun，Yang Chen，Duan Chaoyang.Modern guided missile control system［M］.Beijing:Aviation Industry Press，2005:29-30.

［15］Poulain E，Chatillon O.Nonlinear control of airbreathing hypersonic vehicle［C］∥16th AIAA/DLR/ DGLR Inter-national Space Planes and Hypersonic Systems and Technologies Conference.Bremen，Germany:［s.n.］，2009:19-22.

［16］Clark A D，Mirmirani Maj Dean，Wu C，et al.An aero-propulsion integrated elastic model of a generic airbreathing hypersonic vehicle［R］.AIAA Paper 2006-6560，2006.

［17］Doyle J，Erancis B，Tannenbaum A.Eeedback control theory［M］.London，UK:McMillan Publishing Co.，1990:159-164.

（Executive editor:Zhang Tong）

V271.9 Document code:A Article ID:1005-1120（2015）01-0081-08

*Corresponding author:Xu Xiaodong，Associate Researcher，E-mail:xdxu@nuaa.edu.cn.

How to cite this article:Xu Xiaodong，Huang Yimin，Cai Xiaobin.Elight dynamic analysis of hypersonic vehicle considering liquid-solid coupling［J］.Trans.Nanjing U.Aero.Astro.，2015，32（1）:81-88.

http://dx.doi.org/10.16356/j.1005-1120.2015.01.081

（Received 4 November 2014；revised 24 December 2014；accepted 25 December 2014）