Influence Predicrions of Conracr Effecrs on Mesh Sriffness of Face Gear Drives wirh Spur Gear
Li Zhengminqing(李政民卿)*,Wang Jing(王晶),Zhu Rupeng(朱如鹏)
College of Mechanical and Electrical Engineering,Nanjing University of Aeronautics and Astronautics,Nanjing 210016,P.R.China
(Received 18 November 2014;revised 2 January 2015;accepted 12 January 2015)
Influence Predicrions of Conracr Effecrs on Mesh Sriffness of Face Gear Drives wirh Spur Gear
Li Zhengminqing(李政民卿)*,Wang Jing(王晶),Zhu Rupeng(朱如鹏)
College of Mechanical and Electrical Engineering,Nanjing University of Aeronautics and Astronautics,Nanjing 210016,P.R.China
(Received 18 November 2014;revised 2 January 2015;accepted 12 January 2015)
Absrracr:Mesh stiffness is one of important base parameters of face gear dynamic studies.However,a calculation solution of mesh stiffness of face gear drives is not to be constructed due to complex geometric flakes of face gear teeth.Thus,a calculation solution of mesh stiffness of face gear drives with a spur gear,which is based on the proposed equivalent face gear teeth and Ishikawa model,is constructed,and the influence of contact effects on mesh stiffness of face gear drives is investigated.The results indicate the mesh stiffness of face gear drives is sensitive to contact effects under heavy loaded operating conditions,specially.These contributions will benefit to improve dynamic studies of face gear drives.
face gear drive;mesh stiffness;contact effect;equivalent tooth;Ishikawa model
0 Inrroducrion
A face gear drive can be defined as a kind of intersection gear drives with a spur gear.One of the most important applications of face gear drives can be considered as first stage gear drives of helicopter main gear boxes,which is indicated by Litvin et al.[1-2]Typically,the rotation speed of first stage gear drives of helicopter main gear boxes is very fast due to the output speed of turbo shaft engines.Thus,face gear dynamic studies are focused by scholars.Jin et al.proposed a nonlinear dynamic model of face gear drives[3].Wang et al.investigated dynamic responses of face gear drives[4].Yang et al.studied vibration characterestics of face gear drives[5-6].Wang et al.discussed dynamic responses of face gear drives in a split transmission system[7].Zhang et al.examined natural frequencies of torsional vibrations in a torque-split face gear transmission system[8].Li et al.not only studied the influence of setting errors on contact points,but also assessed coupled vibrations of non-orthogonal face gear drives[9-10]. According to the limited published issues,some achievements of face gear dynamic studies are obtained.However,a calculation solution of mesh stiffness of face gear drives is not to be constructed due to complex geometric flakes of face gear teeth.Thus,in this paper,a calculation solution of mesh stiffness of face gear drives with a spur gear,which is based on the proposed equivalent face gear teeth and Ishikawa model,is constructed.Furthermore,the impact of contact effects on mesh stiffness of face gear drives is discussed. These contributions will be helpful to improve face gear dynamic studies in the future.
1 Numerical Model
A face gear tooth can be assumed as a sequence in which modification involute gears are superimposed along its face width,as shown in Fig.1.
In Fig.1,according to modification gear theories and engagements of face gear drives,modi-fication factors versus the extreme tooth radii,namely xi-limand xo-lim,and extreme inner and outer radii of face gear teeth,mean to ri-limand ro-lim,can be derived in a piecewise form as whereγis the shaft angle,αthe pressure angle,αt'the addendum pressure angle,zgthe tooth number of generation gears,zfthe tooth number of face gears,h*athe addendum coefficient,and c*the clearance coefficient.rfand rbare dedendum and base radii of generation gears,respectively;And a drive ratio for generations igfcan be expressed as
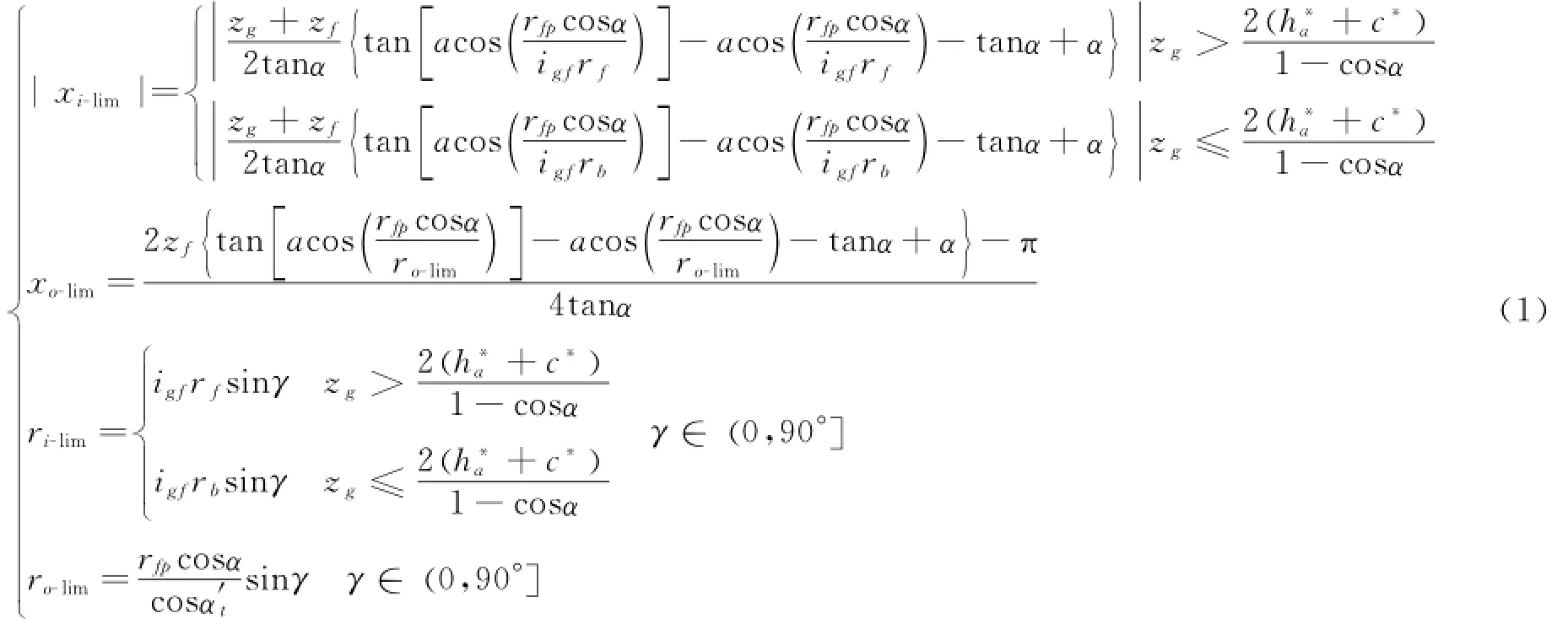
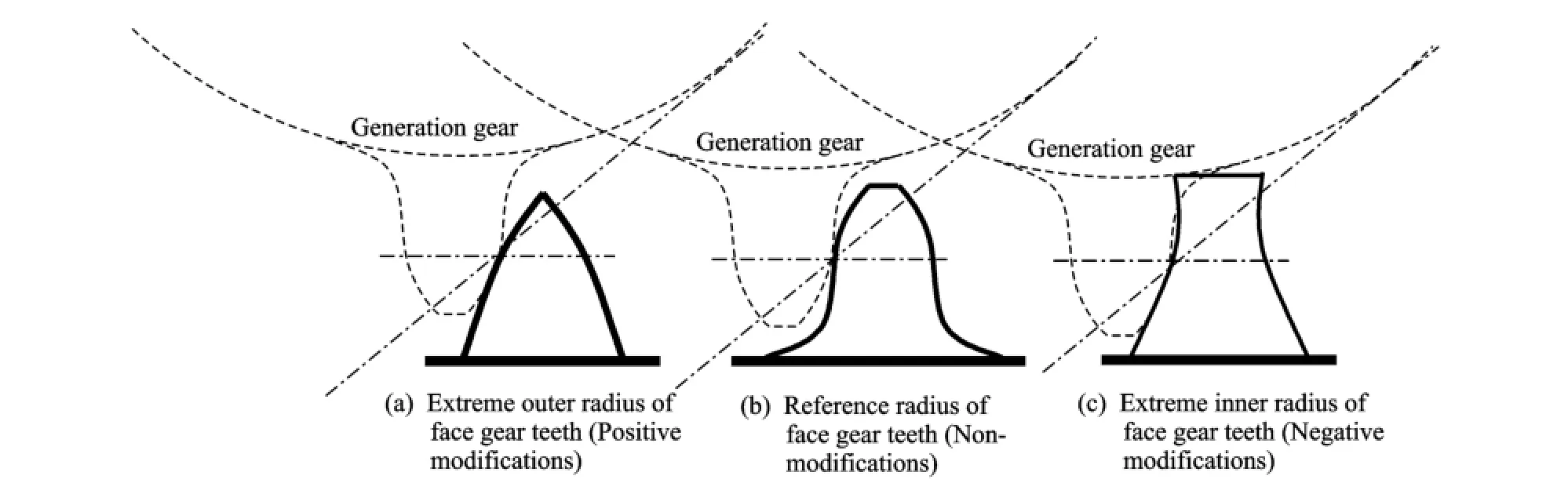
Fig.1 Sketch of face gear tooth changes

Moreover,a non-modified reference radius of face gears rfpmust be existed due to the range from positive modifications to negative modifications,and rfpcan be written in

where m is the modulus of face gear drives.
Due to avoiding offset loads in face gear drives,the tooth number of a pinion,which meshes with face gears,is always less one to three teeth than generation gears,which are used to generate face gears.Therefore,contact points of face gear drives must deviate from reference circles of face gears,and its modification factor can be given as
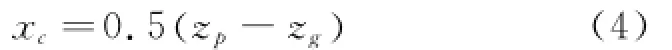
where zpis the tooth number of pinions.Meanwhile,the distance between reference circles and contact positions along face gear face widths can be derived by

Therefore,a contact radius on face gears can be deduces as

In addition,according to Hertz contact theories,long and short radii of contact ellipses of face gear drives can be expressed as[11]

whereρxis the long radius,ρythe short radius,and Fmthe load.ρpp,ρpf,ρfpandρffare the normal curvatures.u and v are the elliptic integral factors andθp/fcan be described as
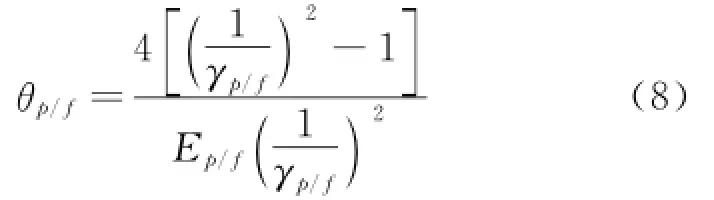
where E is the modulus of elasticity,andγthe Poisson ratio.Subscript p and f refer to the pinions and the face gears.
Thus,mesh stiffness of face gear drives considered contact effects can be assumed as a superposition of a series of mesh stiffness of modification spur gear drives,which can be calculated on Ishikawa model[12-13],along face gear face widths,and the range of modification factors can be derived by

2 Simularions and Analysis
Geometric parameters of an example case of face gear drives are listed in Table 1,which are employed to investigate the impact of contact effects on mesh stiffness.A face gear tooth and its contact points,which are based on the proposed tooth modeling and the geometric parameters listed in Table 1,are simulated,as shown in Figs.2,3,respectively.

Table 1 Geomerric paramerers

Fig.2 Face gear tooth simulation of the example case
Furthermore,in order to discuss the influence of contact effects on mesh stiffness,several operating conditions for this example case are listed in Table 2.
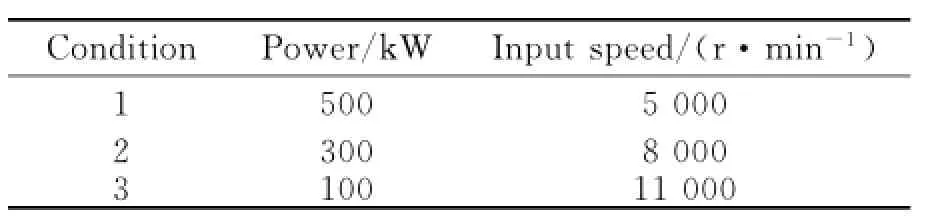
Table 2 Operaring condirions
Based on Eqs.(7,9),the maximum long radii of contact ellipses under three working conditions are calculated,as listed in Table 3.

Fig.3 Simulation of contact points on face gear teeth of the example case

Table 3 Maximum long radii
Using Ishikawa model[12-13]associated with the proposed face gear tooth modeling,and without considering contact effects,the results of time-varying mesh stiffness and average mesh stiffness of this example case are simulated,as given in Fig.4.
Employing Ishikawa model[12-13]associated with the proposed mesh stiffness solution of face gear drives,in which contact effects are considered,the results of time-varying mesh stiffness under three working conditions,which are conducted by fast Fourier transform(FFT),are simulated in Fig.5,and the results of average mesh stiffness under three operating conditions are calculated in Table 4.

Fig.4 Mesh stiffness without contact effects
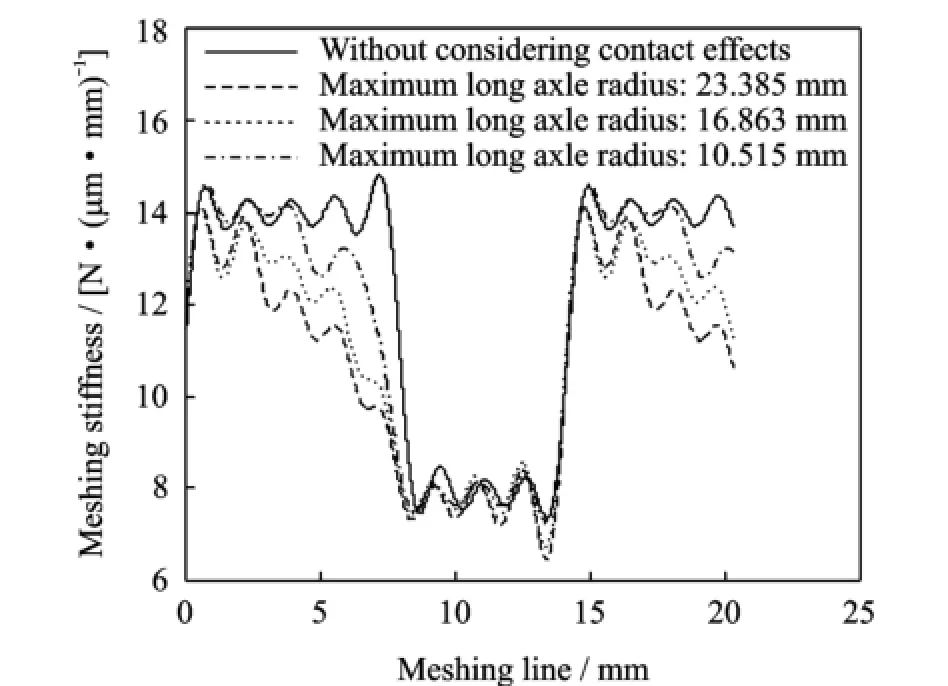
Fig.5 Comparison of time-varying mesh stiffness under different working conditions

Table 4 Average mesh sriffness wirh conracr effecrs
In the case of Figs.4,5,a part of timevarying mesh stiffness at the engagement area of multiple tooth pairs will be decreased with increasing long radii of contact ellipses,but the other part of time-varying mesh stiffness will not be adjusted almost.The difference of average mesh stiffness between without and with contact effects can be defined as
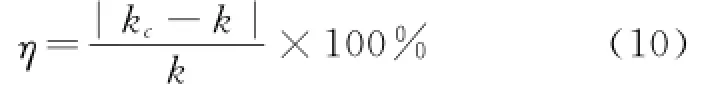
whereηis the difference,kcthe average mesh stiffness with contact effects,and k the average mesh stiffness without contact effects.
Based on Eq.(10),the differences of average mesh stiffness between without and with contact effects,under three operating conditions,are calculated in Table 5.
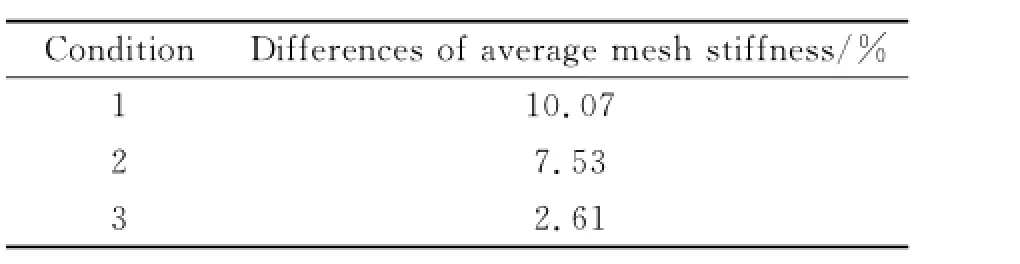
Table 5 Differences of average mesh sriffness
The results as listed in Table 5,indicate mesh stiffness of face gear drives is sensitive to contact effects.
3 Conclusions
In the paper,two important works are extracted as follows:
(1)A calculation solution of mesh stiffness of face gear drives associated with contact effects,which is based on the proposed equivalent face gear teeth and Ishikawa model,is constructed.
(2)The impact of contact effects on mesh stiffness is investigated.The results indicate time-varying mesh stiffness of face gear drives will be changed with operating condition adjustments,and the difference of average mesh stiffness between without and with contact effectsmay be greater than 10%.Thus,contact effects should be considered for face gear mesh stiffness,specially under heavy loaded operating conditions.
Acknowledgemenrs
This work was supported by the National Natural Science Foundations of China(Nos.51105194,51375226)and the Fundamental Research Funds for the Central Universities(No.NS2015049).
[1] Litvin F,Bossler R,Chen Y J,et al.Application of face-gear drives in helicopter transmissions[J]. Journal of Mechanical Design,1994,116(3):672-676.
[2] Litvin F,Egelja A,Tan J,et al.Handbook on face gear drives with a spur involute pinion[M].Chicago:DTIC,2000:54-101.
[3] Jin Guanghu,Zhu Rupeng,Bao Heyun.Nonlinear dynamical characteristics of face gear transmission system[J].Journal of Central South University,2010,41:1807-1813.(in Chinese)
[4] Wang Z,Chen Q,Lin J C,et al.Study of the dynamic behavior of face gear transmission system[J]. Applied Mechanics and Materials,2013,268/269/ 270:1063-1066.
[5] Yang Zhen,Wang Sanmin,Fan Yesen,et al.Bifurcation charactristics of face-gear transmission system[J].Journal of Harbin Institute of Technology,2011,43(3):107-110.(in Chinese)
[6] Yang Zhen,Wang Sanmin,Fan Yesen,et al.Vibration characteristics of face gear transmission system with parametric excitation[J].Journal of Chongqing University,2011,34(1):26-35.(in Chinese)
[7] Wang R,Zhao N,Tao L,et al.Floating shaft load sharing method for face gear split torque transmission system[J].Research Journal of Applied Sciences,Engineering and Technology,2013,5(12):3386-3392.
[8] Zhang Le,Zhu Rupeng,Li Zhengminqing.Research on natural frequency of torsional vibration of torquesplit face gear transmission system[J].Jiangsu Machine Building&Automation,2012,41(5):21-24.(in Chinese)
[9] Li X Z,Zhu R P,Li Z M Q,et al.Influence of setting error of tool on tooth profile and contact point of face gear drive[J].Transactions of Nanjing University of Aeronautics and Astronautics,2014,31(4):370-376.
[10]Li Xiaozhen,Zhu Rupeng,Li Zhengminqing,et al. Analysis of coupled vibration of face gear drive with non-orthogonal intersection[J].Journal of Central South University,2013,44(6):2274-2279.(in Chinese)
[11]Song Lemin.Tooth flank and strength of gear drive[M].Beijing:National Defence Industry Press,1987:164-174.(in Chinese)
[12]Shi J L,Ma X G,Xu C L,et al.Meshing stiffness analysis of gear using the Ishikawa method[J].Applied Mechanics and Materials,2013,401:203-206.
[13]Zhu Xiaolu,E Zhongkai.Analysis of load capacity of gears[M].Beijing:Higher Education Press,1992:25-42.(in Chinese)
(Executive Editor:Xu Chengting)
TH113Documenr code:AArricle ID:1005-1120(2015)05-0566-05
*Corresponding aurhor:Li Zhengminqing,Associate Professor,E-mail:lzmq-cmee@nuaa.edu.cn.
How ro cire rhis arricle:Li Zhengminqing,Wang Jing,Zhu Rupeng.Influence predictions of contact effects on mesh stiffness of face gear drives with spur gear[J].Trans.Nanjing U.Aero.Astro.,2015,32(5):566-570.
http://dx.doi.org/10.16356/j.1005-1120.2015.05.566
 Transactions of Nanjing University of Aeronautics and Astronautics2015年5期
Transactions of Nanjing University of Aeronautics and Astronautics2015年5期
- Transactions of Nanjing University of Aeronautics and Astronautics的其它文章
- Performance of Epoxy-Repaired Corroded Reinforced Concrere Beams
- Merhod for Evaluaring Insranraneous Secror Capaciry
- Solurion ro Beacon Conflicr Based on IEEE 802.15.4
- Topological Srrucrure of US Flighr Nerwork Based on Complex Nerwork Theory
- Design of Ballisric Consisrency Based on Leasr Squares Supporr Vecror Machine and Parricle Swarm Oprimizarion
- Average Incremenral Correlarion Analysis Model and Irs Applicarion in Faulr Diagnosis
