Measurement and Modeling for the Solubility of Hydrogen Sulfide in Primene JM-T*

LI Jie (李杰), LIN Xiao (林晓), NING Pengge (宁朋歌),**, CAO Hongbin (曹宏斌), ZHANG Yi (张懿)and John C. CrittendenResearch Center for Process Pollution Control, Institute of Process Engineering, Chinese Academy of Sciences, Beijing 100190, China

2Key Laboratory of Green Process and Engineering, Institute of Process Engineering, Chinese Academy of Sciences, Beijing 100190, China

3Graduate University of Chinese Academy of Sciences, Beijing 100049, China

4Brook Byers Institute for Sustainable Systems, Georgia Institute of Technology, Atlanta, GA 30332, USA

Measurement and Modeling for the Solubility of Hydrogen Sulfide in Primene JM-T*

LI Jie (李杰)1,2,3, LIN Xiao (林晓)1,2, NING Pengge (宁朋歌)1,2,**, CAO Hongbin (曹宏斌)1,2, ZHANG Yi (张懿)1,2and John C. Crittenden41Research Center for Process Pollution Control, Institute of Process Engineering, Chinese Academy of Sciences, Beijing 100190, China

2Key Laboratory of Green Process and Engineering, Institute of Process Engineering, Chinese Academy of Sciences, Beijing 100190, China

3Graduate University of Chinese Academy of Sciences, Beijing 100049, China

4Brook Byers Institute for Sustainable Systems, Georgia Institute of Technology, Atlanta, GA 30332, USA

The primary aliphatic amine Primene JM-T was investigated as a potential absorbent for H2S removal. The solubility of H2S in JM-T was measured at 298, 313, 333, 353, and 368 K with H2S partial pressures from 20 to 760 kPa and H2S loading from 0.02 to 0.8 mol H2S per mol JM-T. Relevant physical properties, such as density, viscosity and dielectric constant, of the material were measured. The thermodynamic model with two-suffix Margules equation was used to correlate the experimental vapor-liquid equilibrium data. Furthermore, the absorption mechanism in non-aqueous system is suggested and the difference between non-aqueous and aqueous absorption system is pointed out.

Primene JM-T, H2S removal, absorption, thermodynamic model

1 INTRODUCTION

The removal of hydrogen sulfide (H2S) is very important in many industrial processes, such as coke and coal gasification as well as natural gas and synthesis gas purification, to meet the requirements of the industrial process or environmental regulations. Many methods have been developed to remove H2S based on physical, chemical or biological process [1-3]. Among them, the chemical absorption of H2S with various aqueous alkanol amine solutions, such as the primary amine monoethanolamine (MEA) [4], secondary amine diethanolamine [5, 6] piperazine [7, 8], tertiary amine triethanolamine [9], methyldiethanolamine (MDEA) [10-12], and mixed amines [13-15], is the most widely used method. The physical absorption of H2S with brines also has been studied for geochemical applications [16, 17]. However, H2S capture technologies with various current amine absorbents are all in aqueous solutions. Conventional aqueous amine solutions have many disadvantages including corrosion and degradation. Moreover, the loss of volatile amines causes environmental pollution and raises the cost of operation process [18].

Recently, researchers have found that non-aqueous system has advantages in acid gas removal [19, 20]. The amine-functionalized cations and anions are tethered into ionic liquids to absorb acid gas with good result. Primene JM-T is a primary aliphatic amine with the amino nitrogen atom linked to a tertiary carbon. The highly branched alkyl group consists of a mixture of isomers in the C16-C22range. JM-T is insoluble in water and has many special physical and chemical properties, including excellent antioxidant ability and high thermal stability. Moreover, it can remain in liquid state from−50 °C to 220 °C [21]. Primene JM-T thus can potentially be used in the acid gas removal due to its good properties. However, the absorption of JM-T for acidic gases has not been reported. The evaluation of JM-T to be an absorbent needs fundamental solubility data.

Thermodynamic model is a useful tool for the industrial engineering design. There are three approaches for modeling of chemical absorption processes in industry. One approach is empirical, such as Kent and Eisenberg (KE) equation [22], with the chemical equilibrium constant expressed as a function of temperature, absorbent concentration and loading α. This method can fit the experimental data very well, but cannot predict those data out of the correlation. The second approach is to use the activity coefficient model to keep Kaas constant at definite temperature, such as E-NRTL model [23], Li-Mather equation [24] and Pitzer equation [25]. In Li-Mather equation, which is a simplified Clegg-Pitzer equation [26], there are long-range Debye-Hückel term to express the electrostatic interaction between ionic species and short-range term (three-suffix Margules equation) to show the van der Waals force between neutral species and between ionic and neutral species. The third one is to use the electrolyte equation of state to correlate the vaporliquid equilibrium (VLE) data [27]. These models can predict the VLE data outside the correlation. Recently, the Langmuir-type absorption model is also used to correlate the absorption properties for ionic liquids [19]. In this study, the second approach, which is a two-suffix Margules equation for non-aqueous systems, is chosen. This model is rather simple with lessparameters, can calculate the thermodynamic equilibrium constant and thermodynamic functions in the same time, and can be used in multi-component systems.

In this work, Primene JM-T is investigated as a potential absorbent for H2S removal. The solubility of H2S in Primene JM-T is measured at different temperatures and pressures. The experimental values of density, viscosity and dielectric constant of the absorbent are presented. Also, the chemical absorption reaction and a thermodynamic model are suggested.

2 EXPERIMENTAL SECTION AND RESULTS

2.1 Materials

Primary amine Primene JM-T was purchased from Rohm & Haas Company, US, which contains tertiary alkylamines (C16-C22) and octadecene (C18H36). Hydrogen sulfide and nitrogen gases both had the mole fraction purities higher than 0.999. All reagents were used without further treatment.

2.2 Apparatus and methods

Densities were measured in an Anton Paar DMA 5000 densitometer with an uncertainty of 5×10−6g·cm−3. Viscosities were measured in an Anton Paar AMVn automated microviscometer with an uncertainty of less than 0.1%. Dielectric constants were measured in Brookhaven BI-870 dielectric constant meter with an uncertainty of 2.0% in the range of 22 °C to 58 °C. The amine value defined as the moles of the amino group content per gram of sample was determined by acid-base titration with a known molar concentration of perchloric acid.

The apparatus used to measure the solubility of H2S is shown schematically in Fig. 1. The reactor (GCF-0.5, Dalian Automatic Control Equipment Factory, China) consisted of a cylindrical stainless steel tank (595 cm3by calibration) fitted with a magnetically coupled stirrer. The gas container was a 783 cm3stainless steel tank. Two thermometers (TM-301, AS ONE Co.) with an uncertainty of ±0.1 K were used to measure the temperature in the reactor and container. Two pressure transducers (2600T, ABB Ltd.) with an uncertainty of ±0.075% measured the pressures in the reactor and the gas container. A water bath was used to control the temperature of the reactor tank.

The H2S absorption experiment was carried out as follows. First, 200 cm3(25 °C) Primene JM-T was put into the reactor. A vacuum pump was used to remove the remaining air, and then N2gas was introduced into the reactor. The pressure in the reactor was stable after stirring at a speed of 200 r·min−1for approximately one hour. Then vapor-liquid equilibrium in the reactor was achieved, and the pressure was recorded as2NP. H2S gas was introduced into the gas container, for which the pressure, temperature and volume were P1, T1and Vct, respectively. The H2S gas was then transferred from the container to the reactor, and the corresponding pressure, temperature and volume of the container were P2, T2, and Vct, respectively. The temperature in the reactor was then adjusted to the desired level. VLE was achieved again after approximately 10 h, and the equilibrium pressure in the reactor was recorded as PT.

The Peng-Robinson cubic equation of state (P-R EOS) [28] is used to treat the non-ideal gas. The original equation is

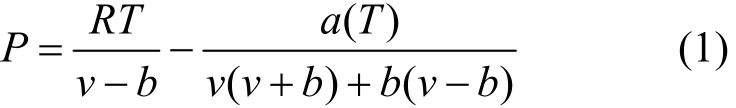
where v represents the molar volume of a gas at a particular pressure and temperature, which can be calculated using the P-R EOS if the volume, pressure and temperature of the gas phase of the system are known. The number of moles of gas in the system (n) can be calculated as

where V is the system volume occupied by the gas phase. The moles of the gas in the container before and after the introduction of H2S are defined as n1and n2. The gas introduced into the reactor is defined as nin.

Figure 1 Schematic diagram of the experimental equipment

In the reactor, when VLE is reached, with the assumption that the gas phase obeys Dalton’s law, the partial pressure of H2S (2HSP) can be determined by

According to the P-R EOS, the number of moles of H2S in the reactor is nH2S. The moles of H2S absorbed in 200 ml Primene JM-T (nab) at the pressure PH2Scan be calculated,

Using the moles of H2S dissolved in Primene JM-T (nab) and the total moles of amine in Primene JM-T introduced into the reactor (namine), the H2S loading (2HSα) in the liquid phase is defined as

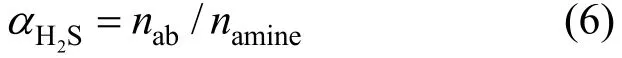
2.3 Experimental results

The densities, viscosities and dielectric constants of Primene JM-T are listed in Table 1. The viscosity values of JM-T are much lower than those of ionic liquids [19] and the dielectric constant values of JM-T are much lower than those of MDEA and MEA [24].

Table 1 Density, viscosity and dielectric constant of Primene JM-T

Temperature dependences of the density, viscosity and dielectric constant of the JM-T liquid are correlated from the experimental data. The correlated equations for ρ and D are empirical. Viscosity is correlated using the Vogel model expressed as

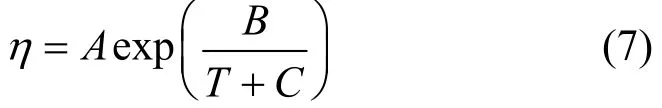
Here C=−86−0.19Tb, Tbis the boiling point and equals 493 K from reference [21], and A and B are fitted parameters. The correlated results are as follows.

The average absolute deviations of the correlation for density, viscosity and dielectric constant are 0.0397%, 0.74% and 0.118%, respectively.

The alkyl group of JM-T does not react withand the functional group is, so the JM-T is expressed asThe amine value(moleper gram) can be used to calculate the mole concentration of

In this paper, the amine value of JM-T is determined as 0.00284 mol·g−1. Thus the value of CRNH2 atcalculated fromis 2.37 mol·L−1. With the amine value mNH2 and the given molecular mass= 269, the effective componentand the inert componentcan be determined by the following equation,

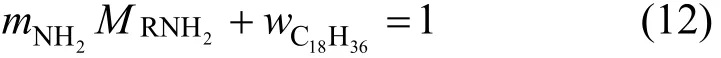
The uncertainty in measured temperature, pressure and volume were ±0.1K, ±0.075% and ±0.5%. The uncertainty in determining the amine value was estimated as ±1%. From Eqs. (3), (5) and (6), the uncertainty of H2S loading is determined by the following equation.

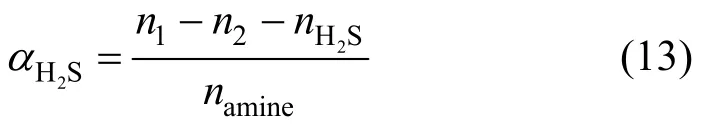
Figure 2 Solubility of H2S in 1.0 mol·L−1MDEA solution at 298 K■ this work; ● Maddox; ▲ Jou

The experimental VLE data of the solubility of H2S in Primene JM-T are listed in Table 2.

Table 2 Experimental solubility data of H2S in Primene JM-T

3 THERMODYNAMIC MODEL AND REACTION MACHANISM

3.1 Physical and chemical absorption equilibria

From Strus et al.’s study [31], Primene JM-T amines are not protonated and they can be considered as neutral substances. In this work, the electrical conductivity of the equilibrated liquid sample loaded with H2S was measured. Its value near zero means that the ionization of RNH2·H2S into ionic species can be neglected. Also, the dielectric constant for JM-T (Table 1) is very low, compared with these for the pure water, MEA and MDEA. Therefore, in JM-T liquid system, the ionization of the neutral species can hardly exist. In the RNH2·H2S non-aqueous system, the following equilibrium is proposed.

This is the main difference in chemical absorption reaction between non-aqueous and aqueous systems. There are quaternary systems in the liquid phase: (1) RNH2, (2) RNH2·H2S, (3) free H2S (liquid) and (4) C18H36. The chemical equilibrium constant K is expressed as

where χiand γiare mole fraction and activity coefficient for component i in the liquid phase, and2HSP is the equilibrated partial pressure of H2S.

There are two steps in the process: (1) H2S gas dissolves into the liquid phase [Eq. (16)], (2) dissolved H2S reacts with liquid RNH2[Eq. (17)].

The Henry constant H and the chemical equilibrium constant Kafor the two steps are described as follows

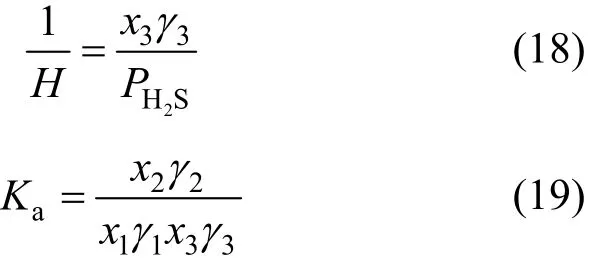
3.2 Two-suffix Margules equations

The two-suffix Margules equation expressed as follows [32, 33] is used to correlate the component activity coefficients γi,

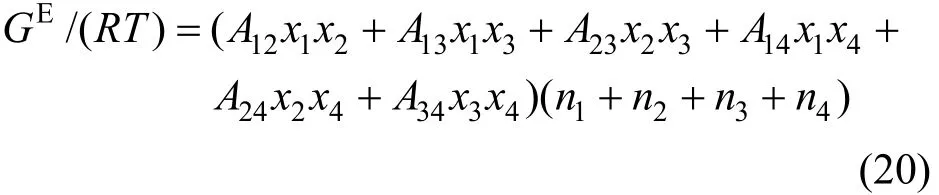
where Aijare the Margules interaction parameters, which represent the difference in the intermolecular forces between like and unlike molecules based on regular solution theory. Similar to the Pitzer’s primitive electrolyte model [34], the solvent molecules are not considered as interaction particles and are taken as medium. Thus the interaction parameters for A14, A24and A34are neglected in this work. The two-suffix Margules equation is simplified as

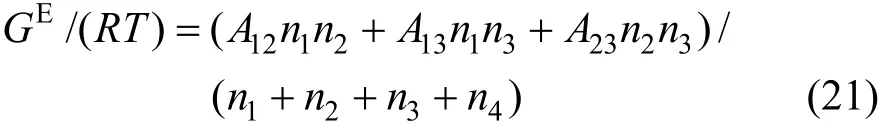
The activity coefficients are expressed as follows

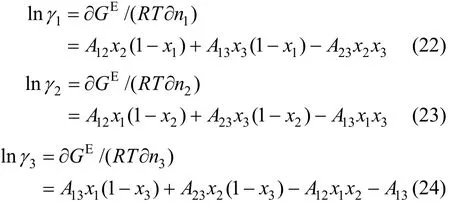
Table 3 Fitted values from the experimental data for JMT-H2S system

3.3 Parameter regression

In the above equations, H, K, Ka, A12, A13and A23are functions of temperature and can be expressed as

The values of K0, K1, H0, H1, a12, b12, a13, b13, a23, and b23are to be regressed by fitting VLE data and the results are listed in Table 3. The simplex program is used to solve the non-linear equations with the objective function

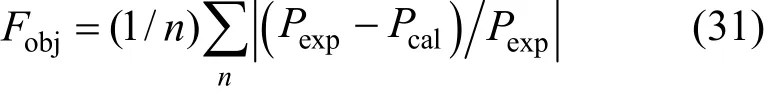
Here Pexpand Pcalrepresent the measured and calculated H2S pressure, respectively. In order to obtain accurate and reliable parameters, the initial values to be put into the program for regression are very important. In this work, the free H2S in the liquid phase is neglected (χ3=0) at first and Eqs. (14) and (15) are used to fit the experimental data to obtain K and A12. Then, RNH2·H2S is neglected in the liquid phase (χ2=0) and Eqs. (16) and (18) are used to fit the experimental data to obtain H and A13. Using the above parameters as initial values and considering all species in the liquid phase, the experimental data are refit. Thus, ten reliable and accurate parameters can be obtained simultaneously.

The conversion of experimental VLE data from H2S loading (α) to mole fraction (χi) in equilibrated liquid phase is illustrated in Appendix.

3.4 Correlated results

Figure 3 shows a plot for experimental data and correlated results. The total average absolute deviation (AAD) for the correlation is 6.48%. Another set of solubility data (30 data points), which were not used for correlation, were measured to verify the thermodynamic model and the interpolation result is good with the AAD of 6.88%. The AAD for each temperature is listed in Table 4. The evaluation result shows that the model is reliable.

Figure 3 Correlation results for the JMT-H2S system (points, experimental data; lines, correlated results)● 298 K; ▼ 313 K; 333 K; ■ 353 K; ▲ 368 K

Table 4 Average absolute deviations for correlation and interpolation of VLE data in H2S-JMT system

Figures 4 and 5 show χ1, χ2, χ3and χ4vs. α at 313 K and 368 K from the correlated data, respectively. At lower temperature (313 K), the concentration of free H2S is rather low, which means that the chemicalreaction is dominating. However, at higher temperature (368 K), χ3becomes larger, which means that the physical absorption becomes more important.

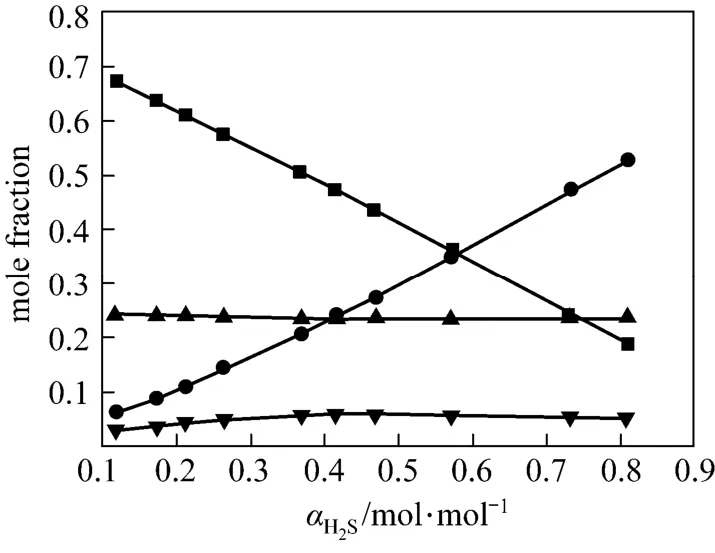
Figure 4 Liquid phase concentration vs. H2S loadingin JM-T at 313 K■ χ1; ● χ2; ▼ χ3; ▲ χ4

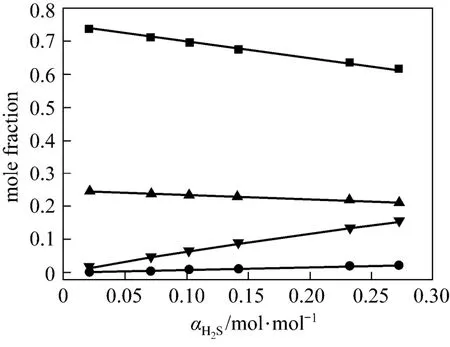
Figure 5 Liquid phase concentration vs. H2S loadingin JM-T at 368 K■ χ1; ● χ2; ▼ χ3; ▲ χ4

Comparing the data of this work with Li and Shen [14], it is clear that with almost the same concentration of amines, JM-T has lower H2S loading than MDEA at 313 K and has lower desorption temperatures at 313 K than MDEA (see Fig. 6).

3.5 Standard thermodynamic functions

Standard thermodynamic functions for Gibbs free energy ΔG, enthalpy ΔH, and entropy ΔS for the physical and chemical absorption are calculated according to the fundamental thermodynamic relationship as follows,

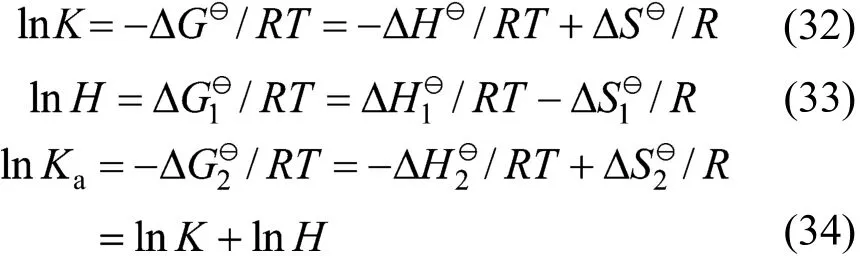
In this work, an ideal gas with2HS1P= kPa in the gas phase, pure RNH2and pure RNH2·H2S in the liquid phase are chosen as the standard states, and infinite dilution for H2S in pure RNH2liquid phase as reference state. The calculated parameters are listed in Table 5. The values of K [reaction (14)] and Ka[reaction (17)] decrease rapidly as temperature increases. It means that the formation of RNH2·H2S is unfavorable at high temperatures. Increase of A12and decrease of A13and A23with increasing temperature means that the interaction between RNH2and RNH2·H2S becomes weaker than that between like molecules at high temperatures, while the interaction between RNH2and H2S is stronger than that between like molecules at high temperatures. Thus the H2S molecules are more stable than RNH2·H2S molecules in organic phase at high temperatures, which can also be seen from Figs. 4 and 5.

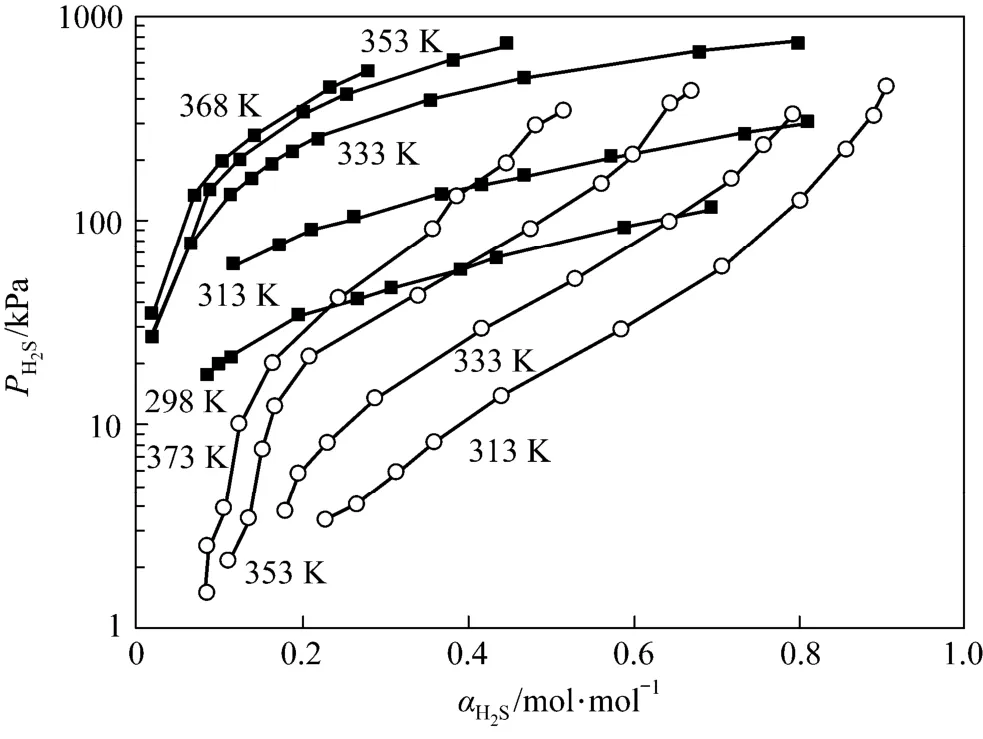
Figure 6 The solubility of H2S in 2.57 mol·L−1MDEA and Primene JM-T (2.37 mol·L−1)○ MDEA; ■ primene JM-T

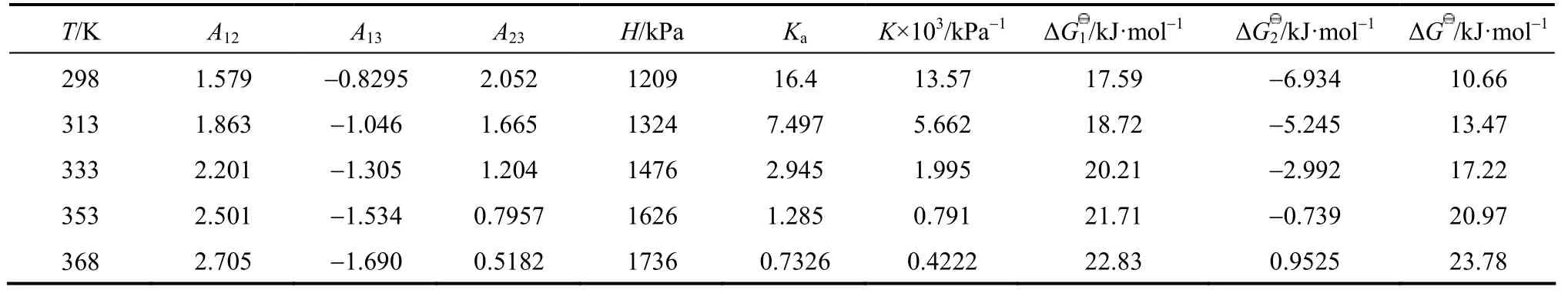
Table 5 Calculated parameters for JMT-H2S system

Table 6 Comparison of H, Ka, K, ΔH1, ΔH2and ΔH for MEA-H2S-H2O, MDEA-H2S-H2O and JMT-H2S systems at 313 K

The comparisons of H, Ka, K, ΔHI, ΔH2and ΔH for the MEA-H2S-H2O, MDEA-H2S-H2O and JM-T-H2S systems are listed in Table 6. The values for MEA-H2S and MDEA-H2S are calculated by using the temperature dependent H and K expressions [12, 24]. The Henry constant for H2S in water is larger than that in JM-T, which means that the equilibrated concentration of free H2S in JM-T is larger than that in H2O due to the ionization of H2S in water. The value of Kafor JMT-H2S is smaller than that for MDEA-H2S-H2O and MEA-H2S-H2O due to the large tertiary carbon group in JM-T. The value of ΔH1for H2S in water is larger than that in JM-T because the solvation of H2S in water is stronger. These are the main difference between aqueous and non-aqueous system. Furthermore, compared with MEA-H2S-H2O and MDEA-H2S-H2O systems, the value of ΔH for JMT-H2S system is lower.

3.6 Prediction of absorption enthalpy

Enthalpy changes happen in the process of absorption and desorption. Therefore, the calculation for absorption enthalpy is important to actual absorption process. The relationship between the absorption enthalpy and the equilibrium pressure can be expressed as follows:

Our model can be used to predict absorption enthalpy directly, as shown in Fig. 7. The absolute desorption enthalpy decreases quickly from 313 K to 333 K and 353 K, which are much less than the standard enthalpy change (∆H).

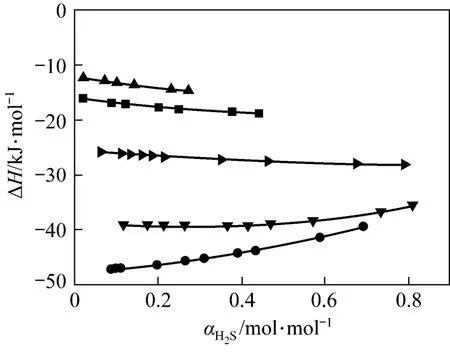
Figure 7 Changes of ΔH value with H2S loading for JMT-H2S systems at different temperatures● 298 K; ▼ 313 K; 333 K; ■ 353 K; ▲ 368 K

4 CONCLUSIONS

In this work, the solubility of H2S in Primene JM-T was measured at different temperatures and pressures. The chemical absorption reaction and a thermodynamic model were proposed. Satisfactory results were obtained for the correlation of the VLE data. The physical and chemical absorptions exist simultaneously in the non-aqueous system. Also, it is found that chemical absorption is dominating at low temperatures, and as the temperature increases, the physical absorption becomes more important. Furthermore, the difference between non-aqueous and aqueous absorption systems is pointed out. The calculations on thermodynamic equilibrium constants and standard thermodynamic functions for these physical and chemical absorptions show that the standard enthalpy for JM-T-H2S is lower than that MEA-H2S-H2O and MDEA-H2S-H2O systems. Compared with MDEA solution, the JM-T has lower absorption capacity for H2S, but has a lower desorption temperature.

NOMENCLATURE

D dielectric constant

G Gibbs free energy, kJ·mol−1

H Henry constant, kPa

H0, H1fitted values for Henry constant

ΔH standard enthalpy for physical and chemical absorption, kJ·mol−1

ΔH1standard enthalpy for physical absorption, kJ·mol−1

ΔH2standard enthalpy for chemical absorption, kJ·mol−1

K, Kachemical equilibrium constant expressed with activity for reactions (14) and (17)

Kχchemical equilibrium constant expressed without activity for reaction (17)

K0, K1fitted values for chemical equilibrium constant of reaction (14)

M molecular mass, g·mol−1

m amine value, mol NH2·g−1

n amount of substance, mol

P pressure, kPa

R gas constant, R=8.3145 J·mol−1·K−1

r ratio of RNH2to C18H36in mole fraction

S entropy, kJ·mol−1·K−1

T temperature, K

Tbboiling point, K

V volume, m3

v molar volume, m3·mol−1

w mass fraction

χ mole fraction in the liquid phase

α gas loading in liquid phase (H2S absorbed/amine), mol·mol−1

γ activity coefficient

η viscosity, mPa·s

ρ density, g·cm−3

NOMENCLATURE

ab absorbed

cal calculated

ct container

exp experimental

i, j species

in gas introduced into reactor

o original liquid sample before loading

1 RNH2

2 RNH2·H2S

3 free H2S (liquid)

4 C18H36

REFERENCES

1 Ahmed, M.S., Attia, Y.A., “Multi-metal oxide aerogel for capture of pollution gases from air”, Appl. Therm. Eng., 18, 787-797 (1998).

2 Lu, J.G., Zheng, Y.F., He, D.L., “Selective absorption of H2S from gas mixtures into aqueous solutions of blended amines of methyldiethanolamine and 2-tertiarybutylamino-2-ethoxyethanol in a packed column”, Sep. Purif. Technol., 52 (2), 209-217 (2006).

3 Lu, Y.X., Schaefer, L., “A solid oxide fuel cell system fed with hydrogen sulfide and natural gas ”, J. Power Sources, 135, 184-191 (2004).

4 Lee, J.I., Otto, F.D., Mather, A.E., “Measurement and prediction of solubility of mixtures of carbon dioxide and hydrogen sulfide in a 2.5 N monoethanolamine solution ”, Can. J. Chem. Eng., 54 (3), 214-219 (1976).

5 Lee, J., Otto, F.D., Mather, A.E., “Solubility of hydrogen sulfide in aqueous diethanolamine solutions at high pressures”, J. Chem. Eng. Data, 18 (1), 71-73 (1973).

6 Vallee, G., Mougin, P., Jullian, S., Furst, W., “Representation of CO2and H2S absorption by aqueous solutions of diethanolamine using an electrolyte equation of state”, Ind. Eng. Chem. Res., 38 (9), 3473-3480 (1999).

7 Xia, J.Z., Kamps, A.P.S., Maurer, G., “Solubility of H2S in (H2O plus piperazine) and in (H2O plus MDEA plus piperazine)”, Fluid Phase Equilibr., 207, 23-34 (2003).

8 Speyer, D., Maurer, G., “Solubility of hydrogen sulfide in aqueous solutions of piperazine in the low gas-loading region”, J. Chem. Eng. Data, 56 (4), 763-767 (2011).

9 Li, Y.G., Mather, A.E., “Correlation and prediction of the solubility of CO2and H2S in aqueous solutions of triethanolamine”, Ind. Eng. Chem. Res., 35 (12), 4804-4809 (1996).

10 Jou, F.Y., Carroll, J.J., Mather, A.E., Otto, F.D., “Solubility of mixtures of hydrogen sulfide and carbon dioxide in aqueous N-methyldiethanolamine solutions”, J. Chem. Eng. Data, 38 (1), 75-77 (1993).

11 Savage, D.W., Funk, E.W., Yu, W.C., Astarita, G., “Selective absorption of H2S and CO2into aqueous solutions of methyldiethanolamine”, Ind. Eng. Chem. Fundam., 25 (3), 326-330 (1986).

12 Li, Y.G., Mather, A.E., “Correlation and prediction of the solubility of CO2and H2S in aqueous solutions of methyldiethanolamine”, Ind. Eng. Chem. Res., 36 (7), 2760-2765 (1997).

13 Mandal, B., Bandyopadhyay, S.S., “Simultaneous absorption of CO2and H2S into aqueous blends of N-methyldiethanolamine and diethanolamine”, Environ. Sci. Technol., 40 (19), 6076-6084 (2006).

14 Li, M.H., Shen, K.P., “Solubility of hydrogen sulfide in aqueous mixtures of monoethanolamine with N-methyldiethanolamine”, J. Chem. Eng. Data, 38 (1), 105-108 (1993).

15 Sidi-Boumedine, R., Horstmann, S., Fischer, K., Provost, E., Furst, W., Gmehling, J., “Experimental determination of hydrogen sulfide solubility data in aqueous alkanolamine solutions”, Fluid Phase Equilibr., 218 (1), 149-155 (2004).

16 Duan, Z.H., Sun, R., Liu, R., Zhu, C., “Accurate thermodynamic model for the calculation of H2S solubility in pure water and brines”, Energ. Fuel, 21 (4), 2056-2065 (2007).

17 Xia, J.Z., Kamps, A.P.S., Rumpf, B., Maurer, G., “Solubility of hydrogen sulfide in aqueous solutions of the single salts sodium sulfate, ammonium sulfate, sodium chloride, and ammonium chloride at temperatures from 313 to 393 K and total pressures up to 10 MPa ”, Ind. Eng. Chem. Res., 39 (4), 1064-1073 (2000).

18 Aaron, D., Tsouris, C., “Separation of CO2from flue gas: A review”, Separ. Sci. Technol., 40, 321-348 (2005).

19 Goodrich, B.F., de la Fuente, J.C., Gurkan, B.E., Zadigian, D.J., Price, E.A., Huang, Y., Brennecke, J.F., “Experimental measurements of amine-functionalized anion-tethered ionic liquids with carbon dioxide ”, Ind. Eng. Chem. Res., 50 (1), 111-118 (2011).

20 Bates, E.D., Mayton, R.D., Ntai, I., Davis, J.H., “CO2capture by a task-specific ionic liquid”, J. Am. Chem. Soc., 124 (6), 926-927 (2002).

21 Rohm and Haas Co., Primene 81R and Primene JMT t-Alkyl Primary Amines, Philadelphia (1992).

22 Kent, R.L., Eisenberg, B., “Better data for amine treating”, Hydrocarb. Process., 55 (2), 87-90 (1976).

23 Austgen, D.M., Rochelle, G.T., Peng, X., Chen, C.C., “Model of vapor liquid equilibria for aqueous acid gas alkanolamine systems using the electrolyte NRTL equation ”, Ind. Eng. Chem. Res., 28 (7), 1060-1073 (1989).

24 Li, Y.G., Mather, A.E., “Correlation and prediction of the solubility of carbon dioxide in a mixed alkanolamine solution”, Ind. Eng. Chem. Res., 33 (8), 2006-2015 (1994).

25 Kuranov, G., Rumpf, B., Smirnova, N.A., Maurer, G., “Solubility of single gases carbon dioxide and hydrogen sulfide in aqueous solutions of N-methyldiethanolamine in the temperature range 313-413 K at pressures up to 5 MPa”, Ind. Eng. Chem. Res., 35 (6), 1959-1966 (1996).

26 Clegg, S.L., Pitzer, K.S., “Thermodynamics of multicomponent, miscible, ionic-solutions generalized equations for symmetrical electrolytes”, J. Phys. Chem., 96 (8), 3513-3520 (1992).

27 Li, C.X., Furst, W., “Representation of CO2and H2S solubility in aqueous MDEA solutions using an electrolyte equation of state”, Chem. Eng. Sci., 55 (15), 2975-2988 (2000).

28 Peng, D., Robinson, D.B., “A new two-constant equation of state”, Ind. Eng. Chem. Fundam., 15 (1), 59-64 (1976).

29 Maddox, R.N., Bhairi, A.H., Diers, J.R., Thomas, P.A., “Equilibrium solubility of carbon dioxide or hydrogen sulfide in aqueous solutions of monoethanolamine, diglycolamine, diethanolamine and methyldiethanolamine”, Research Report RR-104, Gas Processors Association (1987).

30 Jou, F.Y., Mather, A.E., Otto, F.D., “Solubility of H2S and CO2in aqueous methyldiethanolamine solutions”, Ind. Eng. Chem. Proc. Des. Dev., 21 (4), 539-544 (1982).

31 Strus, B., Sobczynska, A., Wisniewski, M., “Solubility of water and association phenomena in gasoline modified with hydrophilic additives and selected surfactants”, Fuel, 87 (6), 957-963 (2008).

32 Prausnitz, J.M., Lichtenthaler, R.N., de Azevedo, E.G., Molecular Thermodynamics of Fluid-Phase Equilibria, 3rd ed., Prentice-Hall PTR (1999).

33 Li, Y.G., Thermodynamics of Metal Solvent Extraction, TsinghuaUniv. Press, Beijing (1988). (in Chinese)

34 Pitzer, K.S., Activity Coefficient in Electrolyte Solutions, 2nd edition, CRC Press, Boca Raton, FL (1991).

APPENDIX

Material balance in regression process

In our regression, the equilibrium concentrations for each component in mole fraction are obtained from experimental H2S loading (α). After equilibrium, the following material balance should be kept,

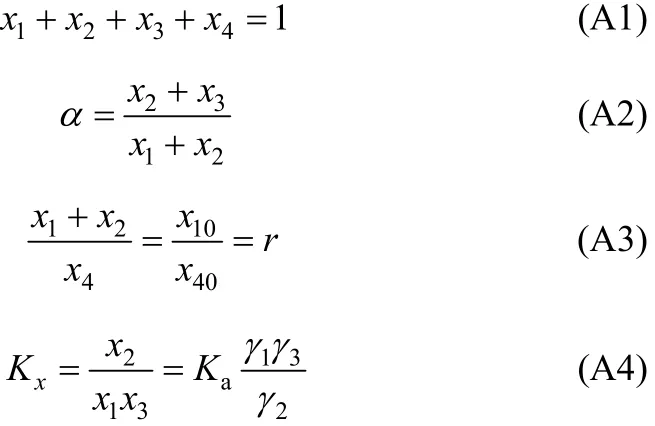
In Eq. (A2), χ10/χ40is the initial ratio of RNH2/C18H36and equals r, which is a constant before and after equilibrium. From the above equation, χ3(free H2S in liquid phase) can be expressed and solved as follows,

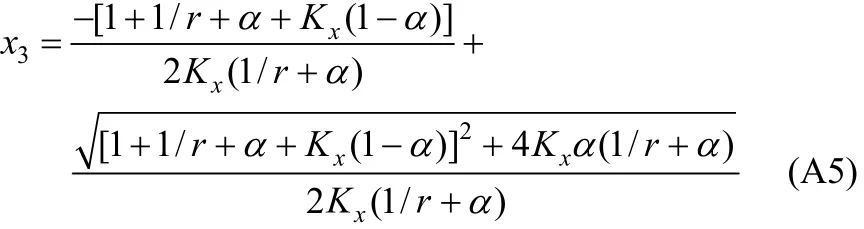
With the value of χ3, other χican be solved by the following equations.

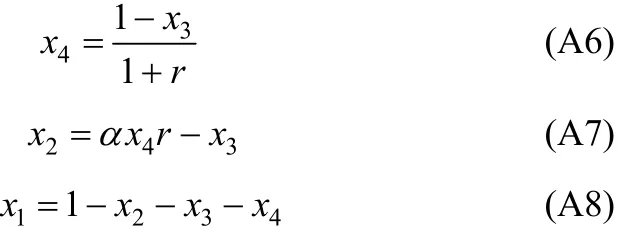
10.1016/S1004-9541(14)60016-1

2012-10-26, accepted 2013-01-15.

* Supported by the National Natural Science Foundation of China (51178446, 21206168).

** To whom correspondence should be addressed. E-mail: pgning@home.ipe.ac.cn