五阶时滞差分方程解的渐近性

沈 洁，冯玲玲，陶雁敏

（辽宁师范大学 数学学院，大连 辽宁 116029）

五阶时滞差分方程解的渐近性

沈 洁，冯玲玲，陶雁敏

（辽宁师范大学 数学学院，大连 辽宁 116029）

考虑五阶时滞差分方程 Δ5yn+f(n,yn,yn-r,yn-l,yn-p)=0,n∈N(n0)，得出了该方程存在具有特殊渐近性的有界非振动解的充分必要条件.

差分方程；时滞；有界解；渐近性

1 引言

下面假设对给定n0和式（2），时滞差分方程（1）有唯一解{yn}，方程（1）的解{yn}称为振动解是指序列{yn}不永远为正也不永远为负；否则，称其为非振动解.

2 主要结论及证明

定义1设S是Banach空间B的一个子集，S中每一个序列都有一个子列收敛到B中元素，则称S是相对紧的.

定义2设S是l∞的子集，∀ε＞0,∀{yn}∈S，存在整数N≥n0，当i，j＞N时，有| yi-yj|＜ε，则称S是一致柯西的.

引理1设Ω是乘积空间l∞×l∞的有界子集，若Ω是一致柯西的，则Ω是相对紧的.

引理2（Schauder不动点定理）设Ω是Banach空间X的一个非空闭凸子集，设T∶Ω→Ω是一连续映射，且TΩ是X中一个相对紧子集，则T中至少有一个不动点.

定理1假设存在常数c≠0，使

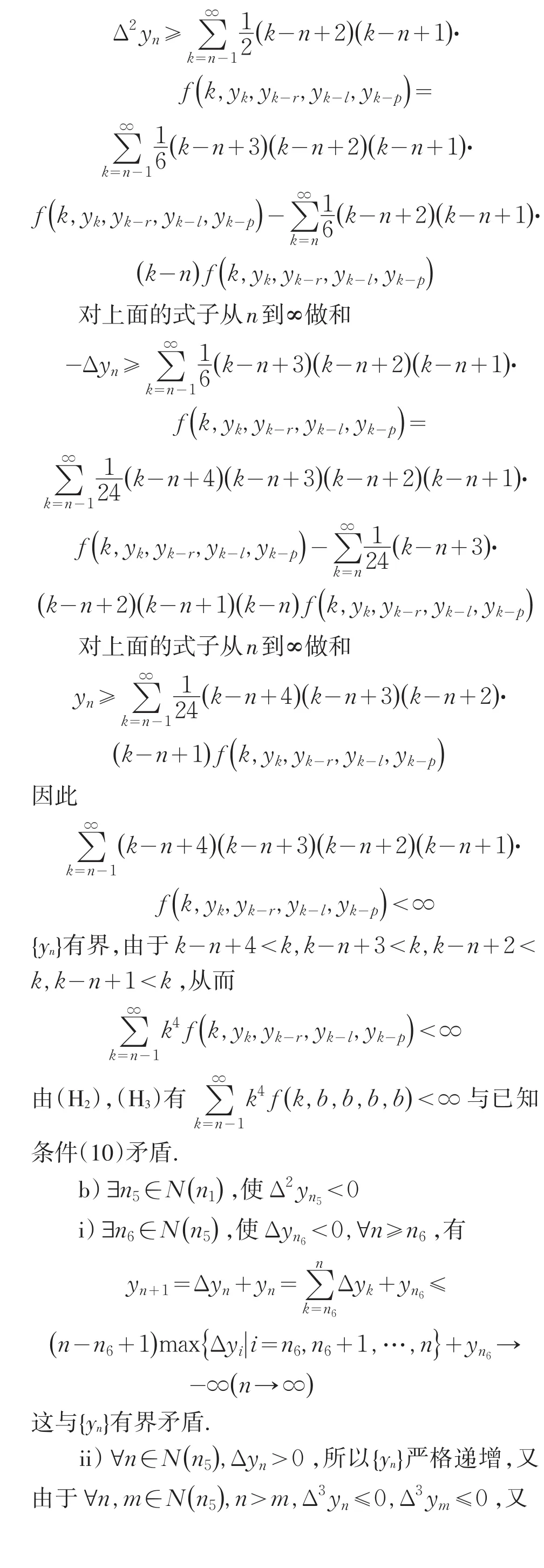
[1]Erbe L H,Kong Q,Zhang B G.Oscillation Theory for Functional Differential Equations[M].Dekker,1995.

[2]Gyori I,Ladas G.Oscillation Theory of Delay Differential Equation with Applications[M].Oxford Clarendon Press,1991.

[3]Agarwal R P.Difference Equations and Inequalities[M].New York,Marcel Dekker,1992.

[4]Philos Ch G.Oscillations in a class of difference equations[J].Appl Math Comp,1992(48):45-57.

[5]Philos Ch G.Oscillations in linear difference equations with variable coefficients[J].J Appl Math Stoch Anal,1991(4):241-258.

[6]Cheng S S,Patula W T.An existence theorem for a non⁃linear difference equation[J].Nonlinear Anal,1992(20):193-203.

[7]Yan J,Liu B.Asymptotic Behavior of a Nonlinear Delay Difference Equation[M].1995.

Asymptotic Behavior of Solutions for 5-th Order Delay Difference Equations

SHEN Jie，FENG Lingling，TAO Yanmin
（School of Mathematics，Liaoning Normal University，Dalian116029，China）

This paper studied a class of 5-th order delay difference equations Δ5yn+f(n,yn,yn-r,yn-l,yn-p)=0,

n∈N(n0)to give the necessary and sufficient condition for the existence of a bounded nonoscillatory solution of this equation.

difference equations；delay；bounded solution；asymptotic behavior

O 175.7

A

1674-4942（2011）03-0242-05

2011-05-07

2010年辽宁省教育厅科研项目（L2010235）；国家自然科学基金资助项目（11171138）

毕和平