Decompositions of the Kadomtsev–Petviashvili equation and their symmetry reductions
Zitong Chen(陈孜童), Man Jia(贾曼), Xiazhi Hao(郝夏芝), and Senyue Lou(楼森岳)
1School of Mathematics and Statistics,Ningbo University,Ningbo 315211,China
2School of Physical Science and Technology,Ningbo University,Ningbo 315211,China
3College of Science,Zhejiang University of Technology,Hangzhou 310014,China
Keywords: Kadomtsev-Petviashvili (KP) equation, decomposition, B¨acklund transformation, symmetry reduction
1.Introduction
Nonlinear systems,fundamental to mathematical physics,often display intricate behaviors due to the interconnectedness of their components.One such behavior is the violation of the linear superposition principle, a foundational concept in linear systems.However, research has demonstrated that many specific nonlinear systems adhere to the superposition principle under certain conditions.[1-10]This balance in nonlinear effects is attributed to the interactions between multiple nonlinear terms present in the system.[11-14]
In the context of a linear system, the presence of linear superposition implies a corresponding decomposition for the system.Furthermore,the nonlinearization[15-17]of eigenvalue problems of an integrable system inherently provides a decomposition for the original system.This observation suggests that,due to the presence of nonlinear effects,it is reasonable to consider decompositions directly for an integrable system,potentially revealing certain superpositions.A recent study[18]adopts this approach, applying it to the B-type Kadomtsev-Petviashvili equation hierarchy, where various linear superpositions can be derived from appropriate decompositions for that nonlinear system.
This paper investigates a fascinating phenomenon where certain types of nonlinear systems, specifically the potential Kadomtsev-Petviashvili (KP) equation (ux=v →KP equations forv)
whereγis a nonzero constant can be decomposed into either a Burgers-Sharma-Tasso-Olver(BSTO)system or a potential KdV system.Further arguments also reveal the relation between those decompositions and the B¨acklund transformation of the KP equation.
The remainder of this paper is divided into two sections.The first section outlines the process of constructing various decompositions of the potential KP equation and presents a B¨acklund transformation theorem by some special decompositions;such a transformation leads to the desired superposition.The second section revisits these specific decompositions and examines the corresponding symmetry reductions.
2.Solution theorems
The recent seminal work[18]introduces a so-called formally variable separation approach(FVSA).This method generally offers a means to decompose a(2+1)-dimensional nonlinear system,even in the absence of lax pairs.[19,20]
Specifically, for any (2+1)-dimensional nonlinear system with its solutionw(x,y,t), it is reasonable to conjecture formally thatwpossesses some decompositions as follows:
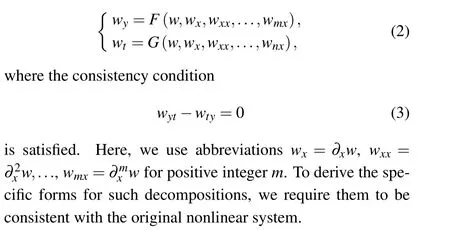
To construct the decomposition of the potential KP equation, we apply the FVSA to Eq.(1) as described above.The following theorems summarize the results.
Theorem 1 Letvbe either a solution of the BSTO equation system
whereC1is an arbitrary constant,or a solution of the potential KdV system
whereC1,C2,andC3are arbitrary constants.Thenu=vis a solution of the potential KP equation(1).
Proof First we directly substitute the formal decomposition (2) into the potential KP equation (1) and the additional consistent condition (3).After some calculations, it is found thatmandnare related withn=2m-1 andmhas to be fixed asm=2.Thus,the formal decompositions of the potential KP equation now become

whereW ≡W(ξ0,ξ1,ξ2,ξ3)is a complicatedξ4-independent function.Hereξm ≡umxfor convenience.Obviously,the coefficient ofξ4must be vanished,and the only possible form ofGreads
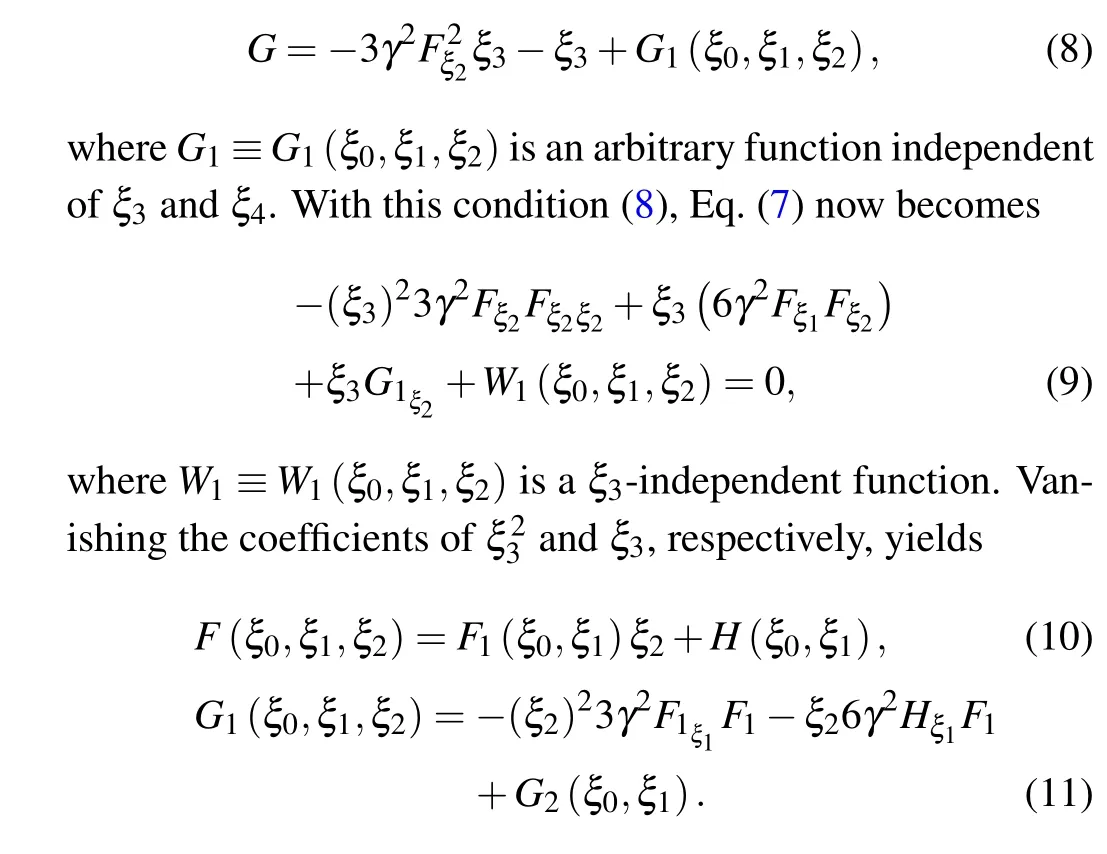
Here the functionsF1≡F1(ξ0,ξ1),H ≡H(ξ0,ξ1),andG2≡G2(ξ0,ξ1)are all dependent at most onξ0andξ1.
Furthermore, by substituting Eq.(6) and the relations mentioned in preceding paragraph into the consistent condition Eq.(3),we obtain that
Observe thatF1is independent ofξ2, it follows by the elimination of the coefficients ofξ4ξ2andξ4ξ1for Eq.(12)that
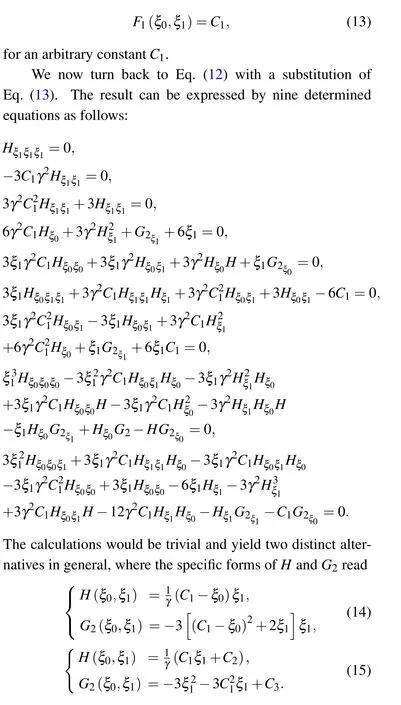
BSTO system(4)is produced by Eq.(14),and potential KdV system (5) is produced by Eq.(15), with all necessary equations we obtained before,respectively,as desired.
The former part Eq.(4) of this theorem aligns with the result obtained by Liu for the KP equation.[21]In fact, these two equations correspond to the well-known Burgers equation and the Sharma-Tasso-Olver (STO) equation,[22-25]respectively.The latter part Eq.(5)of this theorem is associated with a(2+1)-dimensional potential KdV system.[26,27]
For certain valid reasons, we may recast the FSVA method in a more general form that also considers some undetermined B¨acklund transformations for the potential KP equation (1).Letuandvboth be solutions of the potential KP equation (1) and suppose thatuandvsatisfy the following two decompositions:

whereC2is an arbitrary constant, or the variable coefficient BSTO system
whereC2is an arbitrary constant, thenuis a solution of the potential KP equation(1).
ProofFor proving this theorem,we may just assume thatvmeets Eq.(4) in Theorem 2.Againumxandvnxare simply abbreviated byξmandηnfor positive integersmandn.First we substitute Eqs.(16) and (17) to potential KP equation (1)and consistent condition (18), together with our assumption.By the elimination of the coefficientsξ4andη4,it leads to six alternatives.But if we get rid of the cases which eventually indicate again Eq.(4) or Eq.(5), only two different options remain.Namely,
Substituting the above two equations into potential KP equation(1)and consistent condition(18),respectively.After some cumbersome(but similar to what we did in the proof of Theorem 1),the results ensure straightforwardly that Eqs.(19)and(20)are the desired decompositions.
We can verify the integrability for these two variable BSTO systems in the sense of the existence of lax pairs.As being related to the Cole-Hopf transformation[28,29]u=2(lnψ)x,one can easily calculate to obtain the weak lax triple of Eq.(19)as follows:
whereλis the spectral parameter.The weakness of lax triple here means that the variable coefficient BSTO system(19)can be produced by the consistency condition The variable coefficient BSTO system (20) can be obtained by the compatibility condition(25)together with Eq.(27)directly.

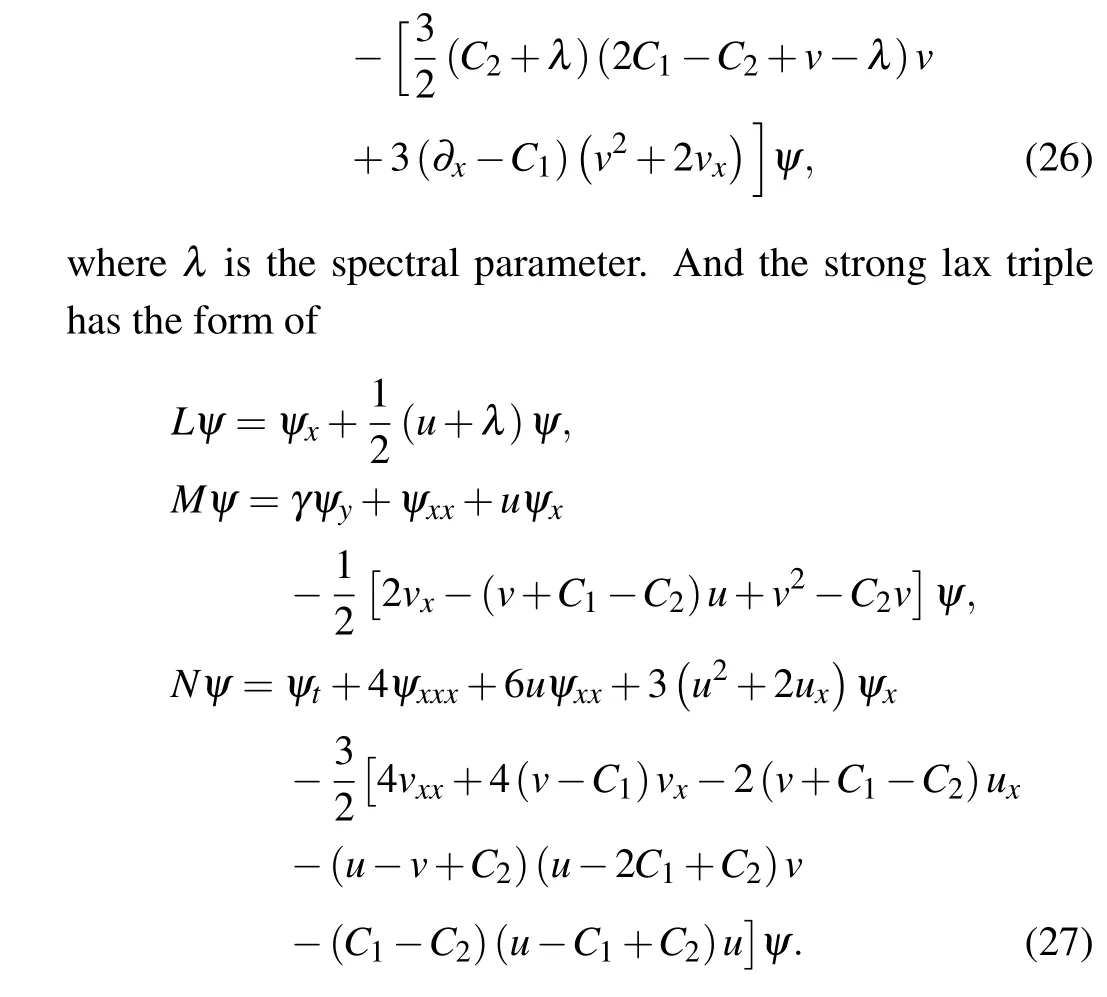
Theorem 3Letvbe a solution of the potential KdV system(5)in Theorem 1.Ifumeets the variable coefficient BSTO system

whereλis the spectral parameter.And if an additional restrictionC3=-3C1C2is accepted,we then have a strong lax triple for Eq.(28)as follows:
Without loss of generality,one can validate the aforementioned three theorems directly by substituting them into the potential KP equation, respectively.These theorems all yield B¨acklund transformations for the potential KP equation (1).As a corollary of the above theorems,we can generally formulate the following theorem.
Theorem 4(B¨acklund transformation theorem)Letuandvbe functions satisfying the coupled equations system
in whichCis an arbitrary constant.Thenuis a solution of the potential KP equation(1)iffvis a solution of the potential KP equation(1).
ProofThe idea is to consider the combination of Eqs.(4)and (19).Suppose that bothuandvmeet Eq.(31).By substituting Eq.(31) into potential KP equation (1) directly, one can check that ifuis a solution of Eq.(1), then so isv.The converse is immediate by symmetry.
This theorem is the same as the result obtained by Chen,[31]Levi,[32,33]et al.It indeed indicates a simple superposition principle for the potential KP equation.Precisely speaking, consider four distinct solutionsu0,u1,u2,u12, andu21for Eq.(31)with
According to the Bianchi’s permutability theorem,[34]we have here thatu12=u21≡u3.By some suitable linear combinations for these four equations,it follows that

With the superposition principle (37), we can now construct a series of novel solutions for the potential KP equation in a remarkably straightforward manner.More precisely, if we have three solutionsu0,u1, andu2for Eq.(1) such thatu0andu1are solutions for the coupled equation system (31)with parameterC=C1, and thatu0andu2are solutions for the coupled equation system(31)with parameterC=C2,then Eq.(37)provides a new solution for Eq.(1)distinct from the three initial solutions.
Next,we will apply this superposition principle to derive some novel solutions for the potential KP equation.Observe that with the trivial solutionv=0,Eq.(31)reduces to Eq.(4).Therefore, it is convenient to consider, for example, any two solutionsu1andu2for Eq.(4)withu0=0.From this, in order to find some solutions for the potential KP equation(1),it suffices to apply Eq.(37)to solutions of Eq.(4).

For instance, the standard truncated Painlev´e method[22,23]reveals that Eq.(4) has the following multiple soliton solution:with the wave numberski,ri,the frequenciesωi,and the original positionsξi.We can also confirm that the dispersion relations here satisfy that
To utilize Eq.(37),we initially setu0=0.Letu1be a singlesoliton solution (takingN=1 in Eq.(44)) with wave numberk1and letu2be a two-soliton solution (takingN=2 in Eq.(44))with wave numbersk2andk3,respectively.Then we must have that bothC1andC2in Eq.(37)are zero because of the fact that these two solutions should satisfy Eq.(4) sinceu0=0.In this situation, it follows that the superpositionu3obtained from Eq.(37)remains a solution for the potential KP equation (we here take the arbitrary functionFto be zero).Figure 1 shows a specific instance with the parameters setting:k1=-0.5,k2=0.2,k3=0.4,ξ1=0,ξ2=5,ξ3=10, andγ=1,at different times.

Fig.1.These three pictures are all obtained by the parameters setting k1 =-0.5, k2 =0.2, k3 =0.4, ξ1 =0, ξ2 =5, ξ3 =10, and γ =1, but occur at distinct time.
3.Symmetry reduction
In the preceding section, we obtain three specific novel decompositions for the potential KP equation (1) (i.e., those three variable coefficients systems in Theorems 2 and 3).They are all integrable systems because of the existence of lax triples.In this section,we study the symmetries of these three systems,and give the corresponding symmetry constraints,respectively.
Recall that a symmetryσof a partial differential equation system

whereξ,η,τ,randsare functions to be determined with respect to{x,y,t,u,v}.We next substitute them into the original symmetry Eq.(46).With some complex but straightforward calculations,one can obtain that

whereFandGare undetermined functions with respect to the group invariantsaandbas shown in Eq.(49).Note that it doesn’t matter what the specific value we select forc1,the invariantsaandbwill eventually be of such forms.The decided procedure for the specific forms ofFandGcan be completed by substituting Eq.(50) into the original system Eqs.(4) and(19).The result shows thatFandGmust satisfy the following equations:

Case (iii) demonstrates the translation group onx, and trivially indicates an uninteresting case that bothuandvare constants.
The remaining case(i.e.,case(iv))is related to the translation invariance withy.The result shows thatuandvare constrained to (1+1)-dimensional situation.In such a case,we have that

And after some complicated computation,the result shows that
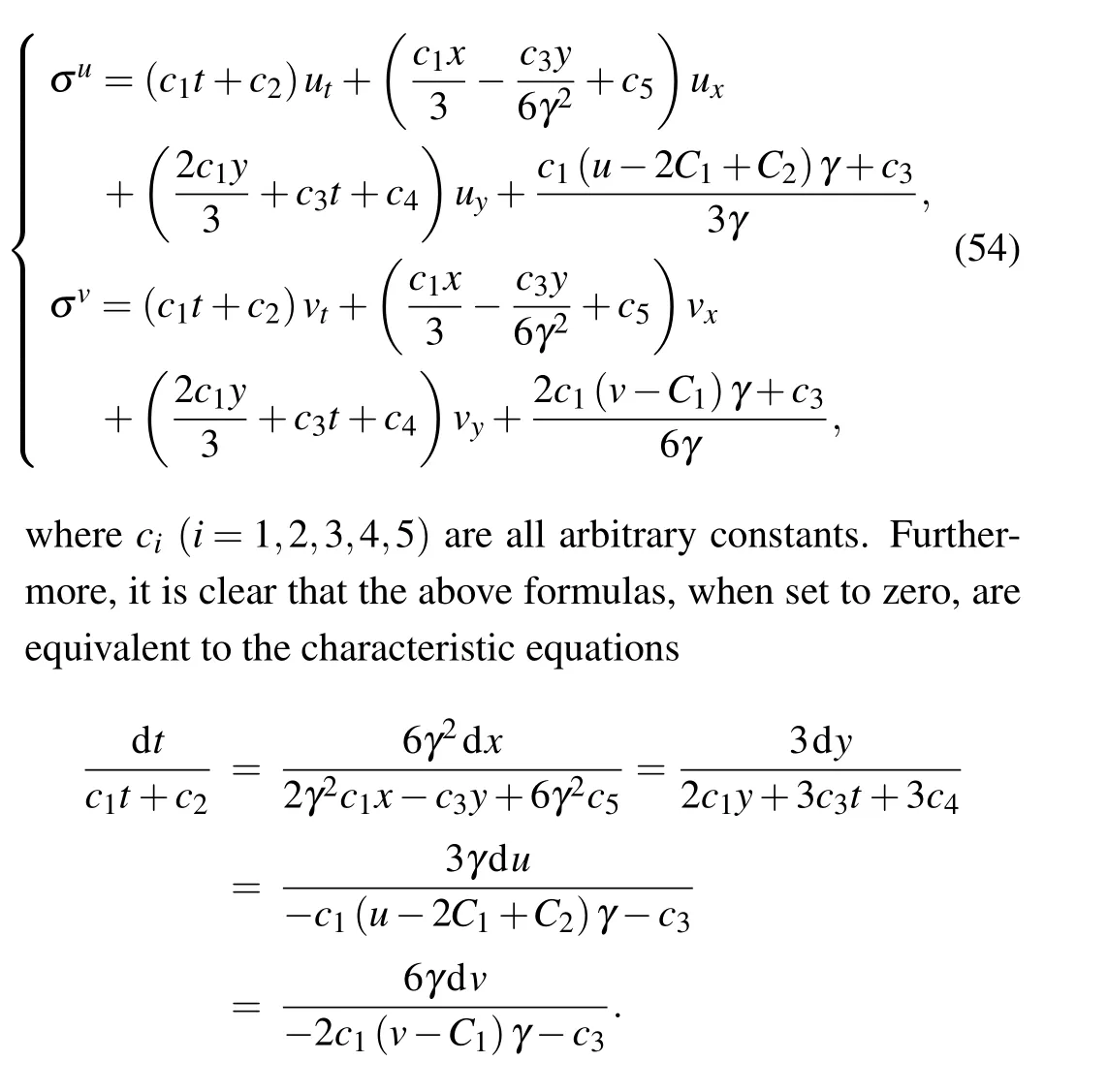
The corresponding symmetry reductions need to be discussed in four different alternatives listed as follows:

Forvin Eq.(55)should be certainly the solution of the BSTO system(4)anduin Eq.(55)should be the solution of the variable coefficient BSTO system(20).
The above discussion is based on the scaling transformation as well as we see in the case of Eq.(4), and the consequence seems to be similar except for the specific forms ofFandG.It is reasonable since the different parts between these two theorems make certainly no formal effects on the scaling symmetry.
For the remaining cases,we eliminate the scaling symmetry.Case (ii) leads to the group invariant solutions related to the rotation symmetry.For a special instance,letc2=c3=1,then the group invariant solutions possess the following forms:

As for case (iii), we again obtain anx-translationinvariant solution as trivial as the similar situation we discussed in Eq.(19).The last case (case (iv)) is related to the translation invariance ony-axis of the PDE systems (4) and(20).It follows that
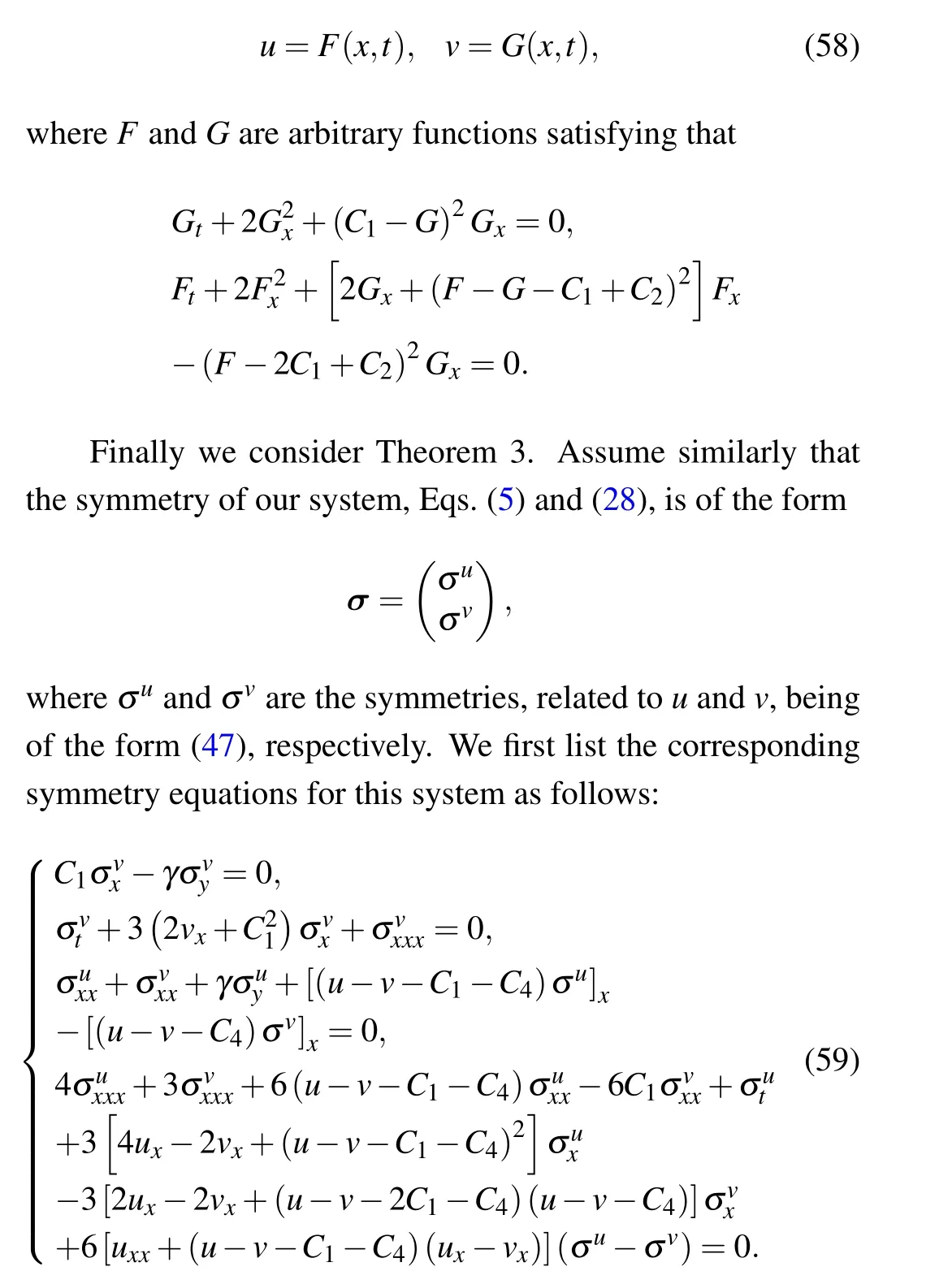
Again, by substituting Eq.(47) into the above equations, we can obtain that
whereci(i=1,2,3,4,5,6)are all arbitrary constants.To give the corresponding symmetry reduction,it is equivalent to solving the characteristic equations
It follows that four alternatives:

The remaining two alternatives lead to some translation invariant solutions.Forc4̸=0(case(iii)),a trivial constant solution would be obtained.Forc5̸=0(case(iv)),uandvare restricted to be functions with respect toxandt.And if we takec5=1,the result shows that
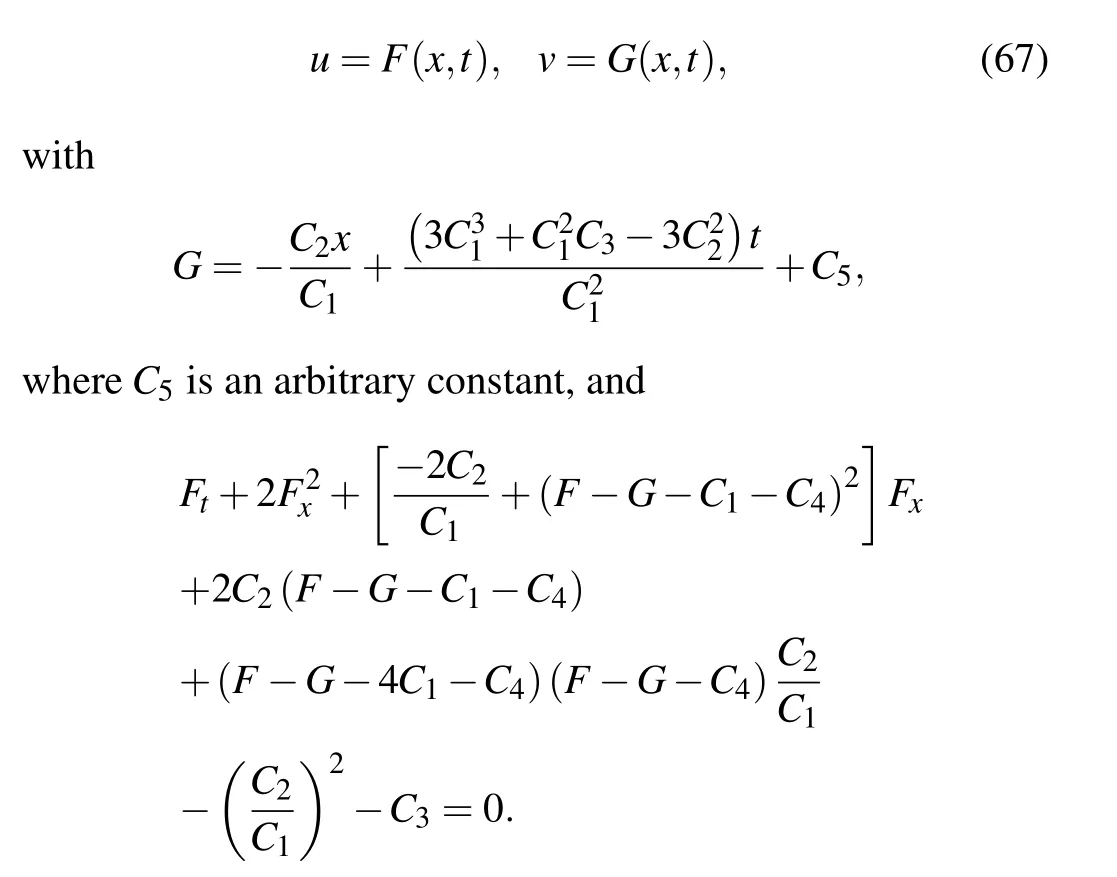
4.Summary and discussion
This study offers a fresh perspective on the decompositions of the potential KP equation.The findings elucidate the relationship between the potential KP equation,the BSTO system, and the BSTO-KdV system.Our research establishes a superposition principle for the solutions of the potential KP equation,subject to a restriction associated with the BSTO decomposition.With these decompositions,one can easily apply the superposition principle to construct,for instance,a(1+2)-soliton solution for the potential KP equation.Not only is such a superposition feasible,but we can also consider anm-soliton solution and ann-soliton solution, the sum of which remains valid.Generally, it indicates that any solutions for the BSTO system (4) can be amalgamated to derive a solution for the potential KP equation.
The symmetry of the BSTO decompositions and the BSTO-KdV decomposition is also examined.The corresponding symmetry constraints are presented in Section 3.For most nontrivial cases,the scaling symmetry plays a significant role.These decompositions are all linked to some novel integrable systems.Future research could further investigate the potential applications of this solution in various domains of mathematical physics.
Acknowledgments
Project supported by the National Natural Science Foundation of China (Grant Nos. 92065113, 11904357, 62075208,and 12174367), the Innovation Programme for Quantum Science and Technology (Grant No. 2021ZD0301604), and the National Key Research and Development Program of China (Grant No. 2021YFE0113100). This work was partially carried out at the USTC Center for Micro and Nanoscale Research and Fabrication. Meng-Jun Hu is supported by Beijing Academy of Quantum Information Sciences.
- Chinese Physics B的其它文章
- A multilayer network diffusion-based model for reviewer recommendation
- Speed limit effect during lane change in a two-lane lattice model under V2X environment
- Dynamics of information diffusion and disease transmission in time-varying multiplex networks with asymmetric activity levels
- Modeling the performance of perovskite solar cells with inserting porous insulating alumina nanoplates
- Logical stochastic resonance in a cross-bifurcation non-smooth system
- Experimental investigation of omnidirectional multiphysics bilayer invisibility cloak with anisotropic geometry