Natural Convection and Irreversibility of Nanofluid Due to Inclined Magnetohydrodynamics(MHD)Filled in a Cavity with Y-Shape Heated Fin:FEM Computational Configuration

Afraz Hussain Majeed,Rashid Mahmood,Sayed M.Eldin,Imran Saddique,S.Saleem and Muhammad Jawad

1School of Energy and Power Engineering,Jiangsu University,Zhenjiang,212013,China

2Department of Mathematics,Air University,Islamabad,44000,Pakistan

3Center of Research,Faculty of Engineering,Future University in Egypt,New Cairo,11835,Egypt

4Department of Mathematics,University of Management and Technology,Lahore,54770,Pakistan

5Department of Mathematics,University of Sargodha,Sargodha,40100,Pakistan

6Department of Mathematics,College of Science,King Khalid University,Abha,61413,Saudi Arabia

7Faculty of Sciences,The Superior University,Lahore,54000,Pakistan

ABSTRACT This study explains the entropy process of natural convective heating in the nanofluid-saturated cavity in a heated fin and magnetic field.The temperature is constant on the Y-shaped fin,insulating the top wall while the remaining walls remain cold.All walls are subject to impermeability and non-slip conditions.The mathematical modeling of the problem is demonstrated by the continuity,momentum,and energy equations incorporating the inclined magnetic field.For elucidating the flow characteristics Finite Element Method(FEM)is implemented using stable FE pair.A hybrid fine mesh is used for discretizing the domain.Velocity and thermal plots concerning parameters are drawn.In addition, a detailed discussion regarding generation energy by monitoring changes in magnetic,viscous,total,and thermal irreversibility is provided.In addition,line graphs are created for the u and v components of the velocity profile to predict the flow behavior.Current simulations assume the dimensionless representative of magnetic field Hartmann number Ha between 0 and 100 and a magnetic field inclination between 0 and 90 degrees.A constant 4%volume proportion of nanoparticles is employed throughout all scenarios.

KEYWORDS Finite element method;nanomaterials;entropy;MHD;square cavity;Y-fin

1 Introduction

Entropy is a fundamental property of any dynamic thermal system, serving as a quantitative representation of the disorder introduced by the irreversibility process at work during heat exchange.The second rule of thermodynamics asserts that total entropy uniformly predominates in reversible processes and rises with time in non-reversible techniques, leading to entropy measurement in thermalized systems.Bejan [1] was one of the pioneers in entropy development by inventing an approach to thermodynamic optimization.The purpose of optimizing the structure of entropy thermal hydraulic systems is to enhance their performance in several fields.Moreover, entropy analysis can improve the performance of heating equipment such as heat engines, heat pumps, coolers, cooling electronic chips,and micro-thermal channels.Parvin et al.[2]investigated the entropy irreversibility of metal nanoparticles in a water-saturated chamber.Rayleigh and Bejan numbers correlate,affecting their impact on entropy variation.Kefayati[3]employed the Lattice Boltzmann method to explore the effects of natural convection and an external magnetic source on a ferrofluid in a cavity.Nanoscale ferromagnetic cobalt particles were suspended in kerosene.There is a negative correlation between the percentage of nanoscale ferromagnetic particles in the volume and the heat transmission for a range ofRa.Rabbi et al.[4]utilized pure mixed convection magnetized investigation directed toward the cavity to investigate the impacts of two different geometric heater configurations.A single-phase model of ferrofluid was employed as a working fluid.Heaters on the bottom wall were always kept on,while those on the vertical side walls were preserved at a steady,low temperature.The top wall permanently moved at the same rate and temperature in geometric layouts.The Galerkin weighted residuals approach of finite element analysis was employed to solve the flow equations.Merji et al.[5] and Mahmoudi et al.[6]investigated the process of entropy and how it is carried out in a square cage filled with water and features the fusing of hybrid nanoparticles.They described the volume of nanoparticle fraction on the accumulative entropy procedure.Including alumina nanoparticles,Armaghani et al.[7]evaluated the entropy generation characteristics in the baffle of an L-shaped water-saturated duct,which are flow-differentiated by buoyant thermal convection.Zamily[8]assessed the various thermal features of water along with TiO2particles,and the randomness of entropy by determining the thermal sources at cavity boundaries.Bouchouch et al.[9]investigated nanofluid irreversibility(H2O/Al2O3)by introducing wavy input in a symmetrical enclosure.They indicated that entropy is enhanced and more massively within the system when considering the free convection environment.It has been shown that scattering solid particles increases entropy and energy transmission.Oztop et al.[10] studied natural convection inside rectangular enclosures that were only partially heated and carried nanofluids.Sheremet et al.[11]deliberately formed thermal flow by transfer of variable wall temperature due to irreversibility changes in a cavity.They revealed that significant change appears in entropy,evaluating the dominance of natural convection by improving the Rayleigh number(Ra).Siavashi et al.[12]capitalized the two-phase mixtures ofH2OandCuto quantify a free-flow entropic characteristic in a fine-furnished permeable cavity.Kashyap et al.[13]examined nanofluid two-phase(water/cu)flow along with the performance under distinct boundary conditions and the irreversibility in a porous cavity.Gibanov et al.[14] demonstrated an analysis of thermophysical characteristics arising from entropy in nanofluid generated by a lid-driven cavity.In the permeable square enclosure under magnetizing devices,Mansour et al.[15]investigated the characteristics of a hybrid nanofluid(water-Cu-Al2O3).They demonstrated that entropy rises asφincreases.Rahimi et al.[16]examined the thermal dimensions of the nanofluids inside the cavity with fins and approximate declines in entropy generation towards fractional volume nanoparticles.

Flow analysis is not only exciting but also thought-provoking.Further numerical and experimental research is needed across various topics, although several technology applications and scientific challenges exist.This study explores the effects of friction and heat transfer in viscous fluid flows across solid surfaces and in ducts and channels.These consequences must be factored into evaluating the fluid’s qualities.The issue can be solved in either an experimental or theoretical setting.Numerical approaches, such as finite differences, finite elements, and finite volumes, are developed for solving incompressible Newtonian flow problems.The most well-known approach is the traditional PARDISO algorithm,which first presents the idea of a stalled grid in conjunction with the pressure adjustment equation.It indicates a dearth of information in the literature on non-Newtonian fluid flows,particularly polymer melts in the molding process,evaluated in[17–20].Khan et al.[21]examined a comprehensive study of hydromagnetic thermal flow effects over a cavity cylinder.They implemented a finite element scheme for solving the nonlinear coupled equations.Soomro et al.[22]considered a constructional design-based study to analyze the features of thermal flows in a semicircular cavity.They concluded that as Richardson’s number increases, heat transport slows down.In addition, as the Hartmann number grows, the heat transmission rate improves.Chelia et al.[23]investigated the characteristics of natural convection with nanofluid in a cavity utilizing the famous numerical approach,the Lattice Boltzmann Method(LBM).In addition,they indicated that the heat transmission rate from a bottom-heated cavity is greater than that of a sidewall-heated cavity.

This study explains an entropy-generating process,including an angled magnetic field induction and a natural convection component.It discusses various types of irreversibility against different effective parameters that have not yet been revealed.The research’s result can be a reference for future studies.

2 Mathematical Modeling

This study considered the unit dimensions of the cavity are saturated by water and copper nanoparticles were induced.Flow domain density variations include the addition of Boussinesq approximation to the buoyancy term,containing the role of natural convection in the flow domain.The constantB0magnetization magnitude measures the maximum change in nanoparticles’flow in an orthogonal direction.The cavity’s top wall is considered insulated,and the fin is heated uniformly while the other walls are colder.There are no slip conditions to all boundaries.Fig.1 shows the physical configuration.

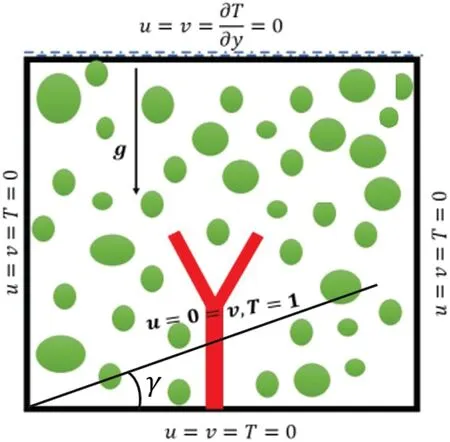
Figure 1:Physical structuring of problem

The continuity,flow velocity,and heat equation[24–26]for a copper fluid are described in a nondimensional mathematical form as follows:

The boundary conditions are given below:

•Th=1,at the fin.

•(u,v)=0,at all walls of the cavity and fin.

•T=0,other walls of the cavity.

The village girls met and danced in a ring, singing, Beautiful spring, how came you here? How came you here? Did you come on a plough, or was it a harrow? Only Snowflake sat quite still by the window of the cottage

2.1 Properties of Copper Fluid

These equations are appropriate for the thermo-physical properties of the nanofluid in this study[13–15].The physical characteristics of copper fluid are listed in Table 1.

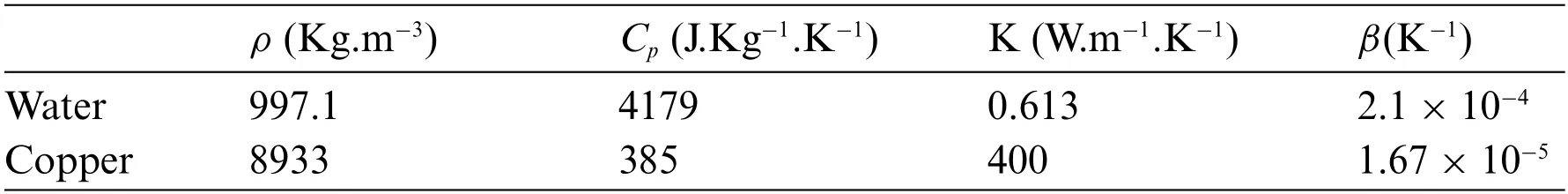
Table 1: The thermophysical characteristics of nanofluid

2.2 Entropy Generation

The term “irreversibility analysis” refers to quantifying the local entropy production resulting from the sum of the conjugated fluxes and the forces that are generated.A convective process subject to a magnetic field yields the following expression for the non-dimensional local entropy production:

On the right-hand side of Eq.(5) initially stands the energy entropy, then viscous entropy, and finally,magnetic entropy located at the top right side.The irreversibility ratio(ψ)of a distribution can be calculated using Eq.(6).

whereTois the average temperature of the heated and cold walls.

3 Numerical Procedure

The velocity field and temperature at every location in the analyzed physical model must be known to numerically calculate the production of local entropy using Eq.(5).A numerical approach called the finite element method (FEM) is applied to compute an estimation of the solution to the model problem because analytical solutions to the coupled equations are not possible due to the nonlinearity of the governing equations.This was conducted to better understand how the model problem will be solved.In order to solve the underlying discrete nonlinear system of equations,Newton’s method was employed to linearize the system, and then the direct solver PARDISO was applied to address the linearized inner systems.The PARDISO solver has numerous benefits.LU matrix factorization is employed to achieve the needed level of convergence with a minimum number of iterations.This reduces the total number of necessary iterations.Fig.2a depicts the computing grid.

Domain coarse-resolution meshing is conducted using a pressure-velocity finite element pair.Fig.2a indicates that coarser-grained rectangular and triangular elements represent the entire computational domain.A nonlinear system is iterated until the convergence requirement is satisfied,typically defined as a residual of less than 10-6.

Fig.2b shows vertical velocity profiles along the center of the cavity,as measured with different nanofluids.The results demonstrate good agreement with the Oztop study[10].

4 Results and Discussion

This section demonstrates how entropy quantities change in response to modifications in flowcontrolling parameters.Galerkin finite element method and hybridized meshing are applied to achieve highly accurate results.Elements with triangular and rectangular shapes are employed to represent domain discretization.Table 2 lists data about the number of elements and degrees of freedom at nine levels.It shows the results of a grid independence analysis forNuavg.

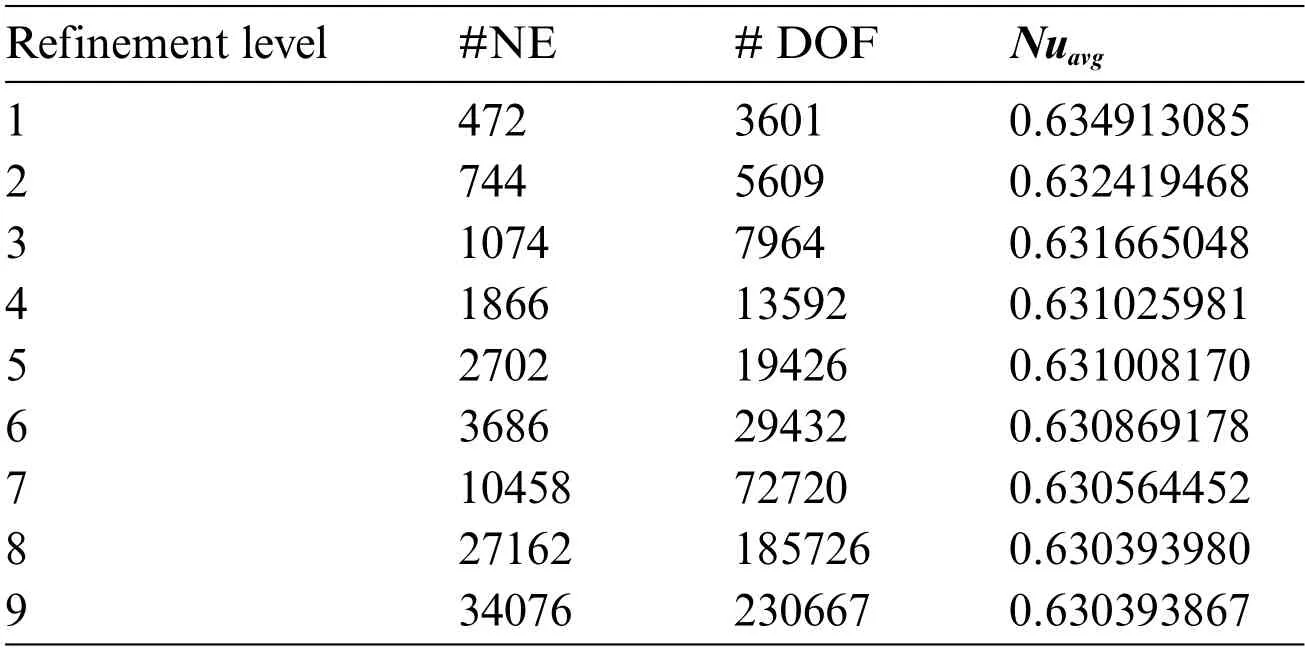
Table 2: Variation in degrees of freedom and grid convergence test

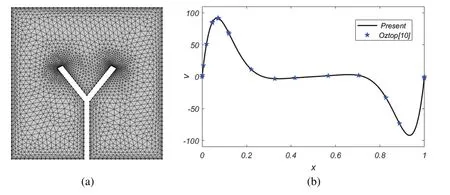
Figure 2:(a):Discretization of the computational domain(b):Comparison of the present study with Oztop[10]

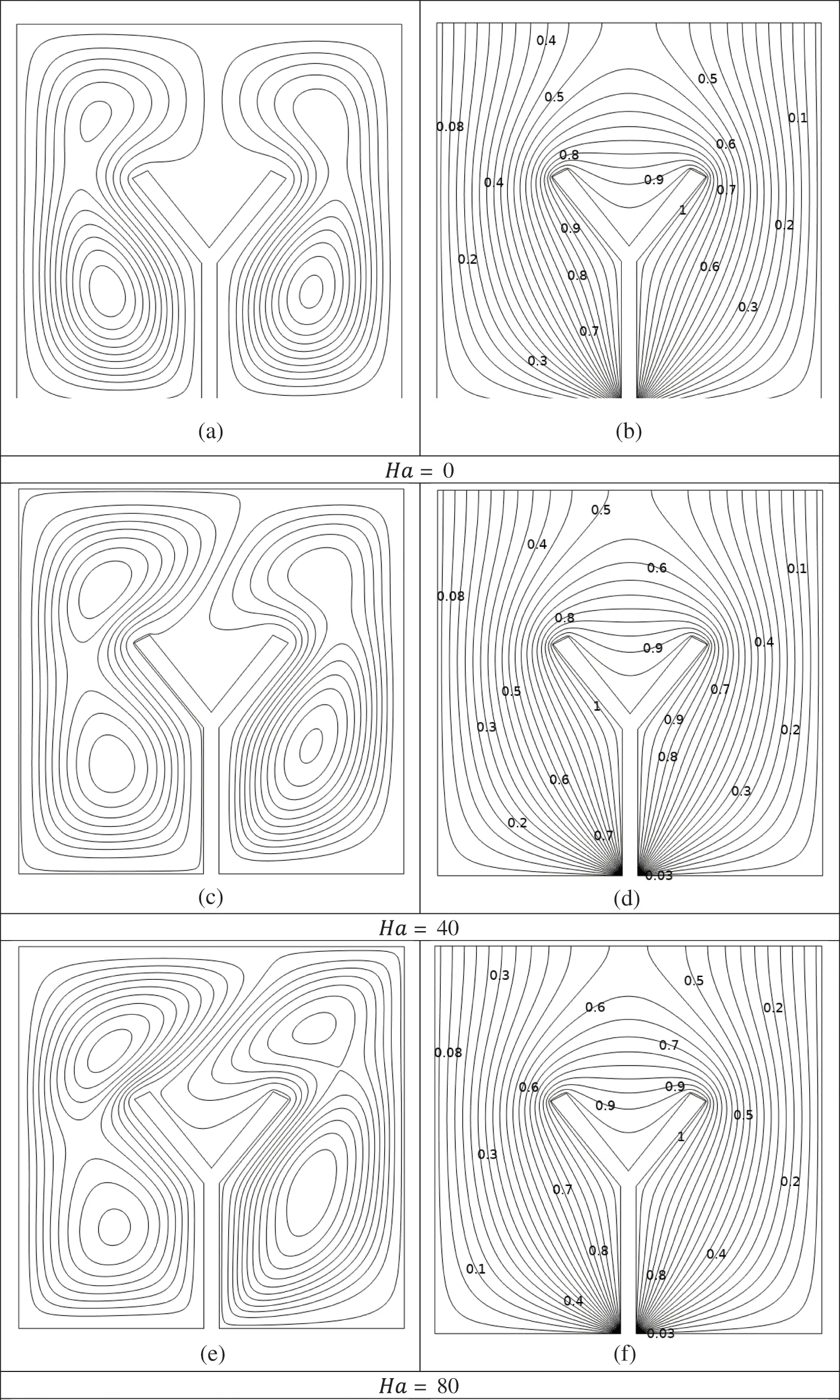
Figure 3: (Continued)

In Figs.4a–4f, theγvaries between 30◦,60◦,and 90◦on velocity and isotherm contours, respectively.The parametersφ= 0.02,Ha= 20,Ra= 103, andPr= 6.2 are used in this study.Asγincreases,so does the intensity of fluid circulation represented by streamlines,and because theγ=90◦fluid flow is severely impeded.The mid-planex= 0.5 symmetry is achieved atγ= 60◦and 90◦, as well as with a growth in the shapes of cells deformed atγ= 60◦and 90◦.Anticlockwise movement is seen in left-hand cells,and clockwise movement is shown in right-hand cells

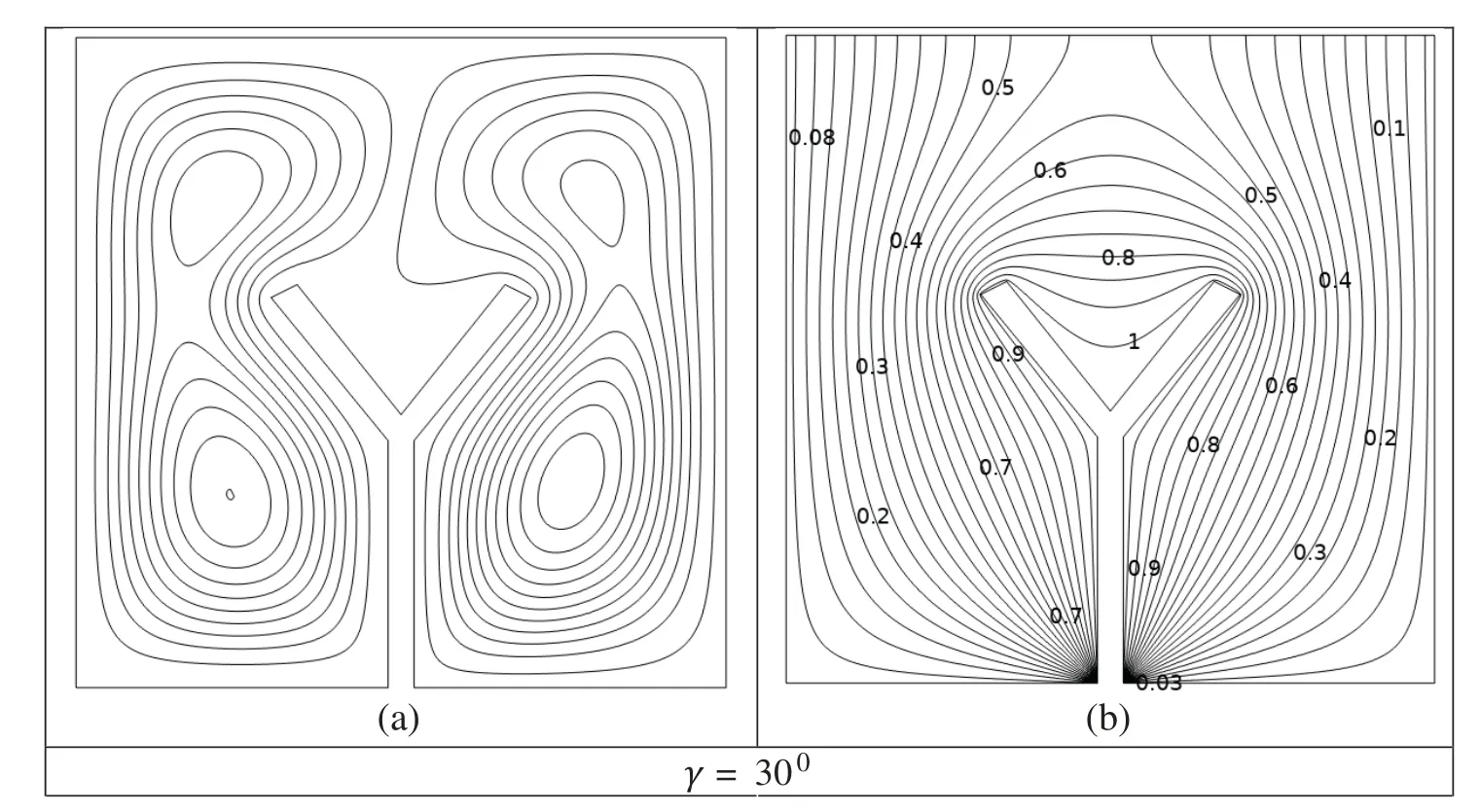
Figure 4: (Continued)

It was reported that nanoparticle volume fraction significantly impactsSTherm,SVisc,andSMagalong with a variety ofHain Figs.5a–5d.Other parameters such asRa= 1000,γ= 300, andPr= 6.2 are fixed to achieve optimal change in this case.Fig.5a demonstrates that theSThermhas a uniform forHa= 0 and increases as Ha rises andφdecreases.By comparing the deprecating behavior of the temperature gradient to an increase inφ,this can be explained.Fig.5b illustrates the behavior of entropy generation due to viscous forces against increasing values ofφandHa.As the value ofHaandφincrease,theSVisccurves are in decrement.As(Ha)increases,viscous forces become dominant,and the reduction movement of fluid particles enriches as a result of viscous forces decaying,this occurs.(SVisc)approaches zero whenHa>0.Fig.5c indicates that it can predict how the entropy generation process will behave when the flow field magnetic strength changes.It is shown thatSmagn= 0 whenHa=0.A maximum ofHa=40 is reached,then it reduces to the maximum amplitude of the curve.By controlling theφ, it can influence the magnitude of randomness generated by a magnetic field.The impact ofφandHadiminishes.Total entropySTotalgeneration coefficient depends onφandHa,as shown in Fig.5d.There is evidence that the total entropy coefficient decreases with an increase in(Ha)in the range of(0.02 ≤φ≤0.08)and(0 ≤Ha≤100).

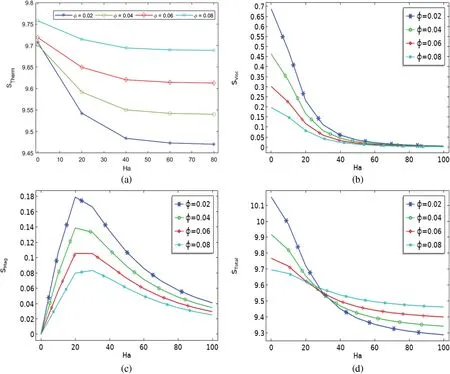
Figure 5:(a–d)Impacts of φ on irreversibilities against Ha

For differentφand Ha,the variations of the components(uandv)of the velocity vector atx=0.5 are shown in Fig.6.When the volume fraction and Hartmann number increase,the u-component of the speed vector decreases,while velocity increases when these parameters decrease in the(0.25 ≤x≤0.45)low regime.When volume fractions and Hartmann numbers reduce,the v-component of velocity increases.

Figs.7a and 7b depict the effect of the magnetic fieldsγand theHaon the thermal,viscous,and magnetic irreversibilities.By fixing theRaand thePrto 103and 6.2,respectively,the response of the irreversibility coefficients can be observed in a meaningful fashion.Fig.7a illustrates the relationship between the Ha and theγ,and the resulting change in thermal entropy production.It indicates that the coefficient of thermal entropy reaches its maximum value of 8.4 along the vertical axis whenHa=0.In addition,the size of(STherm)decreases as theHaincreases from 0 to 100,but the opposite behavior is represented in the case of increasing the magnitude of theγ.An increase in theγexplains this effect by raising the temperature gradients within the enclosure through increased convection currents.Fig.7b shows the variation in the viscous entropy coefficient as a function ofγandHais shown in.It indicates that whenHaincreases,the(SVisc)profile drops,while theγcauses the profile to rise.The argument behind this is that an increase inγcauses a greater temperature difference between the inside and outside of the enclosure, causing thermal buoyancy forces to be formed and, ultimately, executes a convective current.

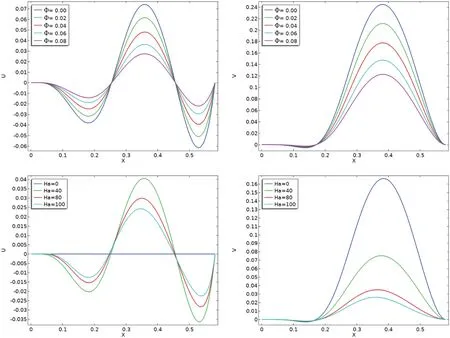
Figure 6:Velocity line graphs for different φ and Ha at x=0.5(verticle)

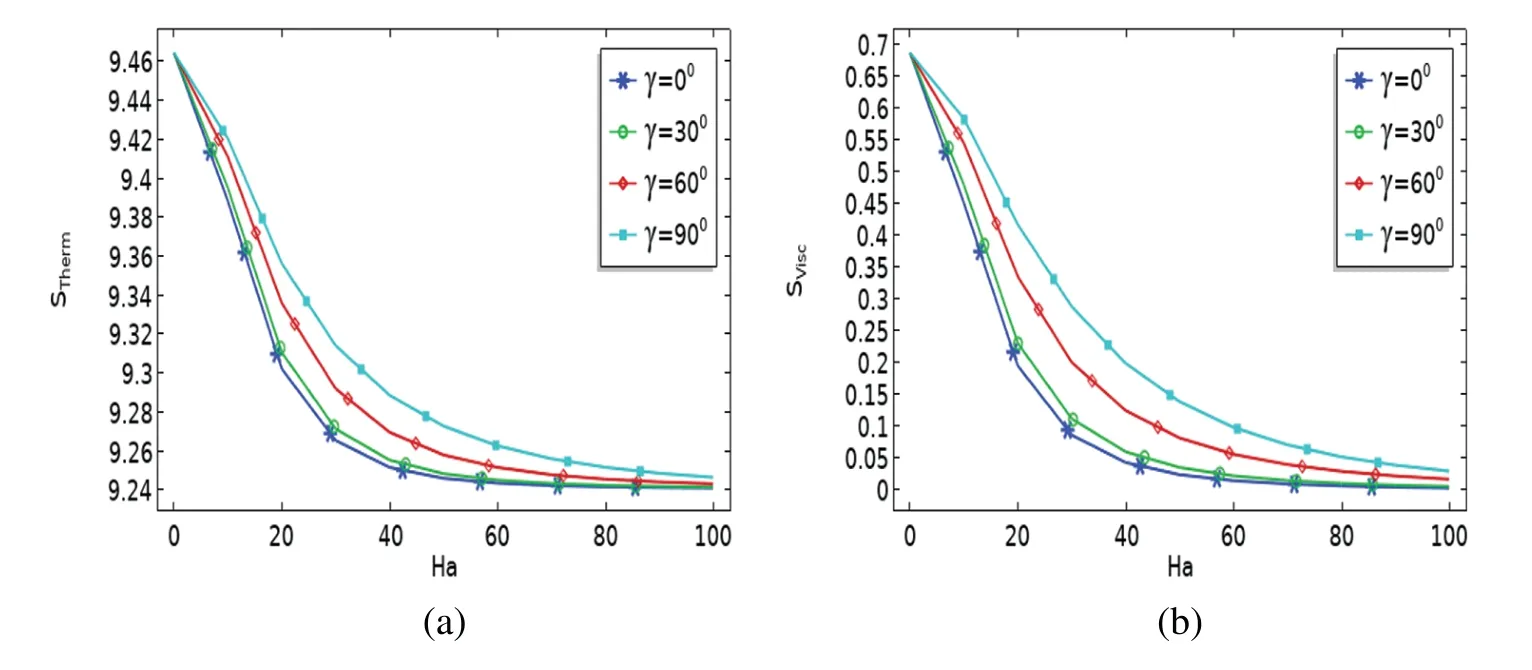
Figure 7:(a–b)Entropy variation against(γ)

Fig.8 reveals the dependence of the heat flux coefficient on theHaand theφ.It indicates that theNuavgrises asHagrows in magnitude,with valuesφranging from 0.02 to 0.08.

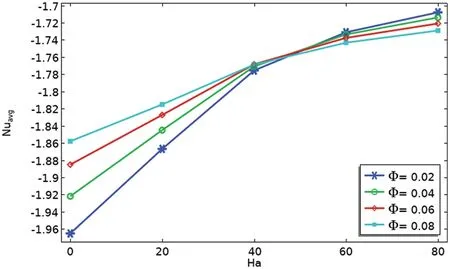
Figure 8:Impacts on Nuavg for numerous Ha

5 Conclusion

This study discusses the issue of irreversibility during the natural convective thermal flow of a Cu-H2Onanofluid inside a cavity.The effect of the magnetic field’s inclination on the irreversibility process is evaluated by applying the field at varying angles.An upward temperature gradient is created in the flow domain by heating the upper wall of the enclosure at a consistent rate while cooling the other borders.The problem considered mathematically is formulated as a connected partial differential system.Utilizing a finite element approach allows the governing equations to be solved.As a result,the current study focuses on making predictions about the elements that influence entropy measurements and disclosing any differences we find in the entropy generated within the enclosure.The most important results are listed below:

• Because of the created temperature disparity,heat is transferred in the shape of parabolic curves and starts at the top of the enclosure.

• ForHa=0 withφ=0.02 andγ=30ofixed,a symmetrical stream line contour is produced at the mid plane,x=0.5.

• ForHa>0,a diminishing trend of(STherm)with an increasing value ofφwas observed.However,forHa= 0, a consistent magnitude of (STherm)across the range ofφfrom 0.02 to 0.08 was observed.

• It can be observed that asφshifts,the corresponding curves show fluctuations in(SVisc)going to zero.

• It is widely accepted that the variation in magnetic entropy becomes zero atHa= 0,rises forHa<40,and then declines after passing this critical range.

Acknowledgement:The authors extend their appreciation to the Deanship of Scientific Research at King Khalid University for funding this work through a small group Research Project under Grant Number RGP1/154/44.

Funding Statement:The authors received no specific funding for this study.

Author Contributions:The authors confirm their contribution to the paper as follows:study conception and design: Afraz Hussain Majeed, Sayed M Eldin; methodology: Imran Siddique, Rashid Mahmood; data collection: S.Saleem, Muhammad Jawad; analysis and interpretation of results: Rashid Mahmood Sayed M Eldin;draft manuscript preparation:Muhammad Jawad,Afraz Hussain Majeed;writing—review and editing:Imran Siddique;funding acquisition:Sayed M Eldin:supervisor:Rashid Mahmood.All authors reviewed the results and approved the final version of the manuscript.

Availability of Data and Materials:The data used to support the findings of this study are available from the corresponding author upon request.

Conflicts of Interest:The authors declare that they have no conflicts of interest to report regarding the present study.