Quantum phase transitions in CePdAl probed by ultrasonic and thermoelectric measurements

Hengcan Zhao(赵恒灿) Meng Lyu(吕孟) Jiahao Zhang(张佳浩) Shuai Zhang(张帅) and Peijie Sun(孙培杰)

1Beijing National Laboratory for Condensed Matter Physics,Institute of Physics,Chinese Academy of Sciences,Beijing 100190,China

2School of Physical Science,University of Chinese Academy of Sciences,Beijing 100049,China

3Songshan Lake Materials Laboratory,Dongguan 523808,China

CePdAl has been recently recognized as a frustrated antiferromagnetic heavy-fermion compound with a pressureor field-tuned, extended quantum critical phase at zero temperature. Identifying characteristic signatures of the emerging quantum critical phase, which are expected to be distinct from those near a quantum critical point, remains challenging.In this work, by performing ultrasonic and thermoelectric measurements down to very low temperatures in a 3He–4He dilution refrigerator in the presence of magnetic field, we are able to obtain some crucial thermodynamic and thermal transport features of the quantum critical phase,including a frustration-related elastic softening detected by ultrasound and a Fermi-surface change probed by thermoelectric effect.

Keywords: quantum phase transition,ultrasound,elastic constant,thermoelectric power

1. introduction

Quantum phase transitions (QPTs) are those that are driven by quantum instead of thermal fluctuations at low enough temperatures.[1,2]They are at the forefront of current condensed matter physics, offering possibilities for a variety of quantum emergent phenomena in their vicinity including unconventional superconductivity and non-Fermi liquid.[3,4]Heavy-fermion materials are ideal systems for exploring QPTs due to the competition between the intersite Ruderman–Kittel–Kasuya–Yoshida (RKKY) interaction and the local Kondo effect. Prototypical examples are secondorder antiferromagnetic(AFM)QPTs which can be smoothly suppressed to absolute zero at a quantum critical point(QCP)by nonthermal tuning parameters like magnetic field and pressure, beyond which a heavy Fermi-liquid phase develops generically as depicted by the Doniach phase diagram.[5]

Thus far, various low-temperature probes have been employed to detect potential QPTs in heavy-fermion materials.Among these,electrical resistivity provides probably the simplest approach through its temperature dependence that frequently deviates from the Fermi-liquid description. On the other hand, low-temperature Hall effect has emerged as a powerful measurement to identify the characteristic feature of Mott-type QPTs, where breakdown of the local Kondo effect occurs with a consequent change of Fermi-surface volume.[6]In addition, thermodynamic and magnetic responses such as the coefficients of electronic specific heat and thermal expansion, and the magnetic susceptibility usually show diverging behavior near a QCP.[2,4]These probes are nevertheless frequently insufficient in characterizing complicated QPTs in,for example,a geometrically frustrated Kondo lattice with enhanced quantum fluctuations and potential metallic spin-liquid phase.[7]

Hexagonal CePdAl shows rich quantum states due to magnetic frustration associated with the distorted kagome lattice.[7,8]At ambient pressure and zero field, it undergoes an AFM transition atTN=2.7 K with only 2/3 of the Ce moments on the kagome lattice involved,[9]see Fig. 1(a) inset.The other 1/3 is Kondo screened, giving rise to the heavyfermion behavior with an electronic specific-heat coefficientγ=270 mJ/mol·K2(Ref.[10]).Applying magnetic field along the Ising moment(thecaxis)causes three AFM phases(a–c)in the low-field phase space that are terminated by three firstorder metamagnetic lines (Bab,Bbc, andBcp), see Fig. 1(a).Further increasing field leads to a quantum critical phase (p)before a Fermi-liquid phase(f)eventually settles down.Alternatively, the AFM phase can be smoothly suppressed to zero by hydrostatic pressure as well, with no metamagnetic transitions taking place. In this case, a much more extendedpphase can be identified by the linear-in-temperature resistivity,a hallmark of non-Fermi-liquid behavior.[7]At elevated temperaturesT ＞1 K,except for theTN(B)line which traces the smoothly reduced AFM transition,two additional linesBm(T)andB*(T) are also shown in Fig. 1(a).Bmis related to the onset of AFM short-range order andB*indicates the Motttype Fermi-surface crossover, which terminates at a Kondobreakdown QCP at zero temperature. As shown in Fig. 1(b),the three metamagnetic transitions can be simply identified by resistivity as a function of field,revealed as sharp anomalies in dρ/dB,where the existence of thepphase can be confirmed by a shallow shoulder.

Fig. 1. (a) Field–temperature phase diagram of CePdAl adapted from Ref. [8]. See text for definition of the symbols used in this figure. Inset illustrates the in-plane magnetic structure determined by neutron powder diffraction.[9] (b) Isothermal resistivity ρ and the derivative dρ/dB as a function of field measured at 0.08 K, from which all the low-temperature phase boundaries can be identified(see vertical lines). Different to the sharp anomalies at the three metamagnetic transitions, the Mott-type transition at B*≈4.6 T behaves as a shallow shoulder in dρ/dB.

This work aims to demonstrate some distinctive features of the quantum critical phase and the related Fermi-surface change in CePdAl by low-temperature ultrasonic and thermoelectric measurement,both of which have so far received much less attention in studying QPTs. Ultrasound can probe magnetic, electronic and lattice instability sensitively because of the spin-lattice and electron–phonon coupling.Heavy-fermion materials are particularly suited for such measurement due to their strongly volume-dependent hybridization effect between the localizedfelectrons and the conduction bands that is described by a strong electron–phonon coupling.[11,12]Likewise,thermoelectric effect measures particle–hole asymmetric band structure at the Fermi level and is therefore suited for detecting Mott-type QPTs where a Fermi-surface change takes place.As will be shown below,the two measurements have revealed characteristic and unique features in support of the occurrence of a frustration-assisted intermediate quantum critical phase and a Fermi-surface change in CePdAl.

2. Experimental details

The ultrasound measurements were performed by using phase comparison technique[13]—a well-established method for probing weak elastic change of solid materials by comparing the input and output ultrasound pulse. High-frequency ultrasound of typically 5 MHz–100 MHz is generated and detected by LiNiO3piezoelectric transducers that are bonded onto two parallel end faces of the sample to be studied, see the inset of Fig. 2. Thiokol liquid polymer is used as bond material between the transducers and the sample. The relative ultrasound velocity changeδυ/υduring a temperature or field scan is proportional to the sum of three parts: the frequency changeδ f/fand the phase shiftδψ/ψof the input and output ultrasound waves, and the sample length changeδL/L, which is however negligible in most cases compared to the former two terms. In this technique the frequency of the input ultrasound wave is continuously adjusted in order to maintain a constant phase of a given output echo (δψ=0),one can therefore probeδυ/υsimply by monitoringδ f/f.Elastic constantCcan be calculated from the sound velocity,C=dυ2, withdbeing the sample density. To ensure a sufficient thermal conductance for achieving temperatures down to below 50 mK,the transducers were bonded on one side by silver paste to the cooper plate that is thermally coupled to the cold finger of a3He–4He dilution refrigerator. The typical sample thickness for ultrasound measurement is 1 mm–3 mm and a single crystal has to be used in order to probe multiple independent elastic modes of the elastic constant tensor.[13]

The thermoelectric effect was measured with a standard setting of thermal-transport measurement by using two thermometers and one heater.A sample of a typical size 0.3 mm×0.5 mm×3.0 mm was used and mounted onto a silver block by silver paste that is directly screwed to the sample holder.[14]The thermometers and heater are,on one hand,suspended by fine nylon wires in a vespel frame in order to be thermally decoupled from their surroundings,and on the other hand,thermally coupled to the sample by using 50-μm gold wires in order to build and measure the temperature gradientδT,which is typically 2%–5%of the base temperature. We use RuO2bare resistive chip sensor (Lake Shore Cryotronics) as thermometer,and 1-kΩ chip resistor as heater. For electrical leads in the sample holder superconducting NbTi wires(φ=25 μm)were used. The measurement was performed in a dilution refrigerator down to approximately 50 mK.

3. Results and discussion

Figure 2 shows relative change of the elastic constantsC11andC33measured at 80 mK in the presence of a sweeping magnetic field(B‖c). They both are longitudinal elastic mode,measured with the ultrasound wave propagating/polarizing along theaandcaxis (Fig. 2 inset), respectively. Upon increasing field, the two elastic constants soften smoothly due to enhanced spin fluctuations, and the first-order metamagnetic transitions atBabandBbcmanifest themselves as a drastic drop. The field variation of elasticity is similar forδC11/C11(B) andδC33/C33(B) up to slightly belowBcp ≈4.2 T, conforming to the low-temperature coefficient of magnetostriction, where sharp peaks indicative of significant lattice-parameter change are observed at the metamagnetic transitions.[15]Further increase of magnetic field results in the most fascinatingpphase in this material, whereδC11/C11(B) andδC33/C33(B) behave totally differently: at the phase boundary between the magnetic phasecand the quantum criticalpphase,Bcp, the former elastic mode reveals a strong softening,whereas the latter turns harder drastically. Consequently,a significant elastic softening is observed in onlyC11(B)in the emerging quantum criticalpphase, see the shaded region in Fig. 2. This distinctive feature offers a piece of compelling evidence for the existence of thepphase.Furthermore,the contrasting elastic behavior between the two elastic modes within the quantum critical phase provides a straightforward and strong implication on the microscopic origin of this phase. Considering thatC11measures the response of the compressional ultrasound wave within theabplane that is composed of distorted kagome lattice, whereasC33measures along thecaxis, one can naturally infer that the additional elastic softening in thepphase observed solely inC11is caused by the in-plane magnetic frustration. On the other hand,the smooth hardening in the heavy Fermi-liquid regime reflects, at least partially, the increased energy scale of the Fermi-liquid phase(TFL(B),Fig.1(a))and the therefore modified electron–phonon coupling.[12]

Fig.2. Relative changes of the isothermal elastic constants C11 and C33 measured by longitudinal ultrasound propagating along the a and c axes,respectively, as a function of field (B‖c) at T = 80 mK. Note that δC11/C11 is multiplied by a factor of 3 for better comparison. The three dashed lines indicate the positions of the metamagnetic critical fields Bab,Bbc,and Bcp. The shaded region marks the field window where the quantum critical p phase takes place,see Fig.1(a). Inset: a sketch of the sample setting for ultrasound measurement, where the directions of propagation k and polarization u are parallel to each other when longitudinal ultrasound is used.

We now turn to the thermoelectric effect, which is also unique in characterizing QPTs for its sensitivity to Fermisurface change. It has been previously studied in the canonical heavy-fermion compound YbRh2Si2,[16]yielding strong evidence for an abrupt Fermi-surface change at the QCP. In Fig.3,we show the isothermal thermoelectric coefficientS(B)measured at various temperatures for CePdAl. A pronounced maximum arising from magnetic scattering is observed in the field window where the sequence of the metamagnetic transitions takes place. While the position of the maximum,marked by downward arrow,traces roughly the metamagneticBcpline(see Fig. 1(a)), unfortunately one cannot clearly identify any of the three metamagnetic transitions. Markedly,at high fieldB ＞BFL(upward arrow), the value ofS(B) remains approximately constant, as expected from a heavy Fermi-liquid with a large Fermi-surface volume that incorporates both the local moments and the conduction electrons. Note that, there appears a valley between the broad maximum and the flatS(B)atB ＞BFL, which falls into the extended intermediate phase space between the AFM and the heavy Fermi-liquid phase.This feature cannot be simply related to thepphase, but is most likely a signature of the thermally-broadened, small-tolarge Fermi-surface crossover as indicated by theB*line.

Though the metamagnetic transitions cannot be identified due to the low signal-to-noise ratio, theS(B) curves can provide a strong evidence for the Fermi-surface change. Comparing the weakly field-dependentS(B) atB ＜2 T in the AFM phase and the nearly constantS(B) atB ＞BFLin the heavy Fermi-liquid phase, one immediately recognizes a change of their absolute values that is attributable to the Fermi-surface change across the Mott-type(Kondo-breakdown)lineB*.This feature becomes actually more apparent at higher temperatures simply because the absolute value of thermoelectric power increases substantially with temperature, see Fig. 3 main panel and inset. To lend further support to this inference, in Fig. 4 we compare the isothermalS(B)and the Hall resistivityρH(B)measured at 0.3 K(Ref.[8]). Significantly,the differentS(B)behaviors at low and high fields, as mentioned above, match well with theB-linearρH(B)of slightly different slope in the two field intervals; see the two dashed lines on top of theρH(B) data. As has been discussed previously, the large-tosmall slope change ofρH(B)is a distinct feature of the smallto-large Fermi-surface change across the Mott-type crossover lineB*(Ref. [8]). Likewise, the afore-mentioned change ofS(B) between low and high field is believed to be a hallmark of the Fermi-surface change, too. The feature that the lowfieldS(B) in the AFM phase with a small Fermi-surface is more susceptible toBin comparison to its high-field counterpart shares strong similarities with the field dependence ofρ(B)(Ref.[8]),and can be ascribed to the enhanced electron scattering from spin fluctuations in the ordered phase.

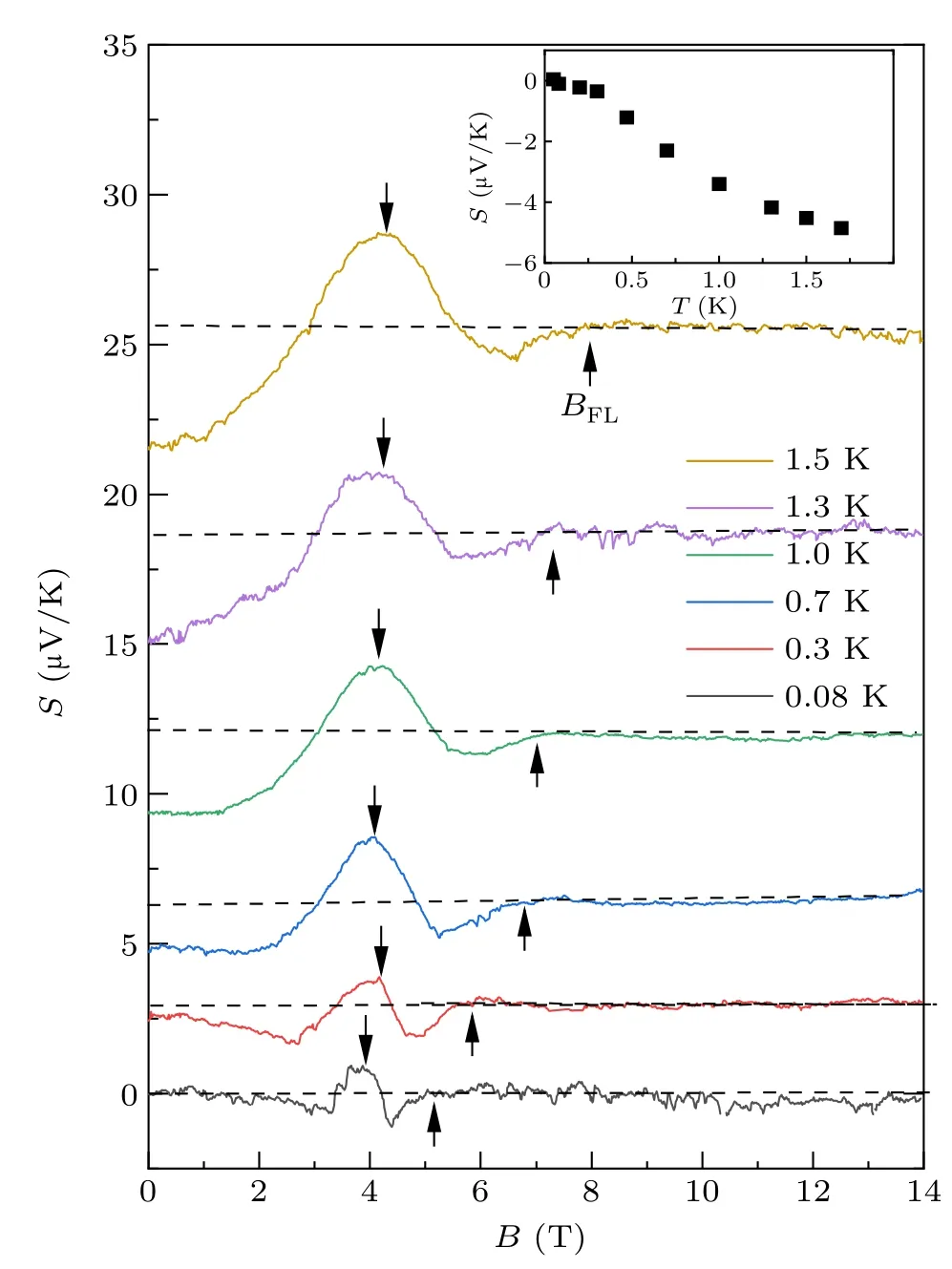
Fig. 3. Isothermal thermoelectric coefficient S(B) measured at various temperatures is shown with proper offset for clarify. Inset: temperaturedependent thermoelectric coefficient S(T)measured in zero field. For these measurements, the temperature gradient is applied within the ab plane and the magnetic field along the c axis. The downward arrows mark the maximums of the S(B) curves, which trace roughly the metamagnetic Bcp line(Fig. 1(a)). The upward arrows indicate the characteristic field BFL above which S(B)tends to be constant, signalling onset of the heavy Fermi-liquid phase.

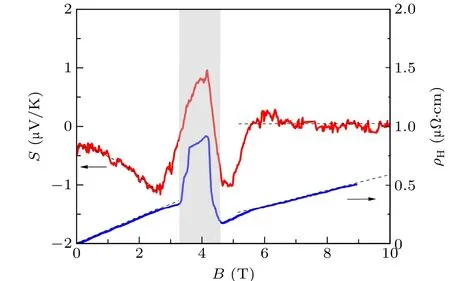
Fig.4.The thermoelectric coefficient as a function of field is compared to the Hall resistivity[8] measured at the same temperature,T =0.3 K.The shaded region indicates the field window where the sequence of metamagentic transitions and the p phase take place. The dashed lines on top of the ρH(B)data indicate the linear variation expected for Fermi liquid,and those for S(B)data are guides to the eyes.

4. Conclusion

To conclude,we have shown that the metamagnetic transitions, the quantum critical phase and the Fermi-surface change across a Mott-type transition/crossover in CePdAl can be probed by ultrasonic and thermoelectric measurements at very low temperatures. In particular, the quantum critical phase can be captured by the in-plane elastic constantC11rather than the out-of-planeC33mode, offering a strong evidence for its existence as well as a strong implication on its origin that is most likely related to the in-plane frustration;the thermoelectric effect can probe the Fermi-surface change,complementary to the Hall-effect measurement.Though much less attention has been paid to these measurements so far,their capability to reveal distinct features on frustration-related fluctuations and Fermi-surface change promises an important role for them in characterizing complicated quantum phases.

Acknowledgments

Project supported by the National Key Research and Development Program of China(Grant No.2017YFA0303100),the National Natural Science Foundation of China (Grant Nos. 12141002, 52088101, and 11974389), the Fund of the Chinese Academy of Sciences through the Scientific Instrument Developing Project (Grant No. ZDKYYQ20210003), the Strategic Priority Research Program(Grant No. XDB33000000), and by China Postdoctoral Science Foundation(Grant No.2020TQ0349).