SU(3)spin–orbit coupled fermions in an optical lattice

Xiaofan Zhou(周晓凡) Gang Chen(陈刚) and Suo-Tang Jia(贾锁堂)

1State Key Laboratory of Quantum Optics and Quantum Optics Devices,Institute of Laser Spectroscopy,Shanxi University,Taiyuan 030006,China

2Collaborative Innovation Center of Extreme Optics,Shanxi University,Taiyuan 030006,China

3Collaborative Innovation Center of Light Manipulations and Applications,Shandong Normal University,Jinan 250358,China

Keywords: spin-orbit coupling,topological phase transition,optical lattice

1. Introduction

Spin-orbit coupling (SOC) plays a key role for several condensed-matter phenomena,such as quantum spin-Hall effect[1-3]and topological insulators,[4-7]and it is also crucial for spintronic devices.[8]The recent experimental realizations of the synthetic SOC in ultracold quantum gases[9-21]provide an ideal platform to investigate quantum many-body physics under a gauge field, in which a lot of parameters can be precisely controlled. In cold-atom systems, the hyperfine states of the alkaline atoms and the clock states of the alkalineearth(-like)atoms have been treated as the internal degrees of freedom of atoms.[22-26]Coupling the hyperfine(clock)states through synthetic gauge fields by the two-photon (singlephoton)process,the SOC has been implemented.[27-32]

The experiments in a gas of87Rb atoms have succeeded in implementing SOC on spin-1 Bose-Einstein condensates(BECs),[33,34]thus paving the way towards the exploration of the interplay between the spinor character and the SOC in determining the novel quantum phases of the system. For example, the interesting magnetic physics have been observed onSU(2) SOC in spin-1 BECs.[35-40]ForSU(2) SOC, the hyperfine states|-1,0,1〉are coupled in forms|-1〉↔|0〉and|0〉↔|1〉by couple two-photon Raman process,with the coupling|-1〉 ↔|1〉missing. After considering the coupling|-1〉 ↔|1〉, theSU(2) SOC will be extended to theSU(3) SOC. Mathematically, theSU(2) spin-1 matrices only include three spin-1 vectorsSσ(σ=x,y,z). However, there exist not only three spin-1 vectors but also spin-1 tensorsNσ´σ=(SσS´σ+S´σSσ)/2-δσ´σS2/3 in theSU(3)spin-1 matrices. TheSU(2) SOC system always has trivial topology.However, theSU(3) SOC involves all the pairwise couplings between the three internal states,and has no analog in ordinary condensed matter systems,hence it may lead to new quantum phases and topological defects.[41-45]

In this paper,we propose an experimental scheme for realizing anSU(3)SOC of fermions in a one-dimensional optical lattice. For single-particle Hamiltonian,the bulk topological physics can be characterized by the Berry phases of the bands and the winding numbers. The local distribution of the edge states inside the band gap under open boundary condition exhibits the bulk-boundary correspondence of the topology.We discuss the interaction-induced topological phase transition via the state-of-the-art density-matrix renormalizationgroup (DMRG) numerical method.[46,47]By calculating the entanglement spectrum, entanglement entropies, energy gaps and magnetic order, we find three topological phase transitions. We then clearly show the three many-body quantum phases, i.e., two topological insulators and a charge density wave phase, by the density profile and edge-model density profile.

The work is organized as follows. In Section 2, we present the experimental setup and give the effective Hamiltonian. We analyze the topological properties of the singleparticle case in Section 3. We study the interaction-induced topological phase transition and show the many-body phase diagram in Section 4. The conclusions are given in Section 5.

2. Model and Hamiltonian

in which the interaction strengthUcan be tuned via the external magnetic field through the Feshbach resonance[48]and the strength of the confine optical lattices.[49]

Since the time-reversal,particle-hole,and chiral symmetries are broken,the topological insulator ofHTBbelongs to the unitary symmetry class A (unitary) of the Altland-Zirnbauer classification and is characterized by a Z invariant.[50-53]In the presence of weak interaction,the topological insulator with the Z invariant still exists since the generalized inversion symmetry remains.[54]

Fig. 1. (a) Schematics of the system setup. Green arrows represent the 1D optical lattice along x-direction. Yellow, blue and red arrows represent three Raman lasers. (b)Energy level and the Raman processing. Ω1, Ω2 and Ω3 couple the ground states |gσ〉 (σ =↑,0,↓) to the excited states to achieve the two-photon Raman process. The polarizations of Ω1,2,3 are σ,π,σ, respectively. (c) Schematic picture of the tight-binding model. Here, ts is the spin-conserved hopping between neighboring sites,tso is the spin-flip hopping between neighboring sites,and U is the on-site interaction.

3. Single-particle physics

For single-particle Hamiltonian,one usually fourier transform the real-space Hamiltonian into momentum-space, due to the fact that the momentumkis a good quantum number.Firstly,we rewrite the Hamiltonian(2)as

withC=-2cos(k)ts,andD=2i sin(k)tso.

3.1. Γz=0

WhenΓz=0, the momentum-space Hamiltonian(5)can be diagonalized analytically to get the eigenvaluesEn(k)with band indexn,as shown in Fig.2(a).The lowest and the second bands are gapless.The second and third bands are fully degenerate. It is hard to explore the band topology. Diagonalizing the tight-binding Hamiltonian Eq. (2) under open boundary condition,we can obtain the energy spectrum as a function of the number of states. There exist four-fold degenerate zeroenergy edge states inside the third and fourth bands,as shown in Fig.2(b).

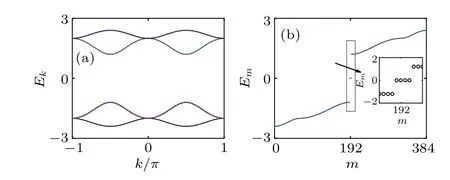
Fig. 2. (a) The dispersion under periodic boundary conditions, and(b)the dispersion under open boundary conditions. For all subfigures,we have tso/ts=0.6,Γz/ts=0 and L=128.

In order to clearly show the band topology, we block diagonalize the momentum-space Hamiltonian (5) in basis

with flavorsξ=⇑,0,⇓. Figure 3 shows the windings ofhξ(k). Numerically, the winding numberω⇑=1,ω0=-1 andω⇓=-1.

Fig.3. (a)h⇑(k),(b)h0(k),and(c)h⇓(k). For all subfigures,we have tso/ts=0.6 and Γz/ts=0.

We fourier transform the block diagonalized Hamiltonian(6)into real-space. The real-space effective Hamiltonian can be written as

whereA=0.75ts+i0.65tso-i0.65ts-0.75tso,B=0.75tsi0.65tso-i0.65ts+0.75tso,C=-0.75ts+i0.65tso+i0.65ts-0.75tsoandD=-0.75ts-i0.65tso+i0.65ts+0.75tso.The energy spectrum ofH′TBunder an open boundary condition has six-fold degenerate edge states in zero energy.

3.2. Γz>0

in which|ψn(k)〉is the corresponding eigenvectors.The Berry phasesγnas a function ofΓz/tsare shown in Fig.4(a). When 0≤Γz/ts<2,γ1/π=1,γ2/π=-1 andγ3/π=-1. When 2<Γz/ts<4,γ1/π=1,γ2/π=-1 andγ3/π=1. WhenΓz/ts> 4,γ1/π= 1,γ2/π= 1 andγ3/π= 1. As demonstrated in Fig. 4(b), we plot the energy spectrumEof tightbinding Hamiltonian (2) under an open boundary condition.When increasing the Zeeman field strengthΓz/tsfrom 0, the zero-energy model splits into two nonzero models, and the gap close atΓz/ts=2.0 and then reopen with two zero-energy models in the gap. Further increasingΓz/ts,the gaps inside the second and third bands close and reopen atΓz/ts=4.0.

We then clearly show the edge-models of differentΓzregimes.As demonstrated in Fig.5,we plot the dispersion and wave functionn(j)of the edge-model inside the energy gaps with (a)Γz/ts=1.0, (b)Γz/ts=3.0, and (c)Γz/ts=5.0. ForΓz/ts=1.0, there are four edge models near the zero energy,with two of them are degenerate, as shown in Fig. 5(a). ForΓz/ts=3.0, there are two-fold degenerate zero-energy edge models. The edge model are occupied by the atom of 0-component in both sides. Inside the second and third band gap, there are two-fold degenerate edge models, as shown in Fig.5(b). ForΓz/ts=5.0,there are two-fold degenerate zeroenergy edge models,as shown in Fig.5(c).

Fig. 4. (a) The Berry phase of each band γn under periodic boundary conditions,and(b)the dispersion E under an open boundary condition as a function of Γz/ts. For all the subfigures, we have tso/ts =0.6 and L=128.

Fig.5. The dispersion E under open boundary conditions and density profile n(j)of the edge states of(a)Γz/ts=1.0,(b)Γz/ts=3.0 and(c)Γz/ts=5.0. For all the subfigures,we have tso/ts=0.6 and L=128.

4. Many-body phases

To understand the many-body topological physics of Hamiltonians (2) and (3), we perform the DMRG method to calculate with the model, for which we retain 300 truncated states per DMRG block and perform 20 sweeps with a maximum truncation error～10-7. Simply, we focus on band topology when the lowest three bands are fully occupied,which corresponds to the fillingn=N/L=3/2 withNbeing the total atoms number.

The topology of many-body system is reflected in the ground-state degeneracy of the entanglement spectrum,which is defined as[58-64]

Here,ρiis the eigenvalue of the reduced density matrix ˆρL=TrR|ψ〉〈ψ|, where|ψ〉is the many-body ground-state wave function,L and R correspond to the left or the right half of the 1D chain. As the entanglement spectrumξiresembles the energy spectrum of edge excitations,the system is topologically non-trivial if and only if each eigenvalueξiis degenerate.[64]In Fig. 6(a), we show the four lowest levels in the entanglement spectrum as functions ofU/ts. While there is a four-fold degeneracy for the ground states in the entanglement spectrum withU=0,the degeneracy is partially lifted in the presence of weakU. As the degeneracy of the entanglement spectrum is generally equal to the dimension of the irreducible projective representation of the symmetry group, the lift of degeneracy can be understood as the reduction of the projective representations into irreducible ones. The existence and the location of the interaction-driven topological phase transition can be further confirmed by entropy.As demonstrated in Fig.6(b),sharp features emerge at the critical points in both the second-order R´enyi entropy[63-69]

As the system crosses the critical point of phase transition,the gap opens. The ground state become from a two-fold degenerate phase to a non-degenerate phase. As the system undergoing another phase transition, the gap close and reopen again,which is the typical of a phase transition.The phase transitions can also be organized by the magnetic order

The derivatives of d〈Sz〉/dUare divergence at the critical points, as seen in Fig. 6(d). The critical points of the phase transitions in Figs.6(a)-6(d)match perfectly.

Fig. 6. (a) The lowest four levels in the entanglement spectrum ξi(i=0,1,2,3), (b)the von Neumann entropy SvN and the second-order R´enyi entropy S2,(c)the energy gap Egap for a chain with L=12 under the periodic boundary condition,and(d)the first derivative of the magnetic order d〈Sz〉/dU,as a function of U/ts. For all the subfigures,we have tso/ts=0.6,Γz/ts=1.4,n=3/2 and L=128.

While the phase boundaries are determined from the above analysis, we further identify the different quantum phases besides the topological phase transitions in Fig. 7.WhenU/tsis negatively large, the ground state of the system is a trivial phase, in which left (right) part of the lattice is vacuum, middle of the lattice is charge density wave, and right (left) part is fully occupied, as shown in Fig. 7(a). In this regime,the ground state is two-fold degenerate due to the mirror symmetry of the 1D lattice.This kind of phase is phaseseparated(PS)phase. WhenU/tsis small and large positive,the ground states are the topological insulators. Here,we use the edge-model density profile to describe the edge states of the topological phases. The edge-model density profile can be defined as

For large positiveU/ts, there exists edge state at one side of the lattice,as shown in Fig.7(b). This topological insulator is two-fold degenerate under the open boundary condition, and is called TI1. Due to the competition between Zeeman fieldΓzand interactionU,〈ˆn↑〉≈0,〈ˆn0〉=0.5,and〈ˆn↓〉≈1. The edge model is occupied by the fermions of|g0〉state,as shown in Figs.5(b),5(c)and 7(b). In this case,the Hamiltonian can map to the spin-1/2 SOC and the effects induced by interaction are weak.For smallU/ts,the topological insulator is four-fold degenerate under the open boundary condition, as shown in Fig.7(c).For each degenerate phase,there are two-component atoms localized at the one side of the lattice. This topological insulator is called TI0.

Fig. 7. (a) Density profile 〈ˆnjσ〉 of PS with U/ts =-7. The edge-model density profile 〈ˆΔnjσ〉 of (b) TI1 with U/ts =6, and (c) TI0 with U/ts=-1. For all the subfigures,we have tso/ts=0.6,Γz/ts=1.4,n=3/2 and L=128.

Based on our understanding of the interaction-induced topological phase transitions and the features of the quantum phases,we map out the ground-state phase diagram of Hamiltonians(2)and(3)in theU/ts-Γz/tsplane,as shown in Fig.8.The phase transition of PS-TI0(red line with circle symbol)is of the first order. The phase transitions of PS-TI1(black line with square symbol)and TI0-TI1(blue line with diamond symbol)are continuous phase transitions. The order of the phase transition can be determined by the derivative of the groundstate energy.

Fig. 8. The phase diagram between U/ts and Γz/ts, with parameters tso/ts=0.6 and n=3/2. The red line with circles shows the first-order phase transition. The blue line with diamonds and the black line with squares denote the continuous phase transitions.

5. Conclusions

Before concluding, we briefly discuss how to observe these quantum phases and phase transitions. In cold-atom experiments, the entanglement entropy can be measured using quantum interference of many-body twins of ultracold atoms in optical lattices.[69]The local distributions can be measured by isolating the sites of interest using additional site-resolved potentials.[70]Thus,all the quantum phases and the phase transitions can be observed experimentally.

In conclusion, we have proposed a scheme to realize theSU(3) spin-orbit coupled three-component fermions in a 1D optical lattice. We study the topological properties of singleparticle Hamiltonian by calculating the Berry phase, winding number and edge states. We also numerically investigate the effects of interactions on the topological properties of the system by employing the DMRG method. The interaction competing with the Zeeman field can induce PS phase and two topological insulators. We have characterized the interactioninduced topological phase boundaries,and map out the phase diagram. Our results provide a way for exploring many-body quantum physics induced bySU(N)SOC.

Acknowledgements

This work was supported by the National Key R&D Program of China(Grant No.2017YFA0304203),the Natural National Science Foundation of China (Grant Nos. 11674200,12074232,and 12004230),the Fund for Shanxi‘1331 Project’Key Subjects Construction, and Research Project Supported by Shanxi Scholarship Council of China.