Suppression and mitigation of inter-ELM high-frequency Alfvén-like mode by resonant magnetic perturbation in EAST

Jiquan ZHAO (赵济全),Youwen SUN (孙有文),Nan CHU (楚南),Tonghui SHI (石同辉),Qun MA (马群),Yumin WANG (王嵎民),Kaiyang HE (何开洋),Youjun HU (胡友俊) and EAST Team

1 Institute of Plasma Physics,HFIPS,Chinese Academy of Sciences,Hefei 230031,People's Republic of China

2 University of Science and Technology of China,Hefei 230026,People's Republic of China

Abstract Suppression and mitigation of a high-frequency Alfvén-like mode(HFAM)between type-I edge localized modes (ELMs) during ELM mitigation by resonant magnetic perturbation (RMP) is observed for the first time in the EAST tokamak.This mode is located near the edge pedestal region.The modeling result of the Alfvén continuum shows that the HFAM is located near the elipical Alfvén eigenmode (EAE) gap.During the application of n=1 RMP for ELM mitigation,the HFAM can be fully suppressed when the RMP amplitude exceeds a threshold,below which the HFAM is mitigated.The suppression is caused by a reduction of pedestal height induced by RMP.In the case using n=3 RMP,the mode is localized toroidally at specific phase depending on the phase of applied RMP,i.e.locked in the three-dimensional equilibrium formed by RMP.The dominant toroidal mode number of HFAM is around n=−6 and it reduces to −3 during the application of n=3 RMP,which indicates the existence of possible nonlinear coupling between the HFAM and RMP.Here the negative mode number denotes that the mode rotates in electron diamagnetic drift direction.The observation reported here improves the understanding of pedestal dynamics and its stability in RMP ELM control.

Keywords: Alfvén-like mode,RMP,HFAM mode,inter-ELM

1.Introduction

High confinement regime (H mode) [1] with Type-I edge localized mode(ELM)[2]is the baseline operational scenario for the ITER.It forms an edge transport barrier that can improve plasma confinement.However,type-I ELM causes pedestal crash,which in turn leads to the loss of particles and energy eventually resulting in damage of the device wall materials.It is a critical issue to understand stability of ELMs in tokamak research.

During the inter-ELM period,there are many unstable modes near the pedestal region in H-mode discharges [3].Among them,a high frequency mode was observed in many tokamaks,i.e.JET[4],C-Mod[5,6],AUG[7,8],DIII-D[9]etc.However,the underline physical mechanism of this high frequency mode is still not clear up to now.Kinetic ballooning mode (KBM) and microtearing mode (MTM) were proposed as two candidates for this mode [6,10],but still need more experimental test to confirm.A high-frequency mode (HFM) has also been observed in EAST [11–13].It propagates in electron diamagnetic direction with a frequency close to that of the Alfvén mode in the range from 150 to 300 kHz withq95～6 in the EAST.It usually appears at the time during the saturation of pedestal pressure,and it is observed in both low field side(LFS)and high-frequency side(HFS).It has an average poloidal wave number ofkθ～0.17 cm−1and inward average radial wave number ofkR～0.13 cm−1.Several instabilities such as KBM and MTM were proposed as candidates[13].However,the nature of the HFM is not clear.Studies on these modes are helpful for understanding the physical mechanism of ELM.

The effects of resonant magnetic perturbation (RMP) on Alfvén instabilities driven by energetic particles [14] have been previously observed in some tokamaks[15–20].RMP is one of the most effective methods for ELM control in present tokamaks [21–26] and will be applied in ITER [27].The general idea of RMP ELM control is to change the edge magnetic topology and enhance the edge transport and hence reduce the pedestal pressure and stabilize ELMs.Therefore,the stability of the high-frequency mode may be influenced during the application of RMP for ELM control.The studies of RMP effects on the stability of this mode may also help the understanding of it.

In this paper,we report for the first time the suppression and mitigation of an inter-ELM high frequency Alfvén-like Mode(HFAM)by RMP in the EAST.The rest of this paper is structured as follows.Section 2 gives a general description of the characteristics of the inter-ELM HFAM observed in the EAST.Section 3 presents the observation of suppression and mitigation of the HFAM by RMP,followed by a summary and conclusion in section 4.

2.Observation of the inter-ELM HFAM in EAST

2.1.Experimental setup

The EAST is a fully superconducting tokamak aiming for demonstration of long pulse operation and steady state physics studies[28–30].Previous results reported in those papers are mainly observed in highq95(～6)operational window.In this experiment,the target plasma is chosen as closer as possible to the ITER ELMy H-mode operational scenario.The main parameters in these discharges are the toroidal fieldBT=1.5 T,safety factor at 95% of the normalized poloidal fluxq95≈3.6,line-averaged densityNe≈4×1019m−3,and normalized plasma beta βN≈1.5.The additional heating powers from the lower hybrid wave(LHW)and neutral beam injection (NBI) arePLHW=1.8 MW andPNBI=1.4 MW,respectively.In these discharges,a HFAM is often observed between two ELM crashes.

2.2.Characteristics of the inter-ELM HFAM in EAST

Figure 1 shows one example of the observation of the HFAM in the EAST,temporal evolution of emission intensity ofDα(a),and the power spectrum of magnetic perturbation measured by the Mirnov probe(b).Three pairs of high frequency Mirnov coils with a sampling rate up to 1 MHz have been installed in the EAST.Each pair has two Mirnov coils.One pair of Mirnov coils with a toroidal separation 22.11° at θ=−13.2° (negative value represents Mirnov coils are located at lower middle plane) has been used for the toroidal mode number analysis.It can distinguish the toroidal mode numbers in the range ofn=[−8,8].In this discharge,a HFAM with a frequency around 200 kHz is observed between two ELM crashes as shown in figure 1(c).This mode usually appears at the density saturation phase during the recovery of pedestal after an ELM crash and keeps almost constant amplitude and frequency until the next ELM crash as shown in figures 1(c) and (d).The mode quickly saturates after its appearance at the density saturation phase,and it disappears after the ELM crash.This suggests that this mode should be located near the pedestal region and there is a threshold pedestal height for the excitation of the mode.Its amplitude and frequency keep almost constant before the next ELM crash.The dominant toroidal mode numbernis about −5 to−6,which will be shown in the later in figure 8.Here negative value represents that the mode rotates in the electron diamagnetic drift direction.The rotation direction of the mode is consistent with the MTM,but not the KBM.

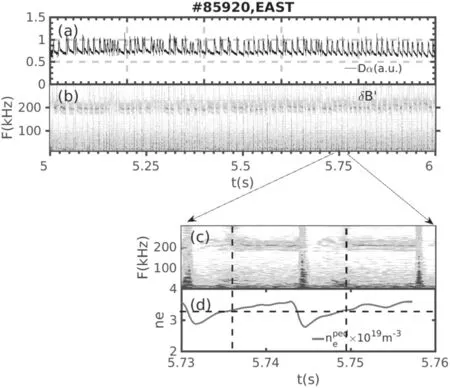
Figure 1.Temporal evolution of emission intensity of Dα (a),the power spectrum of magnetic perturbation measured by the Mirnov probe (b),and zooming in of the power spectrum of magnetic perturbation (c) and pedestal density (d) in a short period in the EAST discharge 85 920.

2.3.Identification of the mode

The frequency of HFAM increases with the decrease of plasma density,indicating that the HFAM might be a Alfvénlike mode.It is shown in figure 2 that the frequency of HFAM depends linearly on the frequency of the toroidal Alfvén eigenmode (TAE) [31],which is evaluated fromtogether with the Alfvén velocityHowever,the observed frequency is much higher than the TAE mode frequency.Figure 3 shows the Alfvén continuum forn=6 mode in this discharge calculated by using the GTAW codes[32].It is shown that the HFAM is located near the gap of the elipical Alfvén eigenmode(EAE).It might be an EAE mode.

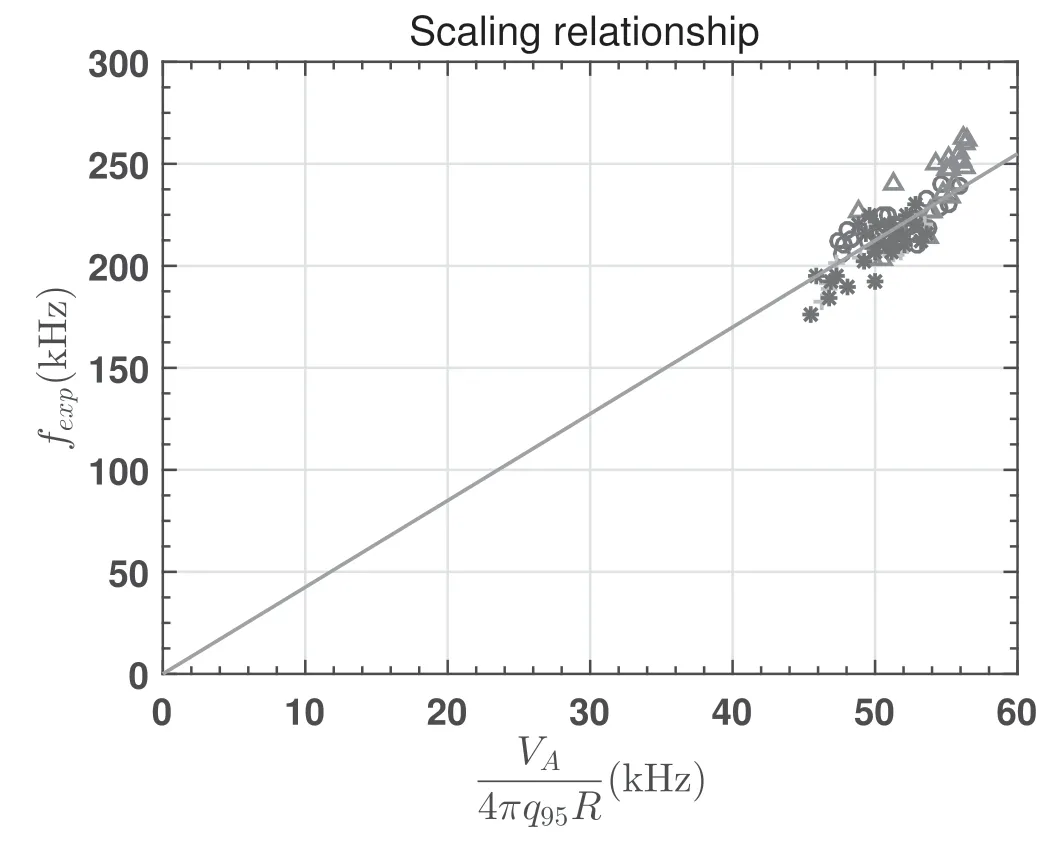
Figure 2.Comparison of the observed mode frequency with the TAE scaling frequency.The data from different discharges is distinguished with different colors and markers.

Figure 3.Alfvén continuum (blue dots) for n=6 mode in EAST discharge 85 920 by GTAW code.The red solid line indicates the possible location of the observed HFAM.

In the Alfvén continuum modeling,the Doppler shift from theE×Brotation is not considered.Figure 4 shows an example of theE×Brotation near the plasma edge at 6 s in this discharge.The maximal Doppler shift for then=−6 mode at the steep pressure gradient region is around−60 kHz.Even subtract this maximal Doppler shift,the frequency of the mode is still around 140 kHz,which is well in the EAE gap and is much higher than the TAE gap frequency.EAE is the ellipticity of the tokamak-induced Alfven eigenmode.The EAE as well as the TAE can be excited by resonance with high energetic particles [33].Unfortunately,we cannot provide the information about the variation of the edge profile of energetic particles to check whether it is driven by energetic particles.

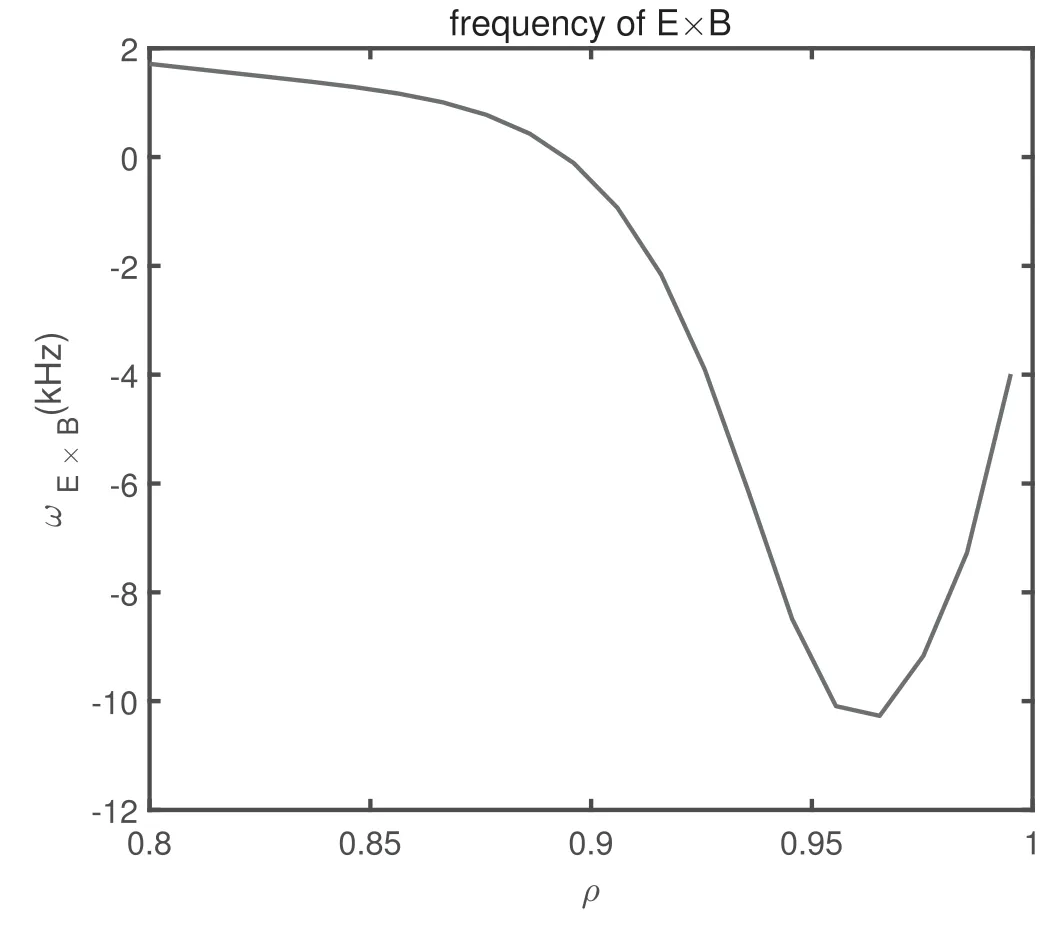
Figure 4.Radial profiles of E×B rotation at 6 s in the EAST discharge 85 920.

3.Suppression and mitigation of the HFAM by RMPs

An in-vessel RMP coil system consisted of 2×8 coils located at low field side upper and lower mid-plane has been installed in the EAST in 2014 [34,35].It can produce magnetic perturbations with a toroidal mode number up ton=4.RMP effects on the stability of the HFAM are further investigated in the following usingn=1 andn=3 RMPs in this kind of target plasmas.Since RMP can form threedimensional equilibrium,the mode behavior may change at different phases.To demonstrate suppression of this mode,it is necessary to rotate the RMP field over one period.Therefore,rotating RMPs are employed in the following studies.

3.1.Suppression of the HFAM by n=1 RMP

Figure 5 shows one example of suppression of the HFAM by an=1 RMP in the EAST discharge 85 907.ELMs are mitigated during the application of then=1 RMP in this discharge.Then=1 RMP is applied at 3.5 s,and the current ramps up from 0 at 3.5 s to 1.5 kA at 4 s.It is rotated for two periods at the RMP current flat top withIn=1=1.5 kA from 4 s to 7 s.It is shown in figure 5(b)that the HFAM amplitude gradually decreases and finally disappears at around 3.74 s when the amplitude of then=1 RMP exceeds a threshold.During the ramp down of RMP current,the HFAM appears again when the RMP current is lower than a threshold.

Figure 5.Temporal evolution of line averaged density (a), Dα emission (b),power spectrum density of Mirnov probe signals (c),and the n=1 RMP(d)current amplitude(red line)and phase(blue line) in the EAST discharge 85 907.

Figure 6.Temporal evolution of power spectrum density of magnetic perturbations aligned with the times for three ELM crashes at t1=3.470 s (a), t2=3.745 s (b), t3=3.862 s (c) and the corresponding evolution of pedestal densities(d).

It is noted that the increase of frequency of HFAM before its full suppression is due to the reduction of plasma density induced by RMP,i.e.the so-called density pump out effect[35].The frequency dependence clearly shows an Alfvén-like one again.

Figure 6 shows the temporal evolution of power spectrum density of magnetic perturbations aligned with the times for three ELM crashes att1=3.470 s (a),t2=3.745 s (b),t3=3.862 s (c) and the corresponding evolution of pedestal densities(d).The first one in figure 6(a)is for the case without RMP,the second one in figure 6(b) is for HFAM mitigation,and the third one is for the case that the HFAM is completely suppressed.The corresponding temporal evolution of the density recovery after ELM crashes in figure 6(d) suggests that the reduction of HFAM amplitude and the suppression of it may be due to the reduction of pedestal height during the application of RMP.This HFAM may enhance the transport near the pedestal region and increase the time for pedestal recovery after an ELM crash,i.e.the ELM period.Therefore,the disappearance of this mode may also contribute an increase of ELM frequency during the application of RMP.Because of ELM mitigation,the pedestal height cannot recover to a level high enough to trigger the HFAM.This suggests again the mode is near the pedestal region.The edge pressure gradient of energetic particles may also recover after the ELM crash.The HFAM suppression may also be caused by the reduction of edge pressure gradient of energetic particles during the application of RMP.However,we cannot measure this information.The HFAM is suppressed while ELMs still exist,which suggests that the HFAM is not a precursor mode that leads to an ELM crash [36].

Figure 7.Temporal evolution of Dα emission (a),power spectrum density of the Mirnov probe signal (b) and the sum of it in the frequency range 150–320 kHz(c),and the RMP coil current (d)amplitude (red line) and phase (blue line) in the EAST discharge 85 900.

3.2.3D distribution of HFAM during its mitigation by n=3 RMP

Figure 7 shows another example for HFAM mitigation by an=3 RMP in the EAST discharge 85 900.Then=3 RMP is rotated for two periods with a constant currentIn=3=3 kA.The HFAM cannot be suppressed with this highest availablen=3 RMP coil current.This means the changes of pedestal height induced byn=3 RMP ELM mitigation in this case might be weaker than those in then=1 RMP case,which might be due to the reason that the highernnumber has a shorter decay length of resonant field around the RMP coil.

The HFAM disappears and reappears periodically depending on the phase,then=3 RMP.It disappears in the phase range around φ∈[100°,200°].Figure 7(b)shows that the HFAM is even enhanced during the phases in the range of φ∈[−60°,60°].Since the amplitude of then=3 RMP is kept constant,the disappearance of the mode at some phases does not mean full suppression.This is due to the formation of 3D distribution of HFAM locked in the 3D equilibrium formed by then=3 RMP,similar to the previous observations on turbulence behavior during the application of RMP[37,38].Because of the formation of 3D structure induced byn=3 RMP,the local pedestal gradients at different toroidal locations might be enhanced or reduced depending on the phase [37].These kind of 3D effects may destabilize the mode at certain toroidal phase but stabilize or suppress it at another phase.

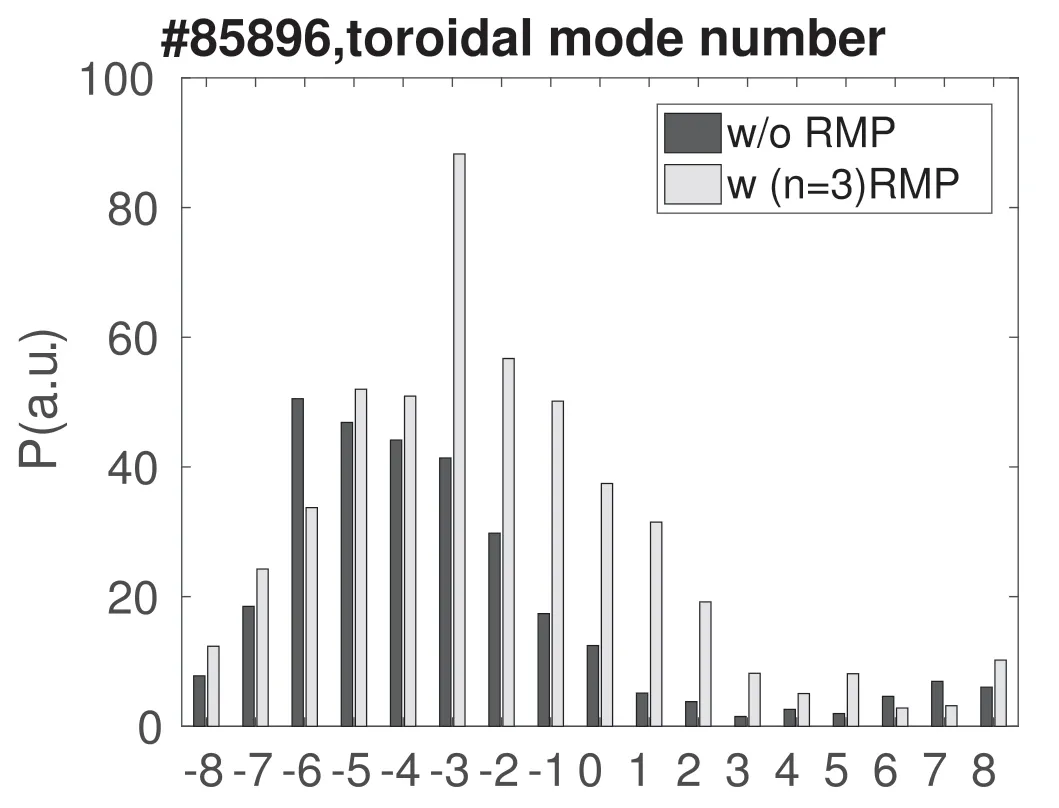
Figure 8.Comparison of power spectrum densities for different toroidal mode numbers of the HFAM with (black bars) and without(yellow bars) n=3 RMP.

3.3.Changes the HFAM mode structure by n=3 RMP

Evaluation of the toroidal mode number of the HFAM using the Mirnov coils at different toroidal positions indicates that it has a broad distribution in toroidal mode number,as shown in figure 8.The dominant mode number without RMP is aroundn=−5 to −6.After the application ofn=3 RMP,the dominant mode number is shifted to aroundn=−3.A shift of dominant mode number is also observed during the application ofn=1 RMP.This suggests that there is a nonlinear coupling effect between HFAM and the RMP.

3.4.Variation of the HFAM frequency during the application of n=3 RMP

Figure 9 shows a comparison of the power spectrum densities of the Mirnov probe signal without (a) and with (b)n=3 RMP in the EAST discharge 85 896.As also presented above the frequency of HFAM without RMP shown in figure 9(a)keeps almost constant in each period.During the application ofn=3 RMP,there is relatively obvious variation of the mode frequency in each period.It ramps up almost linearly with a magnitude about 20 kHz.Since the density increases during this phase,it is impossible due to the change of Alfevén frequency,which should decease in this period.One possible contribution is the change of Doppler shift due to theE×Brotation,as shown in figure 4.Both a shift of the mode location towards the steep gradient region and an increase of localE×Brotation due to pedestal recovery can contribute an increase in mode frequency.Both of these two effects may contribute to the increase of mode frequency during the application of RMP.This again suggests that the HFAM is located near the pedestal region.

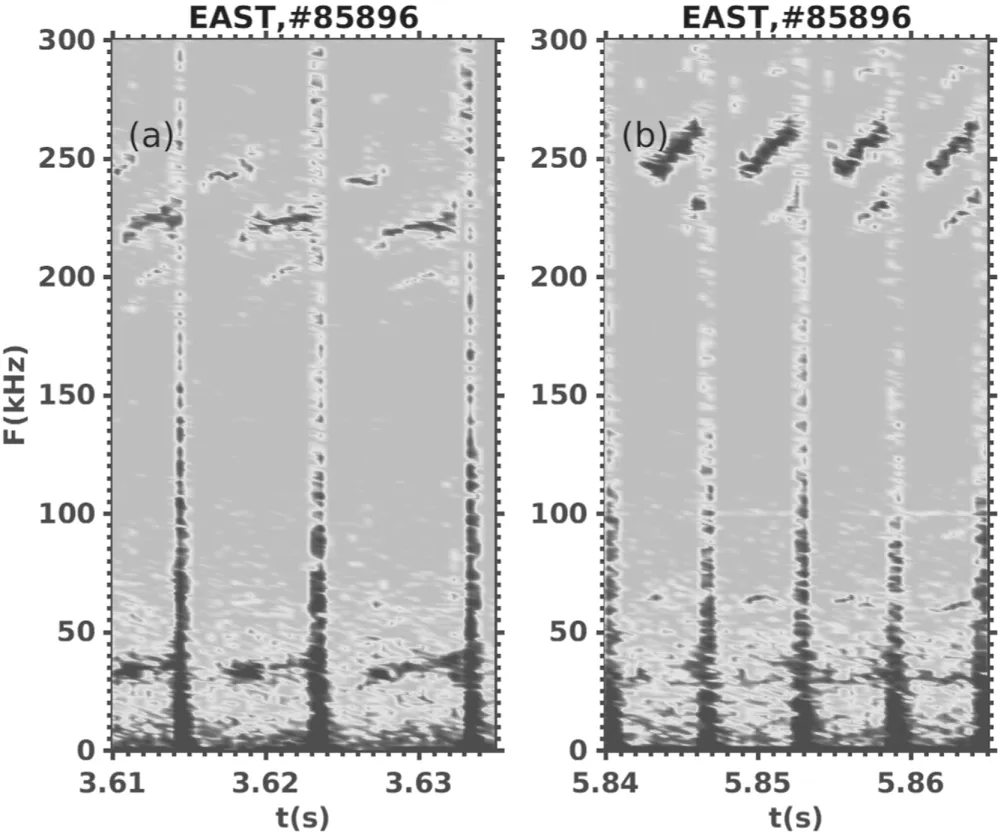
Figure 9.Comparison of the power spectrum densities of Mirnov probe signal without (a) and with (b) n=3 RMP in the EAST discharge 85 896.

4.Summary and conclusion

Suppression and mitigation of HFAM between type-I ELMs during ELM mitigation by RMP are observed for the first time in the EAST tokamak.This mode usually appears at the saturation phase during the recovery of the pedestal after an ELM crash and keeps almost constant amplitude and frequency until the next ELM crash.The mode is located near the edge pedestal region.Frequency of this inter-ELM HFAM is around 150–300 kHz,and it scales linearly with the Alfvén wave frequency.The modeling result of Alfvén continuum shows that the HFAM is located near the EAE gap.During the application ofn=1 RMP for ELM mitigation,the HFAM can be fully suppressed when the RMP amplitude exceeds a threshold,below which the HFAM is mitigated.The suppression is caused by a reduction of pedestal height induced by RMP.The HFAM cannot be suppressed byn=3 RMP with the highest available RMP coil current.In the case usingn=3 RMP,the mode is localized toroidally at a specific phase depending on the phase of applied RMP,i.e.locked in the three-dimensional equilibrium formed by RMP.It can be even enhanced at some specific toroidal phase compared to that in the case without RMP.The dominant toroidal mode number of HFAM is aroundn=−6 and it reduces to −3 during the application ofn=3 RMP,which indicates the existence of possible nonlinear coupling between the HFAM and RMP.Here negative mode number denotes that the mode rotates in the electron diamagnetic drift direction.The HFAM mode frequency is almost constant in each ELM period in the case without RMP,while it ramps up tens of kilohertz in the case with RMP.This cannot be explained by the Alfvén frequency change due to ramping up the edge plasma density.It is possible due to the increase of the amplitude ofE×Brotation during the recovery of pedestal pressure.This suggests that the mode may be shifted a little bit from the position towards pedestal steep gradient region during the application of RMP.The observation reported here improves the understanding of pedestal dynamics and its stability in RMP ELM control.

The detailed information about the driving force of the mode is still not clear to us.It might be linked to the free energy in energetic particles or thermal pressure gradient.Unfortunately,present edge diagnostics in EAST cannot give the detailed evolution of them.This requires the improvement of the edge diagnostics in the future.Detailed dynamics of pedestal structure and its stability change during the HFAM suppression and mitigation by RMP for the deep understanding of ELM physics should be carried out in future studies.

Acknowledgments

This work is supported by the National Key R&D Program of China (No.2017YFE0301100) and National Natural Science Foundation of China (No.11 875 292).