Model Predictions of Charged-Particle Azimuthal Distributions and Forward-Backward Correlations in pp Interactions at = 900 GeV∗
R.Khan, M.Ajaz, Y.Ali, H.Younis, G.Khan, Z.Wazir, A.Zaman, and A.Khan
1Department of Physics,Abdul Wali Khan University,Mardan,23200,Pakistan
2Department of Physics,COMSATS University,Islamabad,44000,Pakistan
3Department of Physics,International Islamic University,Islamabad,44000,Pakistan
Abstract We report inelastic interactions of protons at the center of mass energy =900 GeV,by using different event generators to study the forward-backward (FB) correlation in summed transverse momentum and multiplicity of produced charged particles.The transverse momentum,pT ≥100 MeV,and pseudorapidity,| η |< 2.5,have been employed for both the correlations.The simulation results are compared with the ATLAS experimental data,obtained with the same values of | η | and pT at = 900 GeV.We also measure the jet and jet-like structure as a function of azimuthal distributions in the events relative to the highest transverse momentum particle.Three overlapping regions of pseudorapidity,|η|< 1.0,| η |< 2.0,and | η |< 2.5 are used with transverse momentum,pT > 500 MeV.For FB correlations,EPOS,and Sybill2.3c results are in a good agreement with the data as compared to the other models.Similarly,for the azimuthal distribution,EPOS-LHC,DPMJETIII,and Sibyll2.3c show good agreement with the data for full η region.In general,EPOS provides a better description of the data for both the quantities studied in this paper.
Key words: forward-backward correlation,CRMC models,LHC ATLAS
1 Introduction
Over the past few years,it has been a challenging problem for theoretical physicists to understand the final states of high energy interaction,especially at the Large Hadron Collider (LHC) energies.The momentum range of a huge number of different particles produced during these interactions is of many orders in magnitude.Different techniques have been developed to solve these issues.The factorization tools can be used to separate the different scales of momentum transferred involved.Quantum Chromodynamics (QCD) processes,generating the events of highenergy hadron collisions are thesoft processesand thehard processes.The soft processes,at the very lowest scales,can be pictured in terms of multiple parton interactions,modeled on multiple gluon exchanges.The low momentum soft processes are characterized as the correlations among the number of particles per unit pseudorapidity over an extended pseudorapidity range.[1]The beam confines the incoming partons while the final states hadrons are observed when the outgoing partons interact perturbatively.The first principle calculations cannot be used to calculate these low momentum soft processes; thus,they have to be modeled.For the hard processes,the incoming beam comprises of interacting partons,producing few energetic partons,gauge bosons or leptons that are perturbatively computable.The high momentum hard process can produce particle jets that are defined by a short-range correlation whereas the low momentum soft processes produce low particle multiplicity of weak correlations over an extended pseudorapidity region,called “long-range correlations”.The cross sections for jets produced by hard parton scattering processes quickly decrease as the pseudorapidity distance increases.The strength of each contribution can be probed as one can measure the correlation verses pseudorapidity separation and summed transverse momentum ∑pT,because there is a continuous transition from the soft scale to hard scale as particlepTincreases over a few hundred MeV.
The correlations in pseudorapidity have been widely studied experimentally using pp interactions at the LHC with different detectors.[2−8]These effects have been studied by the UA5 experiment[9]and ATLAS by two-particle angular correlations.[10]We have used the same experimental approach as by ATLAS collaboration,[11]by choosing an equal size of pseudorapidity regions located in the forward and the backward direction symmetrically.This way the forward-backward (FB) correlation of different quantities in specific pseudorapidity regions can be studied.The effects of both scale correlations,the short and the long-range,can be determined as the FB correlations are calculated for the increasing separation of two selected regions of pseudorapidity.
Here,we study FB correlations in multiplicity and in summed transverse momenta(∑pT)of charged particles.One can also study the effects of the soft and the hard process by increasing the minimum values of transverse momentumpTminof charged particles.The later will gradually reduce the effect of the soft process while the effect of the hard process remains.[11]The experimental data used by the ATLAS detector for different center of mass energies can provide insightful information and the experimental results can be compared to the Monte Carlo(MC) models.To explain the background processes and the new physics at LHC energies the relation between soft and hard processes is an important concept to understand.
Different experimental facilities have been used to study particles distribution in azimuthal angles for different energies[12−14]and at the LHC.[15]The distributions indicate jet structure when the two peaks are observed in azimuthal distribution,one at zero and other atπ.The peaks are not observed for the events studied in this measurement,as single event contains a small number of hard particles to form clearly defined jets.However,the peaks become visible statistically by accumulating the distribution over many events.In this paper,we use MC event generators to understand the shape of these peaks.
The rest of the paper is organized as follows.The forward-backward correlations and the azimuthal analysis are presented in Secs.2 and 3,respectively.The different models used for the simulation are briefly described in Sec.4.The results are discussed in Sec.5,and in Sec.6,we give concluding remarks.
2 Forward-Backward Correlations
The correlations measured for symmetric or asymmetric pseudorapidity intervals using charged particles are named as the forward-backward (FB) correlations.The FB multiplicity correlation,,defined as,[11]

It gives the normalized covariance between event distributions,corresponding to the mean value of each.Here,nfandnbrepresent the multiplicities of the particles in the two intervals,Nrepresents the total number of events,represents the mean over the total events in the sample,andare the differences betweennfandnband their means respectively,and the standard deviations ofnfandnbabout their means are,respectively.The sum is taken over all the events.Only those particles of interest are presented for whichpTis above a givenpTmin.
Similarly,the forward-backward(FB)momentum correlation,between two-summed transverse momentums is defined as,
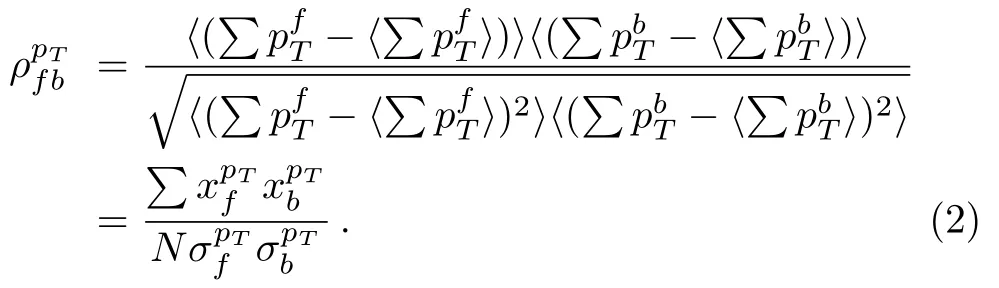
The sum of transverse momentum in the forward and backward regions isrespectively,andare the difference ofwith their means respectively.The standard deviations are represented by,.
3 Azimuthal Distributions
The “leading particle” is the highest transverse momentum (pT) charged particle in a given|η |region.The existence of jet and jet-like structure in an event can be observed with the help of the leading particle.The azimuthal difference (∆ϕ) is defined as the difference of angle between the leading and other accepted particle.The selected number of charged particles isNT,for a given interval ∆ϕof widthδϕ,theδϕhave a fixed value ofπ/50,andpT > pTminis used for simulations as in the experimental analysis.
At first the wily fox refused to tell her what he had gathered from the conversation of the birds, but at last he gave way to her entreaties28, and told her that they had spoken of the misfortunes of a beautiful young Prince, whom a wicked enchantress had turned into a snake for the period of seven years
There should be two peaks in the distributions at∆ϕ=0 and ∆ϕ=π,and a minimum in between,that indicates the case of jet production.Due to the uncorrelated background and overall charge particle activity in the event,these minima become flat or pedestal.[15]In the present study,these pedestal contributions are subtracted and only the peaks of the distributions are focused upon.The normalized subtracted number of particles per radian in azimuthal angle,∆ϕ,gives the quantity,

NTis the observed distributions,fitted with quadratic polynomial to determine the minimum valueto be subtracted from allNTvalues,and summed over various bins of ∆ϕ.is averaged over the number of events in the distribution.It is sensitive to the jet-pairs produced in the acceptable pseudorapidity interval.Due to the quantity,the direct effects of the second jet are subtracted,alternatively suppressing the pedestal effects by studying the second variable.
The leading particles in the same forward-backward(FB) event hemisphere (η × ηlead≥0) inηare distinguished from the ones in opposite hemisphere(η×ηlead<0),and denote it asNsameandNopprespectively.The normalized asymmetry is given as,[11]

The possible existence of a jet can be observed by studying the leading particle.The equation above clearly shows that becomes a large positive value when the azimuthal variable ∆ϕ ≈0 and become small for larger values of∆ϕ.Hence the jet-like structure related to the leading particle is very well described,while the effects of the opposing jets on average cancelled out.Any finite value at large azimuthal distribution reflects long-range correlation in pseudorapidity.Therefore,andimprove the sensitivity of various aspects of jet-like events and are also sensitive to an aspect of long-range correlation that complements the forward-backward measurements.
4 Models and Method
A brief description of the models used to study the FB correlations is presented below.For a detail consideration,one can check the references given for each model.
Energy conserving quantum mechanical multiple scattering approach,based on parton (parton ladders),offshell remnants,and splitting of parton ladders is built into the EPOS simulation.It is a unified formalism based on the combination of the Gribov-Regge theory and the parton model.The soft aspects of the particle interaction,multiple interactions using Pomerons and elementary interaction are the main focus of the Gribov-Regge theory.The precise description of the quark-gluon interactions and the calculations of the cross sections for partons jets by using QCD and QED description are taken care of through the parton model.The EPOS model takes care of the energy conservation for particle production and also for the cross-section.Since 2009,the establishment of the Large Hadron Collider(LHC)has provided a huge amount of data at high energies and to consider all these energies,EPOS was modified to EPOS-LHC.The EPOS-LHC fixes the collective hadronization effects in different interactions.There are basically two flow regimes,the thermalized matter with small volume and high densities to the larger volume produced through heavy ion collisions.A comparison of these flow regimes is also considered in the EPOS-LHC.For a detail discretion of EPOS models can be looked in Refs.[16–17].
The QGSJET model,based on the concept of quarkgluon string model is widely used by a number of experimental and theoretical collaborations for the comparison of data.It works in the framework of the Reggeon field theory[18]for the treatment of hadronic and nuclear interactions.The quark-gluons are treated by Gribov-Regge theory,where creation and fragmentation of two strings arise due to the process of each particle produced.The perturbative parton evolution in the re-scattering process is explicitly treated in this model.The Semi-hard Pomeron approach has been used to represent the sum of the soft parton contribution and the semi-hard parton process in the model.The later dominate the interactions at high energies; hence it permits combining the hadronic scattering amplitudes to the perturbative QCD evolutions.Detail discription of QGSJETII-04 can be found in Ref.[19].
Sibyll is an MC generator used for the extensive air showers.It can be used for general properties of the hadron production i.e.the formation of jets with highpT,the leading particle effects and its distribution,diffractively excited states of the target and projectile,the particle production and the energy flow in the forward space region.The most recent version of this model is Sibyll2.3.The sibyll2.3c includes,data at the LHC energies,high production rate of baryon-antibaryon in string fragmentation,the production of charm particle and the Good-Walker diffraction approach.The two-channel model(ground and excited state)of the hadron interactions with nuclei is implemented that increases the cross-section of the projectile diffraction.Detail discretion of Sibyll can be found in Refs.[20–21].
The DPMJET-III is a Monte Carlo generator,based on the Dual Parton Model (DPM),has a wide range of applications.[22]Most of the Monte Carlo(MC)generators use the basic ideas of the DPM for the soft interactions.The largeNcandNfexpansions of the QCD are combined by the PDM to the Gribov-Reggeon theory.The DPMJET-III is the latest version of DPMJET-II.[23−24]All features of the DPMJET-II,DTUNUC-2,[25−26]and the PHOJET[27−28]are included in the new version.It is possible to simulate the interactions of hadron-hadron,nucleus-nucleus,photon-photon,hadron-nucleus,photonnucleus and photon-hadron from the low energies to the high cosmic ray energies.Detail discretion of DPMJET can be found in Ref.[29].The paper is continuation of the work published in Refs.[30–47]using different hadron production models.
5 Results and Discussions
We have used DPMJETIII,Sybill2.3c,EPOS1.99,QGSJETII-04,and EPOS-LHC Monte Carlo (MC) event generators for pp collisions atwithpT >500 MeV,for three overlapping pseudorapidity intervals:| η |<1.0,| η |<2.0,and| η |<2.5.The model predictions are compared with the ATLAS experimental data[11]obtained with the same parameters.
Figures 1 and 2 show the distributions of the subtracted,,and the same minus opposite charged particle,as a function of azimuthal angle ∆ϕrespectively.The results are compared with the ATLAS data for the center of mass energyThe distributions do not include the leading particle in each event.One notices in Fig.1,as the pseudorapidity increases,the distinction between the peaks becomes more prominent.The visibility of the“opposite”jet peak for increasing value of the| η |in the distributions is related to the fact that more jet-pairs are included in the acceptance range.For|η |<1,the MC models depict the data well.All the models underestimate the data for ∆ϕ<0.6 and overestimate for ∆ϕ >2.5.For 0.5<∆ϕ <2.5,DPMJET-III,EPOSLHC,and the Sibyll2.3c give a good agreement,though the value oscillates around the data.The EPOS1.99 matches the experimental data for 1.0<∆ϕ<2.0.
For| η |<2 and ∆ϕ <1.5,all the models reproduce the data very nicely except EPOS1.99 which underestimate the data about 50%for the initial peak but describes the data for 0.9<∆ϕ <2.7 along with the EPOS-LHC.For the higher values of azimuthal distributions,∆ϕ>2.5,Sybill2.3c,QGSJETII-04,and DPMJET-III overestimate the experimental data.For|η |<2.5,the models behave somewhat similarly,as in the case of|η |<2.The EPOSLHC,DPMJETIII,QGSJETII-04,and Sibyll2.3c describe the data very well for|η |<1.5.
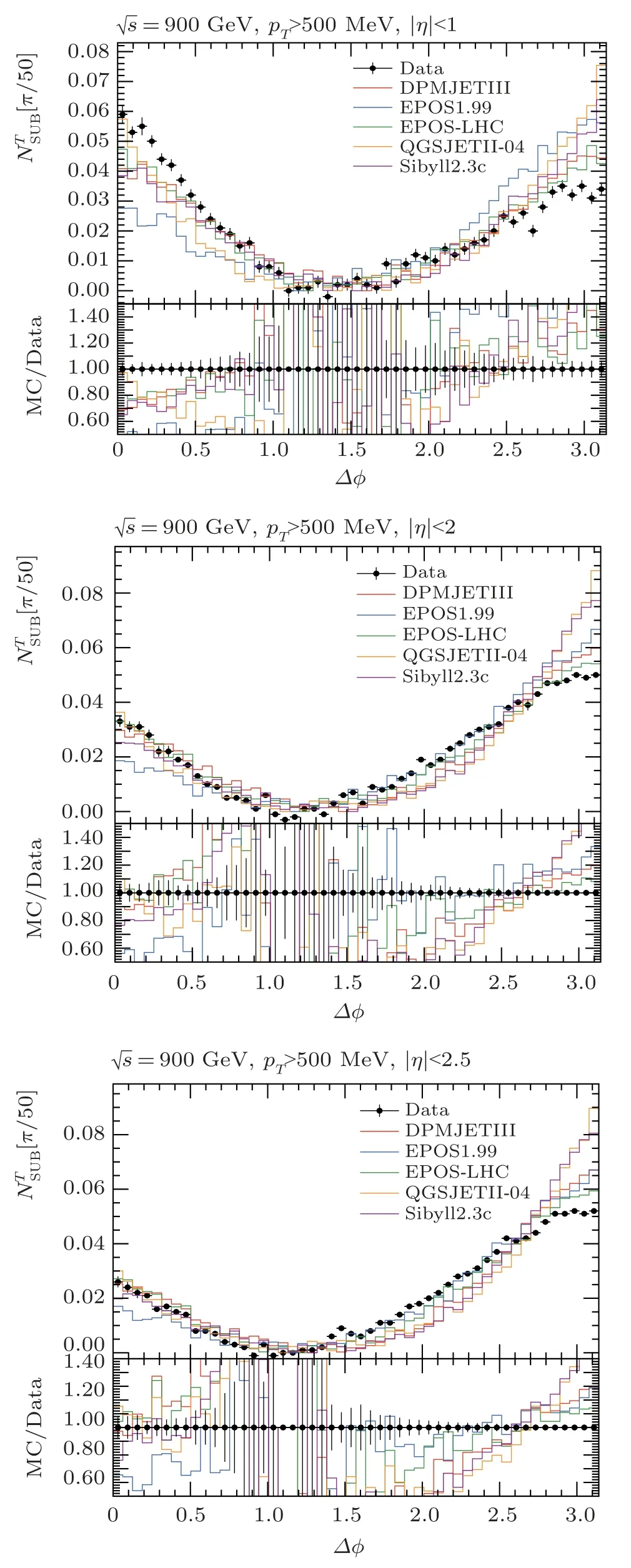
Fig.1 (Color online) Subtracted () and normalized azimuthal (∆ϕ) distributions for different MC generators at =900 GeV.Three pseudorapidity regions used are:| η |< 1.0, | η |< 2.0,and | η |< 2.5.The predictions of different MC models are compared with the data (black symbols).
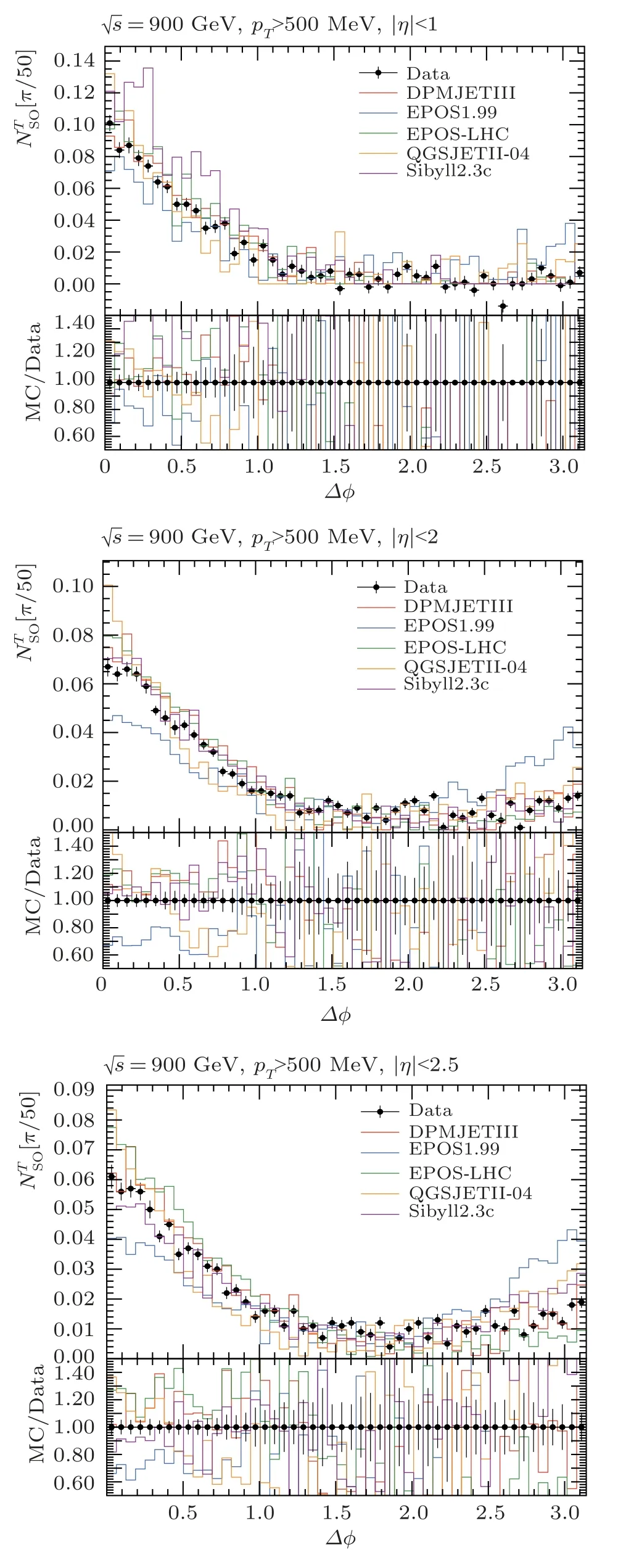
Fig.2 (Color online) “same minus opposite” () and normalized azimuthal (∆ϕ) distributions for different MC generators at = 900 GeV.Three pseudorapidity regions used are: | η |< 1.0, | η |< 2.0,and | η |< 2.5.The predictions of different MC models are compared with the data(black symbols).
In general,the MC models provide a good description of the data for| η |<1 and 0.7<∆ϕ <2.5 but the sharpness of the peak,at the lower values of ∆ϕis underestimated 10% to 72% by the models while at the higher values of ∆ϕthe peak is overestimated by a similar amount.As the rapidity range increases to 2 or 2.5,the agreement of MC models and the experimental data become increasingly poor for the higher values of ∆ϕ,except for the EPOS models.The EPOS models are more successful,showing a good match with the experimental data for the specific higher values of ∆ϕ(0.6<∆ϕ<2.7),|η |<2 and|η |<2.5 bins.The Sibyll2.3c also provides a good match to data but for lower values of ∆ϕ.In all the cases,the same side peak is strengthened compared to the other side peak,showing the inconsistency of the models.A similar comparison,for subtracted distributions,,has been shown in Ref.[11]by using different MC models and tunes atGeV that provides a poor match to data compared to the models used in this study.
In Fig.2,the same-minus-opposite () variable is plotted as a function of ∆ϕfor different models.All the models describe the data well for|η |<1 but for the higher values of ∆ϕ.The EPOS-LHC and DPMJETIII reproduce the data quite well.The Sybill2.3c and QGSJETII-04 exaggerate the sharpness of the peak about 20% while the EPOS1.99 underestimate by a similar amount,for the lower values of ∆ϕ.
For|η |<2,Sybill2.3c,DPMJET-III and EPOS-LHC show a good match to the data for all values of ∆ϕ.The QGSJETII-04 and EPOS-LHC overestimates the peak but gives good results as ∆ϕincreases while the EPOS1.99 underestimate the data 50% for the lower values of ∆ϕand overestimate by a similar amount for the higher ∆ϕ.
As|η |changes to|η |<2.5,all the models give an oscillatory behavior compared to the data except Sibyll2.3c.The later describes the data well for all the values of ∆ϕ.All the models describe the data very well for the sameminus-opposite () variable.The leading particle jet peak and the opposing pedestal are nicely reproduced.The QGSJETII-04 over-emphasizes the sharpness of the peak.The disagreement of the model predictions with the experimental data becomes more and more prominent as the| η |acceptance range is increased but Sibyll2.3c describes the data well.This is from the fact,as the acceptance range increases more and more widely spread pair of jet effects are included in the distributions.
Figure 3 presents the FB multiplicity correlation as a function of pseudorapidityη(for the full pseudorapidity range i.e.| η |<2.5) at the center of mass energy
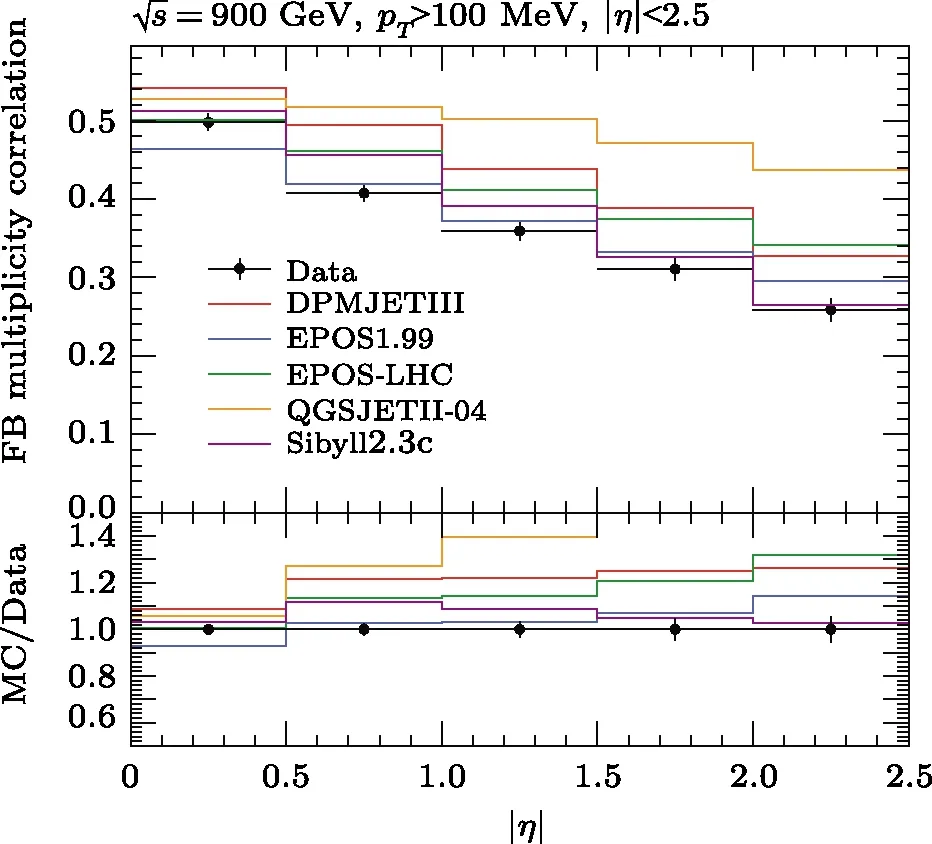
Fig.3 (Color online) The forward-backward (FB) correlation in multiplicity in symmetrically opposite η intervals for events with at least two charged particles with|η |<2.5 and pT ≥100 MeV.Different MC simulations at =900 GeV,compared with ATLAS experimental data (black symbols).
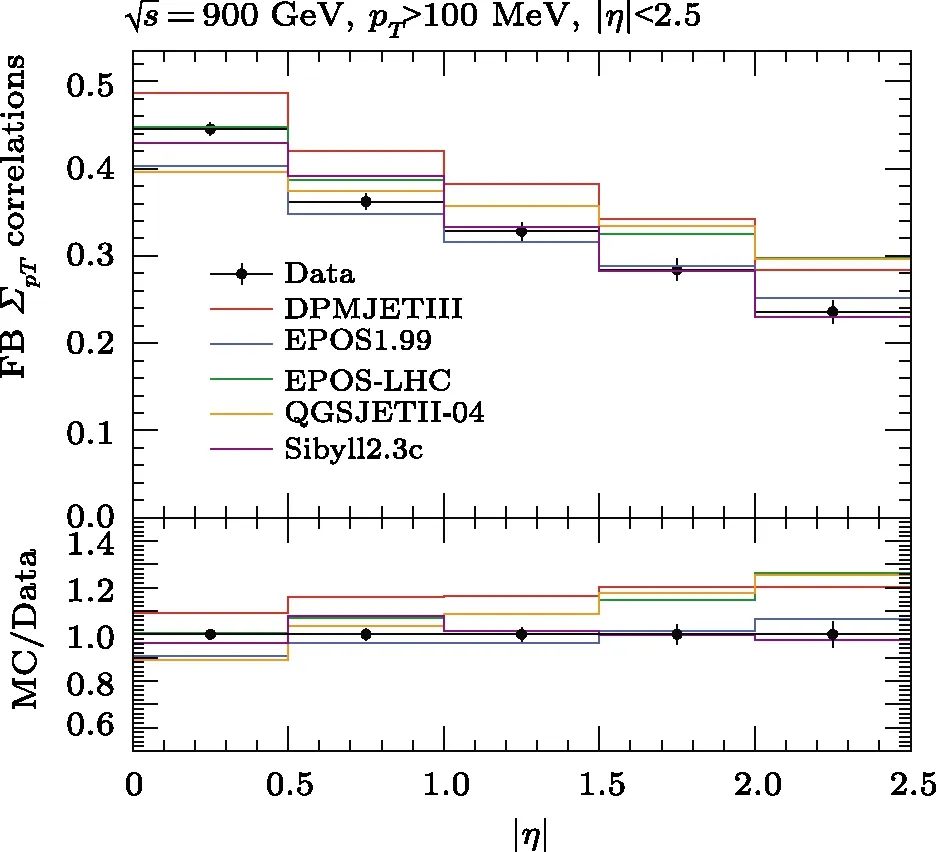
Fig.4 (Color online) The forward-backward correlation in summed transverse momentum (∑pT) in symmetrically opposite η intervals for events with at least two charged particles with | η |< 2.5 and pT ≥100 MeV.Different MC simulations at = 900 GeV,compared with ATLAS experimental data (black symbols).
Similarly,Sybill2.3c depicts the correlations very well forη >1.5 but overestimate for the lower values.The EPOS1.99 gives the consistency and a good agreement to the correlations compared to the other models.The difference between the data and the model predictions of multiplicity correlations are considerably low,for the large pseudorapidity bins.
Figure 4 shows the forward-backward (FB) correlations and the summed transverse momentum (∑pT) of charged particles.The general attributes of these correlations show a similarity to the multiplicity correlations.The Sybill2.3c overestimates the experimental data forη <1.0 but shows a very good match for the higher values ofη.The EPOS1.99 gives a good match with data for 0.5< η <2.0 but underestimates about 10% for the lower values and overestimates for the higher values ofη.All the other models,overestimate the summed transverse momentum correlation for the fullηregion.
6 Conclusions
We have used Monte Carlo models to measure the forward-backward correlation in the summed transverse momentum(∑pT)and,in the multiplicity of charged particles produced inppinteractions atwithpT >100 MeV.For both FB correlations: the FB multiplicity correlation and the FB summed transverse momentum,the EPOS and Sybill2.3c depict the data better compared to the other models.
Two variables,the subtracted () and the sameminus-opposite () have been used to measure the azimuthal distribution (∆ϕ) relative to the leading particle.The results are compared with the ATLAS experimental data for three regions of pseudorapidity:| η |<1.0,|η |<2.0,|η |<2.5 atpT >500 MeV.Both variables reduce the inter-jet effects and give different sensitivities to jet production.In case of,for|η |<1.0 all the models underestimate the peak of the data for the lower values of the azimuthal distributions (∆ϕ) and overestimate as the ∆ϕincreases.While for| η |<2.0 and| η |<2.5 all the models match the peak at the lower values of the ∆ϕand overestimate for the higher ∆ϕ.
For,EPOS,Sibyll2.3c,and DPMJETII show a good agreement to the data for all the values of|η |.Similarly,for thethe second peak is suppressed due to subtraction.In this case,the QGSJETII-04 and Sybill2.3c exaggerate the peak compared to other models.Again,for theas well,we get a very good agreement of the data with the EPOS and Sibyll2.3c.
We have observed discriminating results from different models compared to the experimental data.If one could recommend a single model for the FB correlations and azimuthal distribution,it would be the EPOS model.The EPOS model gives a good agreement to the data for both the quantities studied in this paper.The shortcomings of different models need to be accounted if one is going to reproduce the data at the LHC energies.
 Communications in Theoretical Physics2019年10期
Communications in Theoretical Physics2019年10期
- Communications in Theoretical Physics的其它文章
- Superstatistics of Modified Rosen-Morse Potential with Dirac Delta and Uniform Distributions
- Design of Tunable Devices at Terahertz Frequencies Based on Quasi-Photonic Crystals Incorporated with Graphene
- Coupling Instability of a Warm Relativistic Electron Beam with Ion-Channel Guiding
- Modeling of Neurodegenerative Diseases Using Discrete Chaotic Systems
- Gravitational Deflection of Massive Particles by a Schwarzschild Black Hole in Radiation Gauge∗
- Magnetic Properties of an Antiferromagnetic Spin-1/2 XYZ Model in the Presence of Different Magnetic Fields: Finite-Size Effects of Inhomogeneity Property∗
