Dispersive Solitary Wave Solutions of Strain Wave Dynamical Model and Its Stability

Muhammad Arshad,Aly R.Seadawy, Dian-Chen Lu, and Asghar Ali,3

1Faculty of Science,Jiangsu University,Zhenjiang 212013,China

2Mathematics Department,Faculty of Science,Taibah University,Al-Madinah Al-Munawarah,Saudi Arabia

3Department of Mathematics,University of Education,Multan Campus,Pakistan

Abstract In the materials of micro-structured,the propagation of wave modeling should take into account the scale of various microstructures.The different kinds solitary wave solutions of strain wave dynamical model are derived via utilizing exp(−ϕ(ξ))-expansion and extended simple equation methods.This dynamical equation plays a key role in engineering and mathematical physics.Solutions obtained in this work include periodic solitary waves,Kink and anti-Kink solitary waves,bell-shaped solutions,solitons,and rational solutions.These exact solutions help researchers for knowing the physical phenomena of this wave equation.The stability of this dynamical model is examined via standard linear stability analysis,which authenticate that the model is stable and their solutions are exact.Graphs are depicted for knowing the movements of some solutions.The results show that the current methods,by the assist of symbolic calculation,give an effectual and direct mathematical tools for resolving the nonlinear problems in applied sciences.

Key words: exp(−ϕ(ξ))-expansion method,improved simple equation method,strain wave equation,solitary waves,periodic solutions

1 Introduction

In the past two decades,the search for solutions of diverse types of nonlinear partial differential equations(NLPDEs) have attracted the attention of many scientists.NLPDEs are often utilized for describing complex nonlinear models in the fields of plasma physics,optical fibers,biology,chemistry,and other non-linear sciences.NLPDEs are broadly utilized in applied mathematics,so it is necessary to find different solutions for different types of NLPDEs.Various scholars have designed different effective methods,such as power system method,modified and extended simple equation techniques,expansion methods,decomposition method,reduced differential transform method,direct algebraic technique,homotopy analysis technique,Lie-group symmetry technique,F-expansion technique,mapping technique,first integral scheme,theta and tanh function methods,inverse scattering scheme,extended tanh-function scheme,homogeneous equilibrium and Jacobi elliptic function methods and so on.[1−22]

NLPDEs also describe the mathematical model of wave dissemination in solids of micro-structured,which should take into account numerous scales of microstructures.If scale dependence engrosses dispersion effect and material is nonlinear,dispersion and nonlinear effects may be balanced,leading to solitary waves.In addition to mathematical and physical model equations,the existence and occurrence of solitary waves in complex physical problems must be analyzed accurately enough.Microstructure materials such as alloys,grains,ceramics and functionally gradient materials have been widely used.The modeling of waves dissemination in this material should take into account different micro-structural scales.[1−3,23]In addition to mathematical and physical model equations,the occurrence of waves in complex physical models should be sufficiently correct.Recently,this turns into much gorgeous for obtaining the analytical solutions of NLPDEs via computer algebra,which is helpful for complex and tedious algebraic computation.In mathematical physics,the approximate and exact solutions of NLPDEs,especially the estimation of traveling wave solutions,play a significant part in the theory of solitons.[24]In microstructured solids,there are numerous studies anywhere the governing models of waves have been developed and exact solutions in waves form are scrutinized.[25−26]The modeling in mathematical form of engineering and physics problems are inherent via NLPDEs,so it is very significant for studying the analytical solutions of NLPDEs.Analytical solutions of such equations provide knowledge concerning the structures of these models.Some analytical methods to solve nonlinear equations are mentioned in the introduction.However,a key method called Lie algebra method is missing.Three seminal works are: a Lie algebra approach to susceptible-infected-susceptible epidemics,analytical solution for an in-host viral infection model with time-inhomogeneous rates,Lie algebraic discussion for affinity based information diffusion in social networks.[31−37]

In this paper,we stanched our exertion in constructing novel solitary wave solutions for strain wave model in solids of micro-structured[29]via employing the influential exp(−ϕ(ξ))-expansion[27]and improved simple equation methods.[28]Some researchers have been utilized other techniques for obtaining the traveling wave solutions of this model,for example,generalizedG′/G-expansion,[26]functional variable and Kudryashov technique.[30]The governing micro strain wave model[26,29−30]in the solids of micro-structured has the form as

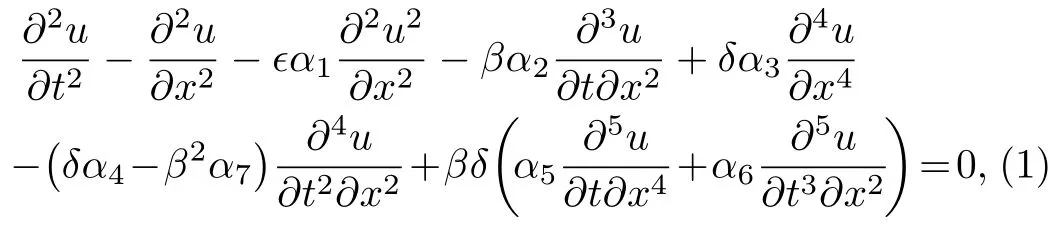
in above model,ϵandδrepresent the elastic strains and ratio among wave length and size of micro-structure,βillustrates the dissipation influence andαi(i=0,1,2,3,...,7) are nonzero real numbers.

The aspire of this paper is to construct the new solitary wave solutions of strain wave model (1) for describing the dissemination of waves in solids of microstructured through modified improved simple equation and exp(−ϕ(ξ))-expansion methods.These solutions have key applications in solids of micro-structured and other areas of sciences.

2 Formation of Solitary Wave Solutions

In this segment,we construct the solitary wave solutions of model (1) in micro-structured solids.

2.1 Exp(−ϕ(ξ))-expansion Method

The traveling wave solutions of Eq.(1) takes the form

whereϕgratifies the below equation

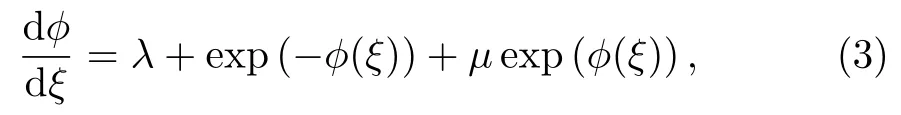
in above equations,theBj,k,µandλare real constants,ωis speed of wave andNis positive integer.The equation(1) reduces into ODE by using Eq.(2) as

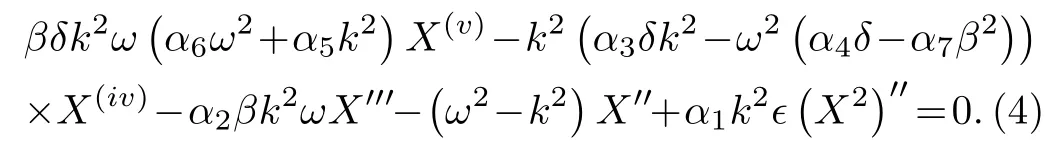
Integrating Eq.(4) twice,we got

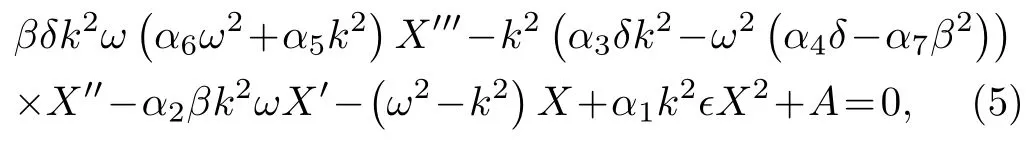
whereAis constant of integration.On Eq.(4),we gotN=3 by using homogeneous balance principle and the solution of Eq.(4) can be written as

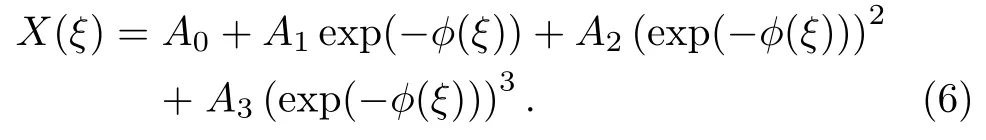
The system of equations inB0,B1,B2,B −3,µ,λ,k,ωare obtained by substitute equations Eqs.(6) and (3) into Eq.(5) and taking the coefficients of exp(−ϕ(ζ))lto zero.The solution set is obtained by solving this system of equations as

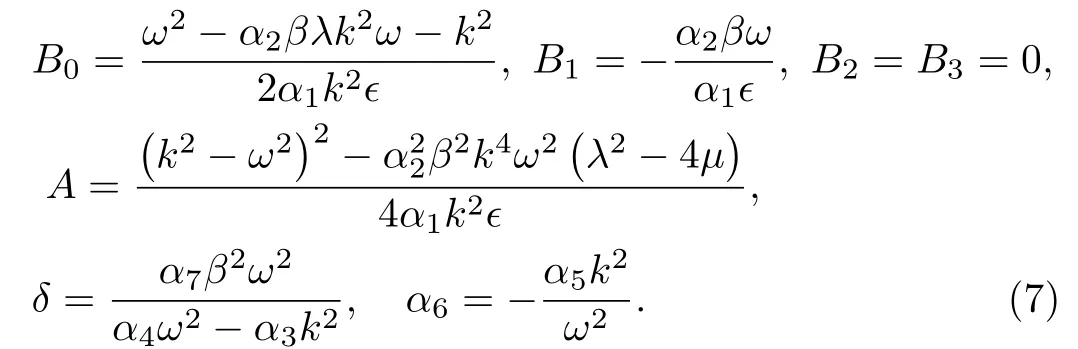
The following types solitary waves of Eq.(1)from solution(7) are constructed as

Type I:Forµ̸=0 andλ2−4µ>0,

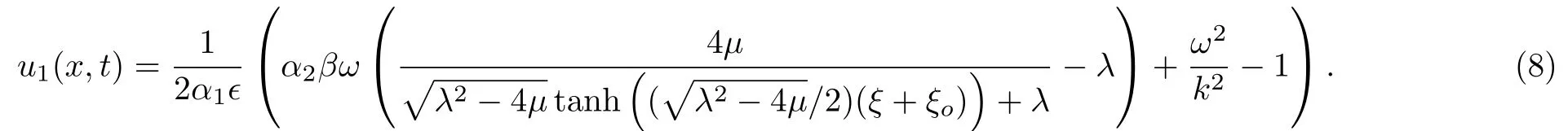
Type II:Forµ̸=0 andλ2−4µ<0,

Type III:Forµ=0,λ ̸=0,andλ2−4µ>0,

Type IV:Forµ̸=0,λ ̸=0,andλ2−4µ=0,

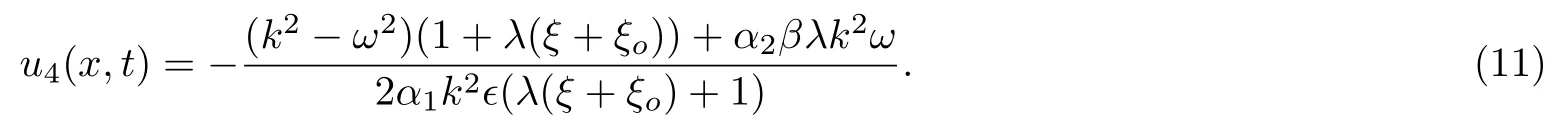
Type V:Forµ=0,λ=0,andλ2−4µ=0,

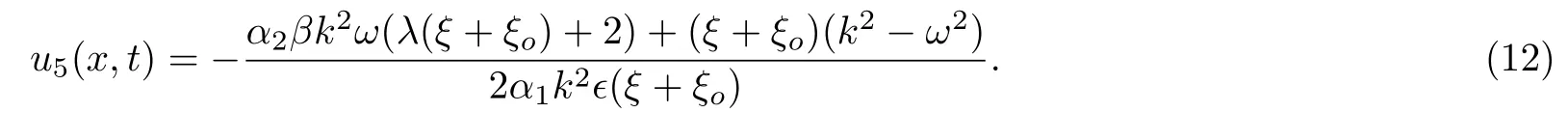
2.2 Improved Simple Equation Method

Supposing the traveling wave solutions of Eq.(1) has as

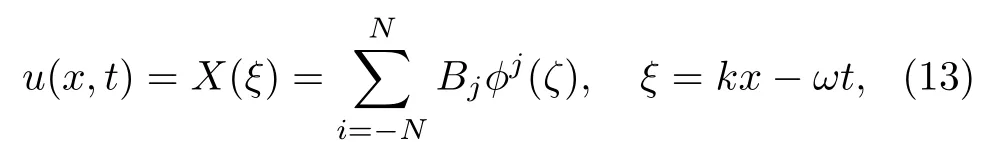
whereϕsatisfies the following equation

in above equations,theBj,k,ρi(i=0,1,2,3) andλare real constants,ωis speed of wave andNis positive integer.Equation (1) reduces into ODE by using Eq.(13)as

Integrating Eq.(15) twice,we got

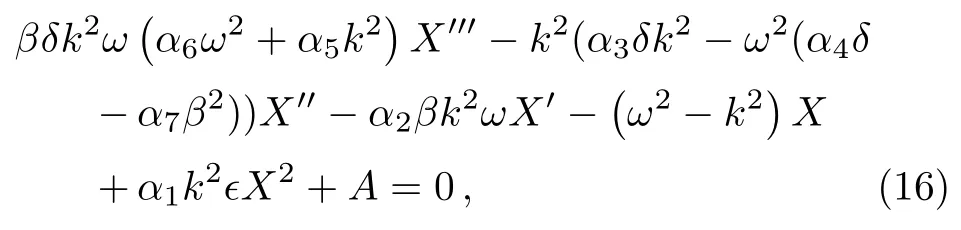
whereAis integration constant.We gotN=3 by using homogeneous balance principle on Eq.(16) and the solution of Eq.(16) has as

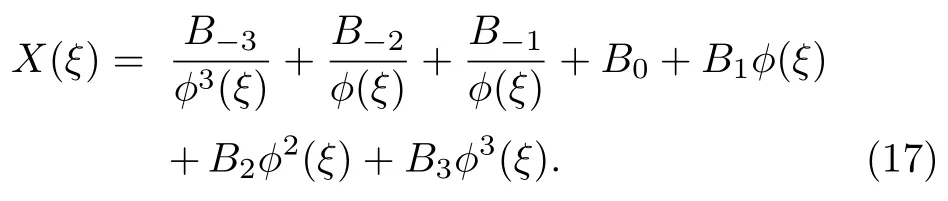
The system of equations inBj(j=−3,−2,...,3),ρj(j=0,1,2,3),k,ωare obtained by substitute Eq.(17)and(14)into Eq.(16)and taking the coefficients of(ϕ(ξ))lto zero.The solutions families are obtained via resolving this system as

First FamilyIn this family,we consider theρ0=ρ2=0,

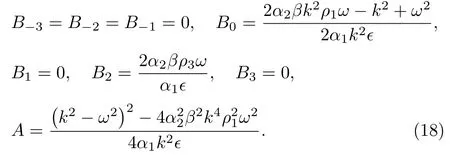
Solitary wave solutions of Eq.(1) from solution (18) are attained as

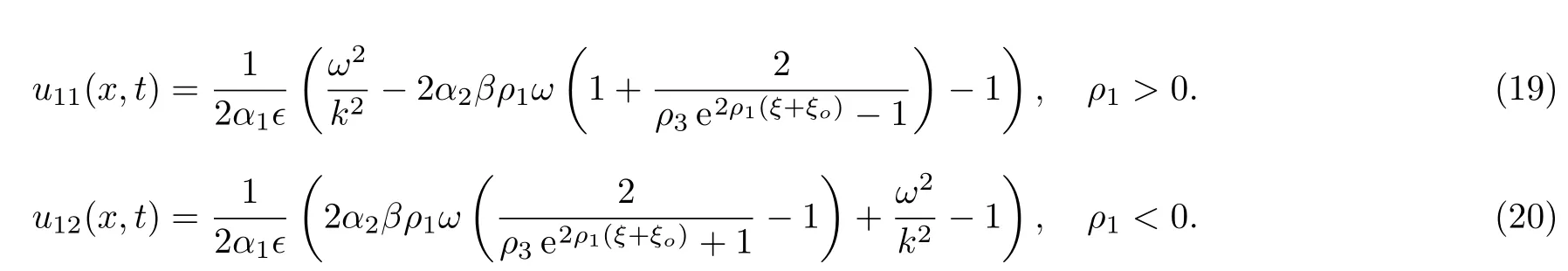
Second FamilyIn this family,we considerρ1=ρ3=0

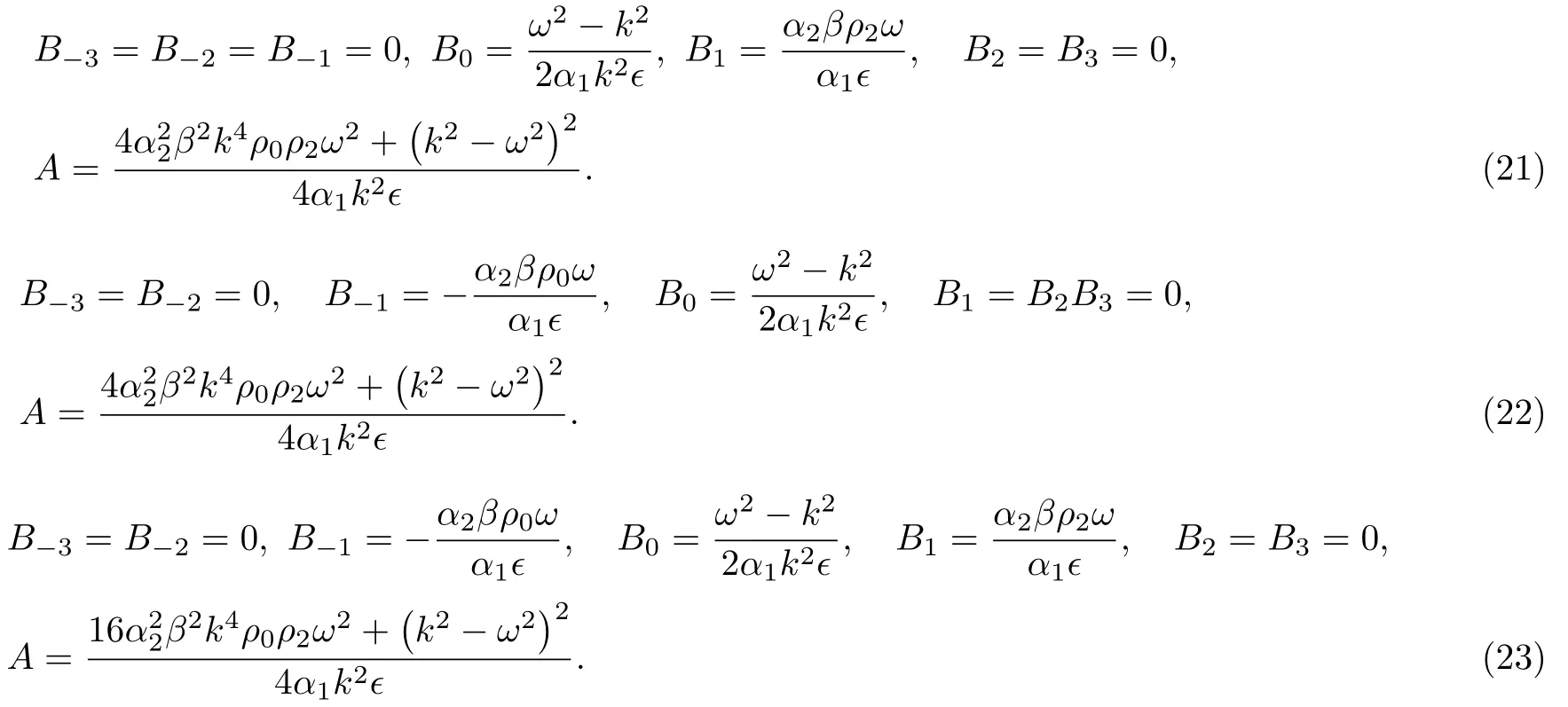
From solutions (21) and (22),the exact solutions of Eq.(1) are attained as

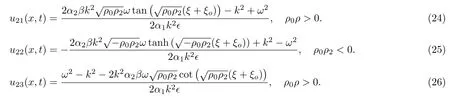
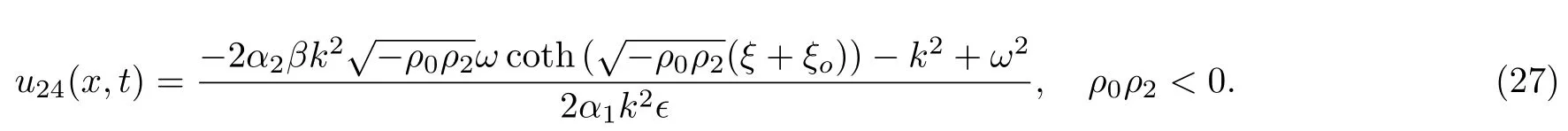
More general solitary wave solutions can be attained of Eq.(1) from solution (23).

Third FamilyIn this family,we considerρ0=ρ3=0,

The solitary wave solutions of (1) from solution (28) are obtained as

Fourth Familyρ3=0,

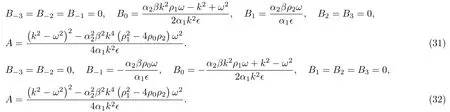
The solitary wave solutions from solutions sets (31) and (32) of Eq.(1) are obtained as

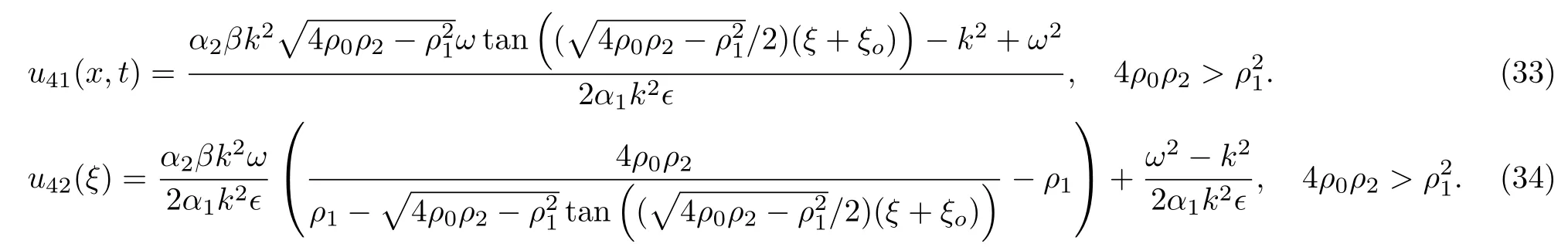
3 Modulation Instability

Several nonlinear equations showing an instability that directs to modulation of the study state as an outcome of interface among the effects of dispersive and nonlinear.The linear stability analysis[13,23]is used to gain the modulation instability of strain wave model.The study state solution of this model has as

in above,optical powerPis normalized.We check progression of the perturbationsψ(x,t) by utilizing the analysis linear stability.Substituting Eq.(35) into Eq.(1) and linearizing it,we have

Assume the solution of Eq.(36) in the form as

in above,thekandωare wave number and frequency in normalized form of perturbations.Using Eq.(37) into Eq.(36),we attain the dispersion relation (DR)ω(k),which is defined for allkand this confirm that steady state is stable stable against wave numbers perturbations.The graph of DR is depicted in Fig.4.

4 Results Discussion and Physical Explanation of Solutions

Fig.1 Dissimilar shapes of wave solutions (8) and (9) are plotted.

In this work,we constructed solitary wave solutions of strain wave dynamical model via two analytical techniques,namely,exp(ϕ(ξ))-expansion and improved simple equation methods.The authors in Refs.[19−20,26,29]constructed the exact solutions of Eq.(1) in special case atβ=0.Therefore,the constructed solutions are novel and not existed in literature.In Fig.1: the solutions (9) and (10) represent anti-kink solitary wave (Fig.1(a)) and periodic solitary wave (Fig.1(d)) by giving particular values to parameters,their one-dimensional and contour plots are depicted in Fig.1(b),Fig.1(c),and Fig.1(e),Fig.1(f) at same values of parameters.In Fig.2,the solutions (19) and (20) represent bright-dark solitary wave(Fig.2(a)and anti-kink soliton(Fig.2(d))through granting special values to parameters,and their one dimensionals and contour plots are depicted in Fig.2(b),Fig.2(c),and Fig.2(e),Fig.2(f) at same values of parameters.In Fig.3,the solutions(25)and(27)signify dark-bright solitary wave((Fig.4(a))and kink soliton(Fig.4(d))through granting special values to parameters,and their one dimensionals and contour plots are depicted in Fig.4(b),Fig.4(c),and Fig.4(e),Fig.4(f) at same values of parameters.In Fig.4,the graph of dispersion relation is shown.

Fig.2 Dissimilar shapes of first family solutions are plotted.

Fig.3 Dissimilar shapes of first family solutions are plotted.

Fig.4 The DR ω = ω(k) among k and ω of perturbations is shown.

5 Conclusion

In this article,the exp(−ϕ(ξ))-expansion and extended simple equation methods have successfully employed and solitary waves solutions in diverse types of strained wave dynamical are obtained.These approaches produce the new solutions that we think other researchers have missed.The constructed waves solutions are helpful to researchers and have important applications in mathematical physics,engineering etc.The kinds of solitary waves vary with the values of arbitrary parameters.The stability of this dynamical model is examined via standard linear stability analysis,which authenticate that the model is stable and their solutions are exact.Graphs are depicted for knowing the movements of some solutions.This paper shows that these are sufficient to find more new exact results of NLPDEs in engineering and mathematical physics.The solution can be used for further analysis of different cases by physicists or engineers.