施密特正交化的几何意义
杜美华 林鑫 马云峰
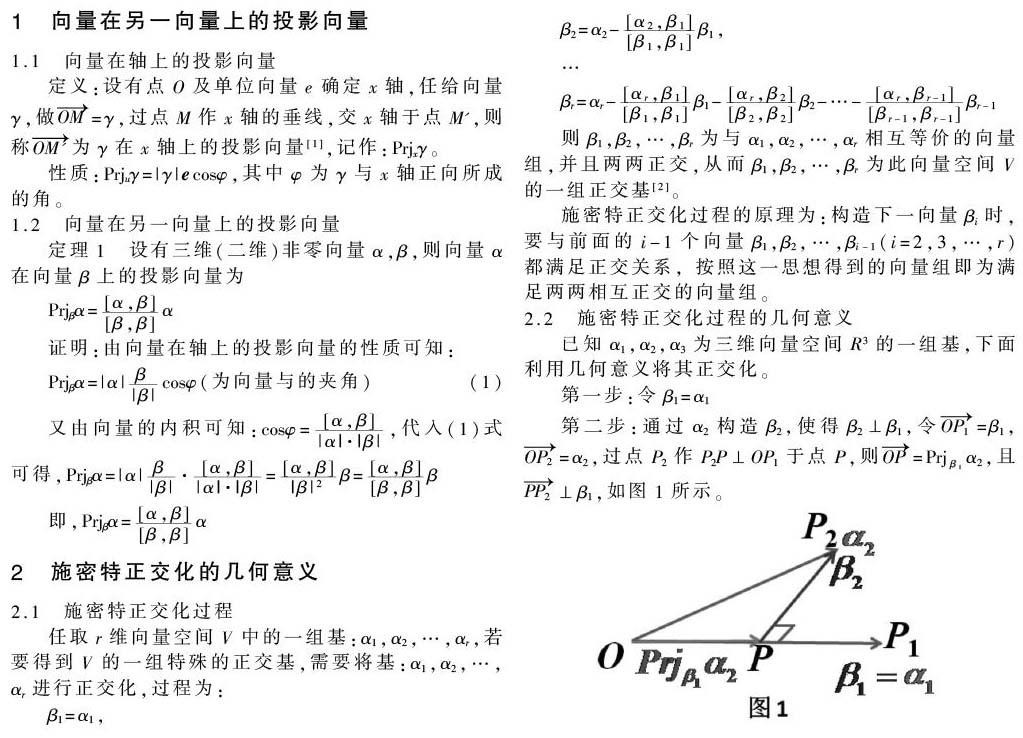

【摘 要】施密特正交化是将一个向量组正交化的重要方法,是求向量空间的规范正交基的一个重要步骤。本文利用向量在另一向量上的投影向量,给出三维空间中施密特正交化的几何意义,并且将此结论推广到一般的维向量的正交化过程。
【关键词】施密特正交化;几何意义;投影向量
中图分类号: O13 文献标识码: A 文章编号: 2095-2457(2019)23-0061-002
DOI:10.19694/j.cnki.issn2095-2457.2019.23.026
【Abstract】Schmidt orthogonalization is an important method to orthogonalize a vector group and an important step to find the standard orthogonal basis of vector space. In this paper, the geometric meaning of Schmidt orthogonalization in three-dimensional space is given by using the projection vectors of vectors on another vector, and the conclusion is extended to the orthogonalization process of general dimensional vectors.
【Key words】Schmidt orthogonalization; Geometric meaning; Projection vector
1 向量在另一向量上的投影向量
1.1 向量在軸上的投影向量
利用几何意义,可以加深学者对施密特正交化过程的理解,同时提高对n维向量空间中向量关系的理解与掌握,降低线性代数的抽象性,增强对抽象线性代数知识的想象力。
【参考文献】
[1]同济大学数学系.高等数学(第七版下册)[M].北京:高等教育出版社,2014:12-13.
[2]吴赣昌.线性代数(理工类简明版第五版)[M].北京:中国人民大学出版社,2017:133-135.

