Study on Practical Mature Age of Individual Pinus thunbergii×P. densiflora

Zhimin CHEN Guoyun YANG Fuming QIAN Xicui WANG Kejia YANG Decui SHU

Abstract This study was conducted on the analytic tree and got the fitting empirical equation of tree growth, in which the tree increment was used as the variable and time as the independent variable. The arithmetical operation to the function gave the mature age of tree growth, and the practical mature age of Pinus thunbergii×P. densiflora was 41 a. In addition, the application as well as the research direction and matters needing attention were proposed.

Key words Mature age; Empirical equation; Analytic tree

Received: July 23, 2019Accepted: November 2, 2019

Zhimin CHEN (1968-), male, P. R. China, researcher, devoted to research about forestry design, resource survey, forestry technology promotion.

*Corresponding author. Email: 344134145@qq.com.

Since the 1980s, researchers from Yantai Forestry Institute have discovered the natural hybrid of Pinus thunbergii and P. densiflora—P. thunbergii×P. densiflora in a valley. Studies have shown that P. thunbergii×P. densiflora grows faster than P. thunbergii and P. densiflora, and has high promotion value. Since 1996, Yantai Forestry Institute has carried out artificial cross breeding for P. thunbergii×P. densiflora. In the past ten years, they have overcome many difficulties and finally revealed the high growth law and resistance to pests and diseases in P. thunbergii×P. densiflora. The planting trials showed that P. thunbergii×P. densiflora is a fine variety with high photosynthetic efficiency and high water use efficiency, and has the characteristics of straight trunk, fast growth, good drought resistance and good tolerance to salt and alkali. Under the same planting conditions, P. thunbergii×P. densiflora is 1/3 higher than its parent P. thunbergii. Meanwhile, P. thunbergii×P. densiflora is highly resistant to Dendrolimus spectabilis, Matsucoccus iaponicus, and Diploelia pinea. It is a pioneer species that can replace P. thunbergii and P. densiflora for construction of coastal defense forests and afforestation in mountainous areas. Some varieties, which are beautiful in tree shape and vigorous through the winter, are very suitable for urban greening needs. However, in the practice of forestry production, it is necessary to urgently solve a technical problem, that is, the problem of mature age of P. thunbergii×P. densiflora. We started with the analysis of analytic tree data to study the mature age of P. thunbergii×P. densiflora.

Source of Information

The analytical tree material was a 40yearold P. thunbergii×P. densiflora tree in midDecember 2009 from Dayao Town, Muping District, Yantai City. The DBH was studied using the section of 2.6 m, and others using the 2 m section. Round disks were cut at the tree height of 5 cm (0 disk), 1.3, 3.6, 5.6, 7.6, 9.6 and 10.6 m, respectively. Interpretation was made strictly according to the technology for analytic tree, and relevant data were collected (process omitted).

Preliminary Study on the Mature Age of the Tree

Through the data analysis of the analytical tree material, the current annual increment and mean annual increment of the ground diameter, the DBH, the DBH square, the tree height and the volume were calculated. And by interpolation, the year with the largest current annual increment tz (the meaning and unit is the same below) and the year with the with largest mean annual increment tm (the meaning and unit is the same below) were obtained, and tm is the age of quantitative maturity. The high growth of trees is greatly affected by natural factors, and may be harmed by wind and snow. Therefore, we took the years with slightly decreased growth rate as the tz and tm of high growth, and the calculated tz and tm of each index are shown in the table. It can also be seen from the table that the DBH growth and the volume growth showed tm≥38 a, which was uncertain. According to the results of other two P. densiflora trees at the same place, tm=2tz, the mature age was determined as 46 and 56 a, respectively, and 46 a (closer to the DBH square tm≥38 a) was given higher reliability. Therefore, the actual mature age of P. thunbergii×P. densiflora was initially determined at 46 a, and 56 a was determined as the ideal mature age of the tree. Next, the mature age was determined by the empirical equation fitting method for comparison and analysis.

Empirical Equation Fitting Research Method

In order to save research costs, we started with the analysis of analytical tree data, and carried out fitting tests on various regression equations, using the research methods introduced in references［1-3］, and referring to the research results of reference［4］ and the research methods and processes of references［5-6］, with the help of tree growth empirical equations. Finally, following mixed empirical equation was selected to study the growth process of trees: Y(t)=ea-b/t (wherein a, b are the index parameters of the function to be solved, and e is the base of natural logarithm, 2.718 28……). Tree growth is affected by a variety of factors, but those with the largest influence on P. densiflora are the precipitation amount and the spatial and temporal distribution. In this study, we tried to use the empirical equations to fit the growth process of trees. The fitting equation of the maximum age of DBH (including the equation from the derivation, which was stated in the research process) could get the age of quantitative maturity of DBH. The fitting equation of the maximum age of tree height could get the mature age of tree height. The same method was used to get the ages of quantitative maturity of DBH, DBH square, wood volume and growth.

Empirical Equation Fitting Research Process

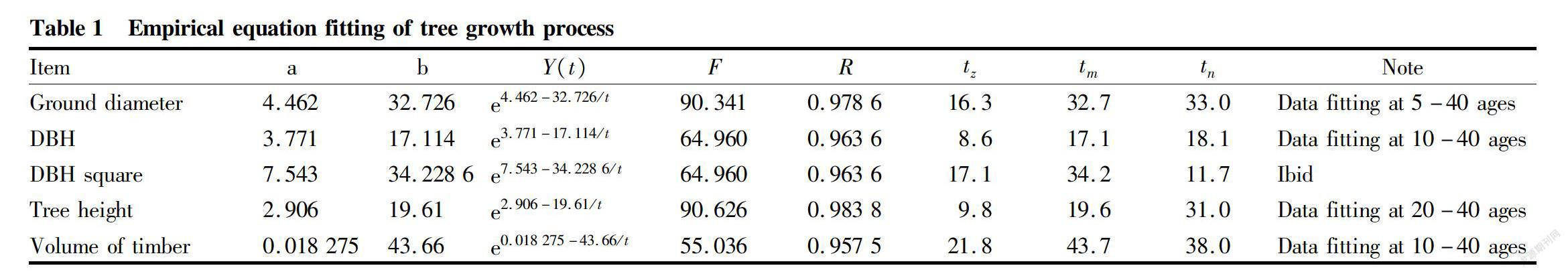
A linear equation was formed by taking the logarithm of the equation for tree growth process, and then, the unary linear regression was used to get the values of parameters a, b, which were tested by F test and correlation coefficient R test. Passing the test means that the equation of tree growth is established. The calculation results were shown in Table 1. By looking up the table, except wood volume, the fitting results of other items all passed the F test with the reliability of over 90%. The F test showed that the mathematical model (the empirical fitting equation) was applicable overall, and all items passed the correlation coefficient R test with the reliability of 99.9%, indicating that the relationship with the fitting equation was set up. Therefore, the item of ground diameter was described as an example to illustrate the problems of using the fitting equation to solve the time for maximum current annual increment and quantitative maturity of trees. The extreme point of the equation for the ground diameter growth speed of the tree (current annual increment, which was completed through the derivation of the Y(t) function in the table, and the process was omitted with only the extreme point given, the same below) was tz=16.3 a, which meant that the growth amount of the tree reached the peak at about the 16th year, and there was only a single peak. The extreme point of the equation for the average growth speed of the tree (mean annual increment, which was completed through the derivation of the Y (t)/t function, and the process was omitted with only the extreme point given, the same below) was tm=32.7 a, indicating that the mature age of the tree was 32.7 a (only the ground diameter fitting equation was given, and the fitting equation for other items were the same, thus omitted). In following discussion, the equations for growth fitting, tree growth speed and average growth speed of the tree were the same, and the meaning of tz and tm were also the same, so the calculation results were given directly. The mature ages of all the items were shown in Table 1. As shown in Table 1, when comparing the item square with the item, the value of DBH square increased by 1 time compared with DBH, and the accuracy was the same with the tested F value and R value, which was caused by the exponential mathematical relation. In order to compare with the cumulative fitting equation, we established the fitting equation, while the age of the accumulated maturity was close to the average mature ages of the DBH square and DBH, and the mature age of ground diameter was close to that of tree height, which is quite coincident. In Table 1, the value of tn was the age at intersection point between the current annual increment and mean annual increment curves of the sample tree (the volume was obtained from the trend graph of the growth curve), which could serve as the practical mature age of the tree. Only the tm values of tree height and DBH square were far from the tn values, and the remaining tm values were close to corresponding tn values, while fitting with empirical equations better solved this technical problem, and obtained more reliable results. Therefore, according to the research results and the actual practice of production, it would be more reliable to set the age of the accumulated maturity as the mature age of the trees.

Conclusions and Application

According to the analysis and judgment of the research results, with the quantitative maturity of volume as the standard, the fitted value of the volume and the average value of the analytical tree were determined. The practical mature age of P. thunbergii×P. densiflora was determined as 41 years, and the age group was divided as the following: young sampling forest of below 15 years, immature forest of 16-30 years, nearmature forest of 31-40 years, mature forest of 41-60 years, and overmature forest with the ages over 61 years.

The results of this study indicate that the practical age for the final felling (regeneration cutting) for P. thunbergii×P. densiflora forests should be set at 41-60 years, which is completely consistent with the original P. thunbergii and P. densiflora (plantation) standards, indicating that although the growth is faster, the mature age does not change. This has a very positive and realistic significance for alleviating the contradiction of wood shortage and strengthening the carbon efficiency of forests.

Discussion

The original mature age is 101 a (natural forests). It seems that P. thunbergii×P. densiflora is not only highyielding, but al

so fastgrowing, and is an ideal afforestation tree species today. In this study, due to the difficulty in collecting tree samples and the limited funds, the lack of age of the analytical tree was compensated with the help of the empirical equations of tree growth, which also avoided the differences in time and space due to various natural conditions and the noise effect of tree differentiation on the test results, and the mature age of the obtained P. thunbergii×P. densiflora forest was confirmed, analyzed and judged repeatedly. The empirical equations are applicable for tree growth, but it is difficult to make a scientific explanation. Limited by various conditions, all kinds of deviations can hardly be avoided, which can only be improved and developed in the research and production practice. However, the suggestions for forest production in this paper were made on the analysis and fitting of individual analytical tree, and were only personal opinions which required the approval and tests from the experts to put into application.

References

［1］ Kuijian Lang. Forest measurement［M］. Beijing: China Forestry Publishing House, 1985. (in Chinese)

［2］ Huahao Chen. Mathematical statistics［M］. Beijing: China Forestry Publishing House, 1985. (in Chinese)

［3］ LIU GJ. Review guide for vocational qualification test of registered consulting engineer (investment)［M］. Tianjin: Tianjin University Press, 2003. (in Chinese)

［4］ GAO JH. Approach into desirable period of forest management in Shandong Province［J］. Journal of Forestry Engineering, 2003, 3: 6-8. (in Chinese)

［5］ HU HY. Study on the actual maturity age of individual Pinus densiflora［J］. Journal of Shandong Forestry Science and Technology, 2010, 6: 36-37. (in Chinese)

［6］ LI LP. Study on expected maturity age of Shandong Pinus densiflora［J］. Journal of Anhui Agricultural Sciences, 2017, 3: 184-186. (in Chinese)